14 marks
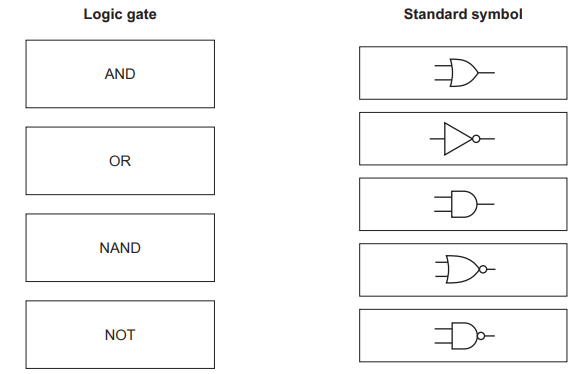
Four logic gates and five standard symbols for logic gates are shown
Draw one line to link each logic gate to its standard symbol. Not all standard symbols will be used.
Did this page help you?
Boolean Logic (Cambridge (CIE) IGCSE Computer Science)
Exam Questions
59 mins9 questions2a2 marks
Complete the truth table in Fig. 1 for the Boolean statement P = NOT(A AND B).
A | B | P |
|---|---|---|
0 | 0 | 1 |
0 | 1 | |
1 | 0 | |
1 | 1 | 0 |
Fig. 1
2b2 marks
Complete the truth table for the following logic gate.
A | B | Q |
|---|---|---|
0 | 0 | 0 |
0 | 1 | 1 |
0 | ||
1 |
Did this page help you?
1a4 marks
Consider this logic expression.
X = ( A AND B ) OR ( B AND NOT C )
Draw a logic circuit for this logic expression.
Each logic gate must have a maximum of two inputs.
Do not simplify this logic expression.
1b4 marks
Complete the truth table from the given logic expression.
A | B | C | Working space | X |
|---|---|---|---|---|
0 | 0 | 0 | ||
0 | 0 | 1 | ||
0 | 1 | 0 | ||
0 | 1 | 1 | ||
1 | 0 | 0 | ||
1 | 0 | 1 | ||
1 | 1 | 0 | ||
1 | 1 | 1 |
Did this page help you?
23 marks
Write the Boolean expression represented by the logic diagram below:
Did this page help you?
1a6 marks
Consider the truth table:
A | B | C | X |
|---|---|---|---|
0 | 0 | 0 | 0 |
0 | 0 | 1 | 0 |
0 | 1 | 0 | 0 |
0 | 1 | 1 | 0 |
1 | 0 | 0 | 1 |
1 | 0 | 1 | 0 |
1 | 1 | 0 | 0 |
1 | 1 | 1 | 1 |
Draw a logic circuit to represent the given truth table.
Each logic gate should have maximum of two inputs.
Do not simplify the logic circuit.
1b3 marks
Write a logic expression for the given truth table.
Do not simplify the logic expression.
Did this page help you?
2a4 marks
Consider this logic expression.
Z = (NOT A OR B) AND (B XOR C)
Draw a logic circuit for this logic expression.
Each logic gate must have a maximum of two inputs.
Do not simplify this logic expression.
2b4 marks
Complete the truth table from the given logic expression.
A | B | C | Working space | X |
|---|---|---|---|---|
0 | 0 | 0 | ||
0 | 0 | 1 | ||
0 | 1 | 0 | ||
0 | 1 | 1 | ||
1 | 0 | 0 | ||
1 | 0 | 1 | ||
1 | 1 | 0 | ||
1 | 1 | 1 |
Did this page help you?
34 marks
Consider this logic expression.
X = (A OR B) AND (NOT B AND C)
Complete the truth table for this logic expression.
A | B | C | Working space | X |
|---|---|---|---|---|
0 | 0 | 0 | ||
0 | 0 | 1 | ||
0 | 1 | 0 | ||
0 | 1 | 1 | ||
1 | 0 | 0 | ||
1 | 0 | 1 | ||
1 | 1 | 0 | ||
1 | 1 | 1 |
Did this page help you?
4a5 marks
Consider the following logic statement:
X = ((A OR B) AND (NOT (B XOR C)) AND C)
Draw a logic circuit to represent the given logic statement.
Do not attempt to simplify the logic statement. All logic gates must have a maximum of two inputs.
4b4 marks
Complete the truth table for the given logic statement.
A | B | C | Working space | X |
|---|---|---|---|---|
0 | 0 | 0 | ||
0 | 0 | 1 | ||
0 | 1 | 0 | ||
0 | 1 | 1 | ||
1 | 0 | 0 | ||
1 | 0 | 1 | ||
1 | 1 | 0 | ||
1 | 1 | 1 |
Did this page help you?
5a6 marks
Consider the following logic statement:
X = (((A AND NOT B) OR (NOT (B NOR C))) AND C)
Draw a logic circuit to represent the given logic statement.
Do not attempt to simplify the logic statement. All logic gates must have a maximum of two inputs.
5b4 marks
Complete the truth table for the given logic statement.
A | B | C | Working space | X |
|---|---|---|---|---|
0 | 0 | 0 | ||
0 | 0 | 1 | ||
0 | 1 | 0 | ||
0 | 1 | 1 | ||
1 | 0 | 0 | ||
1 | 0 | 1 | ||
1 | 1 | 0 | ||
1 | 1 | 1 |
Did this page help you?