Applications of Differentiation (CIE IGCSE Additional Maths)

Revision Note

Test yourself

Author

Amber

Last updated

18 October 2023

Finding Gradients

How do I use the derivative to find the gradient of a curve?

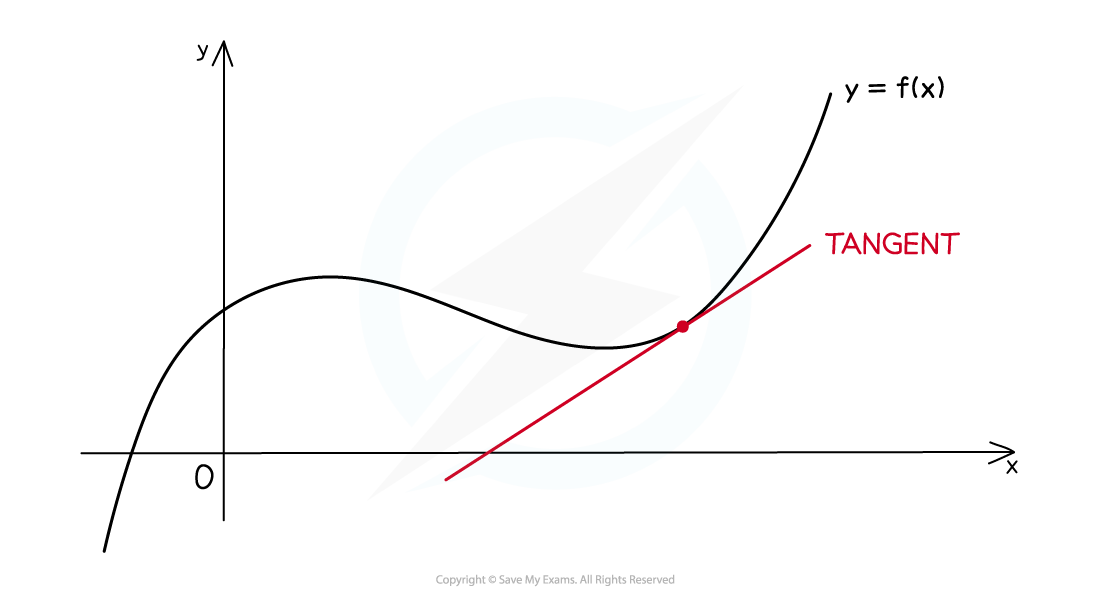
The gradient of a curve at a point is the gradient of the tangent to the curve at that point
To find the gradient of a curve, at any point on the curve
- differentiate to find $\frac{d y}{d x}$ (unless $\frac{d y}{d x}$ is already known)
- substitute the x‑coordinate of the point into the derivative $\frac{d y}{d x}$ and evaluate

Grad Tang Norm Illustr 1, A Level & AS Maths: Pure revision notes

How do I find the approximate change in y as x increases?

$gradient = \frac{change in y}{change in x}$ so, for small changes you can write
- $change in y = gradient \times change in x$
For example, if the gradient of $y = x - \frac{1}{x}$ at $x = 2$ is $\frac{5}{4}$
- what is the approximate change in $y$ as $x$ increases from $2$ to $2 + h$ , where $h$ is small?
  - $change in y = gradient \times change in x = \frac{5}{4} h$

Examiner Tip

Read the question carefully; sometimes you are given $\frac{d y}{d x}$ and so don't need to differentiate initially - don't just automatically differentiate the first thing you see!
The following mean the same thing:
- "Find the gradient of the curve at $x = 2$ "
- "Find the gradient of the tangent at $x = 2$ "
  - the tangent gradient = curve gradient at that point
- "Find the rate of change of y with respect to x at $x = 2$ "

Worked example

A curve has the equation $y = \frac{4}{3} x^{3} + 3 x - 8$ .

(a)

Find the gradient of the curve when $x = 2$ .

$y$ is already in a form that can be differentiated

$\frac{d y}{d x} = 4 x^{2} + 3$

Substitute $x = 2$ into $\frac{d y}{d x}$

$\frac{d y}{d x} = 4 \times 2^{2} + 3 = 19$

The gradient of the curve at $x = 2$ is 19

(b)

Work out the possible values of $x$ for which the rate of change of $y$ with respect to $x$ is 4.

"Rate of change" is another way of describing the derivative

$\begin{array}{rcl} \frac{d y}{d x} & = & 4 \\ 4 x^{2} + 3 & = & 4 \end{array}$

Solve this equation to find $x$
Note that it is quadratic equation so it could have up to two solutions
The question refers to 'values' implying there is (or could be) more than one value for $x$

$\begin{array}{rcl} 4 x^{2} & = & 1 \\ x^{2} & = & \frac{1}{4} \\ x & = & \pm \sqrt{\frac{1}{4}} \\ x & = & \pm \frac{1}{2} \end{array}$

The possible values of $x$ , that give a rate of change of 4, are $x = \frac{1}{2}$ and $x = - \frac{1}{2}$

Did this video help you?

Increasing & Decreasing Functions

What are increasing and decreasing functions?

A function is increasing when $\frac{d y}{d x} > 0$ (the gradient is positive)
- This means graph of a function goes up as $x$ increases
A function is decreasing when $\frac{d y}{d x} < 0$ (the gradient is negative)
- This means graph of a function goes down as $x$ increases

Incr Decr Illustr 1, A Level & AS Maths: Pure revision notes

How do I find where functions are increasing or decreasing?

To identify the intervals on which a function is increasing or decreasing

STEP 1

Find the derivative f'(x)

STEP 2

Solve the inequalities

$f' (x) > 0$ (for increasing intervals) and/or

$f' (x) < 0$ (for decreasing intervals)

Most functions are a combination of increasing, decreasing and stationary
- a range of values of $x$ (interval) is given where a function satisfies each condition
- e.g. The function $f (x) = x^{2}$ has derivative $f^{'} (x) = 2 x$ so
  - $f (x)$ is decreasing for $x < 0$
  - $f (x)$ is stationary at $x = 0$
  - $f (x)$ is increasing for $x > 0$

To identify the intervals (the range of $x$ values) for which a curve is increasing or decreasing you need to:

1. Find the derivative $\frac{d y}{d x}$
2. Solve the inequalities $\frac{d y}{d x} > 0$ (for increasing intervals) or $\frac{d y}{d x} < 0$ (for decreasing intervals)

Examiner Tip

In an exam, if you need to show a function is increasing or decreasing you can use either strict (<, >) or non-strict (≤, ≥) inequalities
- You will get the marks either way in this course

Worked example

For what values of $x$ is $y = 2 x^{3} - 3 x^{2} + 5$ a decreasing function?

The function is decreasing when its gradient $(\frac{d y}{d x})$ is less than 0.

Find the derivative of the function by differentiating.

$\begin{array}{rcl} \frac{d y}{d x} & = & 6 x^{2} - 6 x \end{array}$

Solve the inequality $\frac{d y}{d x} < 0$ to find the set of values where the gradient is negative.

$\begin{array}{rcl} 6 x^{2} - 6 x & < & 0 \end{array}$

Factorise.

$\begin{array}{rcl} 6 x (x - 1) & < & 0 \end{array}$

The solutions to $\frac{d y}{d x} = 0$ are $x = 0$ and $x = 1$ . Find the correct way around for the inequalities by considering the graph of $\frac{d y}{d x}$ . The graph is a positive quadratic, so the function is negative between the values of 0 and 1 (where it is below the $x$ -axis).

Considering a sketch of the graph of the gradient function may help you see this.

3CqZzydq_aqa-fm-increasing-and-decreasing-functions-rn-diagram-we-1

You can check your answers by considering a sketch of the original function, it should be decreasing at the point where $0 < x < 1 .$

aqa-fm-increasing-and-decreasing-functions-rn-diagram-we-2

$0 < x < 1$

Did this video help you?

Tangents & Normals

What is a tangent?

At any point on the graph of a (non-linear) function, the tangent is the straight line that touches the graph at a point without crossing through it
Its gradient is given by the derivative function

Grad Tang Norm Illustr 2

How do I find the equation of a tangent?

To find the equation of a straight line, a point and the gradient are needed
The gradient, $m$ , of the tangent to the function $y = f (x)$ at ${(x}_{1}, y_{1})$ is $f' (x_{1})$
- You can find this by differentiating the function, and then substituting the $x$ -coordinate of the point into the derivative
Therefore find the equation of the tangent to the function $y = f (x)$ at the point ${(x}_{1}, y_{1})$ by substituting the gradient, $f' (x_{1})$ , and point ${(x}_{1}, y_{1})$ into $y - y_{1} = m (x - x_{1})$ , giving:
- $y - y_{1} = f^{'} (x_{1}) (x - x_{1})$
(You could also substitute into $y = m x + c$ )

What is a normal?

At any point on the graph of a (non-linear) function, the normal is the straight line that passes through that point and is perpendicular to the tangent

Grad Tang Norm Illustr 3

How do I find the equation of a normal?

The gradients of two perpendicular lines are negative reciprocals
- This means that if $m_{1}$ is the gradient of the first line and $m_{2}$ is the gradient of a line perpendicular to the first line, then $m_{2} = \frac{- 1}{m_{1}}$
- Rearranging the formula above, $m_{1} \times m_{2} = - 1$ is a useful way to test whether two lines are perpendicular
Therefore gradient of the normal to the function $y = f (x)$ at ${(x}_{1}, y_{1})$ is $\frac{- 1}{f' (x_{1})}$
Find the equation of the normal to the function $y = f (x)$ at the point ${(x}_{1}, y_{1})$ by using $y - y_{1} = \frac{- 1}{f^{'} (x_{1})} (x - x_{1})$
- (or $y = \frac{- 1}{f^{'} (x_{1})} x + c$ )

Examiner Tip

To be successful in this topic, first make sure you are confident with finding the equation of a straight line!

Worked example

The function $f (x)$ is defined by

$f (x) = 2 x^{4} + \frac{3}{x^{2}} x \neq 0$

Find an equation for the tangent to the curve

y = f (x)

at the point where

x = 1

, giving your answer in the form

y = m x + c

First find the derivative

f' (x)

by differentiating

Start by rewriting

f (x)

as powers of x

$f (x) = 2 x^{4} + 3 x^{- 2}$

Now differentiate

f' (x) = 8 x^{3} - 6 x^{- 3}

Now substitute

x = 1

into

f' (x)

to find the gradient of the tangent

\begin{array}{rcl} f' (1) & = & 8 {(1)}^{3} - 6 {(1)}^{- 3} \\ = & 8 - 6 \\ = & 2 \end{array}

We also need the y-coordinate, so substitute

x = 1

into

f (x)

also

\begin{array}{rcl} f (1) & = & 2 {(1)}^{4} + 3 {(1)}^{- 2} \\ = & 2 + 3 \\ = & 5 \end{array}

Now we can substitute the point (1, 5) and the gradient, 2, into

y - y_{1} = m (x_{1}) (x - x_{1})

\begin{array}{rcl} y - 5 & = & 2 (x - 1) \end{array}

Note that we are asked for the final answer in the form

y = m x + c

, so rearrange to this form

\begin{array}{rcl} y - 5 & = & 2 x - 2 \end{array}

y = 2 x + 3

Find an equation for the normal at the point where

x = 1

, giving your answer in the form

a x + b y + d = 0

, where

a

b

and

d

are integers.

We already have the gradient of the tangent; the gradient of the normal is

\frac{- 1}{m}

where

m

is the gradient of the tangent

gradient of normal =

\frac{- 1}{2}

Substitute the point (1, 5) from part (a) and the gradient of the normal into

y - y_{1} = m (x - x_{1})

\begin{array}{rcl} y - 5 & = & (\frac{- 1}{2}) (x - 1) \end{array}

And rearrange into the form required

\begin{array}{rcl} y - 5 & = & - \frac{1}{2} x + \frac{1}{2} \\ \frac{1}{2} x + y - 5 \frac{1}{2} & = & 0 \end{array}

Note that

a

b

and

d

must be integers so multiply by 2 to clear the fractions

\begin{array}{rcl} x + 2 y - 11 & = & 0 \end{array}

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Test yourself

Did this page help you?

Previous:Quotient RuleNext:Second Order Derivatives

Applications of Differentiation (CIE IGCSE Additional Maths)

Revision Note

How do I use the derivative to find the gradient of a curve?

How do I find the approximate change in y as x increases?

What are increasing and decreasing functions?

How do I find where functions are increasing or decreasing?

What is a tangent?

How do I find the equation of a tangent?

What is a normal?

How do I find the equation of a normal?

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Amber