GCSEStatisticsEdexcelHigherRevision NotesProcessing, Representing & Analysing DataUsing Summary StatisticsSkewness

Skewness (Edexcel GCSE Statistics)

Revision Note

Roger B

Written by: Roger B

Reviewed by: Dan Finlay

Edexcel Statistics: HigherEdexcel Statistics: Foundation

Skewness Basics

What is skewness?

  • Skewness describes the way in which data in a distribution is 'leaning' 

    • A distribution that has its 'tail' on the right side has positive skew

      • Most of the data values are on the lower end

      • The distribution is stretched out in the positive direction

      • Values above the median have a greater spread than values below the median

    • A distribution that has its tail on the left side has negative skew

      • Most of the data values are on the higher end

      • The distribution is stretched out in the negative direction

      • Values below the median have a greater spread than values above the median

    • A distribution that is evenly spread out to the left and right is symmetrical

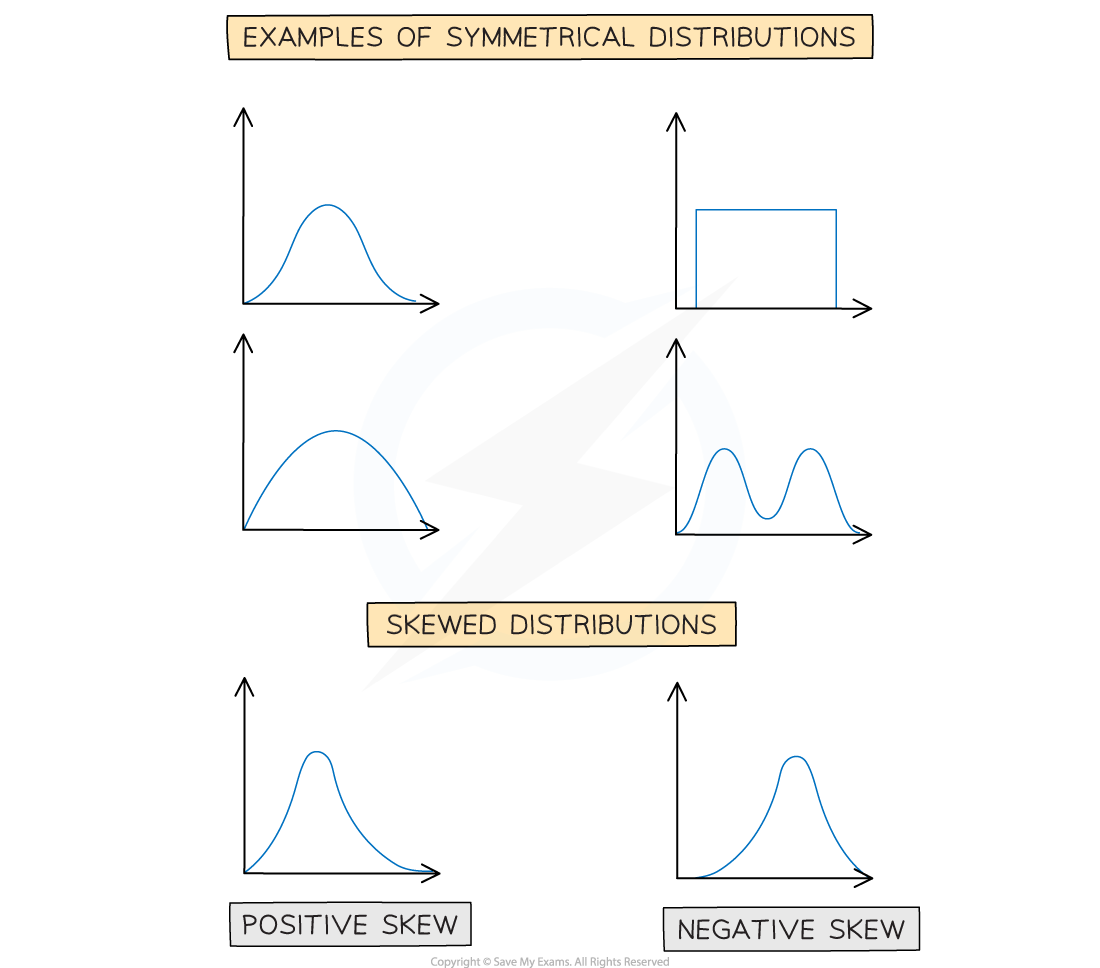
2-3-3-skewness-diagram-1

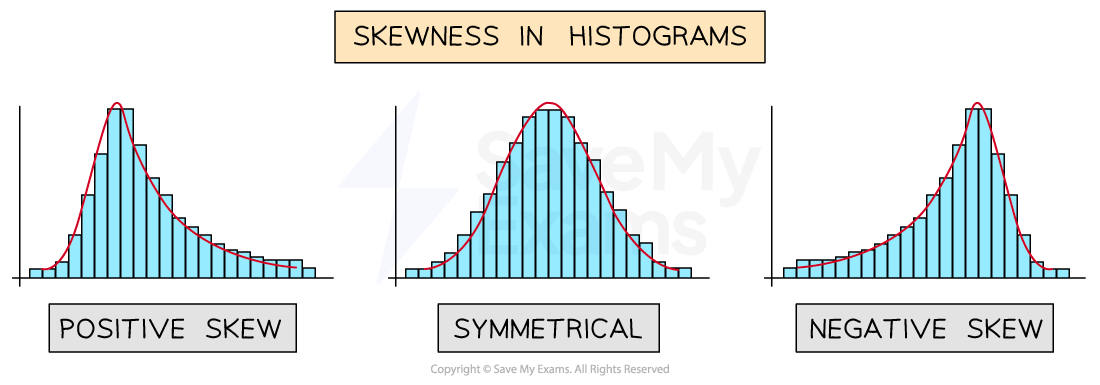
  • Skewness can be spotted quite easily in histograms

Examples of histograms showing positive skew, a symmetrical distribution, and negative skew

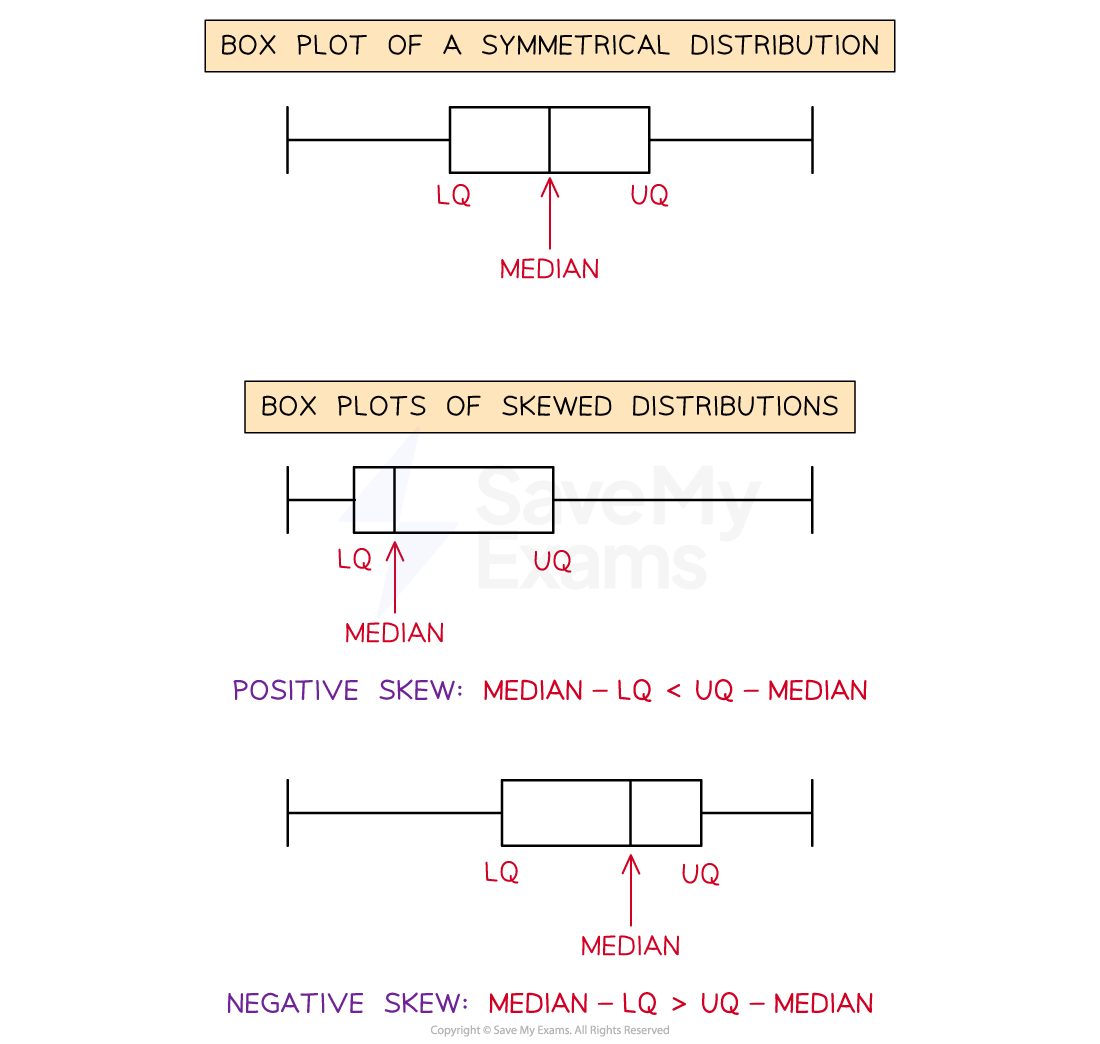
  • On a box plot looking at the median and quartiles can help you decide how a distribution is skewed

    • If the median is closer to the lower quartile then the distribution has positive skew

      • median - LQ < UQ - median

    • If the median is closer to the upper quartile then the distribution has negative skew

      • median - LQ > UQ - median

    • If the median is in the middle of the two quartiles then the distribution is symmetrical

Examples of box plots that are symmetrical or with positive or negative skew

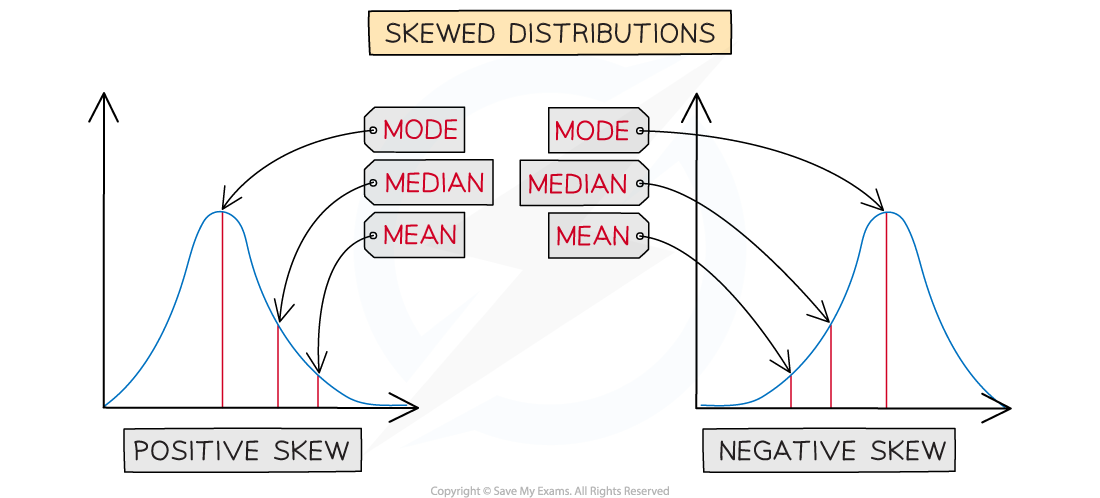
  • Looking at the values of the averages can help you decide how a distribution is skewed

    • mean > median > mode can indicate positive skew

    • mode > median > mean can indicate negative skew

    • In a perfectly symmetrical distribution the three averages are equal

2-3-3-skewness-diagram-3

Examiner Tips and Tricks

  • An exam question may not ask you specifically about skewness

    • But if a question asks about 'the shape of a distribution', you should say whether it is symmetrical or positively or negatively skewed

Worked Example

(a) Lenny collected data on the ages of customers coming into his shop one morning. This data is shown in the following stem-and-leaf diagram:

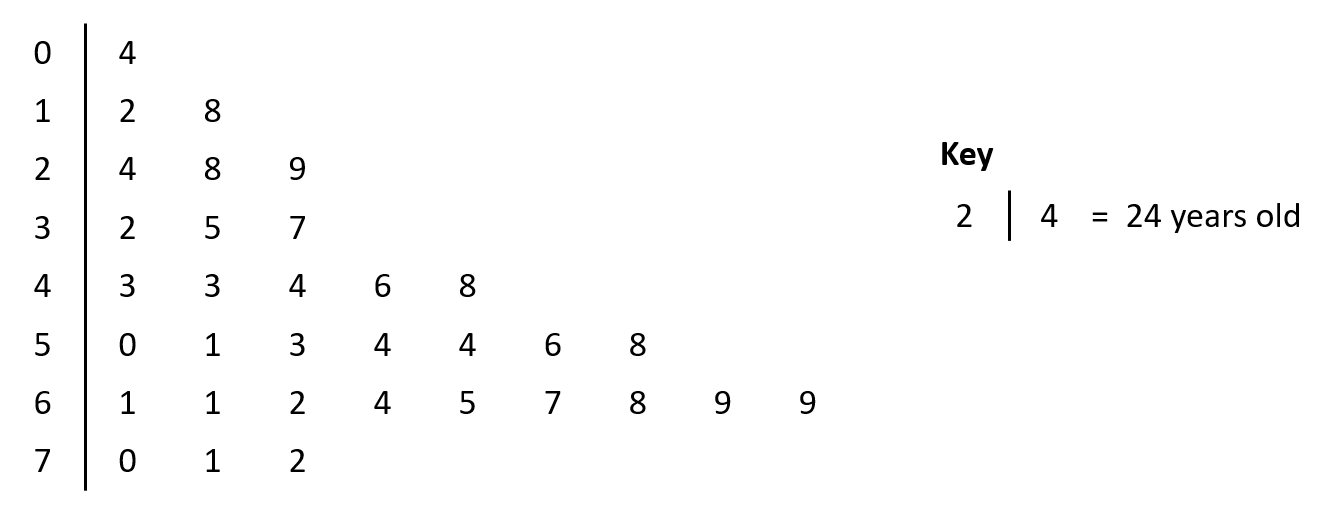
A stem and leaf diagram showing ages of customers entering a shop

Comment on the shape of the distribution.

Most of the data values are on the higher end and the 'tail' is on the lower end
This means that the distribution has negative skew
(Note you can also 'read' the shape of the distribution by the looking at the length of the leaves row next to each stem)

The distribution has negative skew

(b) John also collected data on the ages coming into his shop one morning. He calculated the following statistics from his data:

mean = 32.4           median = 26           mode = 24

Use these statistics to comment on the skewness of the data.

Here mean > median > mode, which suggests that the data has positive skew

We have mean > median > mode
This suggests that the data has positive skew

Calculating Skew

  • It is possible to calculate the skew of a data set using this formula

    • skew equals fraction numerator 3 open parentheses mean minus median close parentheses over denominator standard space deviation end fraction

      • This formula is on the exam formula sheet, so you don't need to remember it

  • If the skew is positive then the data has positive skew

    • The larger the value, the stronger the skew

      • A skew of 7 is stronger than a skew of 3

  • If the skew is negative then the data has negative skew

    • The more negative the value, the stronger the skew

      • A skew of -7 is stronger than a skew of -3

  • If the skew is equal to zero then the data is symmetrical

Worked Example

A medical practice compiles data on the ages of all the patients registered with the practice. The following statistics are calculated from the data:

mean = 37.8           median = 42.5           standard deviation = 12.6

(a) Calculate the skew for the ages of the patients registered with the medical practice.

Use the formula skew equals fraction numerator 3 open parentheses mean minus median close parentheses over denominator standard space deviation end fraction

skew equals fraction numerator 3 open parentheses 37.8 minus 42.5 close parentheses over denominator 12.6 end fraction equals negative 1.119047...

-1.12 (3 s.f.)


(b) Interpret the skew in context.

The skew is negative, so the set of patient ages is negatively skewed
This means most of the patients are older, with fewer younger patients
It also means there is a greater spread of ages at the lower end

Last updated:

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Next topic

    Did this page help you?

    Roger B

    Author: Roger B

    Expertise: Maths

    Roger's teaching experience stretches all the way back to 1992, and in that time he has taught students at all levels between Year 7 and university undergraduate. Having conducted and published postgraduate research into the mathematical theory behind quantum computing, he is more than confident in dealing with mathematics at any level the exam boards might throw at you.

    Dan Finlay

    Author: Dan Finlay

    Expertise: Maths Lead

    Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.