Investigating the Cost of Using Appliances (WJEC GCSE Science (Double Award)): Revision Note

Investigating the Cost of Using Appliances

• Two appliances that have the same function may not always cost the same to run

• On most appliances, manufacturers provide energy rating labels to allow customers to make comparisons

• Energy rating labels describe:

  • The energy consumption of the appliance in kWh per year

  • The efficiency of the appliance by assigning a band value from A to G, where A is the most efficient and G is the least efficient

• Note: In the 2010s, additional bands A+, A++ and A+++ were added to reflect the increased efficiencies of modern appliances, but these have been replaced by A-G

Energy Efficiency Rating Label for a Fridge

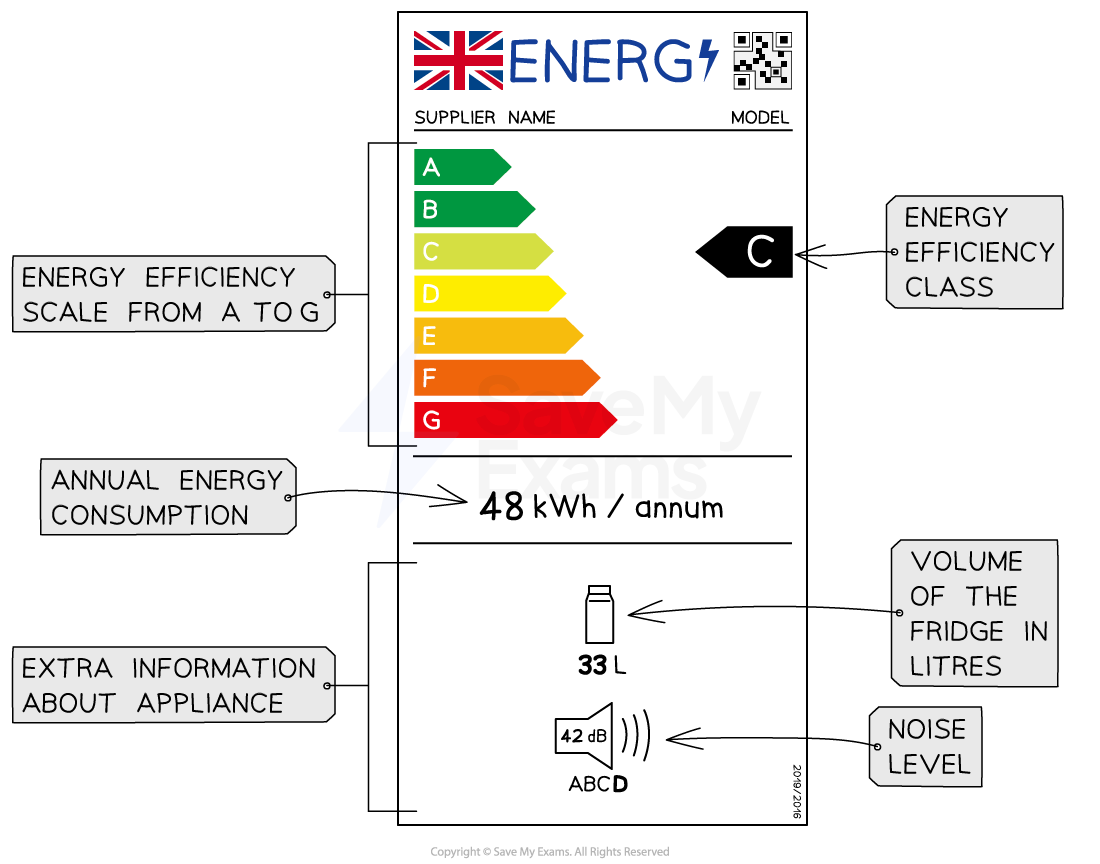
The energy rating label for a fridge tells us how efficient it is, how much energy it uses in a year, as well as additional information about its size and noise level

Comparing the Cost of Appliances

• Over the years, lightbulbs have become increasingly more efficient while generating the same amount of light 

• A comparison of 4 different bulbs is shown below

bulb	image	average lifetime	power rating (equivalent brightness)
light emitting diodes (LED)		25 000 hours	12 W
compact fluorescent (CFL)		8000 hours	15 W
halogen		2000 hours	42 W
incandescent		1000 hours	60 W

• To work out the annual energy consumption of a lightbulb:

units of energy used (kWh) = power used (kW) × time (hours)

• To work out the annual cost of each lightbulb:

cost (£) = units of energy used (kWh) × cost per unit (£ per kWh)

• Substituting in the first expression:

cost (£) = (power used (kW) × time (hours)) × cost per unit (£ per kWh)

• In one year, there are 24 × 365 = 8760 hours

• Assuming a constant usage (24 hours a day) and a tariff of 10p, or £0.10 per kWh

  • Annual cost of an LED lightbulb = 0.012 × 8760 × 0.10 = £10.51

  • Annual cost of a CFL lightbulb = 0.015 × 8760 × 0.10 = £13.14

  • Annual cost of a halogen lightbulb = 0.042 × 8760 × 0.10 = £36.79

  • Annual cost of an incandescent lightbulb = 0.060 × 8760 × 0.10 = £52.56

• Therefore, an LED lightbulb is about 5 times cheaper to run than an incandescent bulb