Graphs of Motion (OCR GCSE Combined Science A (Gateway)) : Revision Note
Graphs of Motion
Distance-Time Graphs
A distance-time graph shows how the distance of an object moving in a straight line (from a starting position) varies over time

This graph shows a moving object moving further away from its origin
Constant Speed on a Distance-Time Graph
Distance-time graphs also show the following information:
If the object is moving at a constant speed
How large or small the speed is
A straight line represents constant speed
The slope of the straight line represents the magnitude of the speed:
A very steep slope means the object is moving at a large speed
A shallow slope means the object is moving at a small speed
A flat, horizontal line means the object is stationary (not moving)
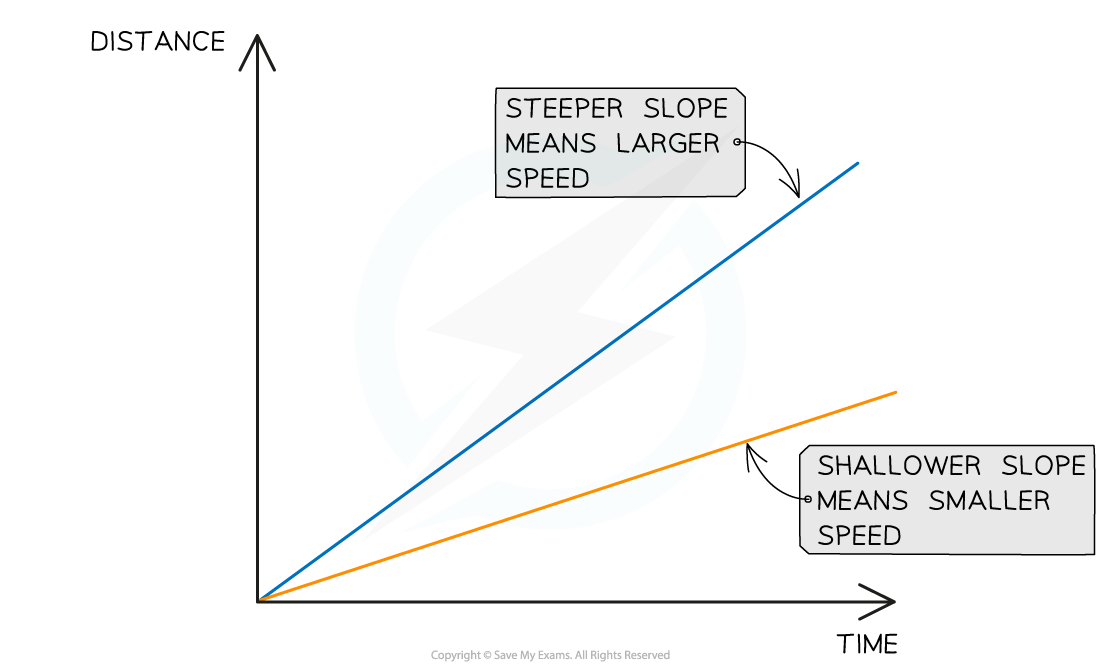
This graph shows how the slope of a line is used to interpret the speed of moving objects. Both of these objects are moving with a constant speed, because the lines are straight.
Changing Speed on a Distance-Time Graph
Objects sometimes move at a changing speed
This is represented by a curve
In this case, the slope of the line will be changing
If the slope is increasing, the speed is increasing (accelerating)
If the slope is decreasing, the speed is decreasing (decelerating)
The image below shows two different objects moving with changing speeds
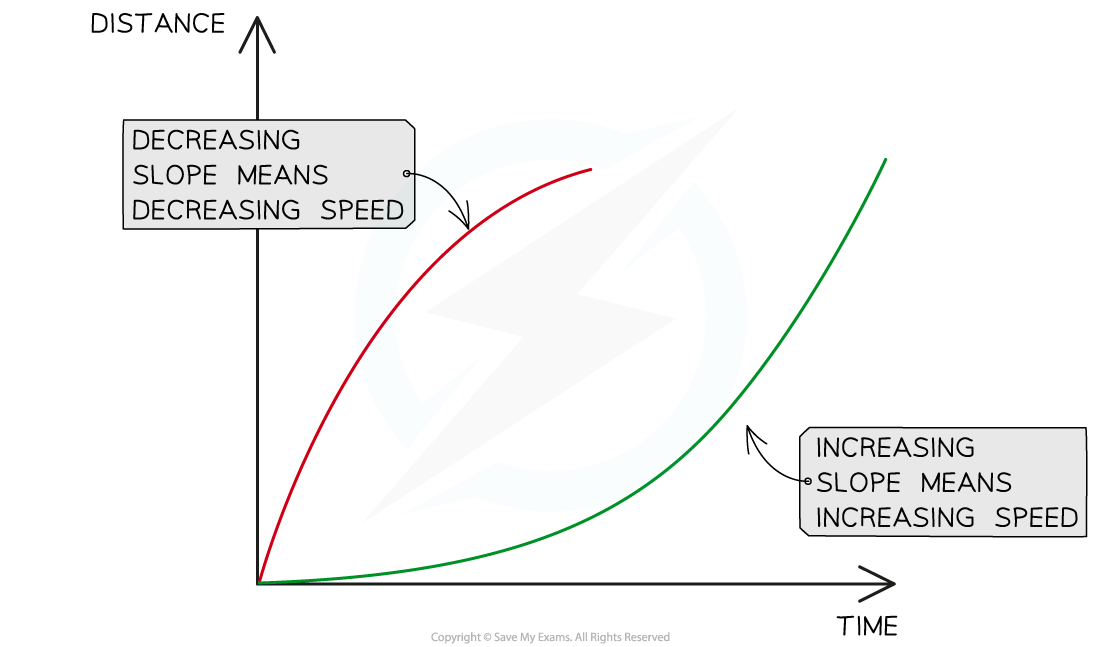
Changing speeds are represented by changing slopes. The red line represents an object slowing down and the green line represents an object speeding up.
Gradient of a Distance-Time Graph
The speed of a moving object can be calculated from the gradient of the line on a distance-time graph:
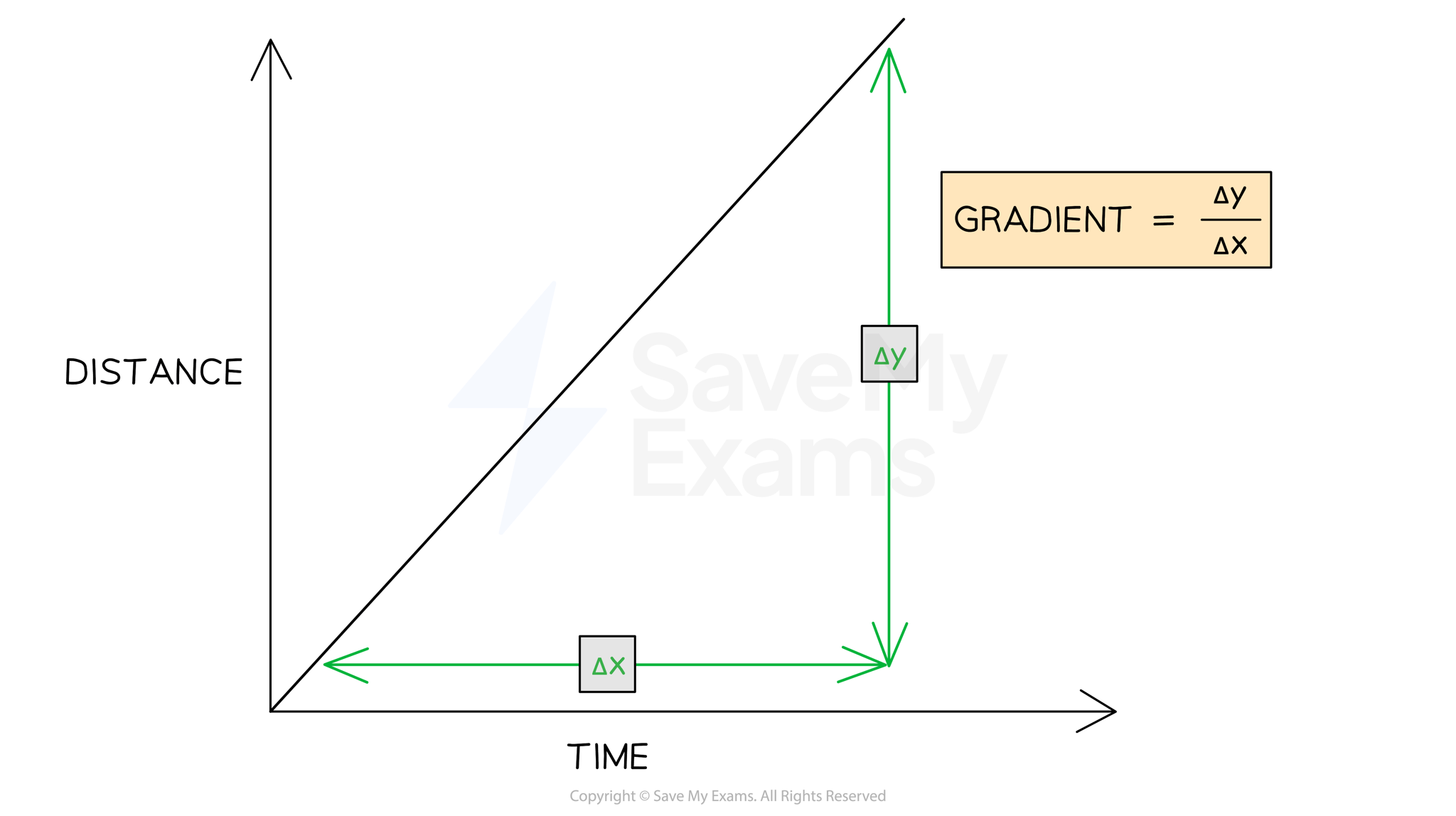
The speed of an object can be found by calculating the gradient of a distance-time graph
is the change in y (distance) values
is the change in x (time) values
Worked Example
A distance-time graph is drawn below for part of a train journey. The train is travelling at a constant speed.
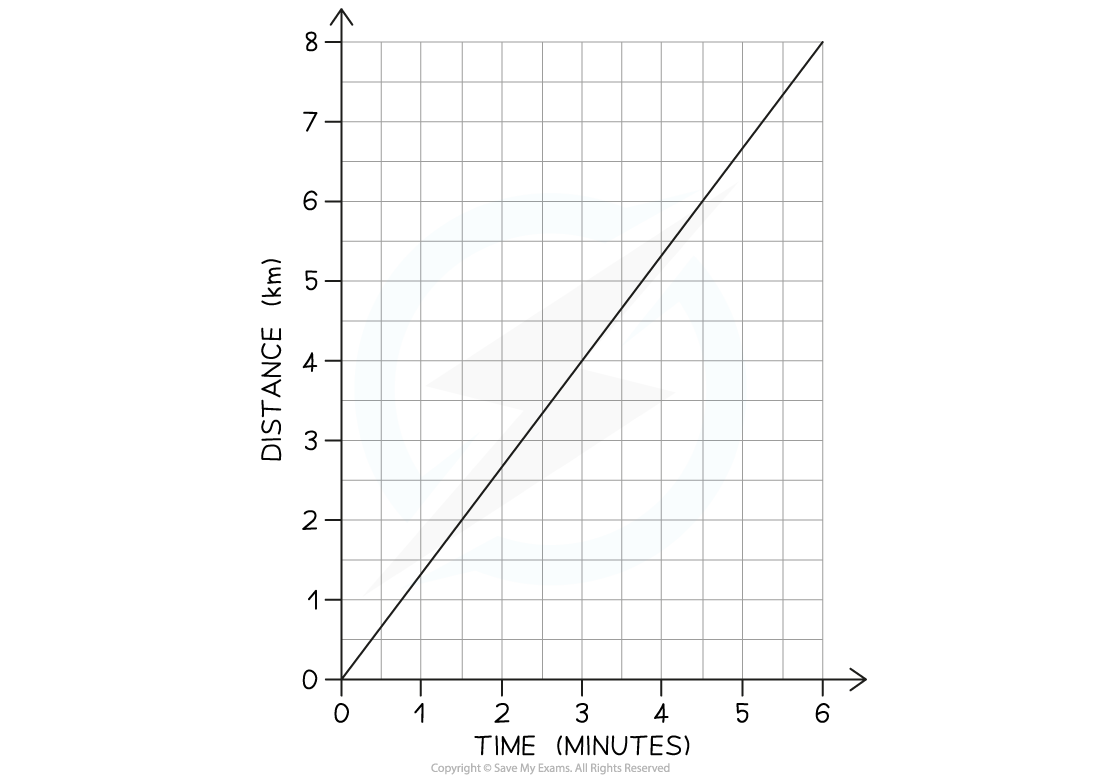
Calculate the speed of the train.
Answer:
Step 1: Draw a large gradient triangle on the graph
The image below shows a large gradient triangle drawn with dashed lines
and
are labelled, using the units as stated on each axis
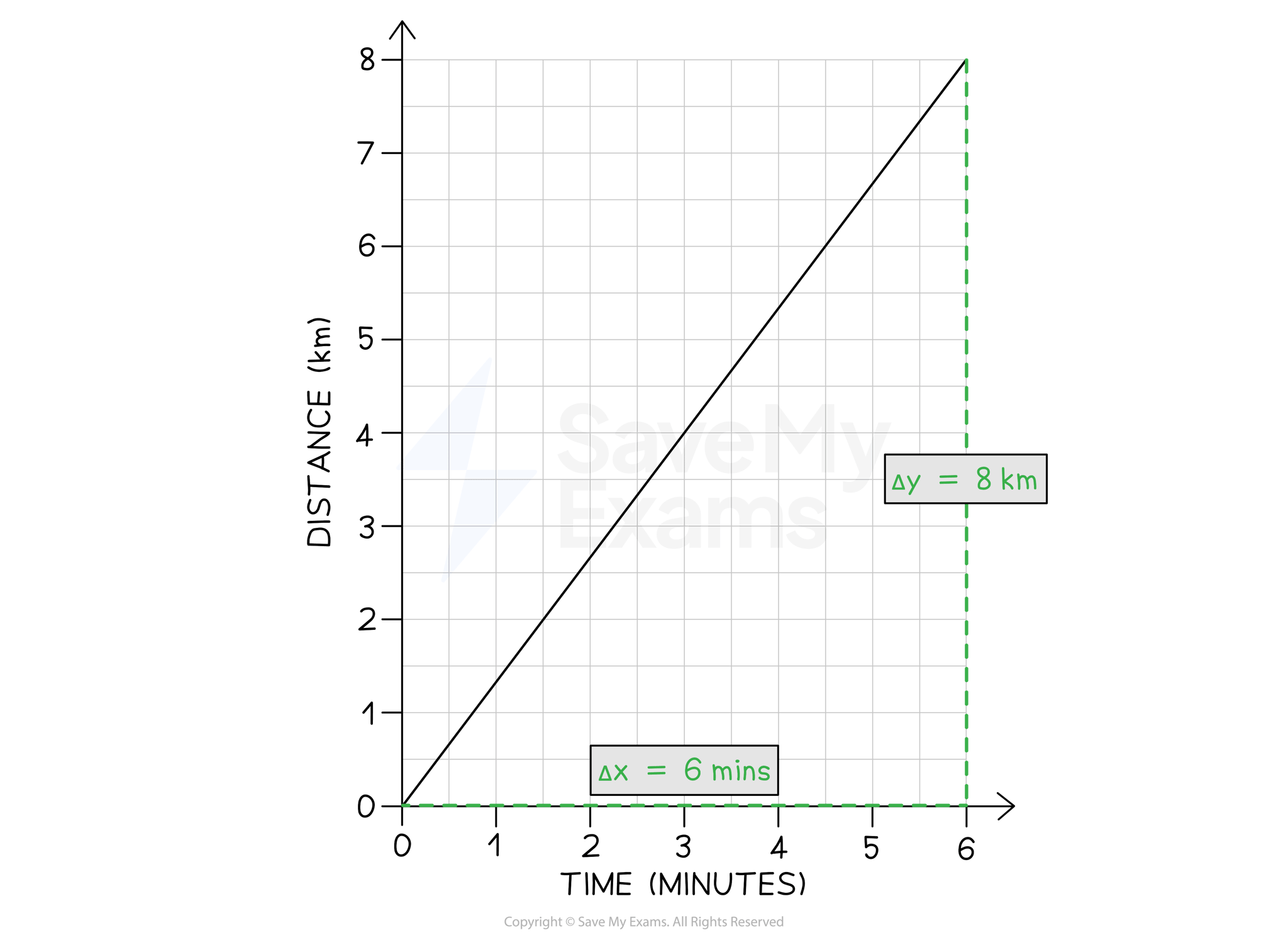
Step 2: Convert units for distance and time into standard units
The distance travelled = 8 km = 8000 m
The time taken = 6 mins = 360 s
Step 3: State that speed is equal to the gradient of a distance-time graph
The gradient of a distance-time graph is equal to the speed of a moving object:
Step 4: Substitute values to calculate the speed
Worked Example
Ose decides to take a stroll to the park. He finds a bench in a quiet spot and takes a seat, picking up where he left off reading his book on Black Holes.After some time reading, Ose realises he lost track of time and runs home.A distance-time graph for his trip is drawn below:
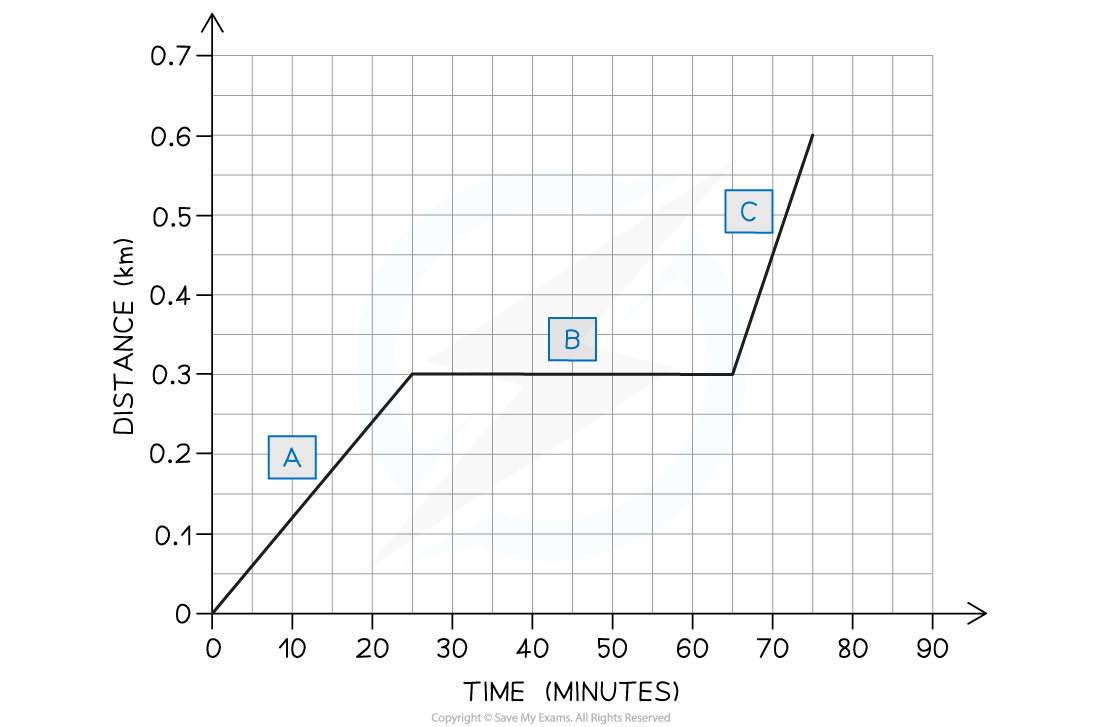
a) How long does Ose spend reading his book? There are three sections labelled on the graph: A, B and C.
b) Which section represents Ose running home?
c) What is the total distance travelled by Ose?
Answer:
Part (a)
Ose spends 40 minutes reading his book
The flat section of the line (section B) represents an object which is stationary - so section B represents Ose sitting on the bench reading
This section lasts for 40 minutes - as shown in the graph below
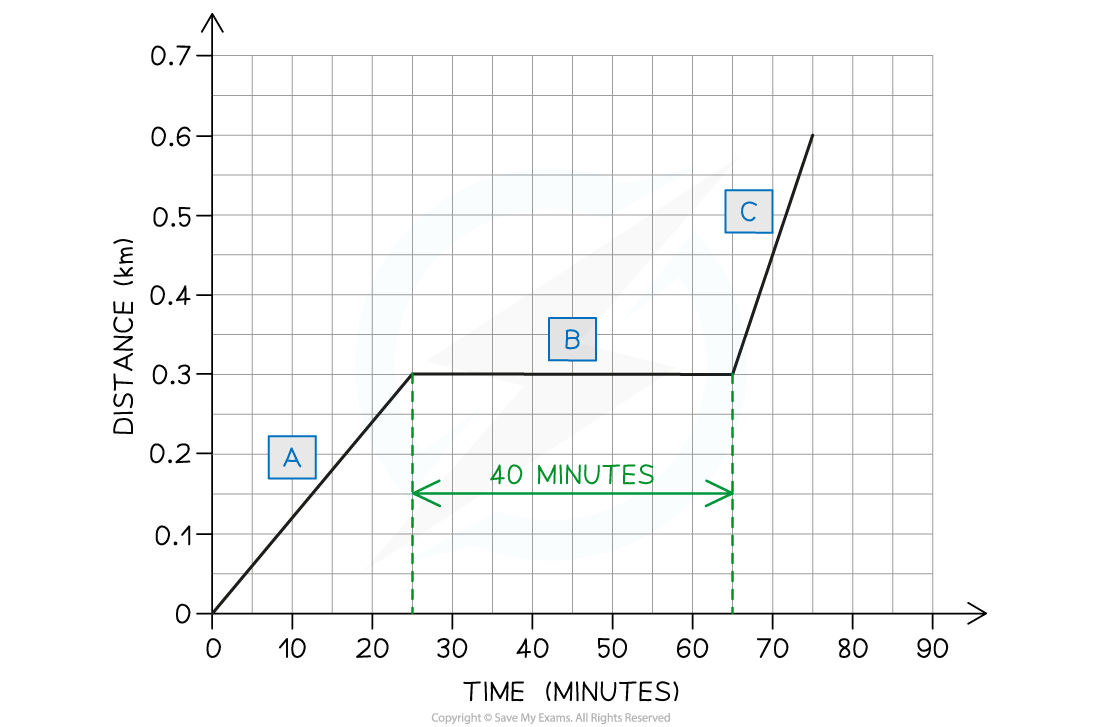
Part (b)
Section C represents Ose running home
The slope of the line in section C is steeper than the slope in section A
This means Ose was moving with a larger speed (running) in section C
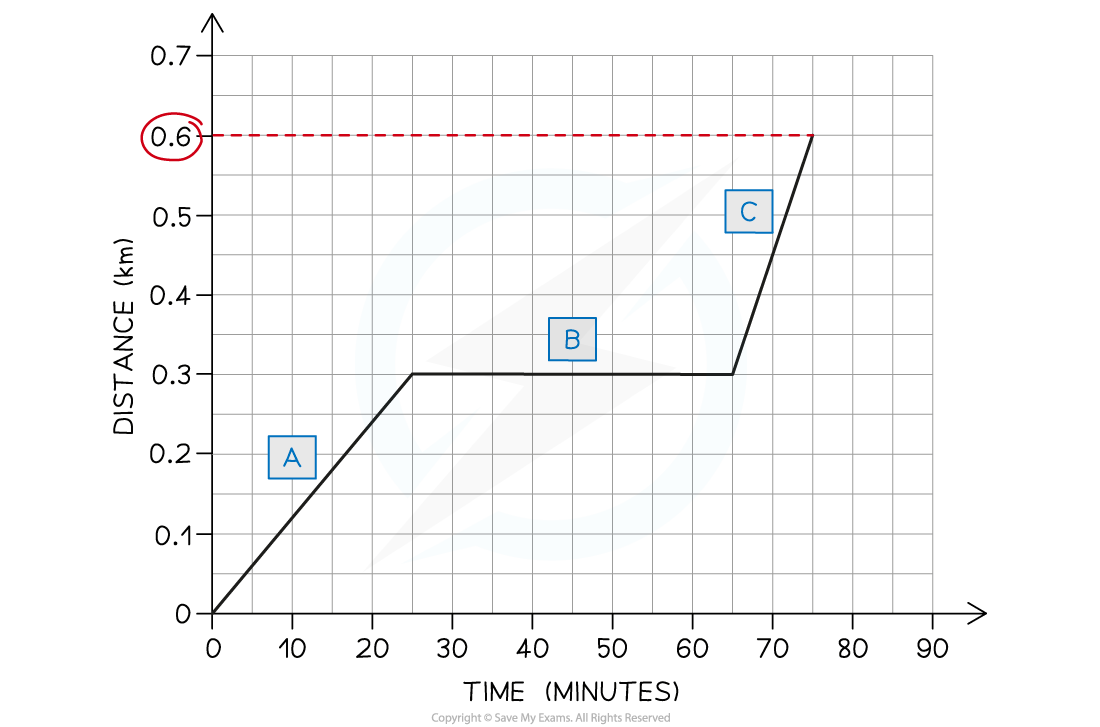
Part (c)
The total distance travelled by Ose is 0.6 km
The total distance travelled by an object is given by the final point on the line - in this case, the line ends at 0.6 km on the distance axis. This is shown in the image below:
Velocity-Time Graphs
A velocity-time graph shows how the velocity of a moving object varies with time
The red line represents an object with increasing velocity
The green line represents an object with decreasing velocity
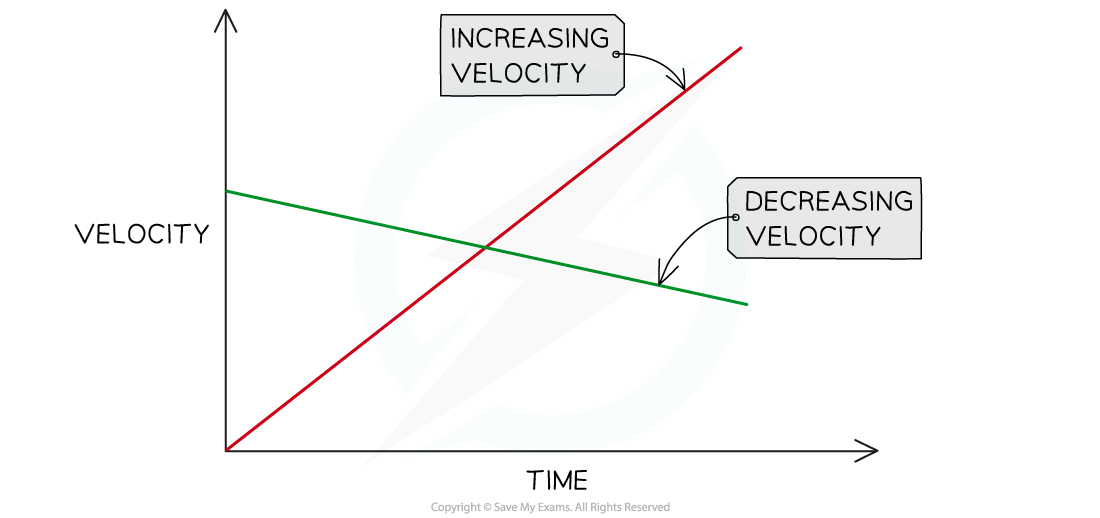
Increasing and decreasing velocity represented on a velocity-time graph
Acceleration on a Velocity-Time Graph
Velocity-time graphs also show the following information:
If the object is moving with a constant acceleration/deceleration
The magnitude of the acceleration/deceleration
A straight line represents constant acceleration
The slope of the line represents the magnitude of acceleration
A steep slope means large acceleration (or deceleration) - i.e. the object's speed changes very quickly
A gentle slope means small acceleration (or deceleration) - i.e. the object's speed changes very gradually
A flat line means the acceleration is zero - i.e. the object is moving with a constant velocity
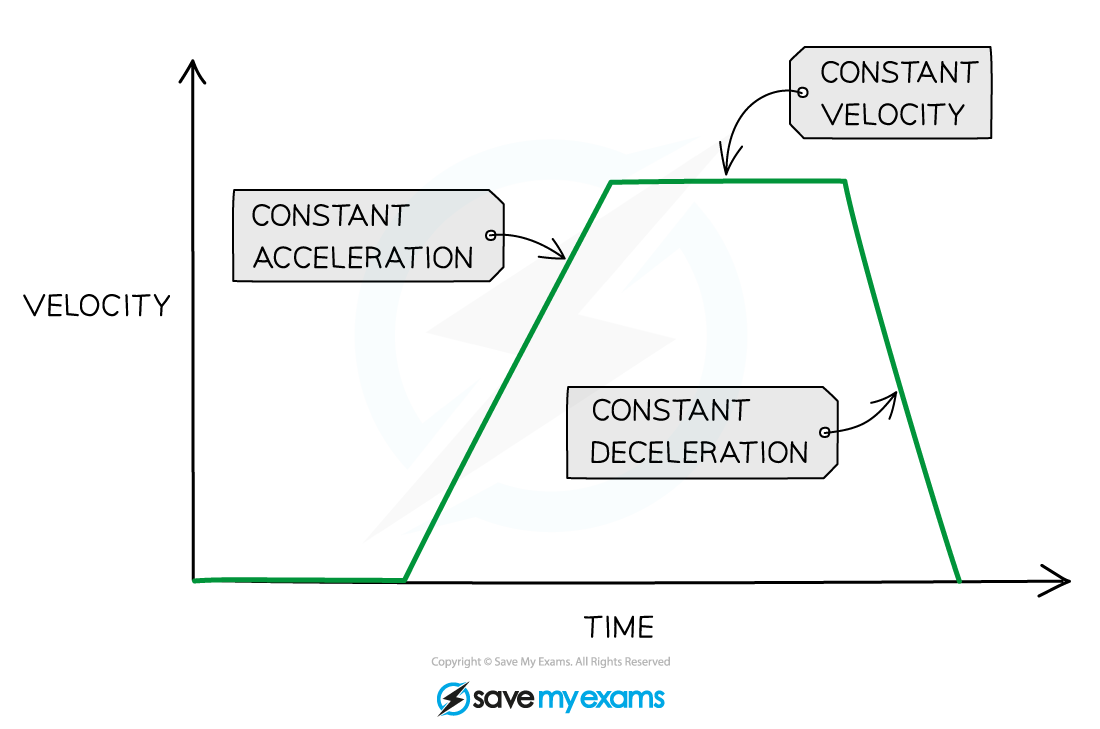
Interpreting the slope of a velocity-time graph
The acceleration of an object can be calculated from the gradient of a velocity-time graph
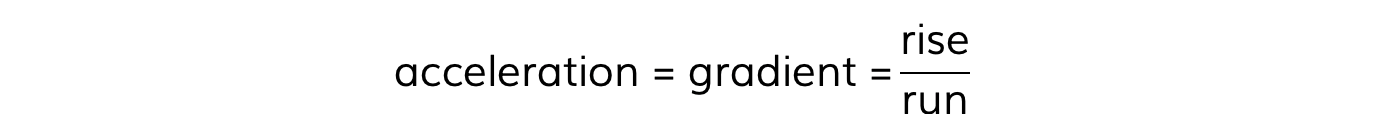
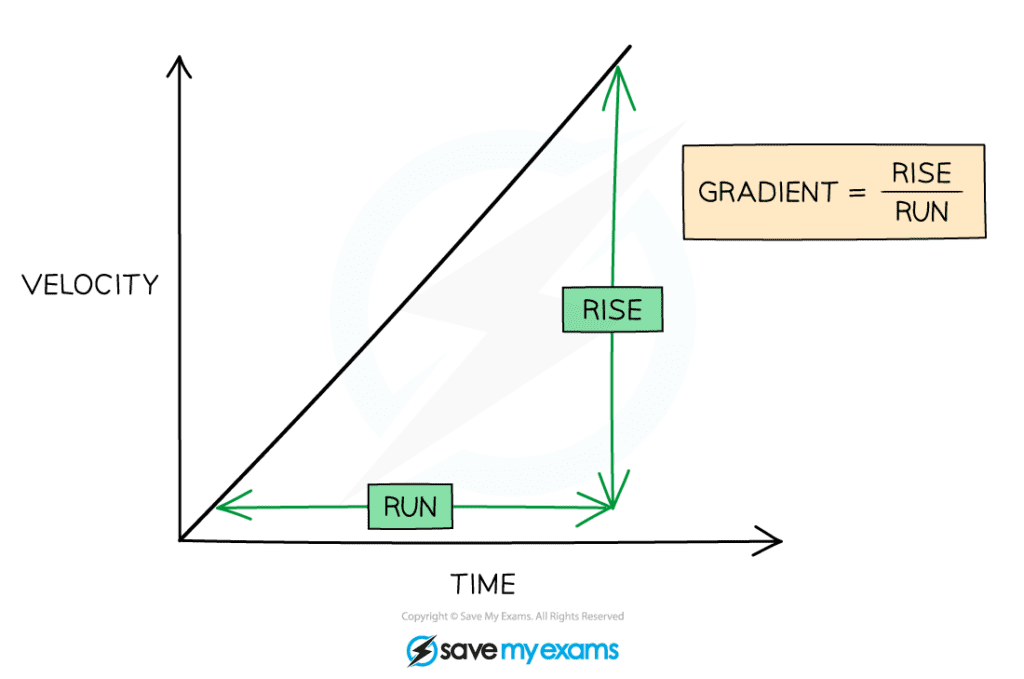
The gradient of a velocity-time graph
Worked Example
Tora is training for a cycling tournament.
The velocity-time graph below shows her motion as she cycles along a flat, straight road.
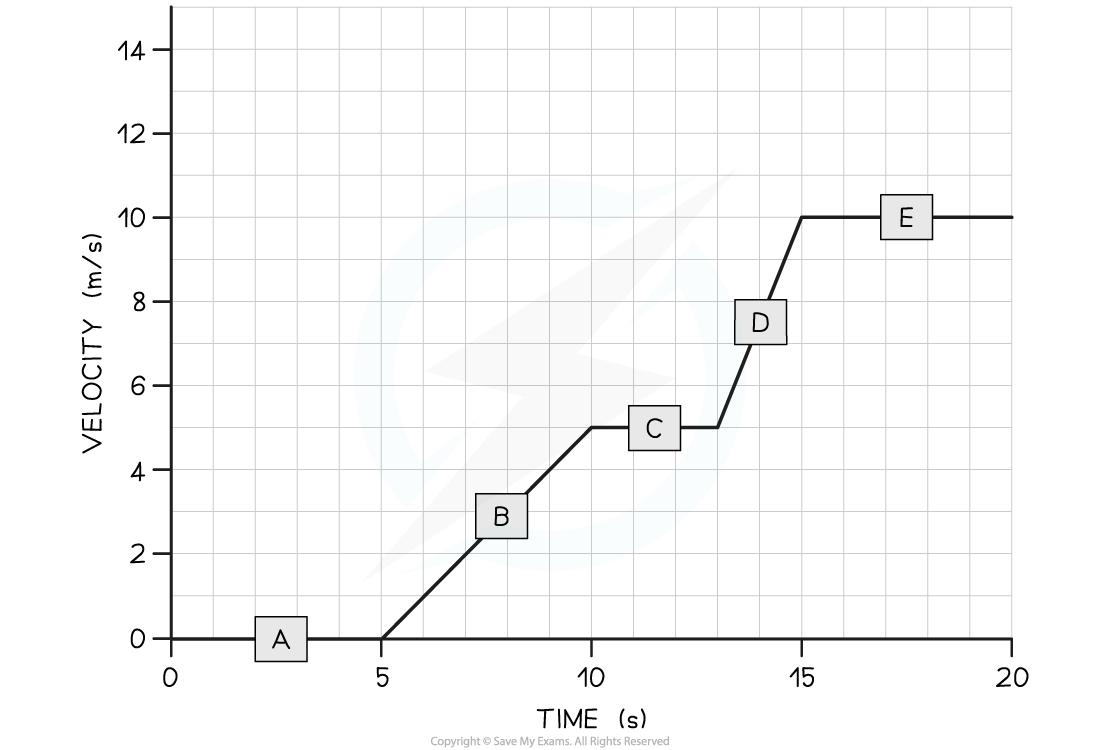
(a) In which section (A, B, C, D, or E) of the velocity-time graph is Tora’s acceleration the largest?
(b) Calculate Tora’s acceleration between 5 and 10 seconds.
Answer:
Part (a)
Step 1: Recall that the slope of a velocity-time graph represents the magnitude of acceleration
The slope of a velocity-time graph indicates the magnitude of acceleration
Therefore, the only sections of the graph where Tora is accelerating is section B and section D
Sections A, C, and E are flat – in other words, Tora is moving at a constant velocity (i.e. not accelerating)
Step 2: Identify the section with the steepest slope
Section D of the graph has the steepest slope
Hence, the largest acceleration is shown in section D
Part (b)
Step 1: Recall that the gradient of a velocity-time graph gives the acceleration
Calculating the gradient of a slope on a velocity-time graph gives the acceleration for that time period
Step 2: Draw a large gradient triangle at the appropriate section of the graph
A gradient triangle is drawn for the time period between 5 and 10 seconds below:
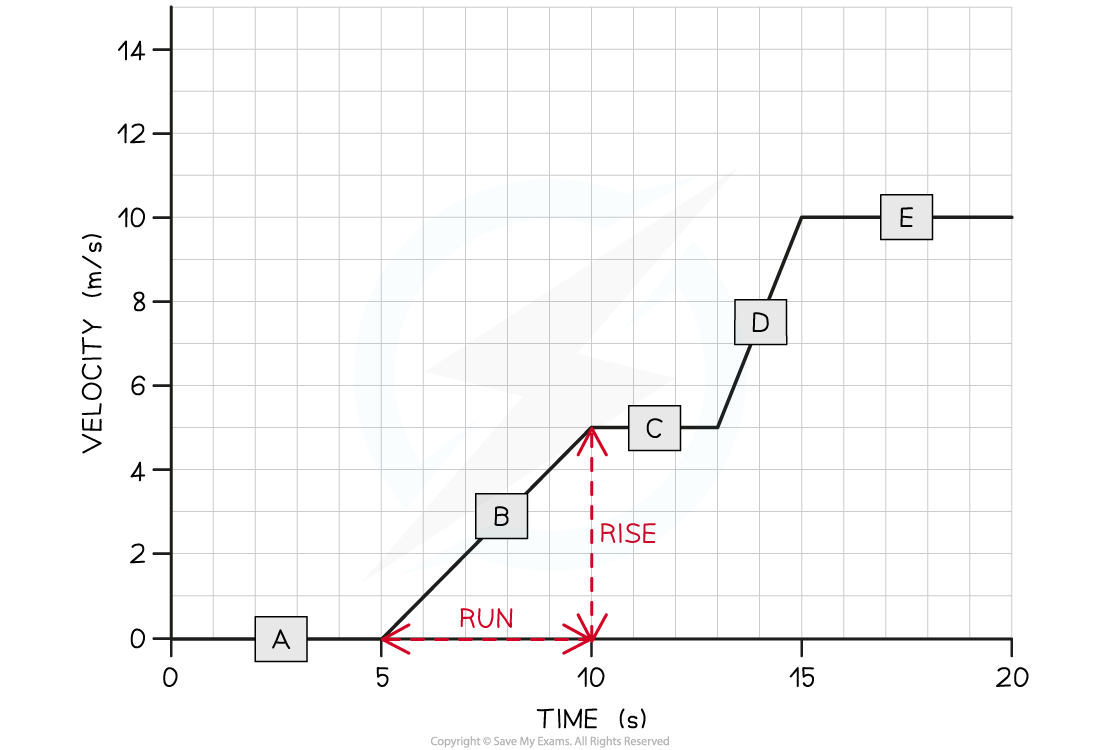
Step 3: Calculate the size of the gradient and state this as the acceleration
The acceleration is given by the gradient, which can be calculated using:
acceleration = gradient = 5 ÷ 5 = 1 m/s2
Therefore, Tora accelerated at 1 m/s2 between 5 and 10 seconds
Examiner Tips and Tricks
Use the entire line, where possible, to calculate the gradient. Examiners tend to award credit if they see a large gradient triangle used - so remember to draw 'rise' and 'run' lines directly on the graph itself!

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?

