Calculating Uniform Acceleration (OCR GCSE Combined Science A (Gateway)) : Revision Note
Calculating Uniform Acceleration
Acceleration is defined as the rate of change of velocity
In other words, it describes how much an object's velocity changes every second
The equation below is used to calculate the average acceleration of an object:
Where:
= acceleration in metres per second squared (m/s2)
= change in velocity in metres per second (m/s)
= time taken in seconds (s)
The change in velocity is found by the difference between the initial and final velocity, as written below:
change in velocity = final velocity − initial velocity
Where:
= final velocity in metres per second (m/s)
= initial velocity in metres per second (m/s)
The equation for acceleration can be rearranged with the help of a formula triangle as shown:
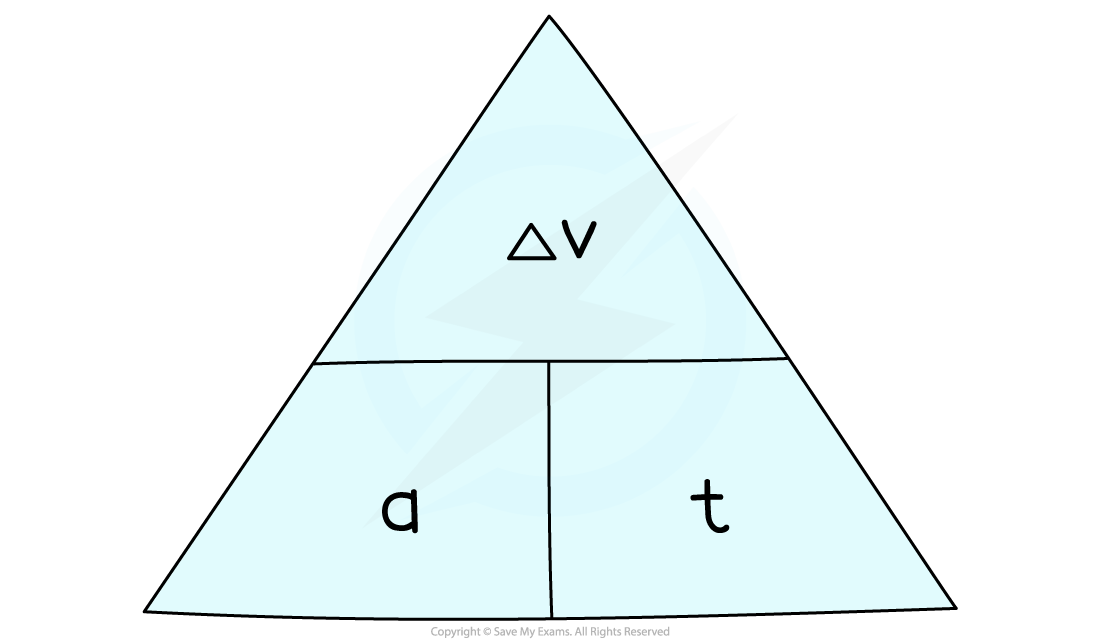
Speeding Up & Slowing Down
An object that speeds up is accelerating
An object that slows down is decelerating
The acceleration of an object can be positive or negative, depending on whether the object is speeding up or slowing down
If an object is speeding up, its acceleration is positive
If an object is slowing down, its acceleration is negative (sometimes called deceleration)
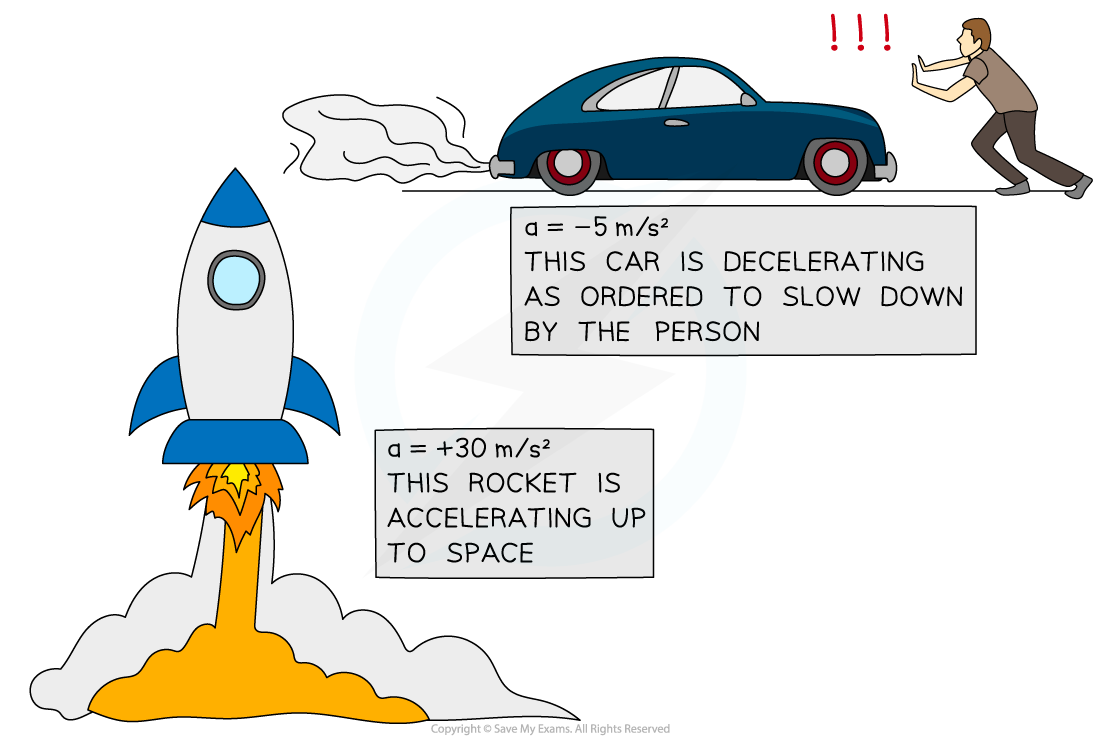
A rocket speeding up (accelerating) and a car slowing down (decelerating)
Uniform Acceleration
The following equation of motion applies to objects moving with uniform (constant) acceleration:
(final speed)2 − (initial speed)2 = 2 × acceleration × distance travelled
Where:
x = distance travelled in metres (m)
u = initial speed in metres per second (m/s)
v = final speed in metres per second (m/s)
a = acceleration in metres per second squared (m/s2)
This equation is used to calculate quantities such as initial or final speed, acceleration, or distance travelled in cases where the time taken is not known
Worked Example
A Japanese bullet train decelerates at a constant rate in a straight line. The velocity of the train decreases from 50 m/s to 42 m/s in 30 seconds.
(a) Calculate the change in velocity of the train.
(b) Calculate the deceleration of the train, and explain how your answer shows the train is slowing down.
Answer:
Part (a)
Step 1: List the known quantities
Initial velocity,
Final velocity,
Step 2: Write down the relevant equation
change in velocity = final velocity − initial velocity
Step 3: Substitute values for final and initial velocity
The velocity of the train decreases by 8 m/s
Part (b)
Step 1: List the known quantities
Change in velocity,
Time taken,
Step 2: Write down the relevant equation
Step 3: Substitute the values for change in velocity and time
Step 4: Interpret the value for deceleration
The answer is negative, which indicates the train is slowing down
Worked Example
A car accelerates steadily from rest up to a speed of 16 m/s at a rate of 2.5 m/s2.
Calculate the distance travelled by the car during this period of acceleration.
Answer:
Step 1: Identify and write down the equation to use
The question says that the car 'accelerates steadily' - so the equation for uniform acceleration can be used:
Step 2: List the known quantities
Initial speed, u = 0 m/s (the car starts from rest)
Final speed, v = 16 m/s
Acceleration, a = 2.5 m/s2
Distance, x = ? (this needs to be calculated)
Step 3: Substitute known quantities into the equation and simplify where possible
This can be simplified to:
Step 4: Rearrange the equation to work out the distance travelled
Kinetic Energy
The kinetic energy (Ek or KE) of an object (also known as its kinetic store) is defined as:
The energy an object has as a result of its mass and speed
This means that any object in motion has kinetic energy
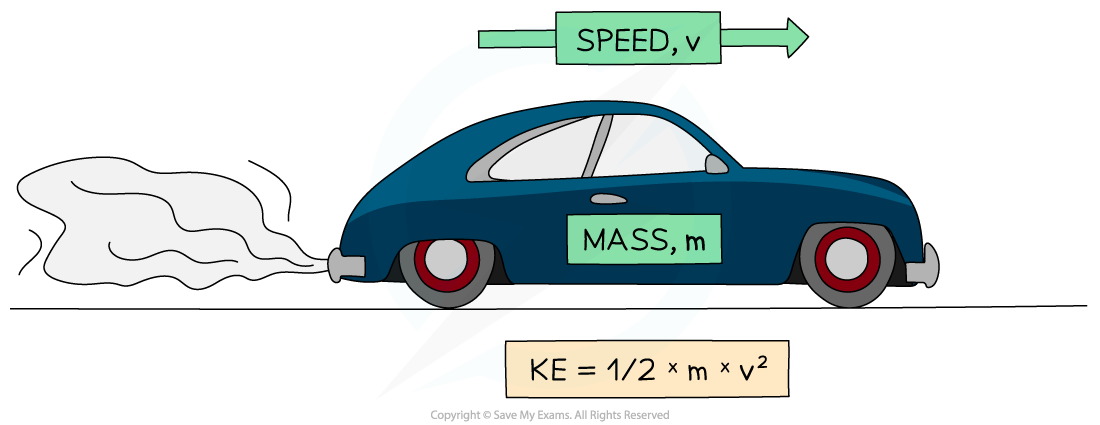
Kinetic energy can be calculated using the equation:
Where:
Ek = kinetic energy in Joules (J)
m = mass of the object in kilograms (kg)
v = speed of the object in metres per second (m/s)
Therefore, an acceleration will result in a change of kinetic energy
This is because the speed is changing
Worked Example
Calculate the kinetic energy stored in a vehicle of mass 1200 kg moving at a speed of 27 m/s.
Answer:
Step 1: List the known quantities
Mass of the vehicle, m = 1200 kg
Speed of the vehicle, v = 27 m/s
Step 2: Write down the equation for kinetic energy
Step 3: Calculate the kinetic energy
Step 4: Round the final answer to 2 significant figures
Examiner Tips and Tricks
Writing out your list of known quantities, and labelling the quantity you need to calculate, is really good exam technique. It helps you determine the correct equation to use, and sometimes examiners award credit for showing this working.

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?

