Revision NotesExam QuestionsPast Papers
GCSEPhysics: Combined ScienceOCR GatewayRevision Notes7. Energy7.1 Conservation of Energy7.1.4 KE, GPE & EPE

KE, GPE & EPE (OCR Gateway GCSE Physics: Combined Science)

Revision Note

Leander

Author

Leander

Last updated

KE, GPE & EPE

Kinetic Energy

  • Energy in an object's kinetic store is defined as:

The amount of energy an object has as a result of its mass and speed

  • This means that any object in motion has energy in its kinetic energy store

1-7-2-kinetic-energy-cie-igcse-23-rn

  • Kinetic energy can be calculated using the equation:

E space equals space 1 half m v squared

  • Where:
    • E = kinetic energy in joules (J)
    • m = mass of the object in kilograms (kg)
    • v = speed of the object in metres per second (m/s)

 

 

Gravitational Potential Energy

  • Energy in the gravitational potential store of an object is defined as:

The energy an object has due to its height in a gravitational field

  • This means:
    • If an object is lifted up, energy will be transferred to its gravitational store 
    • If an object falls, energy will be transferred away from its gravitational store 

  • The gravitational potential energy of an object can be calculated using the equation:

E space equals space m g h

  • Where:
    • E = change in gravitational potential energy, in joules (J)
    • m = mass, in kilograms (kg)
    • g = gravitational field strength in newtons per kilogram (N/kg)
    • h = change in height in metres (m)

1-7-3-gravitational-potential-energy-cie-igcse-23-rn

Energy is transferred to the mass's gravitational store as it is lifted above the ground

 

  

Elastic Potential Energy

  • Energy in the elastic potential store of an object is defined as:

The energy stored in an elastic object when work is done on the object

  • This means that any object that can change shape by stretching, bending or compressing (eg. springs, rubber bands)
    • When a spring is stretched (or compressed), work is done on the spring which results in energy being transferred to the elastic potential store of the spring
    • When the spring is released, energy is transferred away from its elastic potential store

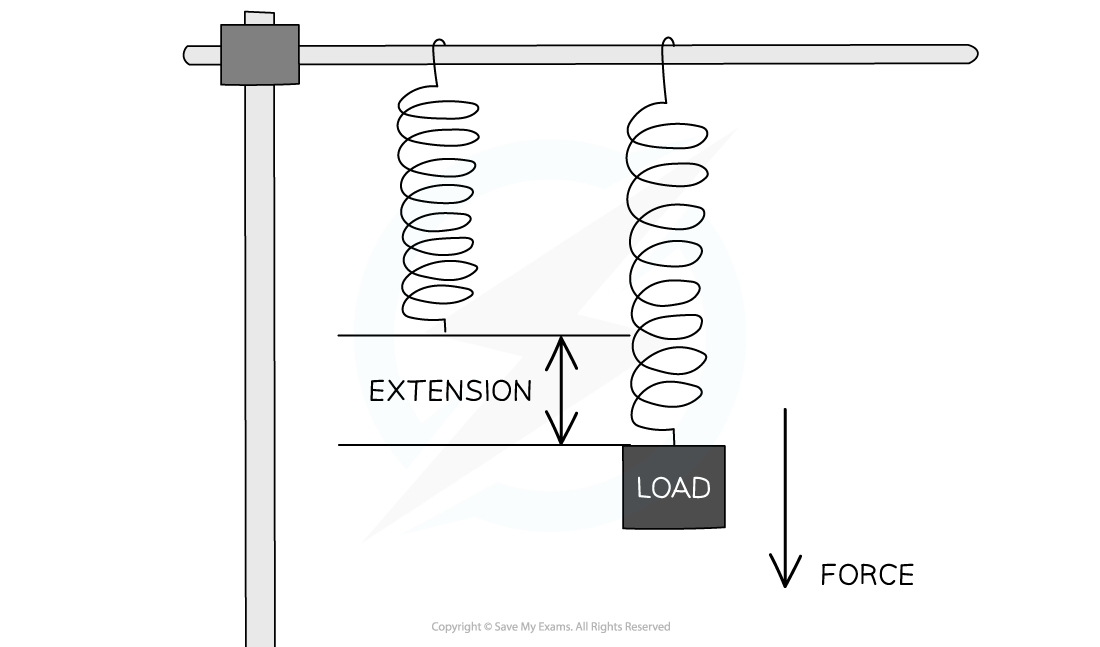
Load extension and force, downloadable AS & A Level Physics revision notes

How to determine the extension, e, of a stretched spring

  • The amount of elastic potential energy stored in a stretched spring can be calculated using the equation:

E subscript e space equals space 1 half k x squared

  • Where:
    • Ee = elastic potential energy in joules (J)
    • k = spring constant in newtons per metre (N/m)
    • x = extension in metres (m)

  • The above equation assumes that the spring has not been stretched beyond its limit of proportionality

Elastic-limit, IGCSE & GCSE Physics revision notes

The spring on the right has been stretched beyond the limit of proportionality

 

 

Energy Transfers in a Vertical Spring

  • When a vertical spring is extended and contracted, energy is transferred
  • Although the total energy of the spring system will remain constant, energy will be transferred between
    • The elastic potential energy store
    • The kinetic energy store
    • The gravitational potential energy store

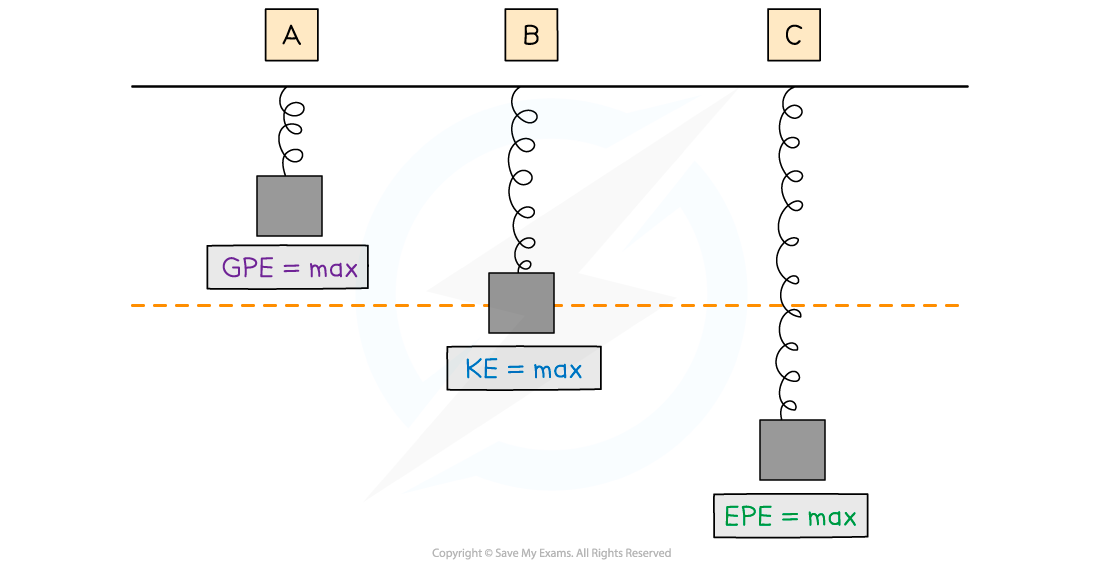
Change in Spring Energy, downloadable AS & A Level Physics revision notes

Energy transfers when a spring oscillates

  • At position A:
    • The spring has some energy in its elastic potential store since it is slightly compressed
    • The spring has zero energy in its kinetic store since it is stationary
    • The amount of energy in the gravitational potential store of the spring is at a maximum because the mass is at its highest point

  • At position B:
    • The spring has some energy in its elastic potential store since it is slightly stretched
    • The amount of energy in its kinetic store is at a maximum as it passes through its resting position at its maximum speed
    • The spring has some energy in its gravitational potential store since the mass is at its midway point in height
  • At position C:
    • The amount of energy in the elastic potential store of the spring is at its maximum because it is at its maximum extension
    • The spring has zero energy in its kinetic store since it is stationary
    • The amount of energy in the gravitational potential store GPE is at a minimum because it is at its lowest point in the oscillation

Worked example

The diagram below shows a student before and after a bungee jump. The bungee cord has an unstretched length of 30.0 m.KE GPE EPE Worked Example, downloadable AS & A Level Physics revision notes

 

The mass of the student is 60.0 kg. The gravitational field strength is 9.8 N / kg.

Calculate:

a) The change in gravitational potential energy of the student at 30.0 m

b) The maximum change in the gravitational potential energy of the student

c) The speed of the student after falling 30.0 m if 90% of the energy in the student's gravitational potential store is transferred to the student's kinetic store

d) The spring constant of the bungee cord if all the energy in the gravitational potential store of the student is transferred to the elastic potential store of the bungee cord

 

Part (a)

Step 1: List the known quantities

      • Mass of the student, m = 60.0 kg
      • Gravitational field strength, g = 10 N/kg
      • Change in height, h = 30.0 m

Step 2: Write out the equation for gravitational potential energy

E space equals space m g h

Step 3: Calculate the change in gravitational potential energy

E space equals space 60 space cross times space 10 space cross times space 30

E space equals space 18 space 000 space straight J

 

Part (b)

Step 1: List the known quantities

      • Mass of the student, m = 60.0 kg
      • Gravitational field strength, g = 10 N/kg
      • Maximum change in height, h = 75.0 m

Step 2: Calculate the maximum change in gravitational potential energy

E subscript space m a x end subscript space equals space m g h subscript m a x end subscript

E subscript space m a x end subscript space equals space 60 space cross times space 10 space cross times space 75

E subscript space m a x end subscript space equals space 45 space 000 space straight J

 

Part (c)

Step 1: List the known quantities

      • Mass of the student, m = 60.0 kg
      • E at 30.0 m = 18 000 J

Step 2: Determine 90% of the E at 30.0 m 

E space equals space 0.9 space cross times space 18 space 000

E space equals space 16 space 200 space straight J

Step 3: Write out the equation for KE

E space equals space 1 half m v squared

Step 4: Rearrange to make speed the subject

      • Multiply both sides by 2:

 m v squared space equals space 2 space E

      • Divide both sides by m:

 v squared space equals space fraction numerator 2 space E over denominator m end fraction

      • Take the square root of both sides:

 v space equals space square root of fraction numerator 2 E over denominator m end fraction end root

Step 5: Calculate the speed

 v space equals space square root of fraction numerator 2 space cross times space 16 space 200 over denominator 60 end fraction end root space

v space equals space 23.2 space straight m divided by straight s

 

Part (d)

Step 1: List the known quantities

      • E subscript space m a x end subscript = 45 000 J
      • E subscript e at 75.0 m =  E subscript e space m a x end subscript 
    • Step 2: Determine the extension of the bungee cord 

 

e space equals space 75.0 space minus space 30.0

e space equals space 45.0 space straight m

Step 3: Write out the equation for elastic potential energy

E subscript e space equals space 1 half k x squared

 

Step 3: Rearrange to make spring constant, k, the subject

      • Multiply both sides by 2:

 k x squared space equals space 2 space E subscript e

      • Divide both sides by x squared:

 k space equals space fraction numerator 2 E subscript e over denominator x squared end fraction

Step 4: Calculate the spring constant

 k space equals space fraction numerator 2 space cross times space 45 space 000 over denominator 45 squared end fraction

k space space equals space 44.4 space straight N divided by straight m

Examiner Tip

If a question asks you to "state" a value, you do not need to carry out a calculation: The answer will almost certainly be a number either from a previous answer or which was given somewhere in the question.

For example, if you have just calculated the gravitational potential energy of an object and are then asked to state the kinetic energy a moment later, the answers are very likely to be the same.

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:7.1.3 Changes in EnergyNext:7.2.1 Wasted Energy
    Leander

    Author: Leander

    Expertise: Physics

    Leander graduated with First-class honours in Science and Education from Sheffield Hallam University. She won the prestigious Lord Robert Winston Solomon Lipson Prize in recognition of her dedication to science and teaching excellence. After teaching and tutoring both science and maths students, Leander now brings this passion for helping young people reach their potential to her work at SME.