Pressure in a Liquid (OCR GCSE Physics A (Gateway))

Factors Affecting Pressure in a Liquid

Higher Tier Only

• When an object is immersed in a liquid, the liquid will exert a pressure, squeezing the object

• This pressure is exerted evenly across the whole surface of the liquid and in all directions

  • The greater the depth of the liquid, the greater the pressure

  • The greater the density of the liquid, the greater the pressure

• In a liquid, the pressure at a point increases with the height of the column of liquid about that point

  • If there is more liquid above that point, then the pressure is more

• This is because the pressure in a liquid is caused by the weight of the liquid pushing against objects immersed in the liquid

  • As the liquid becomes deeper, the amount of liquid (and hence the weight) increases which causes the pressure to increase

• This is why, for example, the pressure increases with the depth of the ocean

  • The pressure on the seabed is far higher than that on the surface of the ocean

• The weight of the liquid also depends on its density

  • A more dense liquid has a greater weight and therefore will exert a higher pressure

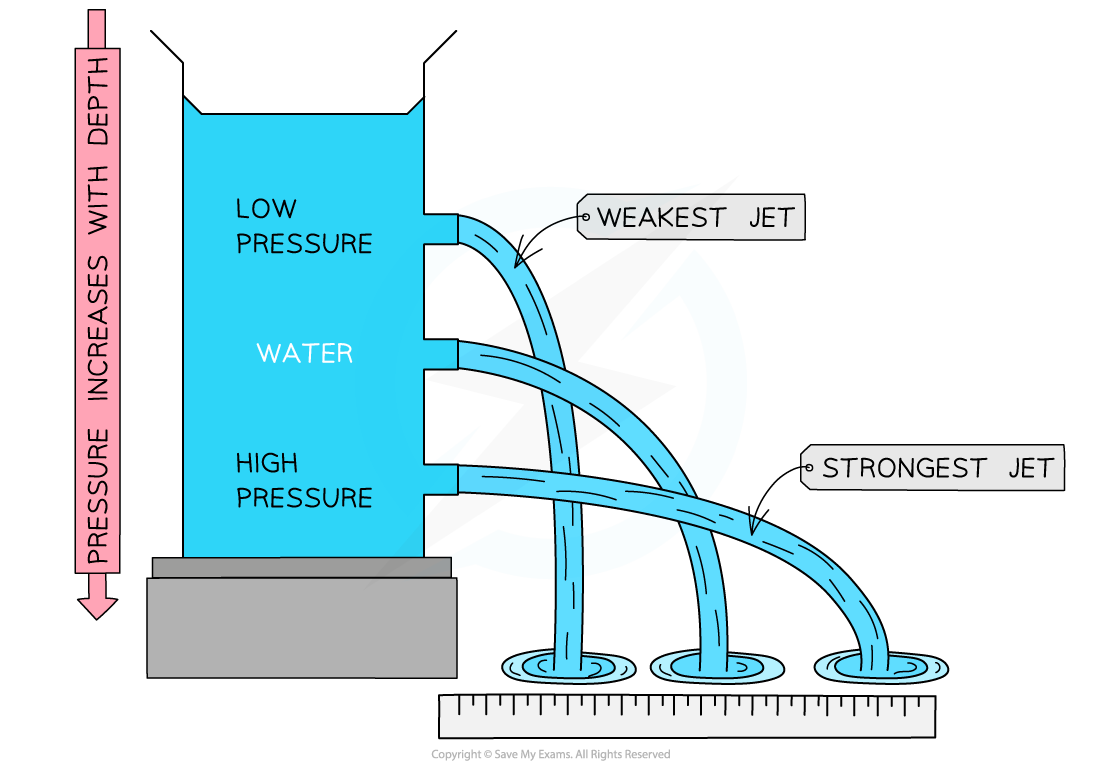
Pressure in a column of water increases with depth, shown by the strong and weak jet of water

• In a column of water, the highest pressure would be at the bottom

  • If a hole is made at the bottom of the column, the water will pour out with a large force

  • If a hole was made at the top of the column, the water will pour out with a small force

  • This is because of the difference in pressure in the column caused by the weight of the water

Pressure Differences & Upthrust

• Upthrust is due to the difference in pressure between the top and the bottom of the submerged object

• A partially (or totally) submerged object experiences a greater pressure on the bottom surface than on the top surface

  • This is because the pressure p is proportional to the depth h of the object

• The difference in pressure creates a resultant force upwards (upthrust)

• Upthrust is why objects appear to weigh less when immersed in a liquid

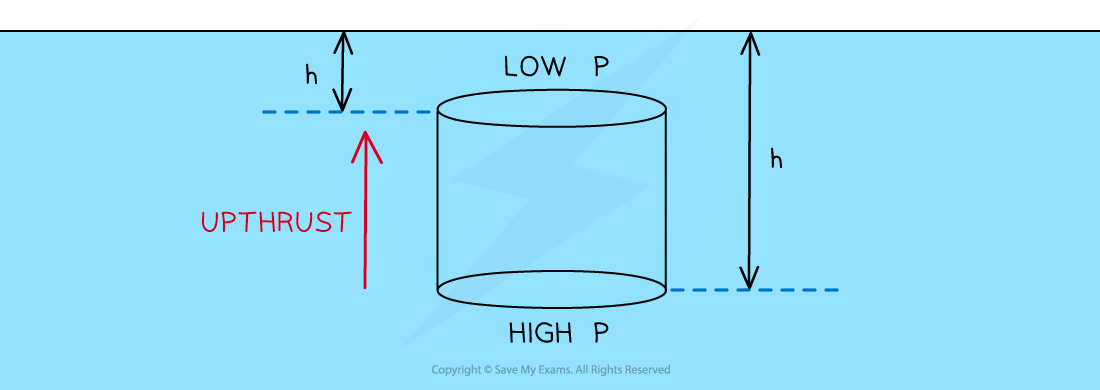
Upthrust is due to the different pressure at the top and bottom of this cylinder

• The amount of upthrust on an object depends on the weight of the fluid that the object displaces

  • Upthrust is equal to the weight of fluid displaced

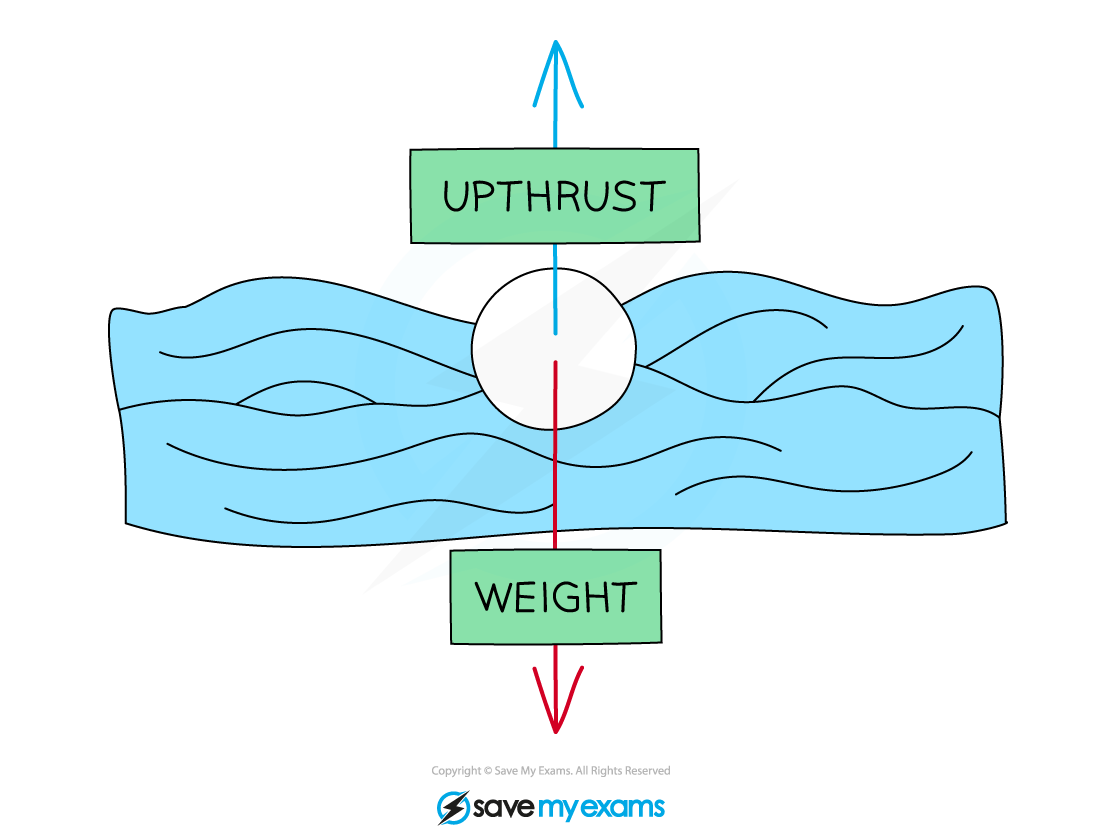
Liquids exert an upwards force (upthrust) on objects immersed in them equal to the weight of the fluid displaced

Calculating Pressure in a Liquid

Higher Tier Only

• The pressure due to a column of liquid can be calculated using the equation

p = h × ρ × g

• Where:

  • p = pressure in pascals (Pa)

  • h = height of the column in metres (m)

  • ρ = density of the liquid in kilograms per metre cubed (kg/m³)

  • g = gravitational field strength on Earth in newtons per kilogram (N/kg)

• The force from the pressure is exerted evenly across the whole surface of an object in a liquid, and in all directions

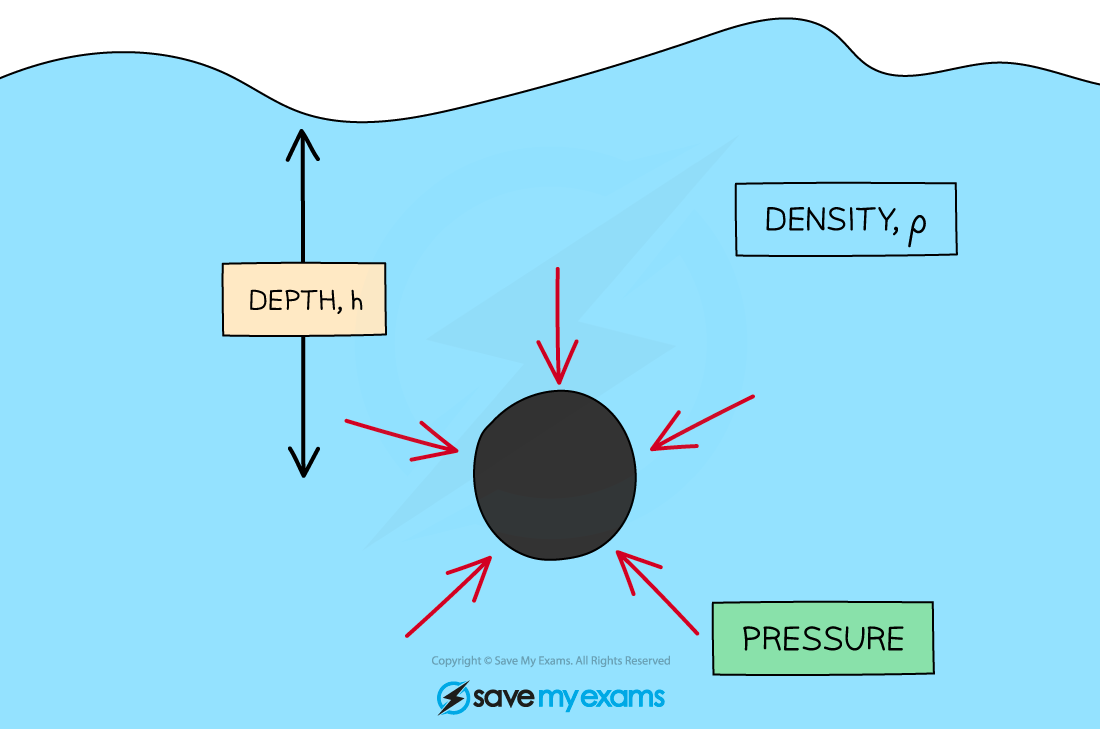
The force from the pressure of objects in a liquid is exerted evenly across its whole surface

• The pressure is more accurately a difference in pressure at different depths h in a liquid, since the pressure changes with the depth