Calculating Depth & Distance (Edexcel GCSE Physics) : Revision Note
Calculating Depth & Distance
Higher Tier Only
If the speed of a wave is known, it can be used to calculate the distance to an object, or the depth of an object - say, underwater
Calculating Distance
The worked example below demonstrates how the speed of sound in air can be used to determine how far away objects are from an observer
Worked Example
A clap of thunder is heard 4 seconds after the corresponding flash of lightning. How far away is the thunderstorm? (The speed of sound in air is 330 m/s)
Answer:
Step 1: List the known quantities
Wave speed, v = 330 m/s
Time, t = 4 s
Step 2: Write out the wave speed, distance and time formula
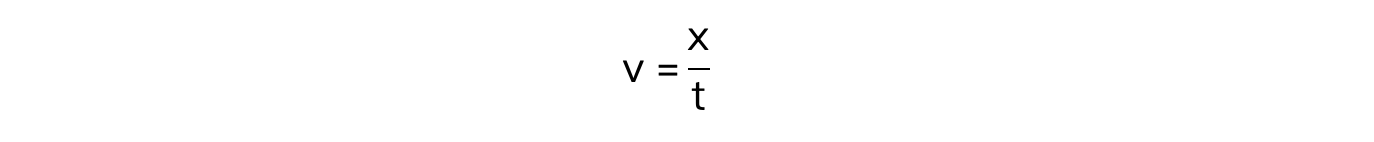
Step 3: Re-arrange the equation to make distance (x) the subject
x = v × t
Step 4: Put known values into the equation
x = 330 × 4 = 1320 m
So the distance to the thunderstorm is 1320 m
Calculating Depth
Echo sounding uses ultrasound to detect objects underwater
The sound wave is reflected off the ocean bottom
The time it takes for the sound wave to return is used to calculate the depth of the water
The distance the wave travels is twice the depth of the ocean
This is the distance to the ocean floor plus the distance for the wave to return
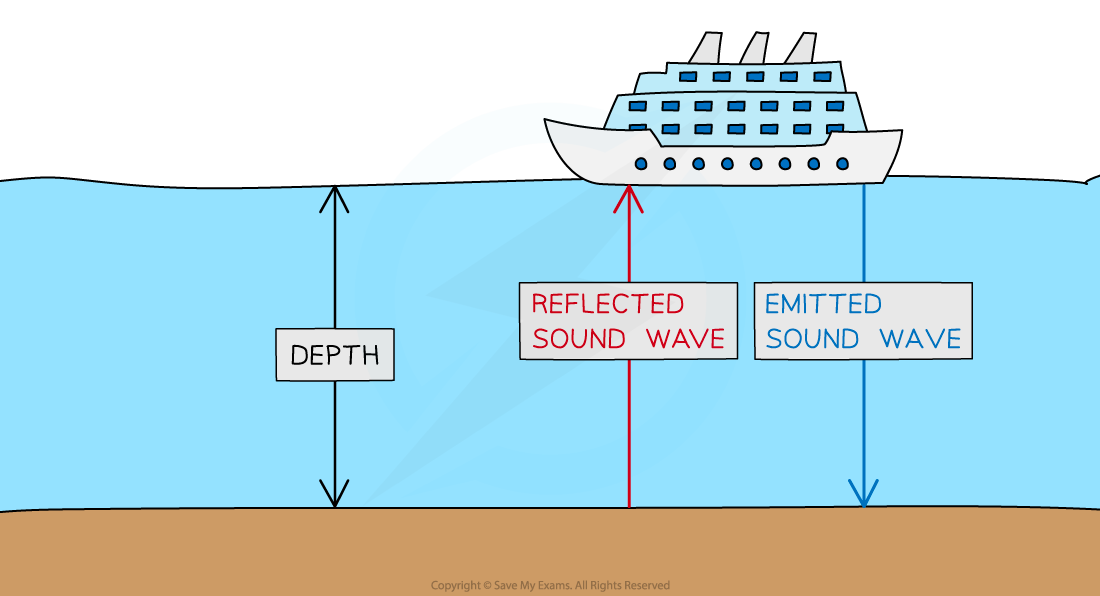
Echo sounding is used to determine water depth
Worked Example
The sound wave released from a ship took 0.12 seconds to return. The speed of sound in water is 1500 m/s. What was the depth of the sea?
Answer:
Step 1: List the known quantities
Wave speed, v = 1500 m/s
Time, t = 0.12 s
Step 2: Write out the wave speed, distance and time formula
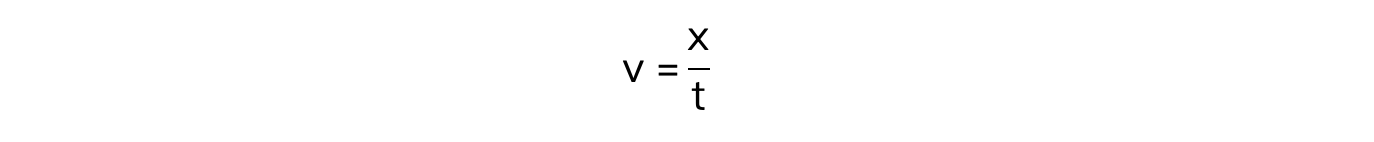
Step 3: Rearrange the equation to make distance (x) the subject
x = v × t
Step 4: Put known values into the equation
x = 1500 × 0.12 = 180 m
Step 5: Half the distance to obtain the depth
d = 180 ÷ 2
Depth, d = 90 m
Examiner Tips and Tricks
Don't forget to take into account if a sound wave has travelled twice the distance You can do this one of two ways:
Halve the time at the beginning, or
Halve the distance at the end

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
