Work Done & Energy Transfer (AQA GCSE Physics) : Revision Note
Units for Work & Energy
The formula for work is:
W = F × s
Multiplying force and distance produces units of newton-metres (N m)
Work is measured in joules (J)
This leads to a simple conversion:
1 J = 1 N m
Therefore, the number of Joules is equal to the number of newton-metres, making conversions between the units very straightforward, for example:
1000 J = 1000 N m
One Joule is equal to the work done by a force of one newton acting through one metre
Examiner Tips and Tricks
You must include the correct units in your answer, forgetting to include units will lose you a mark - in the case of work, you may use either newton-metres (N m) or Joules (J)
Work Done & Energy Transfer
Whenever any work is done, energy is transferred from one store to another
Mechanical (or electrical) working is an energy transfer pathway
The amount of energy transferred (in joules) is equal to the work done (also in joules)
energy transferred (J) = work done (J)
If a force acts in the direction that an object is moving, then the object will gain energy (energy is transferred to its kinetic store)
If the force acts in the opposite direction to the movement then the object will lose energy (energy is transferred away to the thermal store of the object and the surroundings)
Take the example of an object which is lifted vertically with a 100 N force for a distance of 10 m
The work done on the object is equal to:
W = F × s
W = 100 N × 10 m = 1000 N m
Energy is transferred mechanically to the gravitational potential store of the object
During that energy transfer 1000 J of energy was transferred
Worked Example
A woman draws a bucket up out of a well. The bucket has a mass of 10 kg when filled with water and the well is 15 m deep. Take the gravitational field strength to be 9.8 N/kg.
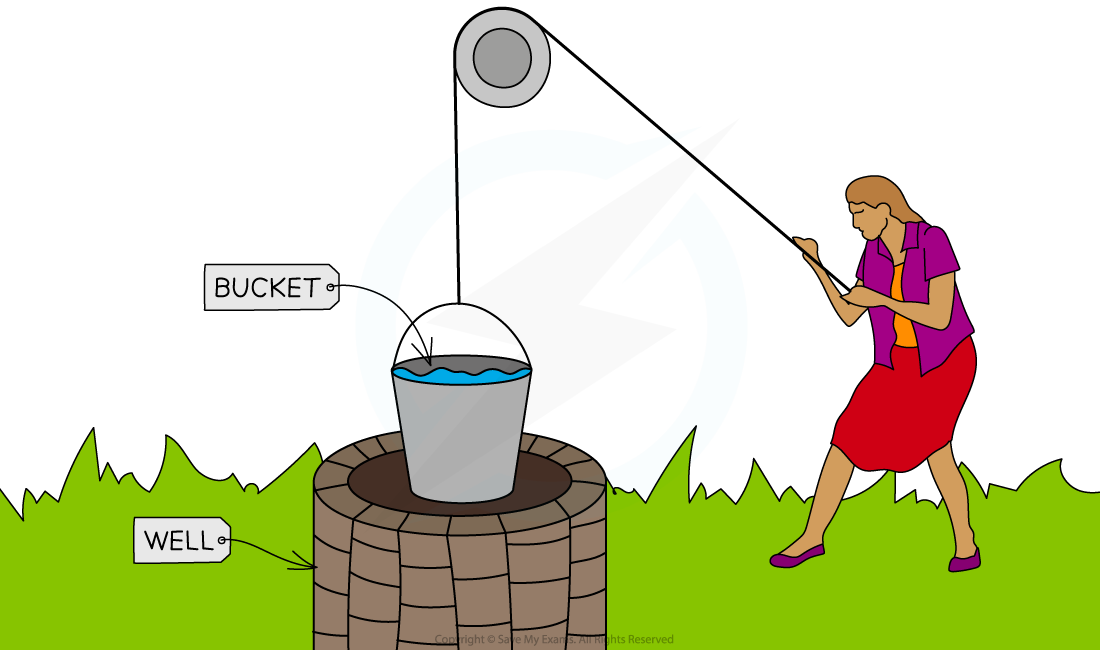
a) Describe the energy transfer involved in raising the bucket out of the well
b) Calculate the energy transferred to the bucket
Answer:
Part (a)
Work is done by the woman as she exerts a force on the rope to pull the bucket up
The work done on the bucket is due to overcoming the weight of the bucket for a distance of 15 m
As the bucket rises, energy is transferred to the gravitational potential store of the bucket
Part (b)
Step 1: List all of the known values
Mass, m = 10 kg
Gravitational field strength, g = 9.8 N/kg
Height, h = 15 m
Step 2: Write the equation relating work, force and distance
Work = Force × Distance
Step 3: Write out the equation for weight and substitute it into the work equation
Weight = m × g
Work = m × g × h
Note: This is the equation for gravitational potential energy
Step 4: Calculate the work done on the bucket
Work = 10 × 9.8 × 15 = 1470 N m
Step 5: Convert the work done into energy transferred
Energy transferred in joules = Work done in newton-metres
Energy transferred = 1470 J
The bucket gained 1470 J of gravitational potential energy
Examiner Tips and Tricks
Remember:
Changes in speed are related to kinetic energy
Changes in height are related to gravitational potential energy
Changes in the shape of materials are related to elastic potential energy

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
