Did this video help you?
Scalars & Vectors (AQA GCSE Physics)
Revision Note
Scalars & Vectors
- All quantities can be one of two types:
- A scalar
- A vector
Scalars
- Scalars are quantities that have only a magnitude
- For example, mass is a scalar quantity since it is a quantity that has no direction to it
Vectors
- Vectors have both a magnitude and a direction
- Velocity is a vector quantity since it is described with both a magnitude and a direction
- When describing the velocity of a car it is necessary to mention both its speed and the direction in which it is travelling
- For example, 60 km per hour in a Westerly direction
- Distance is a value describing only how long an object is or how far it is between two points - this means it is a scalar quantity
- Displacement on the other hand also describes the direction in which the distance is measured - this means it is a vector quantity
- For example, 100 km in a Northern direction
Comparing Scalars & Vectors
- The table below lists some common examples of scalar and vector quantities:
Scalars & Vectors Table
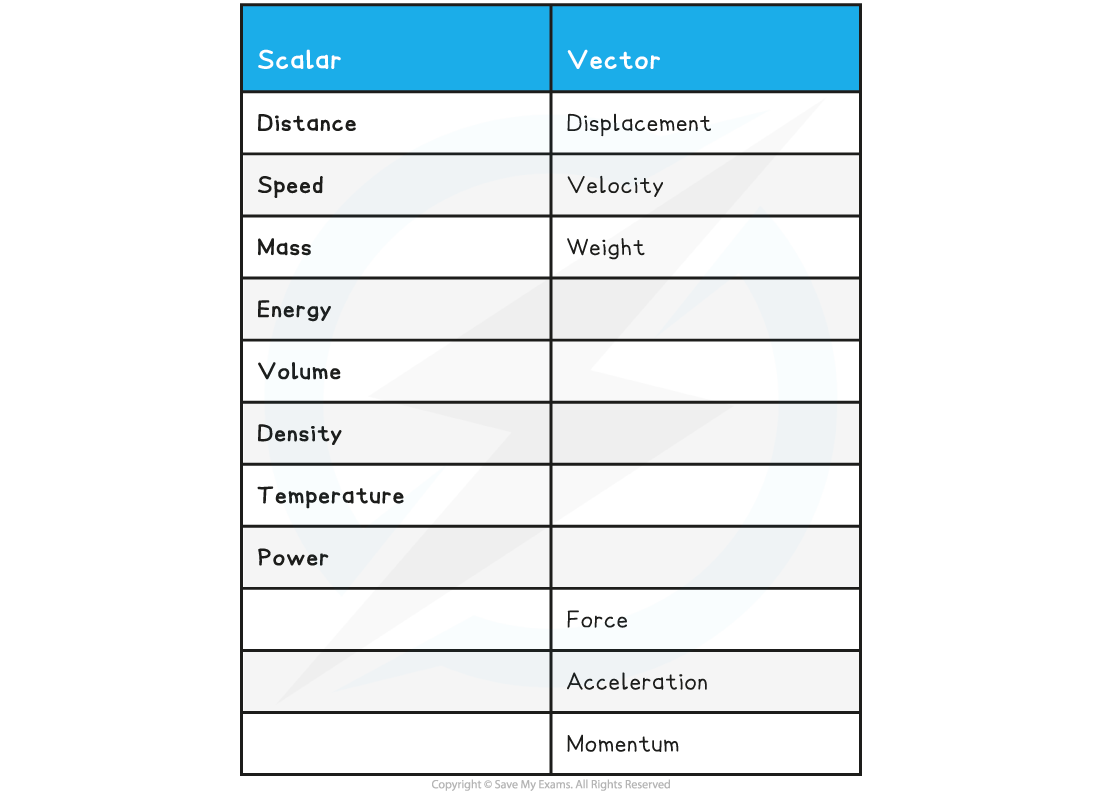
- Some vectors and scalars are similar to each other
- For example, the scalar quantity distance corresponds to the vector quantity displacement
- Corresponding vectors and their scalar counterparts are aligned in the table where applicable
Examiner Tip
Make sure you are comfortable with the differences between similar scalars and vectors, the most commonly confused pairings tend to be:
- Distance and displacement
- Speed and velocity
- Weight and mass
In my experience of teaching this topic to GCSE students, the most confusing aspect is the difference between distance and displacement. I always tell my students that distance is what your phone or watch measures when it counts the number of steps you take. You could be walking on a treadmill going nowhere but you still walk 2000 steps or 2 km. Displacement is the difference in position between where you started and where you ended up, so on a treadmill your distance would be 2 km, but your displacement would be zero.
Similarly, if you drew on a map the route you took to school, that would be your distance travelled. However, if you got a ruler and drew a straight line from your house to your school, that would be your displacement. Just remember that displacement has to include a direction as well as a magnitude. So your distance travelled to school maybe 2 km, but your displacement maybe 1.5 km north.
Representing Vectors
- A vector can be represented by using an arrow
- The length of the arrow represents the magnitude of the vector
- The direction of the arrow indicates the direction of the vector
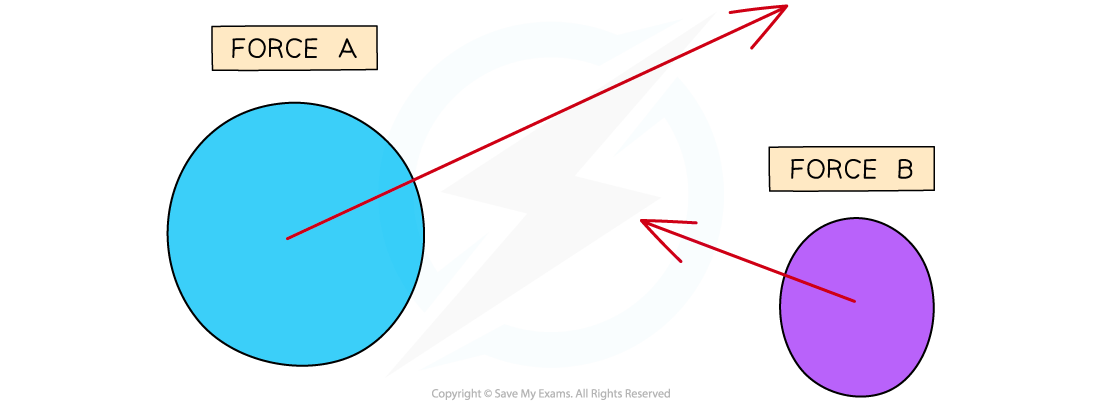
Two forces represented by arrows.
Force A has a larger magnitude than Force B. Force A is directed to the right and slightly upwards, whereas Force B is directed to the left and slightly upwards.Worked example
A tennis ball is thrown at an angle of 45° to horizontal at a speed of 5 m/s. A second tennis ball is thrown in the same direction at a speed of 10 m/s.Draw the velocity vectors of the balls.
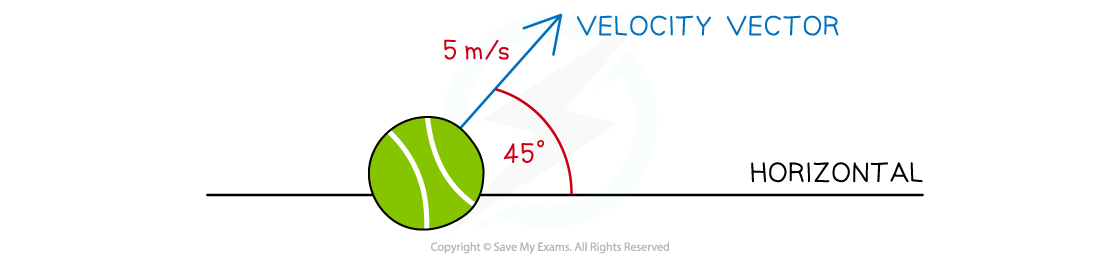
Step 1: Draw the first tennis ball and its velocity vector
- Measure the 45° angle with a protractor
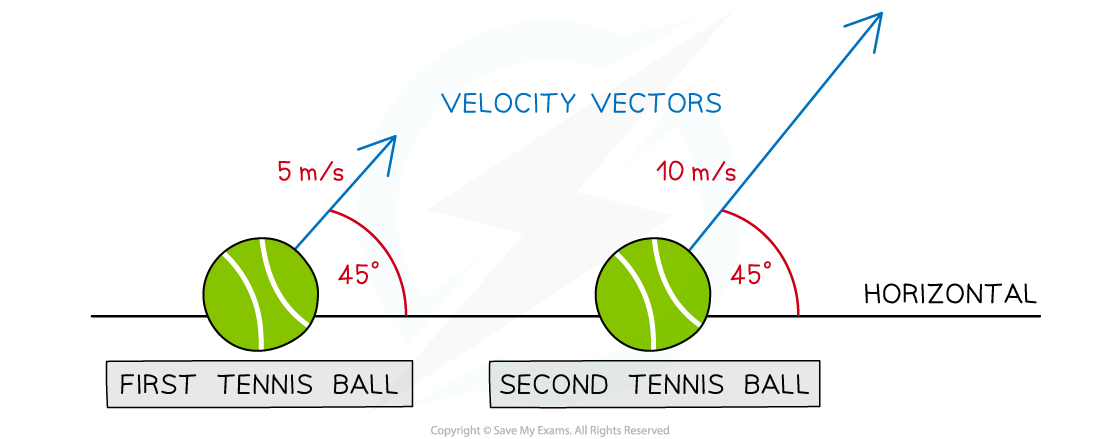
Step 2: Draw the second tennis ball and its velocity vector
- The second ball has a speed of 10 m/s, so the arrow will be twice as long
You've read 0 of your 10 free revision notes
Unlock more, it's free!
Did this page help you?