Time Series Graphs (Edexcel GCSE Maths) : Revision Note
Time Series Graphs
What is a time series graph?
A time series graph is sometimes called a line graph
A time series graph shows how a quantity (continuous data) changes over time
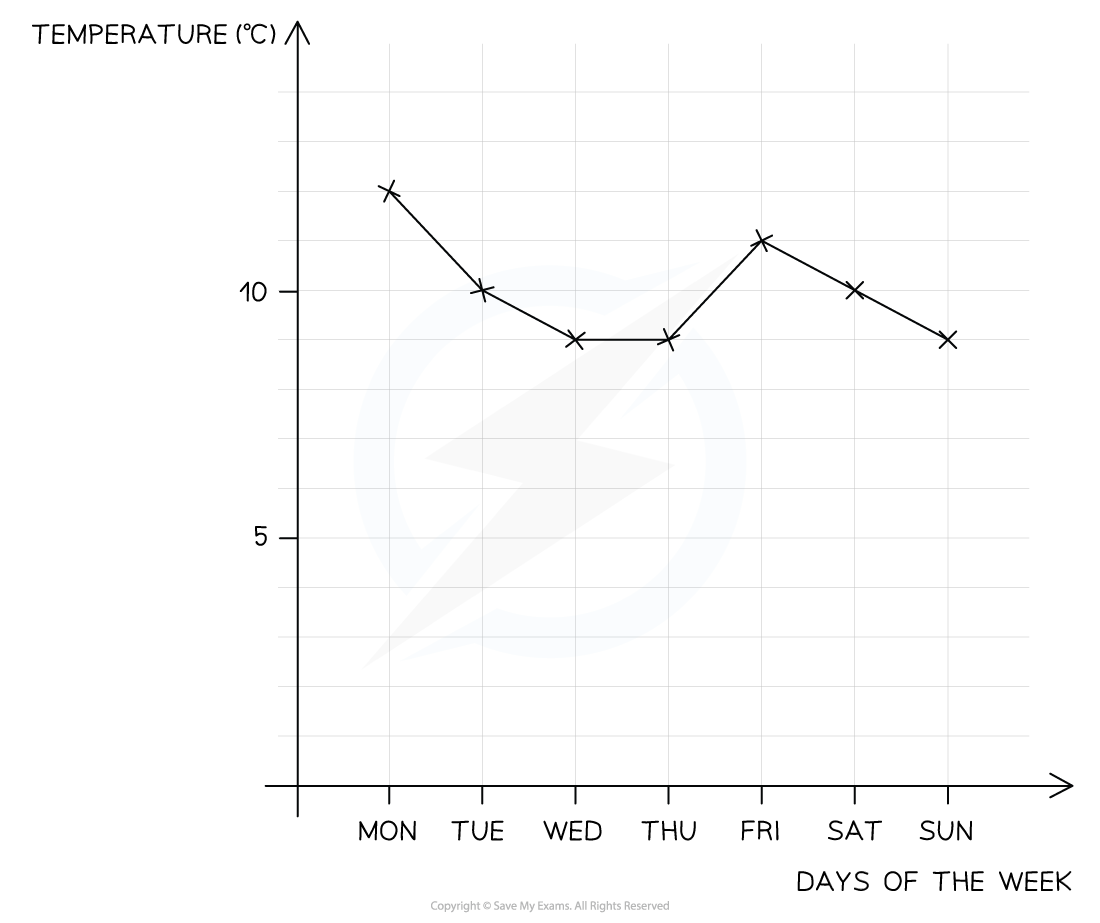
e.g. How the outside temperature changes during a week (shown below)
Measurements of the quantity are taken at particular times
Measurements should be taken at regular time intervals
These are then plotted as points on a time series graph and joined together with straight lines
The straight lines help us to identify patterns and features in the data
Time series graphs can show changes over short or long periods of time
e.g. Changes to the temperature of two chemicals for the first few minutes after they've been mixed
Or changes to the temperature of the earth over several years
How do I draw a time series graph?
The horizontal axis (x-axis) will be the time axis
The vertical axis (y-axis) will be the quantity being measured/recorded
Plot the data as a series of points
Join one point to the next, in order, with straight lines
Use a ruler
Sometimes a time series graph may have more than one data set
e.g. one line for car emissions and one line for motorbike emissions
Plot one data set and join the points up before moving on to the second data set
This will ensure you do not muddle the points up
You could use crosses (×) for one set of points, and dots (•) for the other
You could use different colours or dotted/dashed lines when joining the points up
Always include a key in such cases to make it clear which line is which data set
How do I use and interpret a time series graph?
This involves looking at patterns in the data as well as specific points
If a question asks you to interpret or describe a time series graph look for:
A general trend
e.g. The rate of inflation may fluctuate (go up and down) but is generally going down over a decade
Seasonal differences
e.g. Sales are higher in the summer months than winter months
Other things to look for
a horizontal line between points - no change (constant)
the steepest line (gradient) would indicate the greatest change
this could be an increase ('uphill' left to right, like /)
or a decrease ('downhill' left to right, like \)
Unusual 'one-off' readings - 'spikes' or 'dips'
e.g. A spike in mobile phone network activity at midnight on New Year's eve
For line graphs with two (or more) data sets, be clear about which line you are describing
Use the key
Double check which data set is 'higher' or 'lower' (or they may be equal) at a particular time
Examiner Tips and Tricks
If you are asked to describe or interpret a line graph then it may help to:
Draw vertical or horizontal lines on the graph from the respective time or measurement to ensure an accurate reading
Use a ruler to do this!
Highlight any particular points that you mention in your description
Worked Example
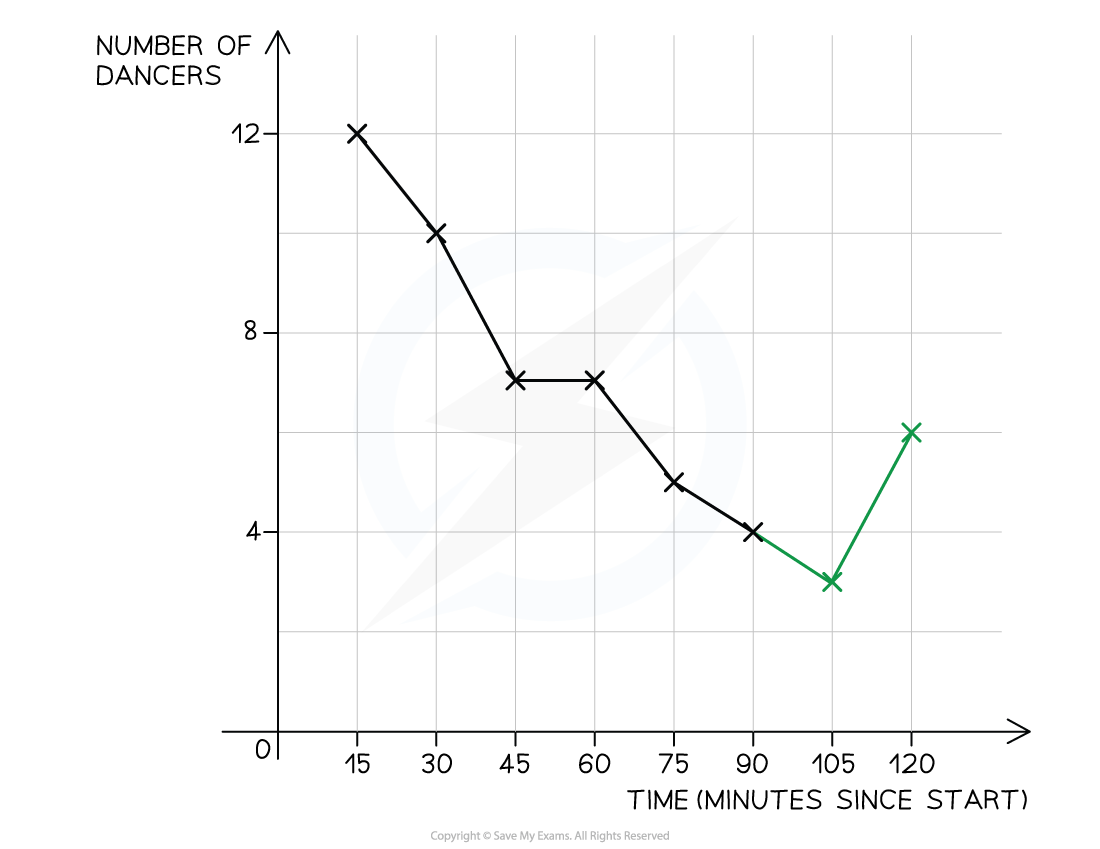
In a two-hour charity 'dance-a-thon' dancers can join and leave the dance floor as they choose.
The number of dancers on the dance floor is recorded every 15 minutes.
The times-series graph below shows the data for the first two hours of the 'dance-a-thon'.
At 1 h 45 m and 2 h the number of dancers were 3 and 6 respectively.
(a) Add these two recordings to the time-series graph.
Plot the points 105 minutes (1 h 45 m) on the time axis against 3 on the number of dancers axis and 120 minutes against 8 dancers.
(b) Use the time-series graph to find
(i) the time at which there were the fewest number of dancers,
(ii) the two times at which the number of dancers remained the same.
(i) Look for the lowest point on the graph, including those added in part (a)
The lowest point is (105, 3)
The final answer is the time
105 minutes (1 h 45 m)
(ii) The keyword here is remain - a horizontal line would indicate the number of dancers remaining the same
There is a horizontal line between 45 and 60 minutes
The number of dancers remained the same at 45 m and 60 m (1 h)
(c)
Comment on the general pattern of the number of dancers shown by the time-series graph.
Apart from the last point, and where it remained the same, the number of dancers decreased over the two hours.
In general, the number of dancers decreased during the first two hours of the 'dance-a-thon'

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
