Exact Trig Values (Edexcel GCSE Maths) : Revision Note
Did this video help you?
Exact Trig Values
What are exact values in trigonometry?
For certain angles the values of sin θ, cos θ and tan θ can be written exactly
This means using fractions and surds
You are expected to know the exact values of sin, cos and tan for
0°, 30°, 45°, 60°, 90°, 180° and their multiples
0º | 30º | 45º | 60º | 90º | |
|---|---|---|---|---|---|
sin | 0 | 1 | |||
cos | 1 | 0 | |||
tan | 0 | 1 |
|
How can I remember these exact trig values?
Look at patterns in the table
Note the values of sin θ from 0° to 90° match cos θ in reverse, from 90° to 0°
Some people remember sin θ using the trick
which simplifies to
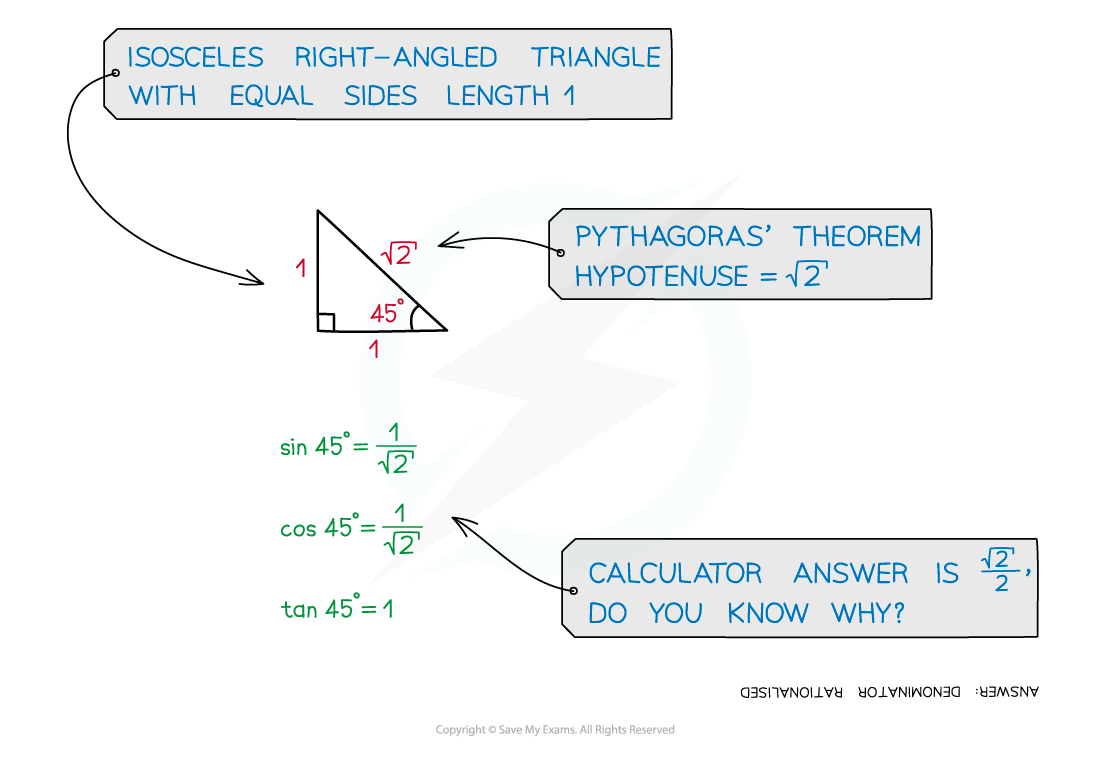
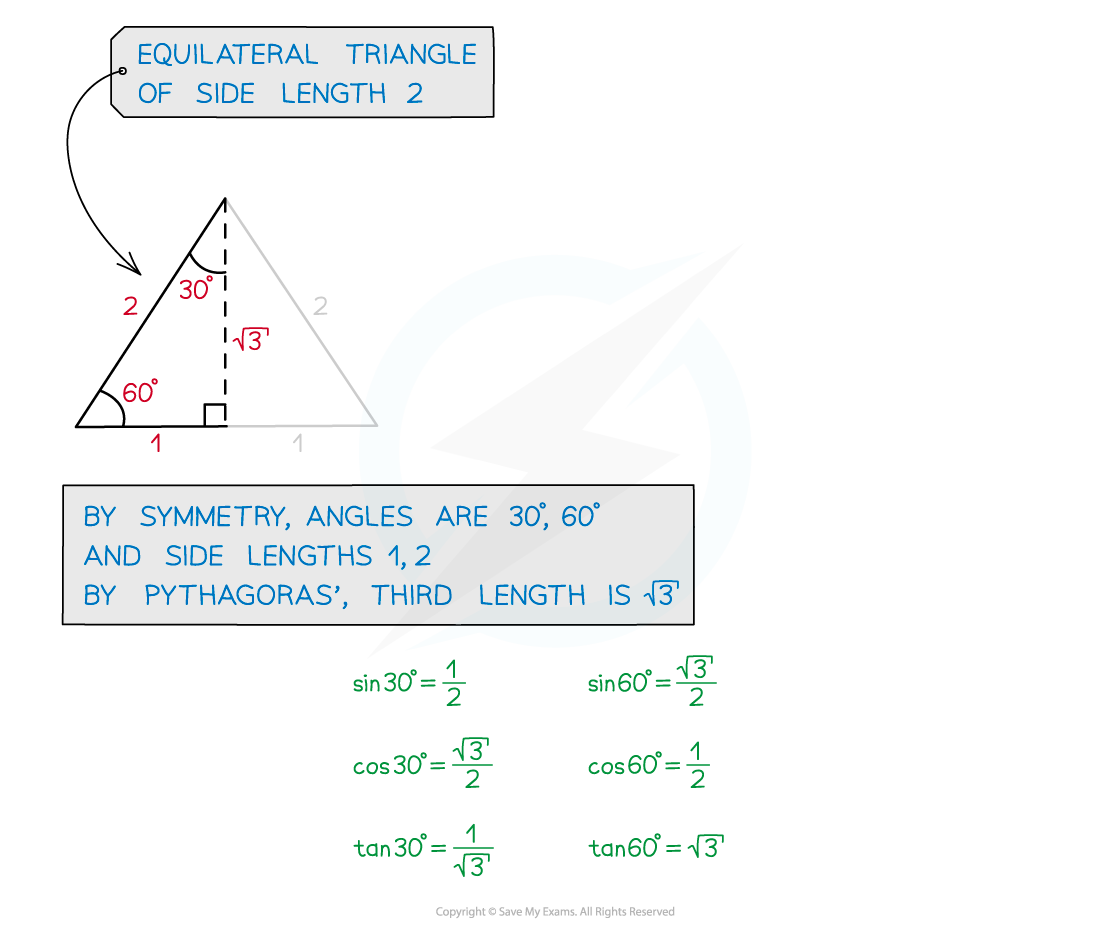
Two special right-angled triangles below can help you to find the exact values for 30º, 45º and 60º
Remember that by rationalising the denominator,
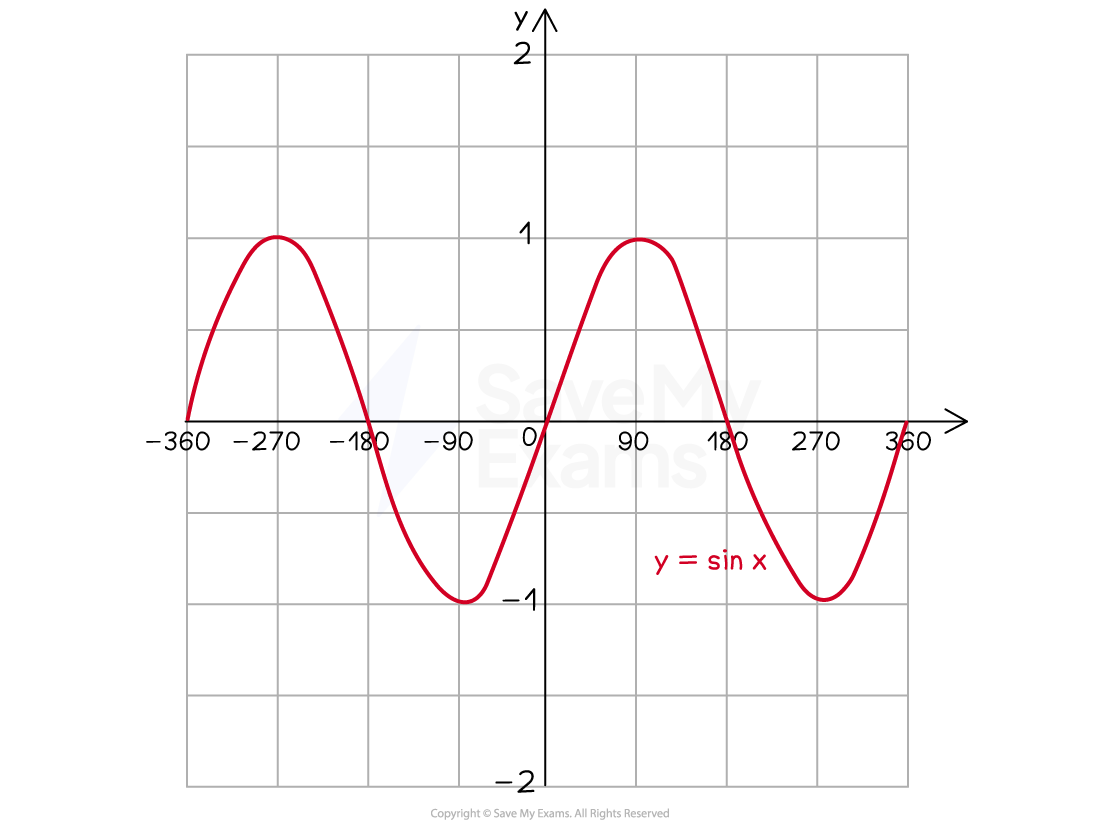
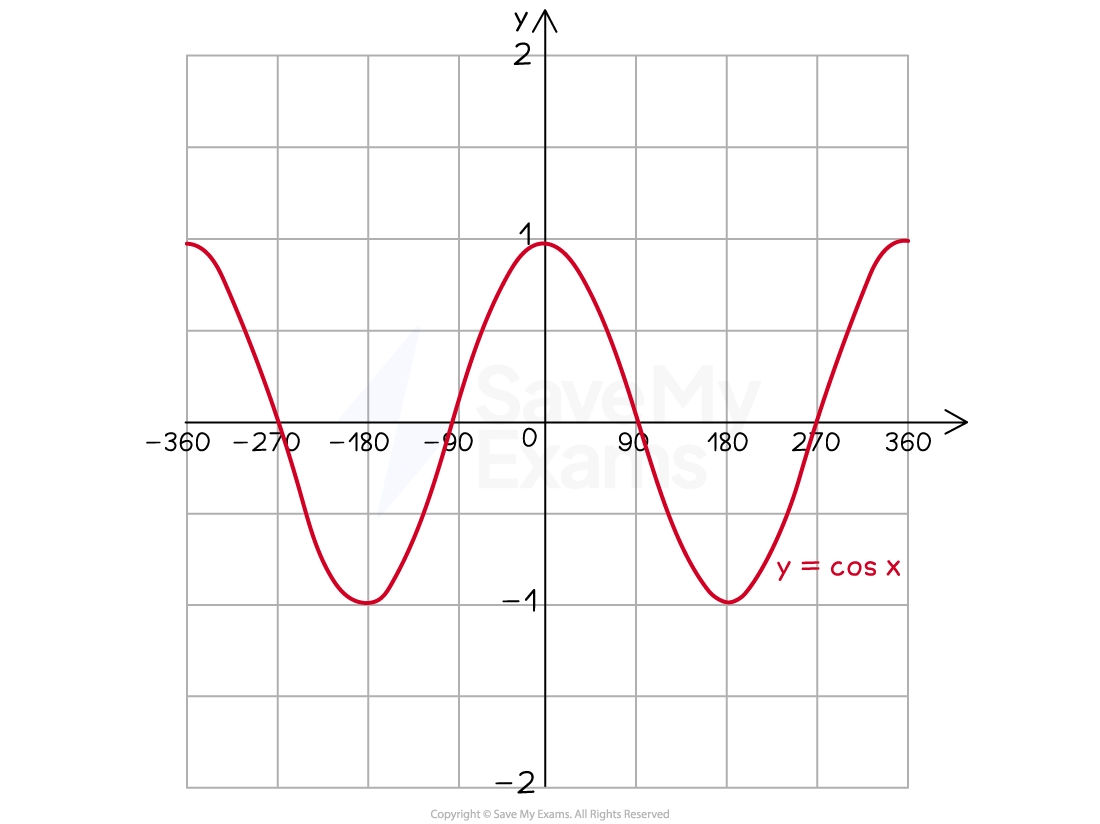
The trig graphs can help you to remember the exact values for 0º and multiples of 90º
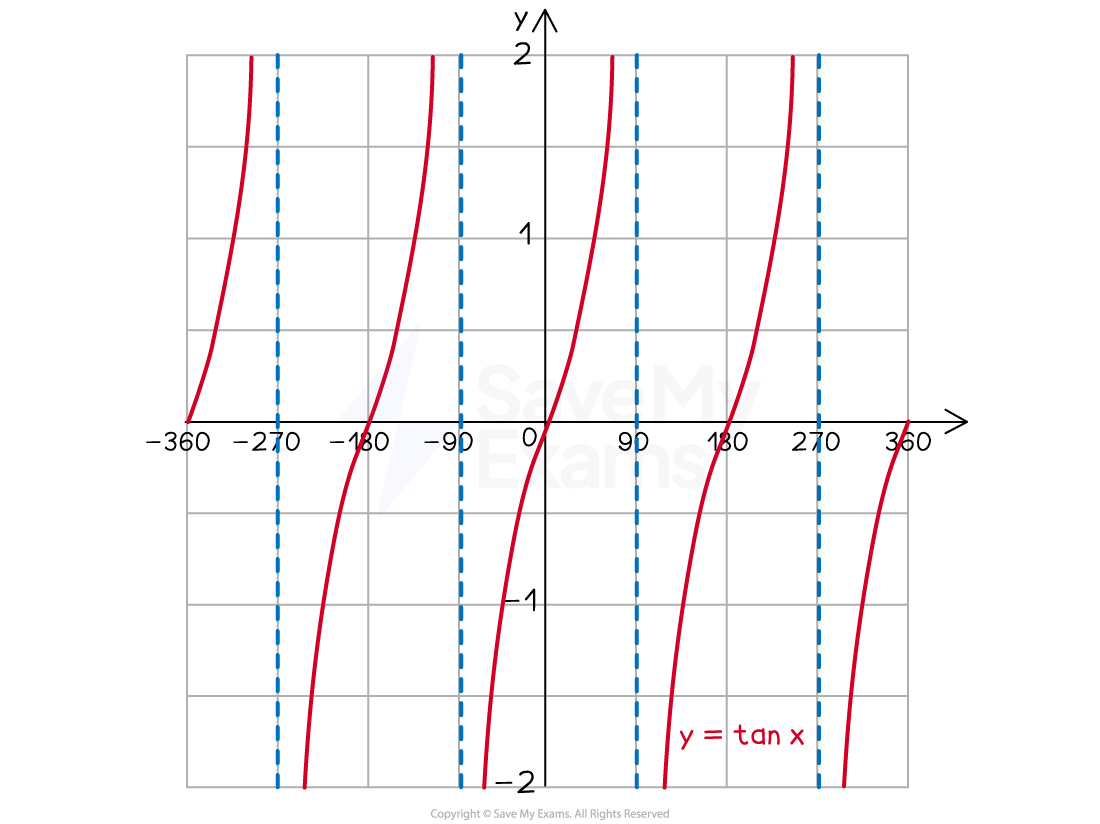
How do I use exact trig values?
You may come across trig questions in a non-calculator question
In trig calculations, substitute in the exact trig values and solve as usual
E.g. Solve the equation
Replace
with
to give
Then you can solve for
On trig graphs, you may be expected to find a coordinate
E.g. The coordinates
lie on the graph
, find
will be equal to
The exact value of
is
Therefore
Examiner Tips and Tricks
Writing these out (or sketching the triangles/graphs) on your paper at the beginning of the exam means that you can use them as many times as you need to during the exam!
Worked Example
Find the value of in the diagram below.
Give your answer as an exact value.
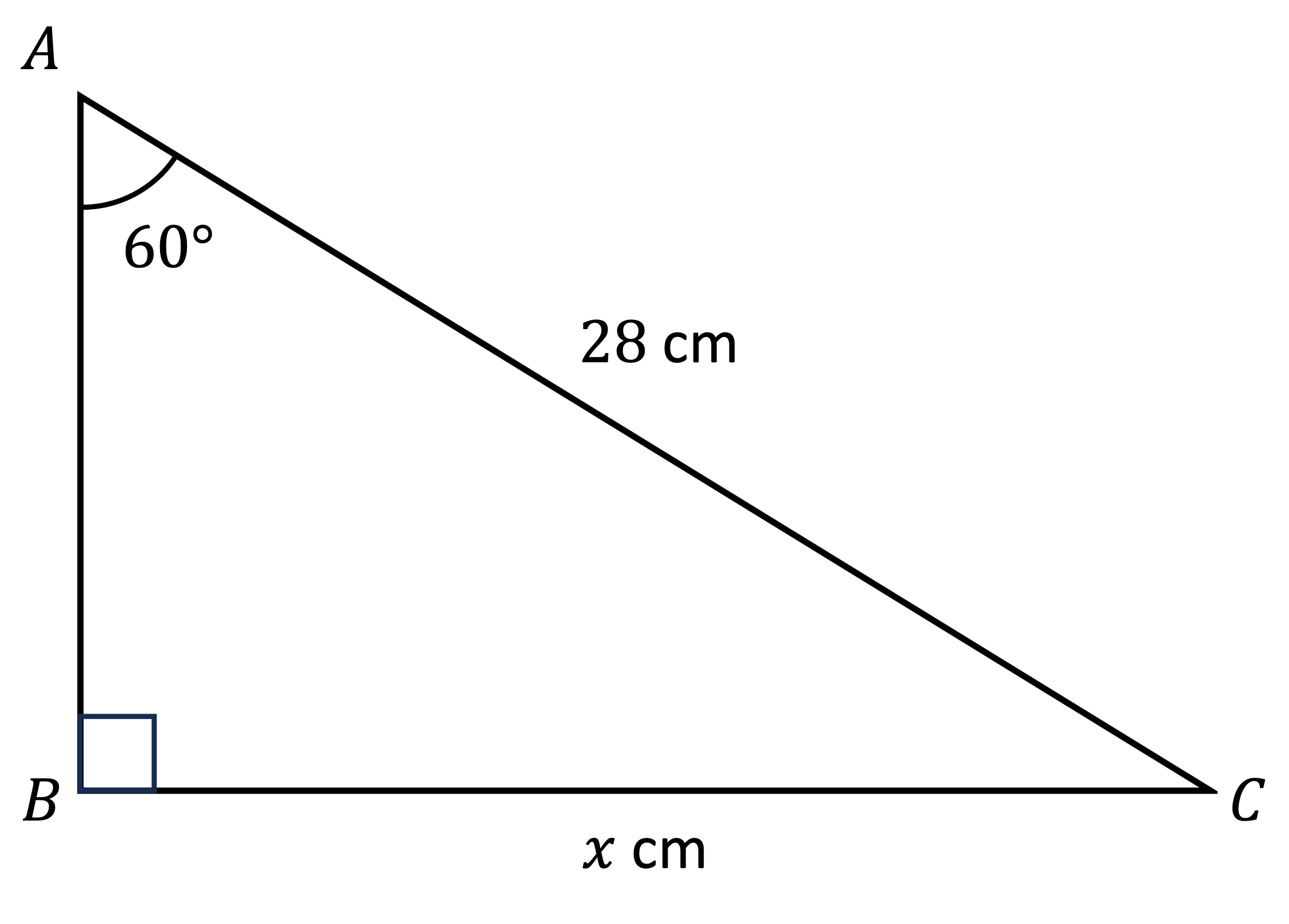
Triangle ABC is a right-angled triangle, so use SOHCAHTOA
We know the hypotenuse (AC) and we want to calculate the opposite (BC), so use
Remember that
So,
Leave in exact (surd) form
cm

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?

