Revision NotesExam QuestionsPast Papers
GCSEMathsEdexcelFoundationRevision NotesRatio, Proportion & Rates of ChangeStandard & Compound UnitsCompound Measures

Compound Measures (Edexcel GCSE Maths)

Revision Note

Naomi C

Written by: Naomi C

Reviewed by: Dan Finlay

Edexcel Maths: FoundationEdexcel Maths: Higher

Did this video help you?

Compound Measures

What is a compound measure?

  • A compound measure is something that is calculated by using more than one measurement

  • Compound measures can be used to measure rates

    • This measures how much one quantity changes when the other is increased by 1

    • Examples include:

      • Speed – how much the distance changes for each unit of time

      • Flow rate – how much the volume changes for each unit of time

      • Population density – how many people there are for each unit of area

      • Fuel consumption - volume of  fuel used for each unit of distance travelled

How do I find the units for a compound measure?

  • You can use the formula for a compound measure to derive its units

    • Use the units for the quantities in the formula to derive the units of the compound measure

    • Write a division as a/b or ab-1 and pronounce it as “a per b”

  • Examples include:

    • Speed space equals fraction numerator space Distance over denominator Time end fraction

      • If the distance is measured in km and the time is measured in minutes then the speed is measured in km/min or km min-1

    • Flow space rate space equals fraction numerator Volume space over denominator Time end fraction

      • If the volume is measured in m3 and the time is measured in minutes then the flow rate is measured in m3/min or m3min-1

How do I find the formula for a compound measure?

  • You can use the units for a compound measure to help remember its formula

    • You just need to remember what each unit measures

    • If the unit is a/b then the formula will be the quantity that a measures divided by the quantity that b measures

  • Examples include:

    • Density can be measured in kg/cm3

    • kg is a measure of mass and cm3 is a measure of volume

    • Therefore Density space equals space Mass over Volume

What is a formula triangle?

  • A formula triangle shows the relationship between the different measures in a compound formula 

    • E.g. for Speed, Distance and Time

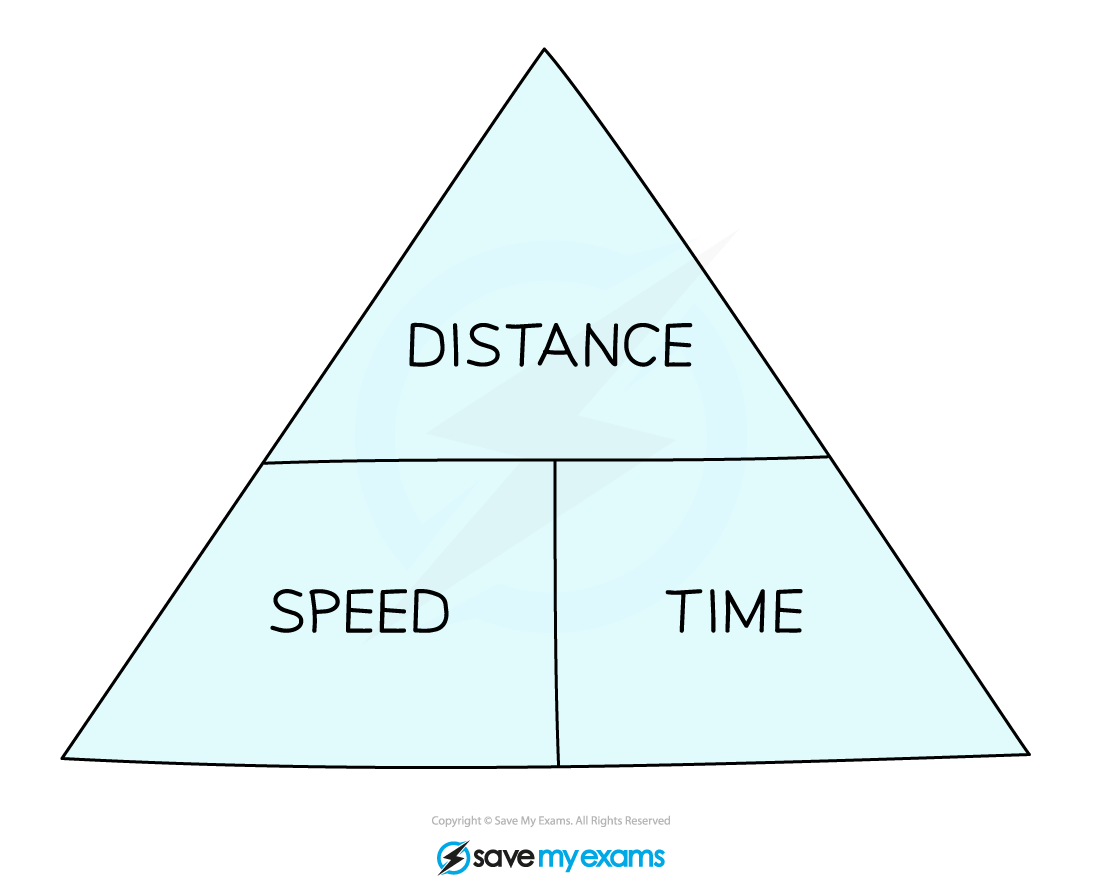
Formula triangle: Speed, Distance, Time

  • If you are calculating a variable on the top of the triangle, multiply the two variables on the bottom

    • For example,  Distance space equals space Speed space cross times space Time 

  • If you are calculating a variable on the bottom of the triangle, divide the top by the other variable on the bottom

    • For example,  Speed space equals space Distance space divided by space Time  and  Time space equals space Distance space divided by space Speed

Examiner Tips and Tricks

  • Check in the exam to see if the answer needs to be in different units

    • For example, the question may use metres and seconds but want the answer in km/h

  • You need to remember the relationship between speed, distance and time

Worked Example

A high-speed racing car has an average fuel consumption of 3 km per litre during a race.

1 lap of the racing circuit is 5.9 km in length.

(a) Calculate the volume of fuel used, in litres, to complete 15 laps of the circuit.

The units for the fuel consumption are km per litre, which suggests the formula is fuel space consumption space equals fraction numerator distance space over denominator volume end fraction

Calculate the total distance covered for the 15 laps

15 space cross times space 5.9 space km space equals space 88.5 space km

Use the above formula to find the volume of fuel, V litres, used

table row cell fuel space consumption space end cell equals cell fraction numerator distance space over denominator volume end fraction end cell row cell 3 space km space per space litre space end cell equals cell fraction numerator 88.5 space km space over denominator V space litres end fraction end cell end table

Rearrange the equation by multiplying both sides by V, and dividing both sides by 3

table attributes columnalign right center left columnspacing 0px end attributes row cell 3 V end cell equals cell 88.5 end cell row V equals cell fraction numerator 88.5 over denominator 3 end fraction equals 29.5 end cell end table

29.5 litres of fuel

The race car then requires a pit-stop to refuel to complete the final laps of the race.

The flow rate of the fuel pump is 720 litres per minute, and fuel is pumped into the car for 3.1 seconds.

(b) Calculate the volume of fuel, in litres, pumped into the car in this time.

The flow rate is 720 litres per minute which suggests the formula is flow space rate space equals fraction numerator volume space over denominator time end fraction

Before we can use the formula, we need to change the units of time to both be the same

Change 720 litres per minute, into litres per second (to match the time fuel is pumped for, which is in seconds)

If 720 litres are pumped in 1 minute, 60 times less will be pumped in 1 second

720 space divided by space 60 space equals space 12 space litres space per space second

Substitute these values into the formula

12 space litres space per space second space equals fraction numerator volume space in space litres over denominator 3.1 space seconds end fraction

Multiply both sides by 3.1

volume space in space litres space equals space 12 space cross times space 3.1 space equals space 37.2

37.2 litres

Last updated:

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Squared & Cubic UnitsNext:Speed, Density & Pressure
    Author
    Reviewer
    Naomi C

    Author: Naomi C

    Expertise: Maths

    Naomi graduated from Durham University in 2007 with a Masters degree in Civil Engineering. She has taught Mathematics in the UK, Malaysia and Switzerland covering GCSE, IGCSE, A-Level and IB. She particularly enjoys applying Mathematics to real life and endeavours to bring creativity to the content she creates.

    Dan Finlay

    Author: Dan Finlay

    Expertise: Maths Lead

    Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.