Surface Area (Edexcel GCSE Maths)

Surface Area

What is surface area?

• The surface area of a 3D object is the sum of the areas of all the faces that make up the shape

  • Area is a 2D idea being applied into a 3D situation

  • A face is one of the flat or curved surfaces that make up a 3D object

How do I find the surface area of cubes, cuboids, pyramids, and prisms?

• In cubes, cuboids, polygonal-based pyramids, and polygonal-based prisms (ie. pyramids and prisms whose bases have straight sides), all the faces are flat

• The surface area is found by

  • calculating the area of each individual flat face

  • adding these areas together

• When calculating surface area, it can be helpful to draw a 2D net for the 3D shape in question

  • For example, consider a square-based pyramid where the top of the pyramid is directly above the centre of the base

    • Its net will consist of a square base and four identical isosceles triangular faces

    • Calculate the area of a square and the area of each triangle then add them together

How do I find the surface area of a cylinder?

• A cylinder has two flat surfaces (the top and the base) and one curved surface

• The net of a cylinder consists of two circles and a rectangle

  

• The curved surface area (which is a rectangle) of a cylinder, A, with base radius, r, and height, h, is therefore given by

  • 

  • This is the circumference of the circle, multiplied by the height

  • This formula is not given to you in the exam

• The total surface area of a cylinder, A_Total, can be found using the formula

  • 

  • This is the area of the curved surface (a rectangle), plus two circles of radius r

  • This formula is not given to you in the exam

How do I find the surface area of a cone?

• A cone has one flat surface (the base) and one curved surface

• The net of a cone, with radius, r, perpendicular height, h, and sloping edge, (slant height), l, consists of

  • A circular base

  • A sector with radius, l, and an arc length equal to the circumference of the base

• The curved surface area of a cone, A, with radius, r, perpendicular height, h, and sloping edge, l, can be found using the formula

  • 

  • This formula is given to you in the exam if it is needed

• The total surface area of a cone, A_Total, can be found using the formula

  • 

  • This formula is not given to you in the exam

    • It is just the curved surface area formula above, plus the area of a circle

How do I find the surface area of a sphere?

• A sphere has a single curved surface

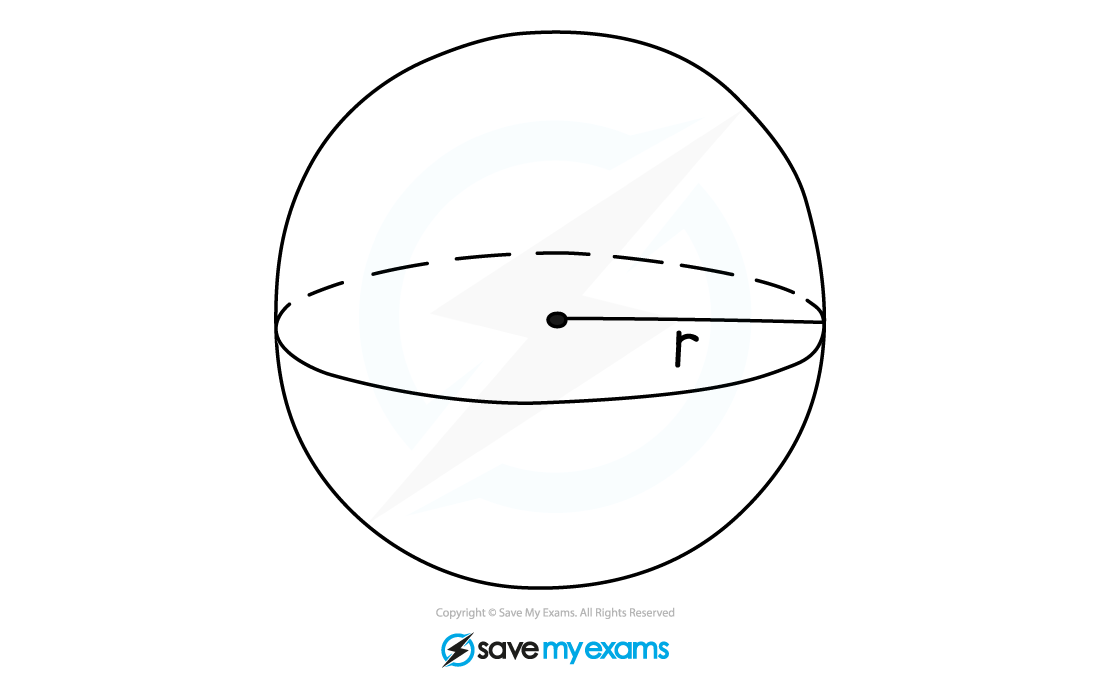
• The surface area of a sphere, A, with radius, r, can be found using the formula

  • 

  • This formula is given to you in the exam if it is needed