GCSEMaths: FoundationEdexcelRevision NotesGeometry & MeasuresTransformationsTranslations

Translations (Edexcel GCSE Maths: Foundation)

Revision Note

Test yourself
Dan

Author

Dan

Last updated

Transformations

What are transformations in maths?

  • There are four transformations in GCSE Maths
    • translations, rotations, reflections and enlargements
  • A transformation can change the position, orientation and/or size of a shape
    • The original shape is called the object
    • The transformed shape is called the image
  • Vertices are labelled to show corresponding points
    • Vertices on the object are labelled A, B, C, etc.
    • Vertices on the image are labelled A’, B’, C’ etc.

Translations

What is a translation?

  • A translation moves a shape
  • The size and orientation (which way up it is) of the shape stays the same
    • The object and image are congruent

What is a translation vector?

  • The movement of a translation is described by a vector
  • You need to know how to write a translation using a vector (rather than words)
  • Vectors are written as column vectors in the form  stretchy left parenthesis table row bold italic x row bold italic y end table stretchy right parenthesis  where:
    • x is the distance moved horizontally
      • Negative means move to the left
      • Positive means move to the right
    • y is the distance moved vertically
      • Negative means move down
      • Positive means move up

How do I translate a shape?

  • STEP 1

    Interpret the translation vector

    • open parentheses table row 3 row cell negative 1 end cell end table close parentheses  means 3 to the right and 1 down

  • STEP 2
    Move each vertex on the original object according to the vector

  • STEP 3
    Connect the new vertices and label the translated image

    • It should look identical to the original object just in a different position
  • In some cases the image can overlap the object

How do I describe a translation?

  • To describe a translation, you must:
    • State that the transformation is a translation
    • Give the column vector that describes the movement
  • To find the vector:
    • Pick a point on the original shape
    • Identify the corresponding point on the image
    • Count how far left or right (x) you need to go from the object to get to the image
      • If you go to the left then x will be a negative number
    • Count how far up or down (y) you need to go from the object to get to the image
      • If you go down then y will be a negative number
    • Write these numbers as a vector
      • open parentheses table row x row y end table close parentheses

Examiner Tip

  • The vector is how the shape moves not the size of the gap between the object and the image
    • Watch out for this common error!
  • Use tracing paper to check your answer

Worked example

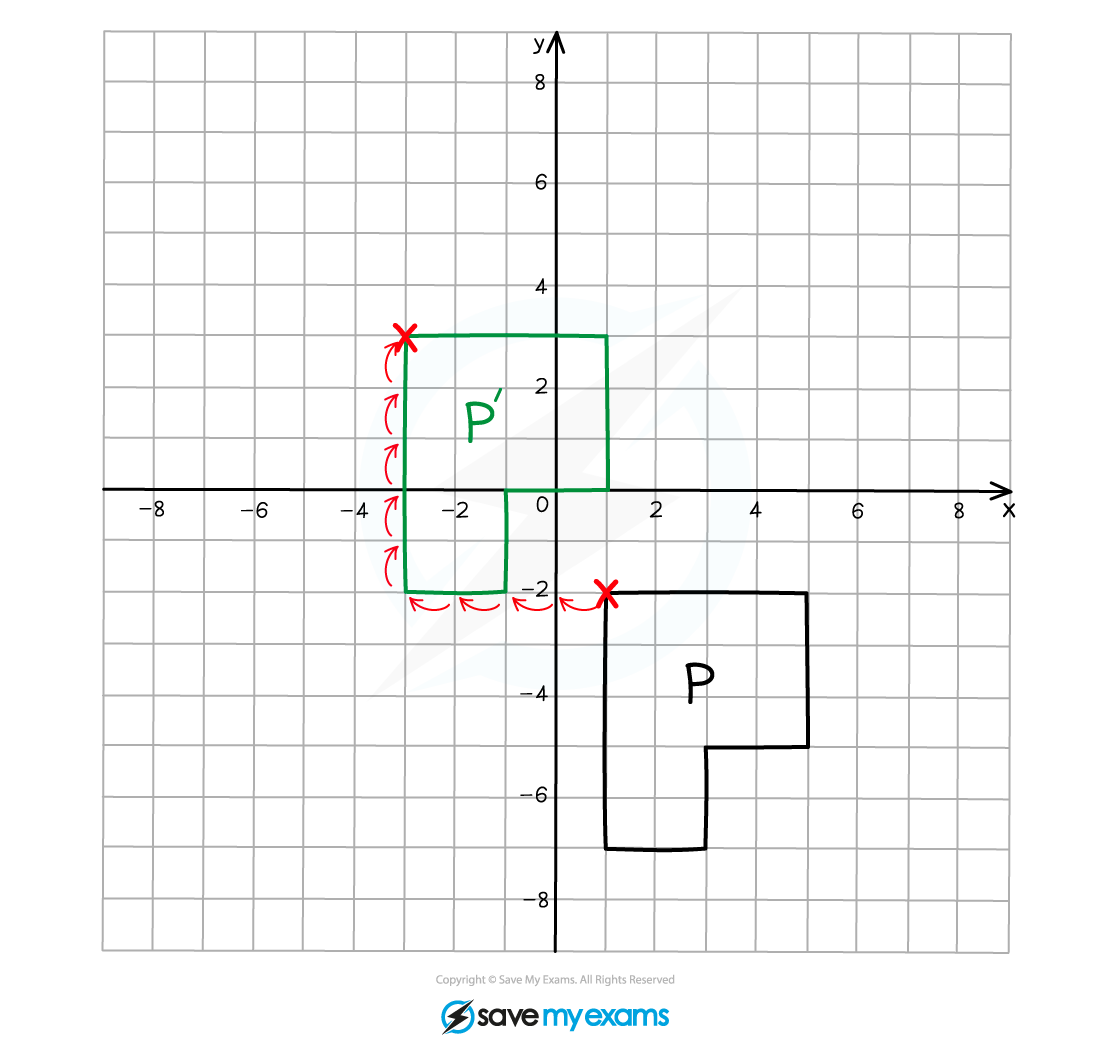
(a)table row blank row blank end table
On the grid below translate shape P using the vector open parentheses table row cell negative 4 end cell row 5 end table close parentheses.
Label your translated shape P'.
 

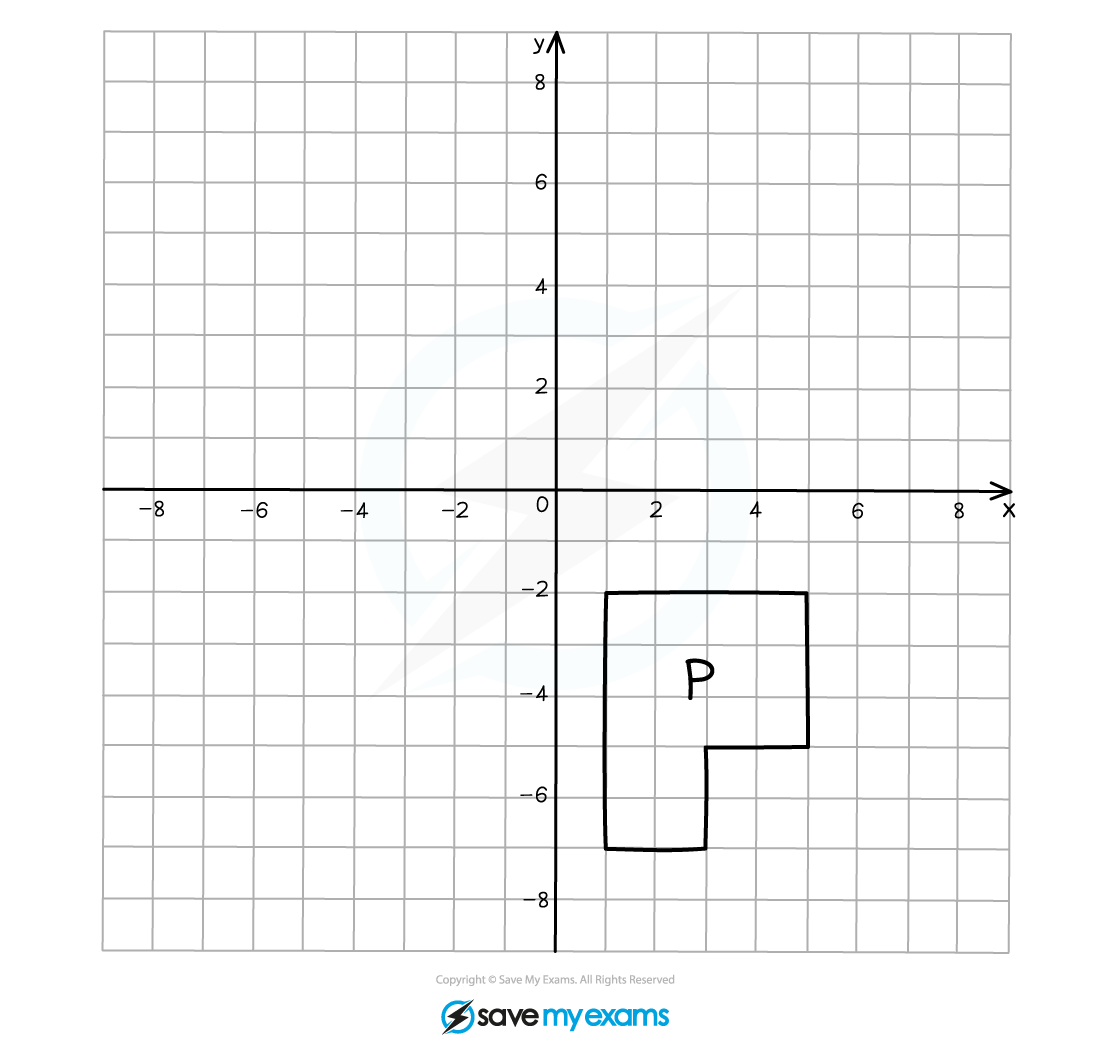
Grid showing an object P

The vector means "4 to the left" and "5 up"
You don't have to draw in any arrows but it is a good idea to mark your paper after counting across and up a couple of times to check that you are in the correct place

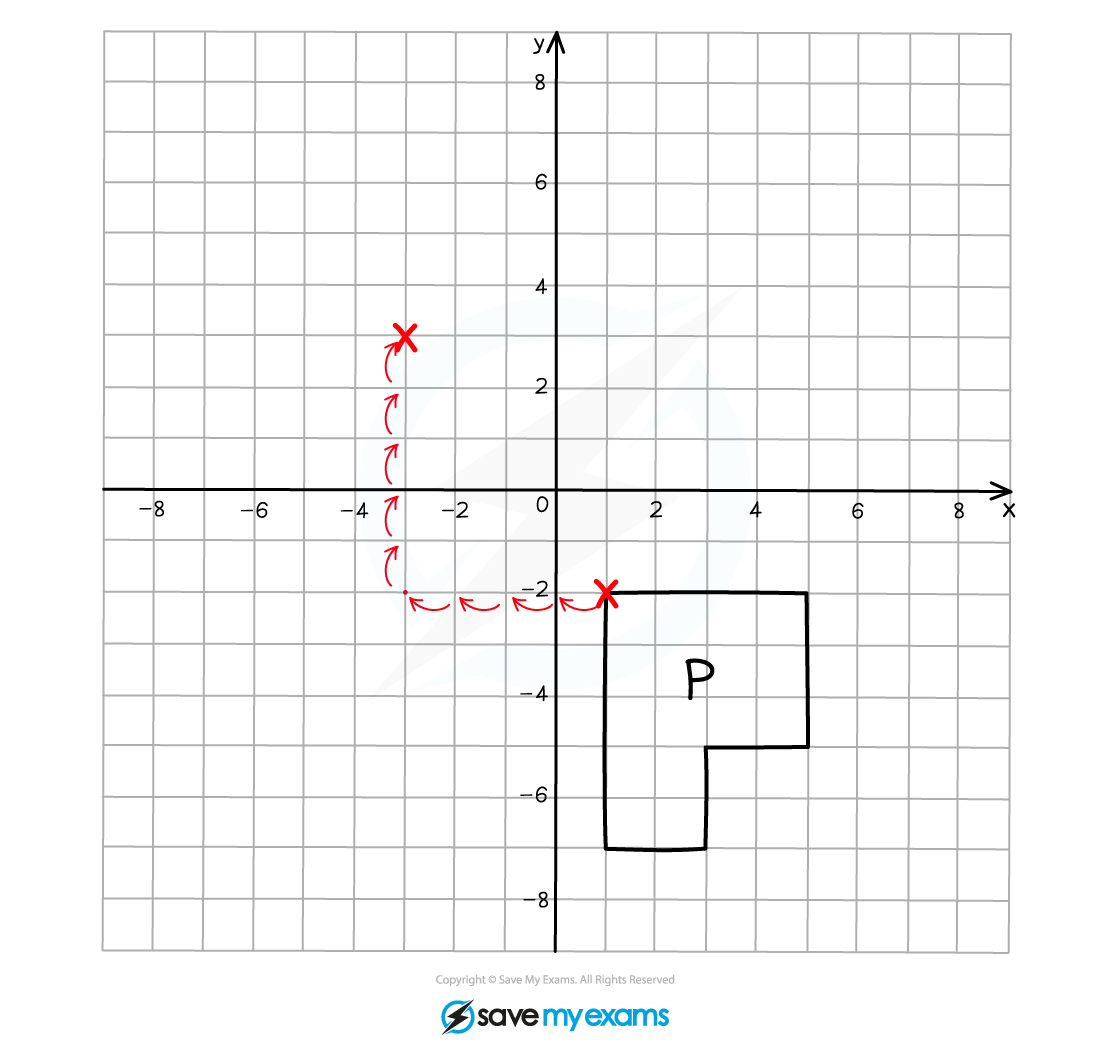
A grid showing the translation of a vertex on an object P

Translating one vertex and then following around the shape one vertex at a time makes it easier to get the shape in exactly the right position!

A grid showing an object P and its translated image P'

 

(b)
Describe fully the single transformation that creates shape B from shape A.

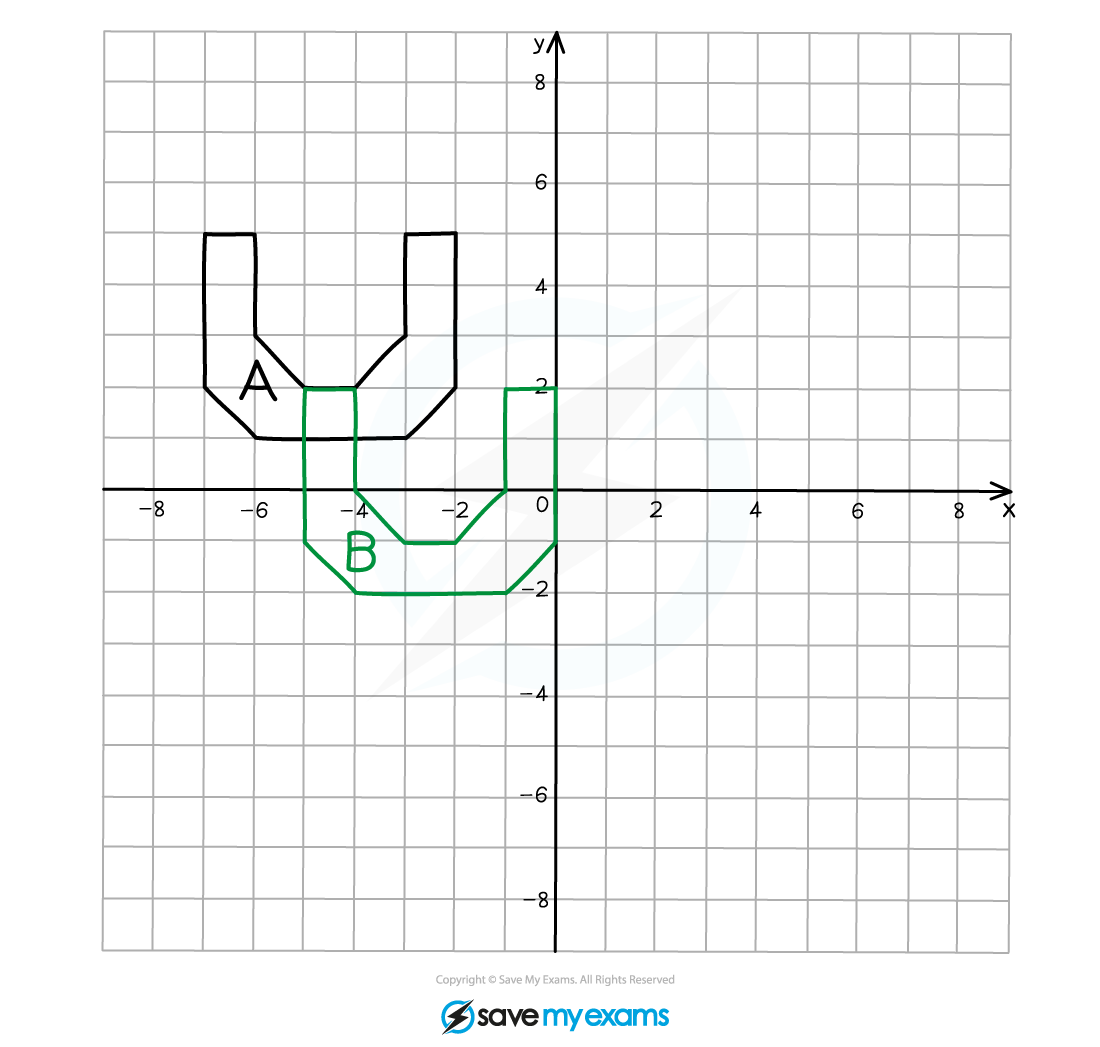
A grid showing an object A and its transformed image B

This is a case where the image overlaps the object
You should still see that the shape is the same size and the same way up so it is a translation
Start at a vertex on the original object that is well away from any overlap area to avoid confusion and count the number of position left/right and up/down that you need to move to reach the corresponding vertex on the translated image
Take care when counting around the axes!

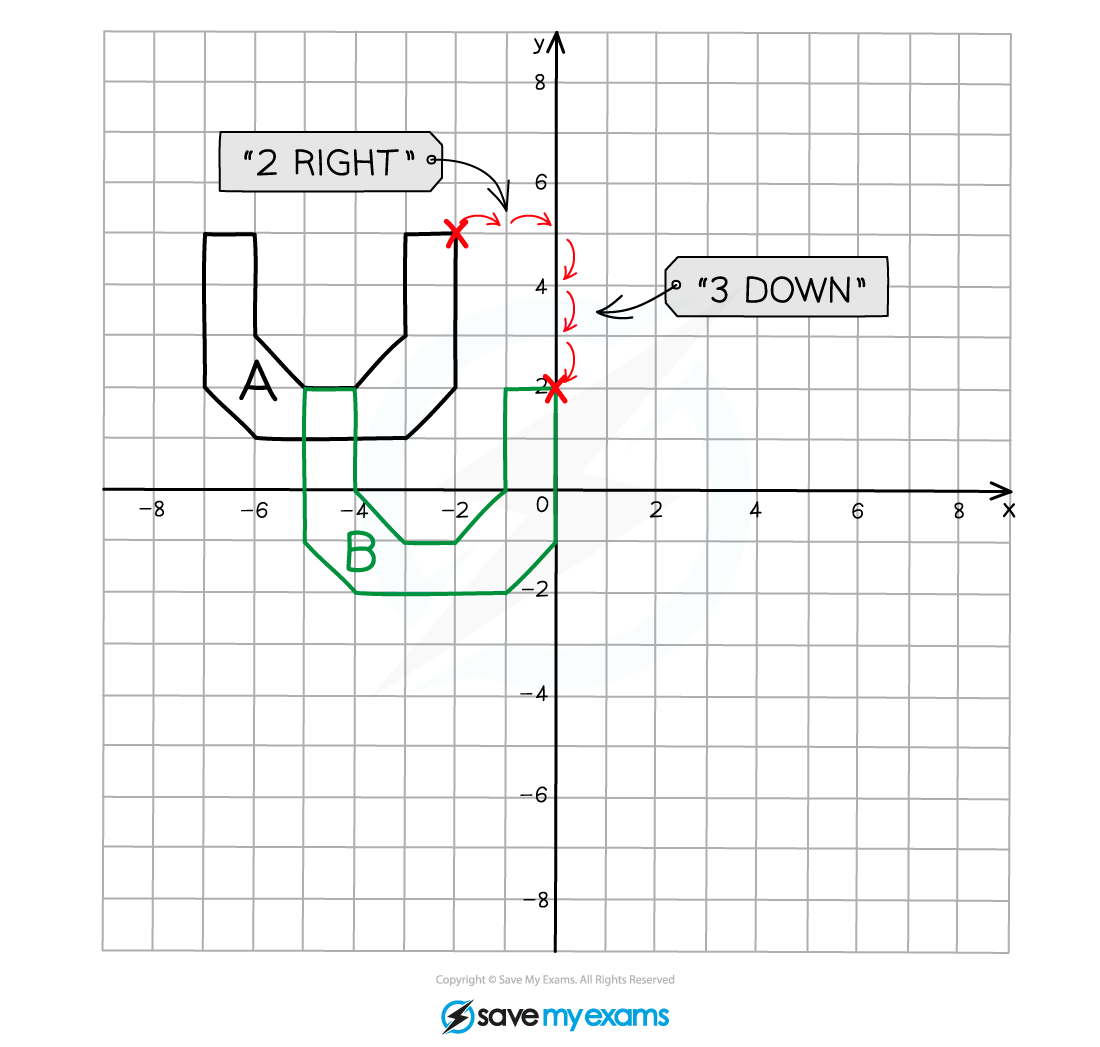
A grid showing the translation of a vertex between an object A and its image B

Shape A has been translated using the vector begin bold style stretchy left parenthesis table row 2 row cell negative 3 end cell end table stretchy right parenthesis end style

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourselfNext topic

    Did this page help you?

    Dan

    Author: Dan

    Expertise: Maths

    Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.