Reflections

What is a reflection?

A reflection flips a shape across a mirror line
- This is called the line of reflection
The reflected image is the same size as the original object
- It has been flipped across the mirror line to a new position and orientation
The following two distances will be equal for each point:
- The perpendicular distance between the original point and the mirror line
- The perpendicular distance between the reflected point and the mirror line
Any points that are on the mirror line do not move

How do I reflect a shape?

STEP 1
Draw the line of reflection
- This will usually be a vertical line ( $x = k$ ) or a horizontal line ( $y = k$ )
- A diagonal line will either be $y = x$ or $y = - x$
STEP 2
From each vertex on the original object measure the perpendicular distance to the mirror line
- You can usually do this by counting squares on the grid
- If the line is diagonal then count the diagonals of the squares
STEP 3
Find the reflected point by measuring the same distance in the same direction from the point on the mirror line
STEP 4
Join together the reflected points and label the reflected image

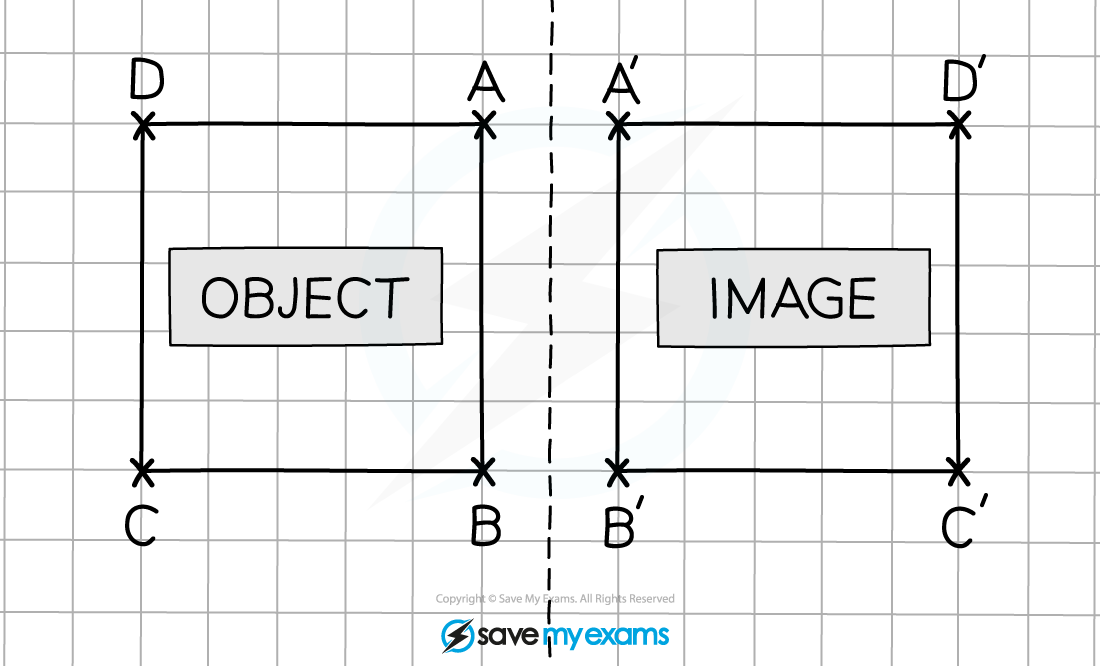
Reflection of a shape

How do I reflect a shape when the line of reflection goes through the shape?

You follow the same steps as above
Part of the shape gets reflected on one side of the mirror line, and the other part gets reflected on the other side

Reflection of a shape where the mirror line goes through the shape

How do I describe a reflection?

To describe a reflection, you must:
- State that the transformation is a reflection
- Give the mathematical equation of the mirror line
To find the equation of the reflection line:
- Horizontal lines are of the form $y = k$
  - $k$ is the number that the line passes through on the y-axis
- Vertical lines are of the form $x = k$
  - $k$ is the number that the line passes through on the x-axis
- A diagonal line with a positive gradient will be $y = x$
- A diagonal line with a negative gradient will be $y = - x$

Examiner Tip

It is very easy to muddle up the equations for horizontal and vertical lines, remember:
- If the line crosses the x-axis then it will be $x = k$
- If the line crosses the y-axis then it will be $y = k$
You can use tracing paper to check that your object has remained the same shape

Worked example

On the grid below, reflect shape S in the line

x = - 1

State the coordinates of all of the vertices of your reflected shape.

Draw in the mirror line;

x = - 1

will be a vertical line passing through -1 on the x-axis
Measure or count the number of units from the shape "diagonals" on the other side of the mirror line to find the position of the corresponding vertex on the reflected image

cie-igcse-core-reflections-rn-image

List the vertices of the reflected image.
Work your way around the shape vertex by vertex so that you don't miss any out as there are quite a few!

Vertices of the reflected shape: (1, 6), (2, 6), (2, 4), (3, 4), (3, 6), (4, 6), (4, 3), (3, 3), (3, 1), (2, 1), (2, 3), (1,3)

Describe fully the single transformation that creates shape B from shape A.

Refelction-Q2, IGCSE & GCSE Maths revision notes

You should be able to "see" where the mirror line should be without too much difficulty.
Draw the mirror line on the diagram.
You can check that it is in the correct position by measuring/counting the perpendicular distance from a pair of corresponding points on the original object and the reflected image to the same point on the mirror line.
Be careful with mirror lines near axes as it is easy to miscount.

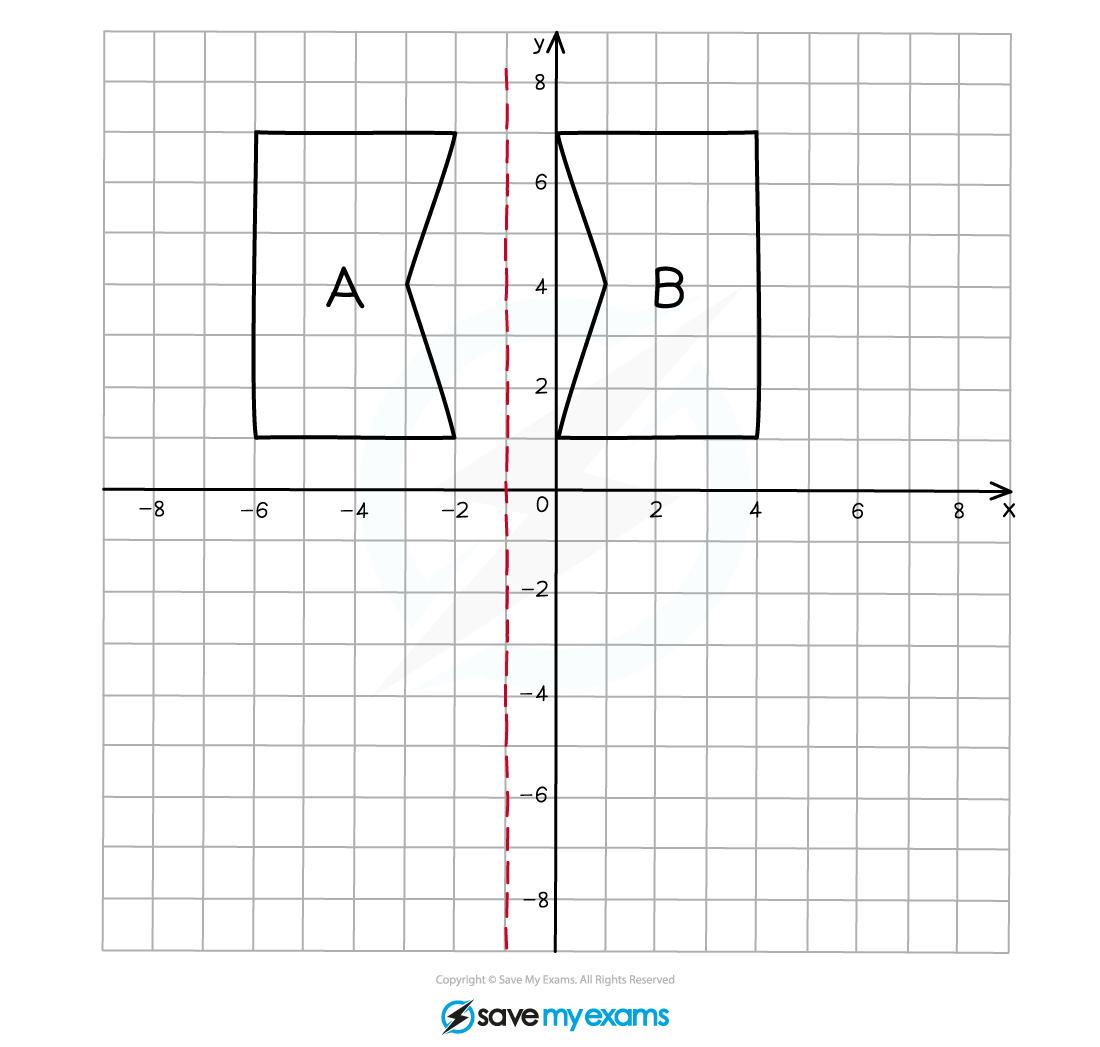
Reflection-Q2-working, IGCSE & GCSE Maths revision notes

Write down that the transformation was a reflection and the equation of the mirror line.

Shape A has been reflected in the line $x = - 1$ to create shape B

Reflections (Edexcel GCSE Maths: Foundation)

Revision Note

Reflections

What is a reflection?

How do I reflect a shape?

How do I reflect a shape when the line of reflection goes through the shape?

How do I describe a reflection?

Examiner Tip

Worked example

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Dan