Problem Solving with Areas

Adding & Subtracting Areas

What is a compound shape?

Sometimes you will have a shape that is not a standard shape such as a rectangle, triangle, trapezium etc.
- These are often called compound shapes
- We can split the non-standard shape into standard shapes

How do I find the area of a compound shape?

Split the compound shape into standard shapes
Find the areas of the standard shapes
Add these areas together to find the area of the compound shape
Occasionally it may be easier to add an extra shape to the diagram and subtract the area of the extra shape from the new bigger shape
- For the shape below you might complete the rectangle by putting a triangle in the top right corner
- The area of the original shape is the rectangle minus the triangle

IGCSE & GCSE Maths revision notes

Examiner Tip

Take a moment to think about how to split up the shape into the easiest shapes possible – there will probably be more than one way to do it!

Worked example

Find the area of the pentagon shown in the diagram below.

Pukoyl43_edexcel-3-5-2-adding-and-subtracting-areas-we-diagram

Separate the diagram into two shapes that you are familiar with and know the area formulae for
This pentagon can easily be split into a rectangle and a triangle

Use the values given to find the length of the base and the height of the triangle and add these to the diagram.

A compound shape split into standard shapes

The total area will be the area of the rectangle + the area of the triangle.

$\begin{array}{rcl} Total area & = & (12 \times 4) + \frac{1}{2} (7 \times 5) \\ = & 48 + \frac{1}{2} (35) \end{array}$

Area = 65.5 cm²

What is problem solving?

Problem solving, usually has two key features:
- A question is given as a real-life scenario
  - eg. Mary is painting a bedroom in her house...
- There is usually more than one topic of maths you will need in order to answer the question
  - eg. Area and percentages

What are common problems that involve area?

Area is a commonly used topic of 'real-world' maths
- For example, laying a carpet, painting a house or designing a sports field all involve area
Typically, the 'real-world' scenarios also have a cost
- A lot of area problems also involve calculations with money

How do I solve problems that involve area?

There is often a lot of text in a problem solving question, which can make it seem harder than it is
- Avoid focusing only on what the question asks you, think about what you can do with the information given
  - This may lead you to think of something else you can do
  - Eventually you may be able to see your way to answering the original question
- Think about the context of the question, what makes sense?
Look out for key information in the text:
- Real-life context
  - A question involving the size of a field, will mean be talking about its area
- Key words
  - Types of measure: area, length, cost, ...
  - Conditions: minimum, maximum, greatest, ...
- Units
  - You may see compound units, e.g. $/m² , these may help you to identify calculations that you need to do
Annotate diagrams with information that you can work out
- Remember to do this in pencil in case you need to erase it!
Problem solving questions could appear on either a non-calculator paper or a calculator paper

Examiner Tip

Even if you never get to a final answer always try to do some maths with the information from the question.
- You are likely to score some extra marks from your working!

Worked example

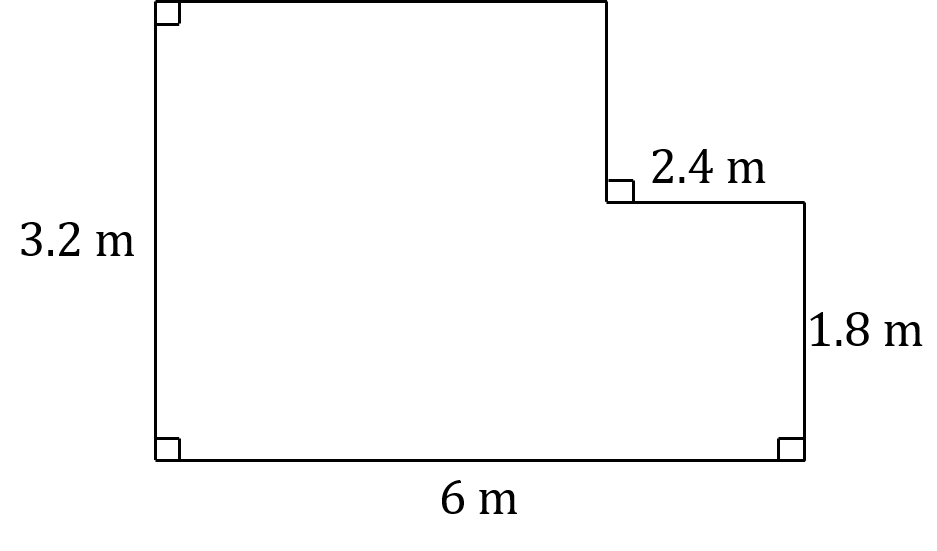
John wants a new carpet for the lounge in his house.

A sketch of his lounge is given below.

Compound shape made up of two rectangles

He gets quote from two local companies, Company A and Company B.

The amount they charge for laying a carpet is given below.

Company A: Fixed price of $5.50 per square metre
Company B: $6 per square metre for the first ten square metres, then $4 per square metre for anything over that.

Which company should John choose in order to keep the cost of laying the carpet to a minimum?

Although this question doesn't specifically tell you you need to find the area, it is implied as the costs both use 'square metre'

The shape of the lounge is a compound shape consisting of two rectangles
Split the area into these two rectangles and find the missing distances by subtracting the smaller length (2.4 m) from the longer one (6 m)

6 - 2.4 = 3.6

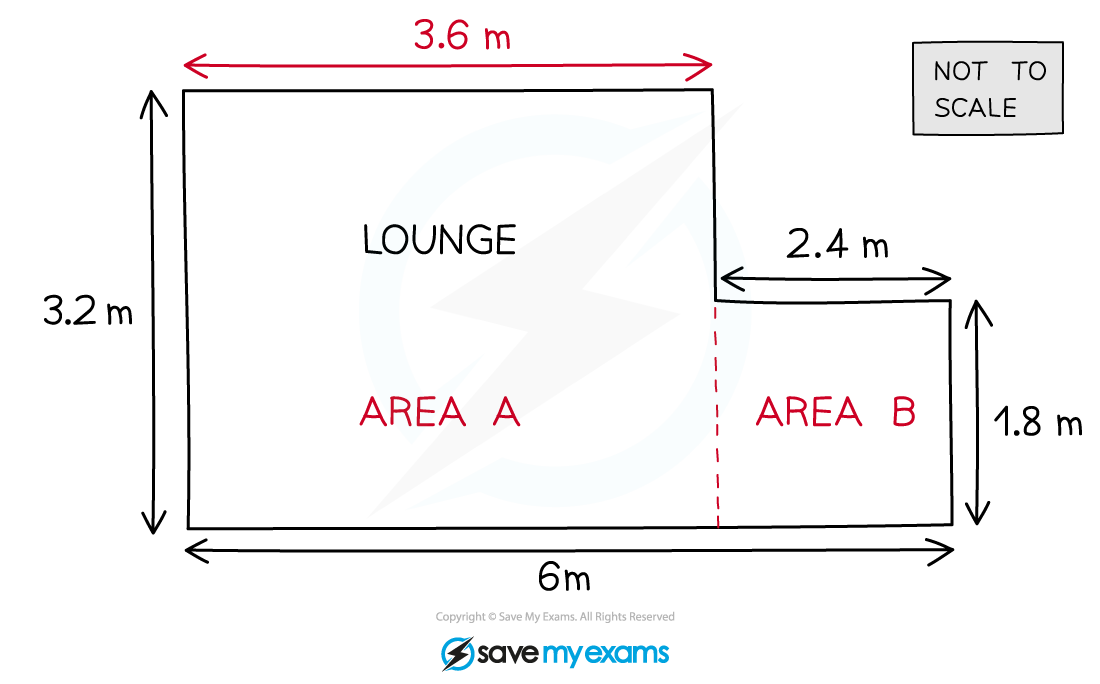
Lounge-Floor-Area, downloadable IGCSE & GCSE Maths revision notes

Find the area of the lounge by adding the two areas together

$\begin{array}{rcl} Total Area & = & Area A + Area B \\ = & (3.2 \times 3.6) + (2.4 \times 1.8) \\ = & 11.52 + 4.32 \\ = & 15.84 m^{2} \end{array}$

Find the cost for each of the two companies separately

Company A:

$\begin{array}{rcl} Total Cost & = & 15.84 \times 5.50 \\ = & $ 87.12 \end{array}$

Company B:

$\begin{array}{rcl} Total Cost & = & $ 6 \times first 10 m^{2} + $ 4 \times remaining \\ = & (6 \times 10) + 4 \times (15.84 - 10) \\ = & 60 + 23.36 \\ = & $ 83.36 \end{array}$

John should choose Company B as it will cost $3.76 less than Company A

Problem Solving with Areas (Edexcel GCSE Maths: Foundation)

Revision Note

Adding & Subtracting Areas

What is a compound shape?

How do I find the area of a compound shape?

Examiner Tip

Worked example