Angles in Parallel Lines

Parallel lines are lines that are always equidistant (the same distance apart)
- No matter how far the lines are extended in either direction, they will never meet
Angles are formed when a straight line cuts through two parallel lines

Corresponding angles

Alternate angles

Co-interior angles

Look for shapes that look like F, Z, or C
Vertically opposite angles can also be used in problems involving parallel lines
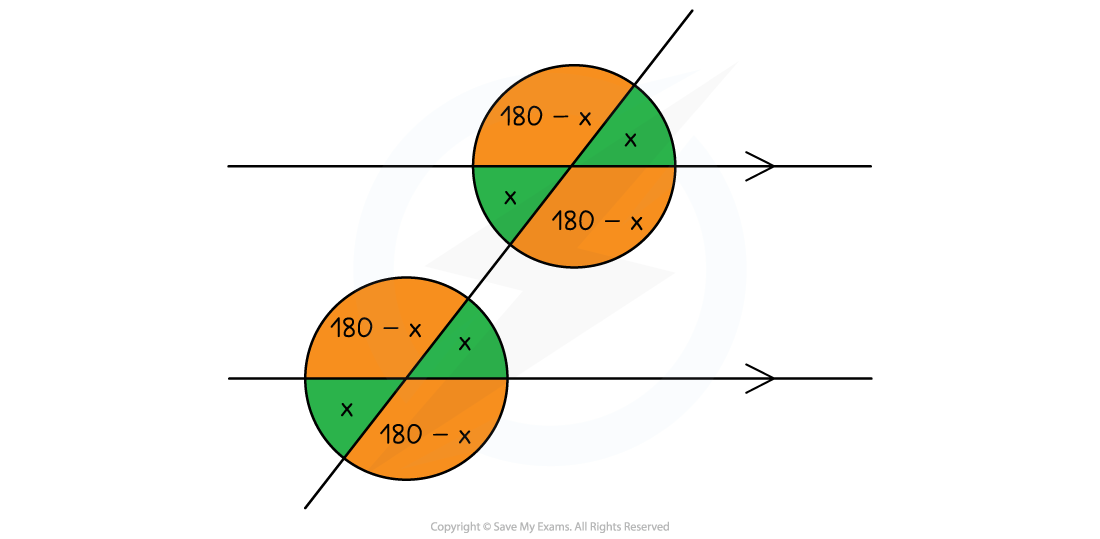
- The below diagram shows how identifying angle x, can lead to knowing information about several other angles
  - The green angle opposite is also x, as it is vertically opposite
  - The orange angle must be 180-x as angles on a straight line sum to 180°
You should also be able to spot corresponding angles, alternate angles, and co-interior angles in this diagram

vertically opposite angles and parallel lines

In some problems you may not be told directly that two lines are parallel
The problem may instead involve a geometric shape
You should know that:
- Parallelograms, rhombuses, rectangles, and squares have 2 pairs of parallel sides
- Trapeziums have 1 pair of parallel sides
Once the parallel lines are identified, use corresponding, alternate, and supplementary angles to find any missing angles

Do not forget to give reasons for each step of your working in an angles question
- These are often needed to get full marks
- You must use the correct names as listed above
  - Do not use F, Z and C angles otherwise you will lose marks!

Find the size of the angles a and b in the diagram below.

Give a reason for each step in your working.

Angles in Parallel Lines Worked Example question

Vertically opposite angles are equal.
Corresponding angles on parallel lines are equal.

You must write down both of these reasons for full marks.

a = 64° (Vertically opposite angles are equal)
b = 64° (Corresponding angles on parallel lines are equal)

Angles in Parallel Lines (Edexcel GCSE Maths: Foundation)