Did this video help you?
HCF & LCM (OCR GCSE Maths)
Revision Note
Highest Common Factor (HCF)
What is the highest common factor (HCF) of two numbers?
- A common factor of two numbers is a number that both numbers can be divided by
- 1 is a common factor of any two numbers
- To find common factors you can write out the factors of each number and identify the numbers that appear in both lists
- The factors of a common factor of two numbers will also be common factors
- 6 is a common factor of 24 and 30
- Therefore 1, 2, 3, 6 are also common factors of 24 and 30
- The factors of a common factor of two numbers will also be common factors
- The highest common factor is the largest common factor between two numbers
- This is useful when simplifying fractions or factorising expressions
How do I find the highest common factor (HCF) of two numbers?
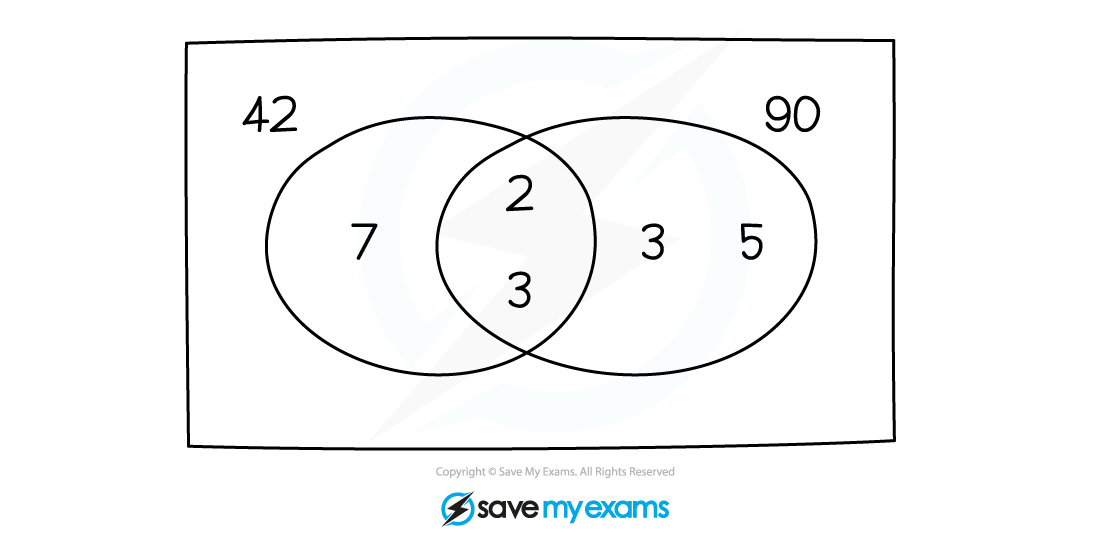
- Write each number as a product of its prime factors
- Find the prime factors that are common to both numbers
- Be careful with how many times a prime factor appears
- 12 = 2 × 2 × 3 and 10 = 2 × 5
- So only one of the 2s is a common prime factor
- Be careful with how many times a prime factor appears
- Multiply the common prime factors together
- Using a Venn diagram can help
- Put the common prime factors in the centre
- Put the other prime factors in the relevant circles
- The HCF is the product of all the numbers in the centre
Worked example
Find the highest common factor of 42 and 90.
Write as a product of prime factors
42 = 2 × 3 × 7
90 = 2 × 3 × 3 × 5
Write the prime factors in a Venn diagram if needed.
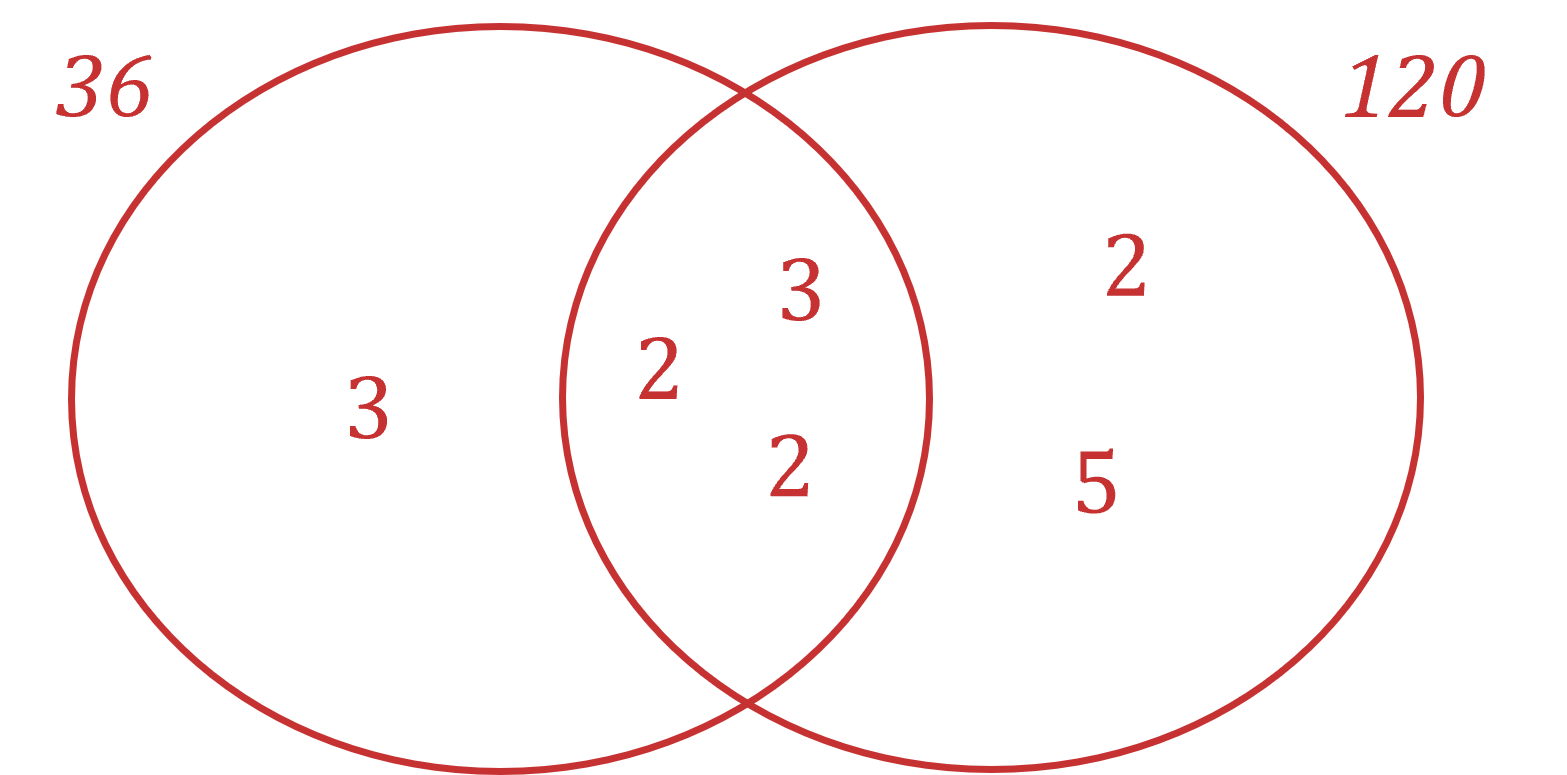
Multiply the common prime factors.
HCF = 2 × 3
HCF = 6
Lowest Common Multiple (LCM)
What is the lowest common multiple (LCM) of two numbers?
- A common multiple of two numbers is a number that appears in both of their times tables
- The product of the two numbers is always a common multiple
- To find common multiples you can write out the multiples of each number and identify the numbers that appear in both lists
- The multiples of a common multiple of two numbers will also be common multiples
- 60 is a common multiple of 12 and 10
- Therefore 60, 120, 180, 240, etc are also common multiples of 12 and 10
- The multiples of a common multiple of two numbers will also be common multiples
- The lowest common multiple is the smallest common multiple between two numbers
- This is useful when adding or subtracting fractions
How do I find the lowest common multiple (LCM) of two numbers?
- Write each number as a product of its prime factors
- Find the prime factors of the first number that are not prime factors of the second number
- Be careful with how many times a prime factor appears
- 12 = 2 × 2 × 3 and 10 = 2 × 5
- So 3 and one of the 2s are not prime factors of the 10
- Equivalently 5 is not a prime factor of 12
- Be careful with how many times a prime factor appears
- Multiply the first number by these extra prime factors
- Either multiply 10 by 3 and 2
- Or multiply 12 by 5
- Both ways will give you the same answer
- Using a Venn diagram can help
- Put the common prime factors in the centre
- Put the other prime factors in the relevant circles
- The LCM is the product of all the numbers in the Venn diagram
Worked example
Find the lowest common multiple of 42 and 90.
Write as a product of prime factors.
42 = 2 × 3 × 7
90 = 2 × 3 × 3 × 5
Write the prime factors in a Venn diagram if needed.
Multiply all the prime factors.
LCM = 7 × 2 × 3 × 3 × 5
This is the same as doing 42 × 3 × 5 or 90 × 7
LCM = 630
You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?