Translations of Graphs (OCR GCSE Maths): Revision Note
Exam code: J560
Translations of Graphs
What are translations of graphs?
The equation of a graph can be changed in certain ways
This has an effect on the graph
How a graph changes is called a graph transformation
A translation is a type of graph transformation that shifts (moves) a graph (up or down, left or right) in the xy plane
The shape, size, and orientation of the graph remain unchanged

A particular translation is specified by a translation vector
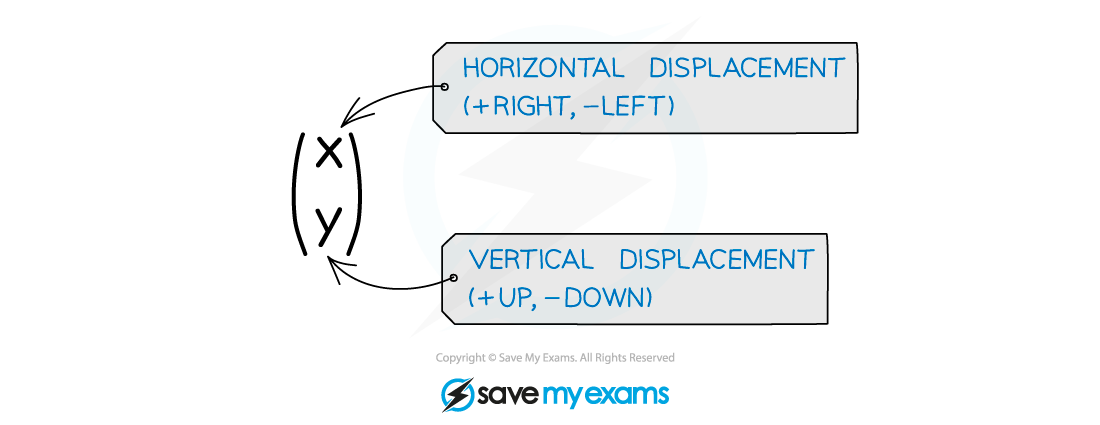
How do I translate graphs?
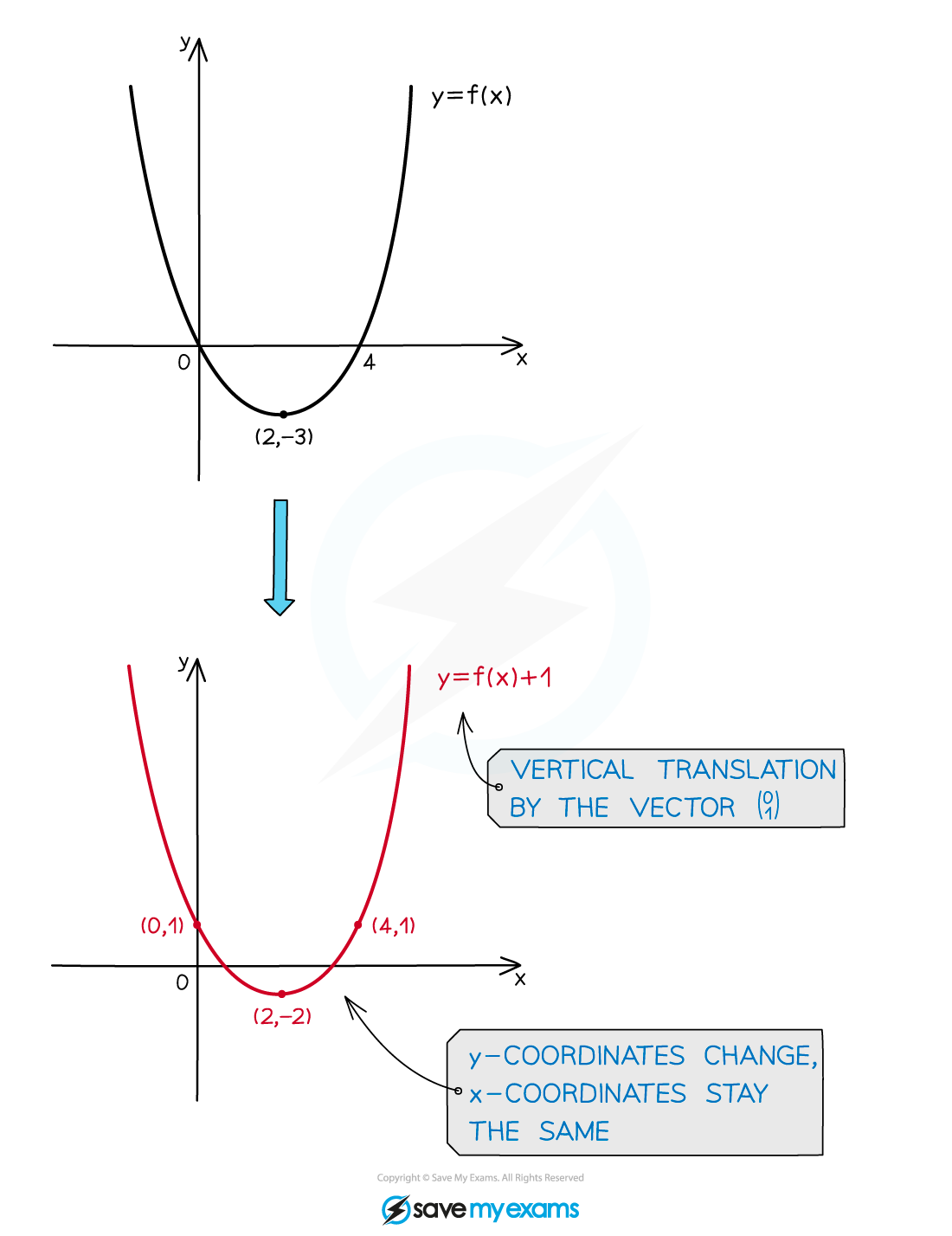
Vertical translations: y=f(x) + a
Given an original equation in x, the graph of that equation will be translated +a units in the y direction by adding a outside the bracket
The graph moves up for positive values of
The graph moves down for negative values of
The x-coordinates stay the same
For example, in relation to y = x2;
y = x2 + 2 is a translation 2 units up/ a translation by +2 in the y-axis
y = x2 − 3 is a translation 3 units down/ a translation by −3 in the y-axis
Another example, in relation to y = sin(x) where x is in degrees;
y = sin(x) − 2 is a translation 2 units down/ a translation by −2 units in the y-axis
y = sin(x) + 1 is a translation 1 unit up/ a translation by +1 unit in the y-axis
Note that for translations in the y direction, the direction is the same as the sign of a
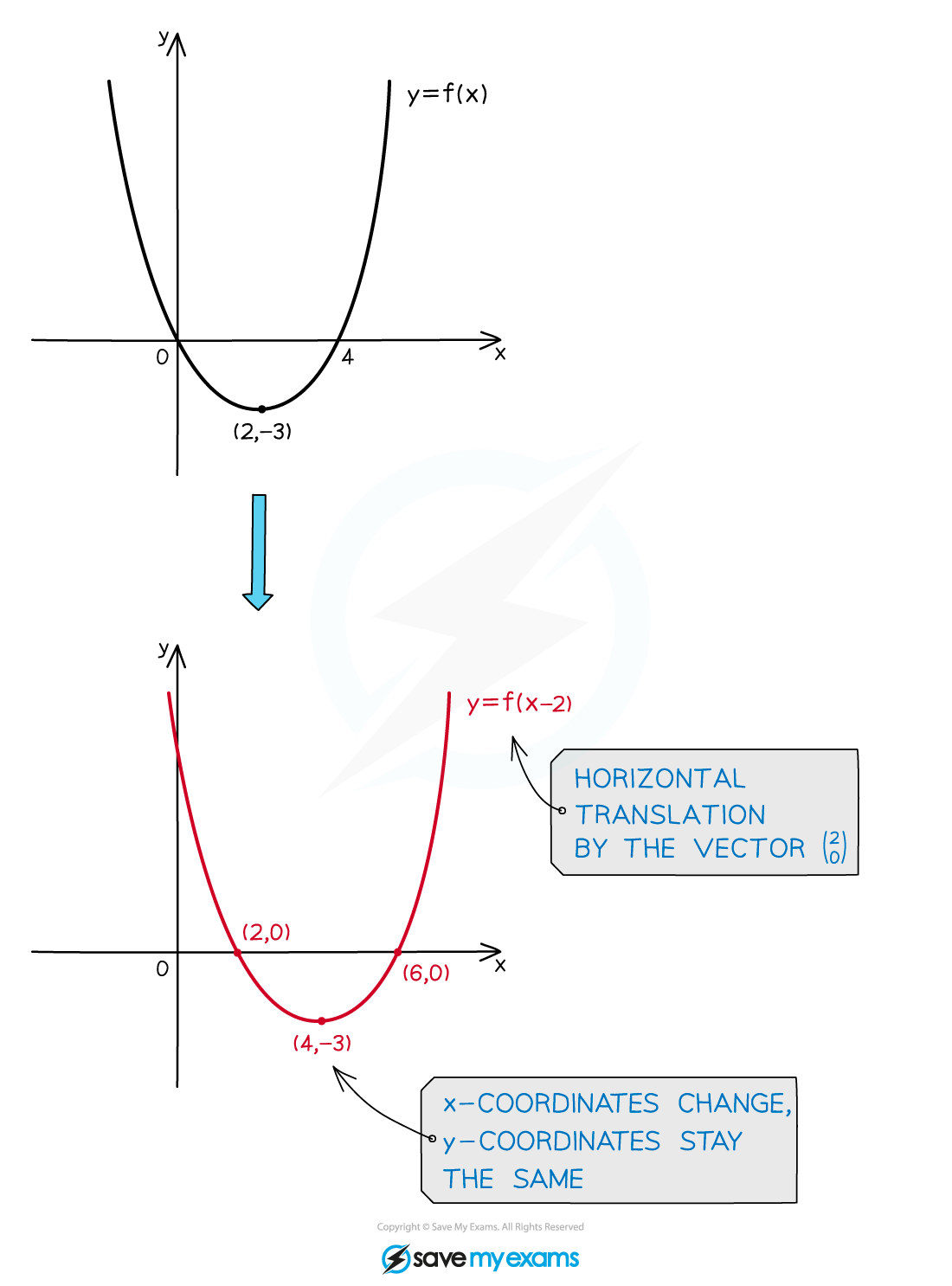
Horizontal translations: y=f(x + a)
Given an original equation in x, the graph of that equation will be translated −a units in the x direction by adding a inside a bracket next to x
The graph moves left for positive values of
This is often the opposite direction to which people expect
The graph moves right for negative values of
The y-coordinates stay the same
For example, in relation to y = x2;
y = (x − 2)2 is a translation 2 units to the right/ a translation by +2 in the x-axis
y = (x + 3)2 is a translation 3 units to the left/ a translation by −3 in the x-axis
Another example, in relation to y = sin(x) where x is in degrees;
y = sin(x + 90) is a translation 90 degrees to the left/ a translation by −90 degrees in the x-axis
y = sin(x − 180) is a translation 180 degrees to the right/ a translation by +180 degrees in the x-axis
Note that for changes in the x direction, the translation is in the opposite direction to the sign of a (as highlighted)!
How does a translation affect the equation of the graph?
For a horizontal translation of a units to the right
is subtracted from
throughout the equation
Every instance of
in the equation is replaced with
E.g. the graph
undergoes a translation of 6 units to the right
is replaced throughout the equation by
is the new equation
The equation can be left in this form or expanded and simplified
For a vertical translation of a units up
is added to the equation as a whole
E.g. the graph
undergoes a translation of 5 units down
5 is subtracted from the equation as a whole
The equation can be left in this form or simplified
How do I apply a combined translation?
For a horizontal translation of
units and vertical translation of
units combined
E.g. the graph
undergoes a translation of 2 units up and 1 unit to the left
is replaced throughout the equation by
2 is added to the equation as a whole
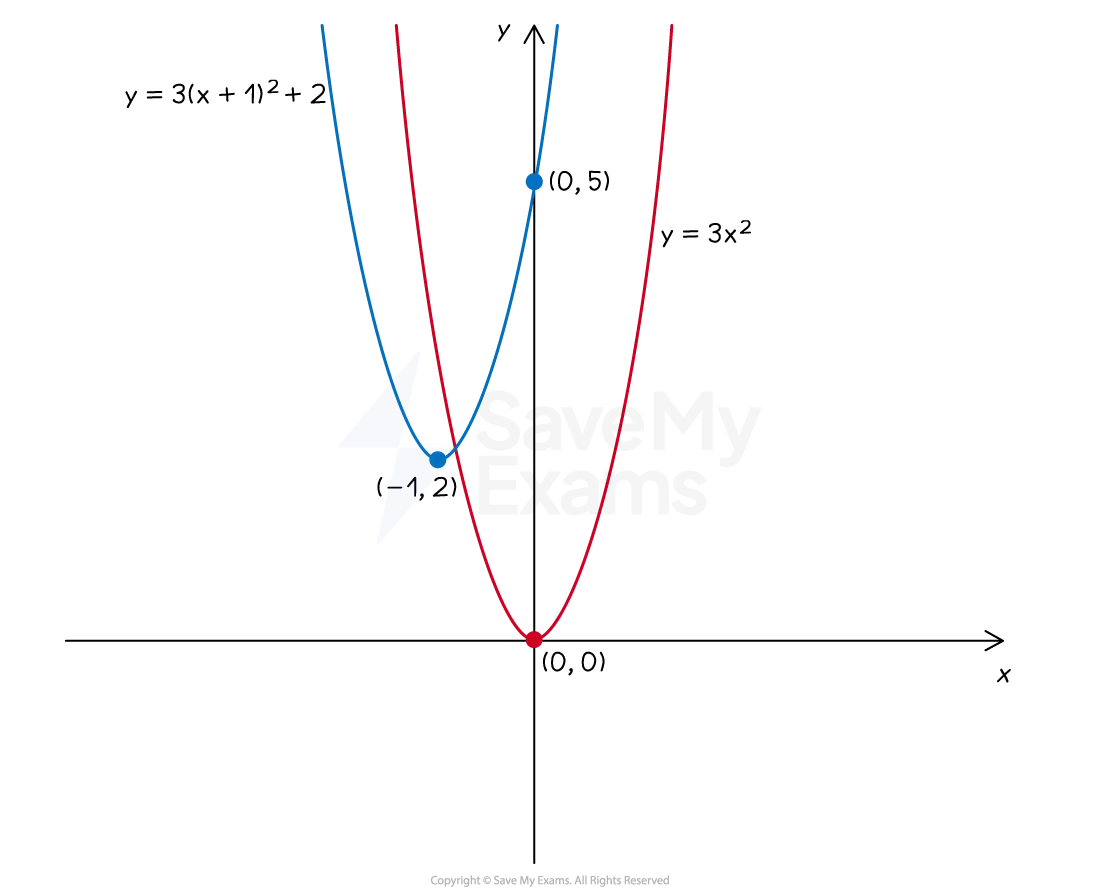
Note that when the equation is in the form
the vertex is
the value of
does not affect the vertex coordinates
Worked Example
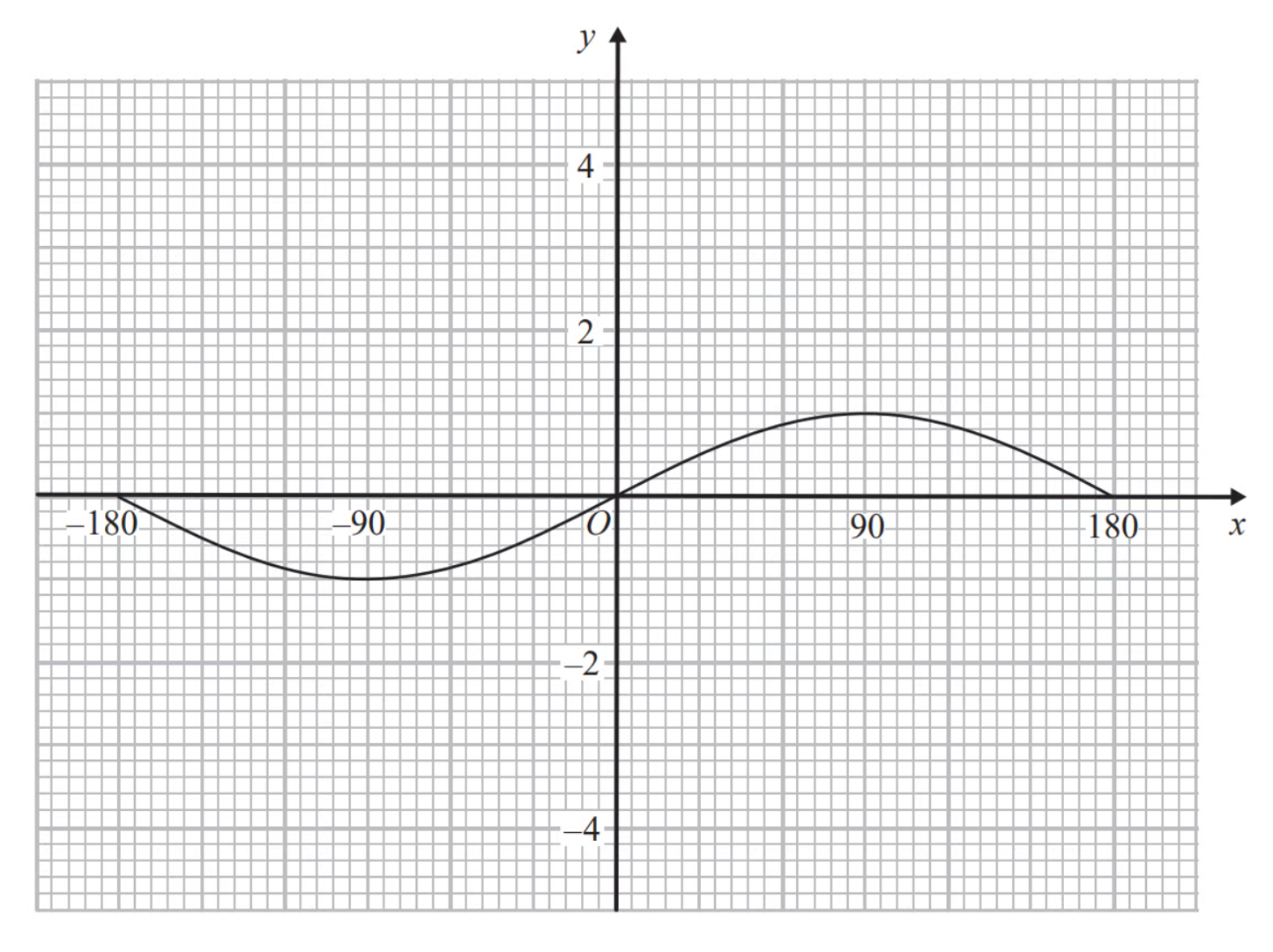
The graph of is shown on the graph below.
On the same graph sketch .
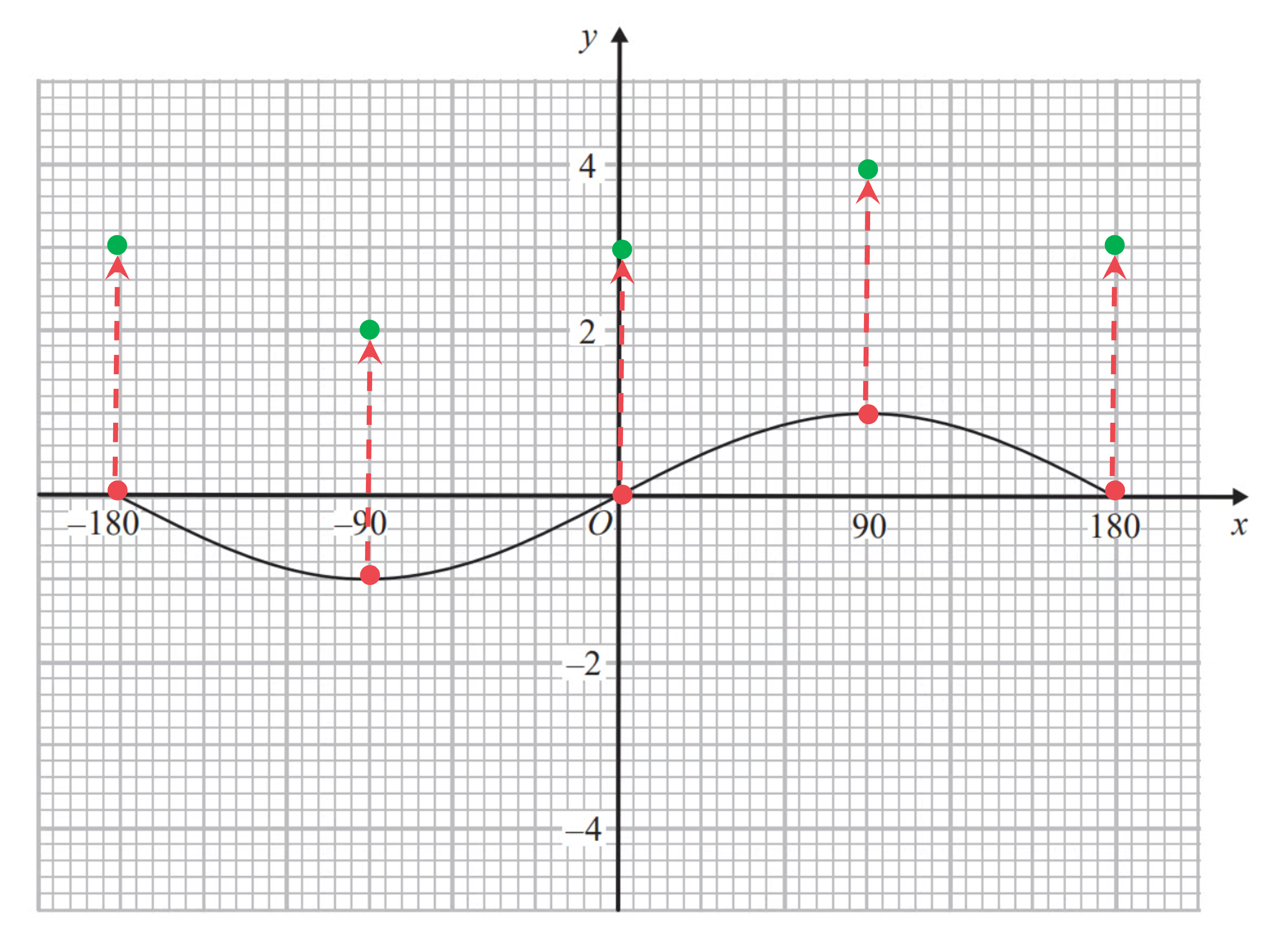
This is a translation by +3 in the y direction (i.e. 3 up)
So we copy the given graph in its new position. Translate key points first- x-intercepts, maximums and minimums as shown below
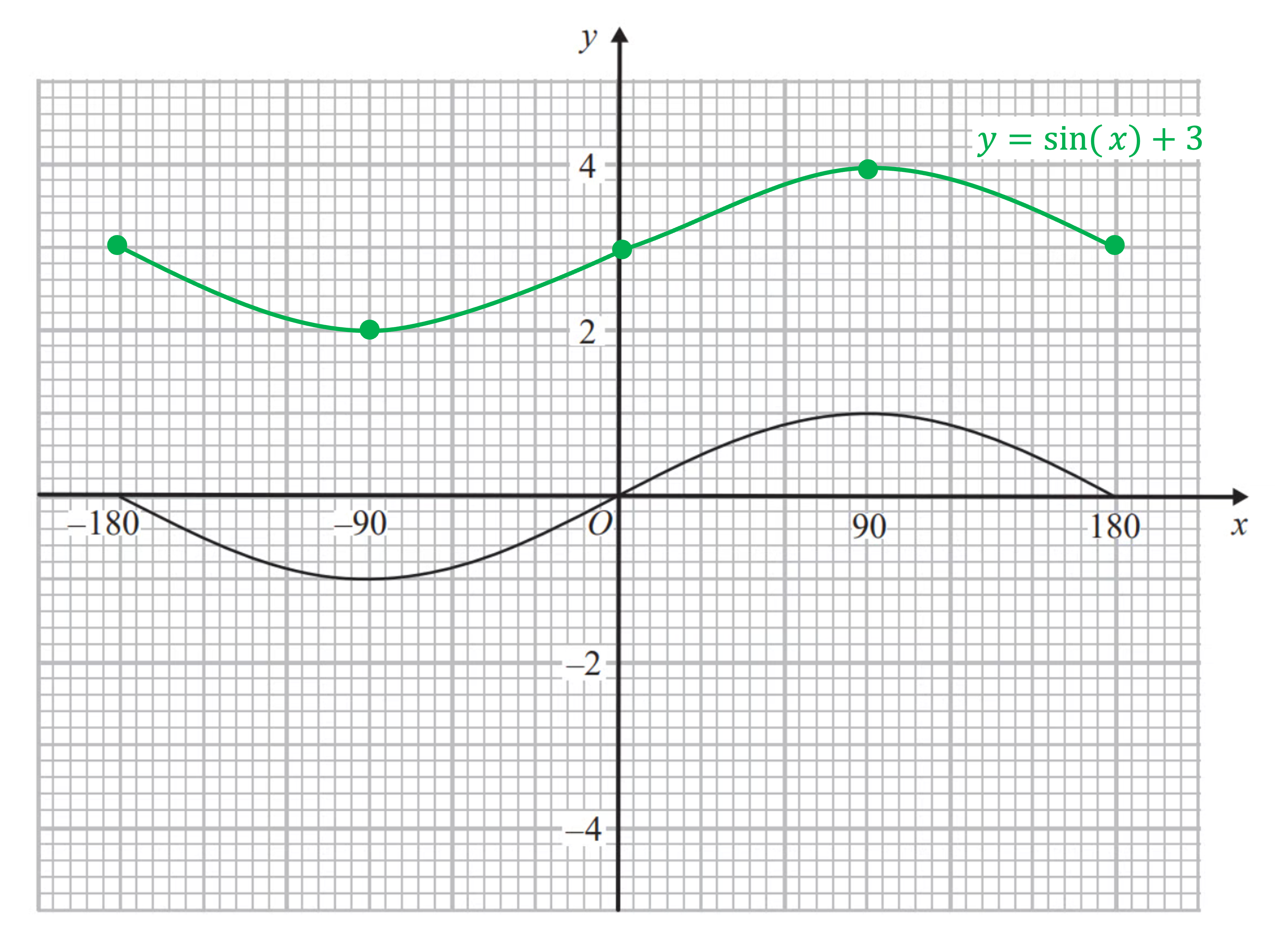
Now join your new points with a curved line. The new curve should go through the key points shown in the answer below
Worked Example
Describe the transformation that maps the graph of to the graph of
.
The number inside the bracket (next to x) is −4 so this is a translation by +4 in the x-axis (note the change in sign again)
The number outside the bracket (not next to x!) is −6 so this is a translation by −6 in the y-axis
Translation by
or Translation 4 right and 6 down
or Translation by +4 in the x-axis and −6 in the y-axis

You've read 0 of your 5 free revision notes this week
Unlock more, it's free!
Did this page help you?