Converting Fractions, Decimals & Percentages (Edexcel GCSE Maths)

FDP Conversions

How do I convert from a percentage to a decimal?

• Divide by 100 (move digits two places to the right)

  • 6% as a decimal is 6 ÷ 100 = 0.06

  • 40% as a decimal is 40 ÷ 100 = 0.4

  • 350% as a decimal is 350 ÷ 100 = 3.5

  • 0.2% as a decimal is 0.2 ÷ 100 = 0.002

How do I convert from a decimal to a percentage?

• Multiply by 100 (move digits two places to the left and add a % sign)

  • 0.35 as a percentage is  0.35 × 100 = 35%

  • 1.32 as a percentage is 1.32 × 100 = 132%

  • 0.004 as a percentage is 0.004 × 100 = 0.4%

How do I convert from a decimal to a fraction?

• If it has one decimal place, write the digits over 10

  • 0.3 is 

  • 1.1 is 

• If it has two decimal places, write the digits over 100

  • 0.07 is 

  • 0.13 is  

  • 30.01 is  

• If it has n decimal places, write the digits over 10ⁿ

  • 0.513 is 

  • 0.0007 is  

• Learn simple recurring decimals as fractions

  • 0.33333… =   is   

  • 0.66666… =  is 

• Whole numbers can be written as fractions by writing them over 1

  • 5 is 

How do I convert from a percentage to a fraction?

• Write the percentage over 100

  • 37% is

How do I convert from a fraction to a decimal?

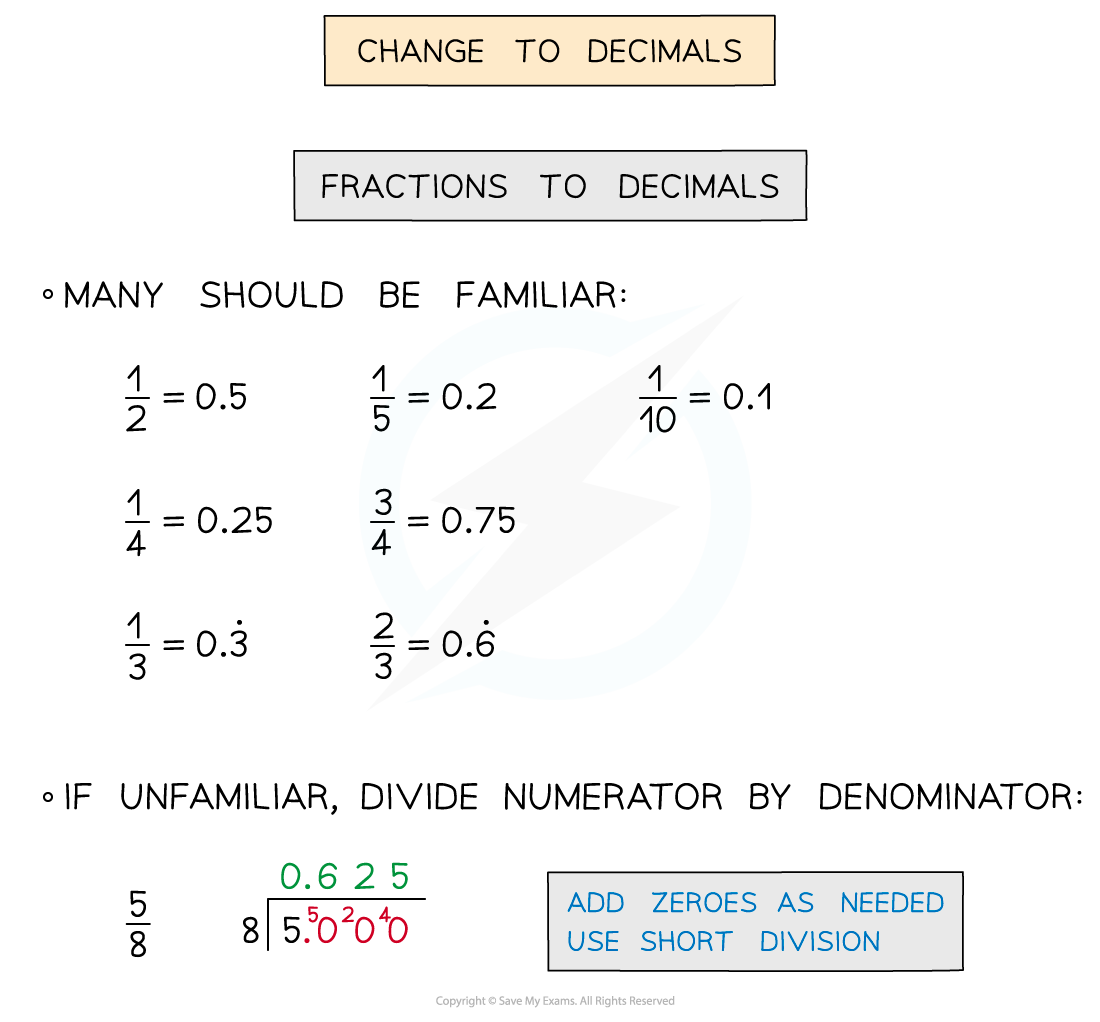
• Fractions written over powers of 10 are quicker

  •  which is 0.6

  •  which is 0.35

  •  which is 0.002

How do I convert from a fraction to a percentage?

• Change fractions into decimals then multiply by 100

  •  which is 0.8 as a decimal, which is 0.8 × 100 = 80%