Angles of Elevation & Depression (Edexcel GCSE Maths) : Revision Note
Elevation & Depression
What are angles of elevation and depression?
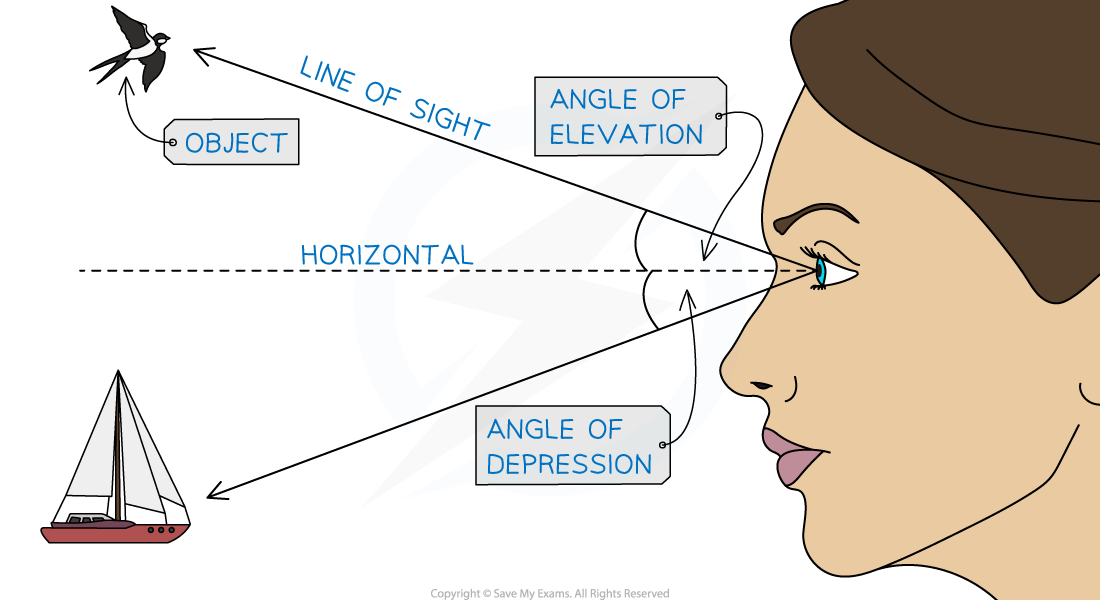
An angle of elevation or depression is the angle measured between the horizontal and the line of sight
Looking up at an object creates an angle of elevation
Looking down at an object creates an angle of depression
Right-angled trigonometry can be used to find
an angle of elevation or depression
or a missing distance
The tan ratio is often used in real-life scenarios
You may know the height of an object and want to find the distance you are from it
You may know the distance you are from an object and want to find its height
Examiner Tips and Tricks
It may be useful to draw more than one diagram if the triangles that you are interested in overlap one another.
Worked Example
A cliff is perpendicular to the sea and the top of the cliff, T, stands 24 metres above the level of the sea.
The angle of depression from the top of the cliff to a boat at sea is 35°.
At a point metres vertically up from the foot the cliff is a flag marker, M.
The angle of elevation from the boat, B, to the flag marker is 18°.
(a) Draw a diagram of the situation. Label all the angles and distances given above.
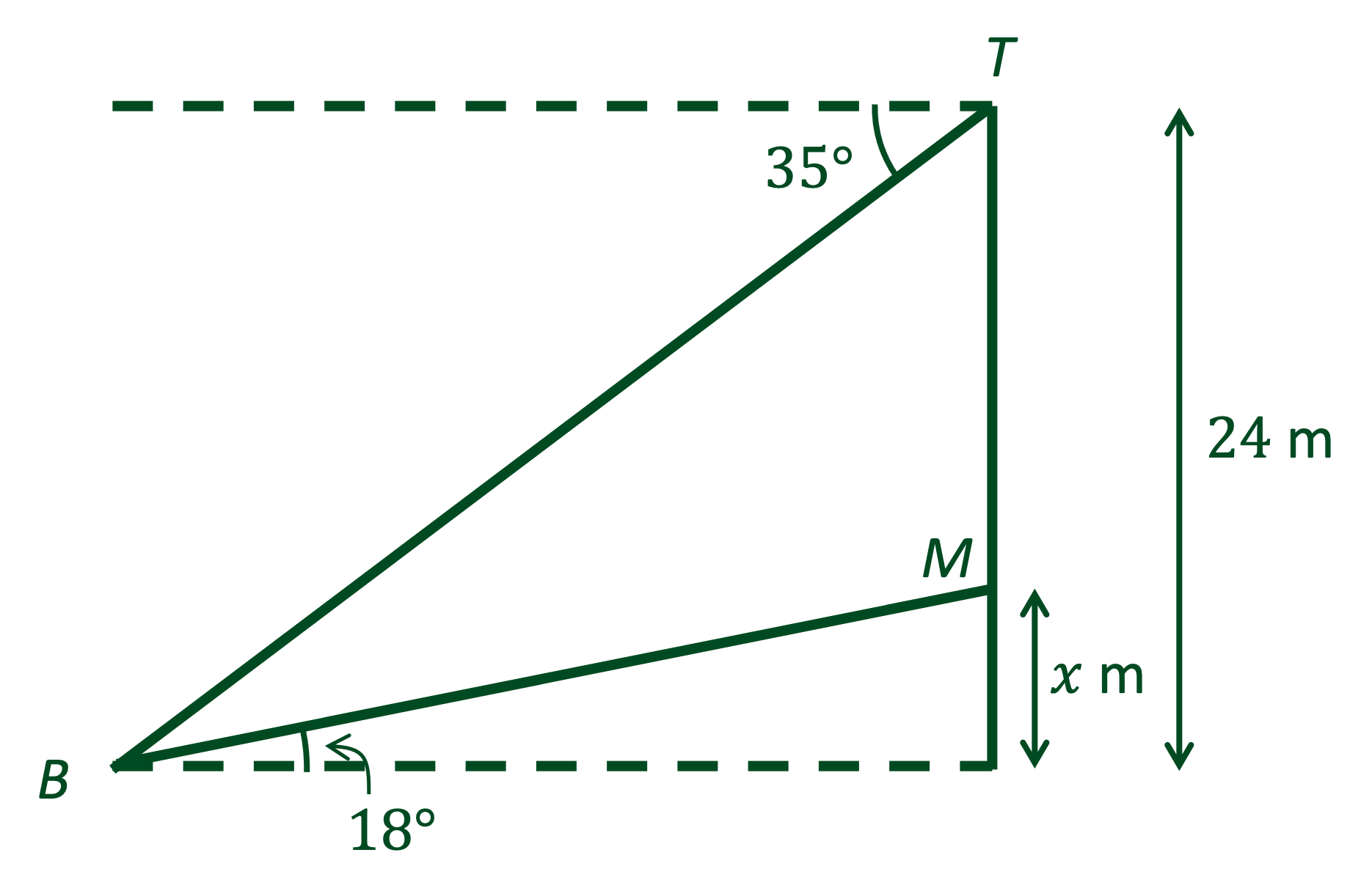
(b) Find the distance from the boat to the foot of the cliff.
Consider triangle TBF where F is the foot of the cliff
Angle TBF = 35º because of alternate angles
Use SOHCAHTOA to find the missing distance
We know the opposite (TF) and we want to find the adjacent (BF), so use
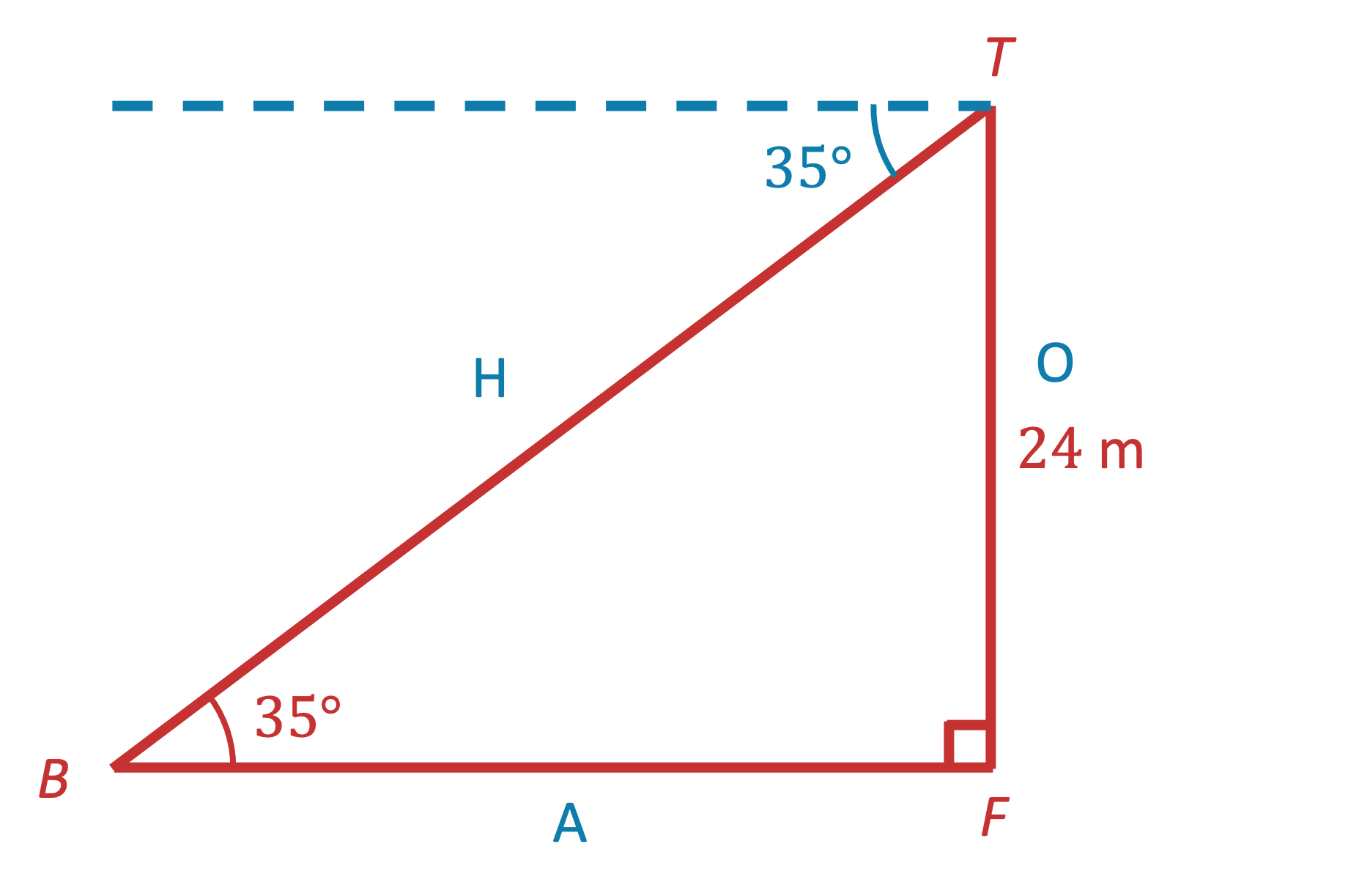
BF = 34.3 m (3 s.f.)
(c) Find the value of .
Consider triangle MBF
Use SOHCAHTOA to find the missing distance
We know the adjacent (BF) and we want to find opposite (MF), so use
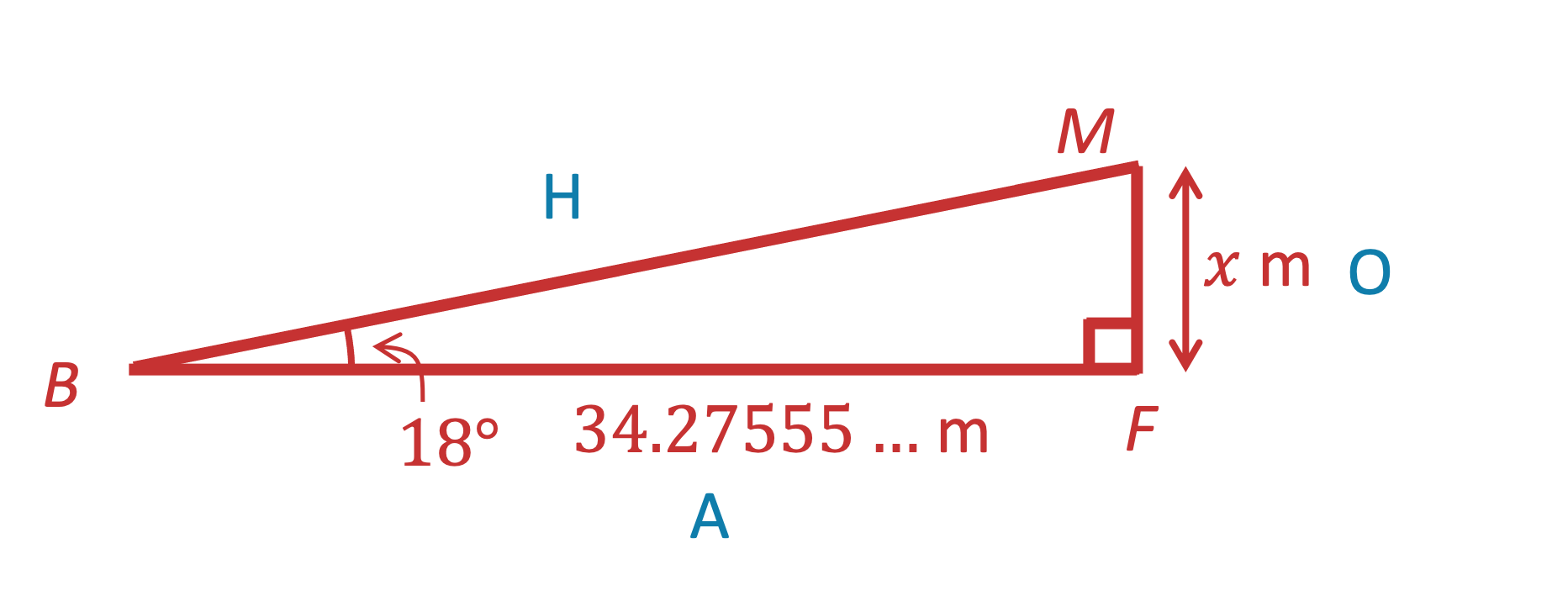
m (3 s.f.)

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?

