GCSEMathsEdexcelFoundationRevision NotesAlgebraSolving InequalitiesSolving Linear Inequalities

Solving Linear Inequalities (Edexcel GCSE Maths)

Revision Note

Test yourself
Naomi C

Author

Naomi C

Last updated

Solving Linear Inequalities

How do I solve a linear inequalities?

  • Solving linear inequalities is just like Solving Linear Equations

    • Follow the same rules, but keep the inequality sign throughout

    • If you change the inequality sign to an equals sign you are changing the meaning of the problem

  • When you multiply or divide both sides by a negative number, you must flip the sign of the inequality 

    • E.g. 
      space 1 less than 2 open parentheses cross times negative 1 close parentheses space space space space space space space space space space space space space space space space space space space open parentheses cross times negative 1 close parentheses space minus 1 greater than negative 2

  • Never multiply or divide by a variable (x) as this could be positive or negative

  • The safest way to rearrange is simply to add and subtract to move all the terms onto one side

How do I solve double inequalities?

  • Inequalities such as a space less than space 2 x space less than space b can be solved by doing the same thing to all three parts of the inequality

    • Use the same rules as solving linear inequalities

Examiner Tips and Tricks

  • Do not change the inequality sign to an equals when solving linear inequalities.

    • In an exam you will lose marks for doing this. 

  • Remember to reverse the direction of the inequality sign when multiplying or dividing by a negative number!

Worked Example

Solve the inequality 2 x minus 5 less or equal than 21.

Add 5 from both sides

2 x less or equal than 26

Now divide both sides by 2

x less or equal than 13

bold italic x bold less or equal than bold 13 

Worked Example

Solve the inequality 5 minus 2 x less or equal than 21.

Subtract 5 from both sides, keeping the inequality sign the same

negative 2 x less or equal than 16

Now divide both sides by -2.
However because you are dividing by a negative number, you must flip the inequality sign

x greater or equal than negative 8

bold italic x bold greater or equal than bold minus bold 8 or bold minus bold 8 bold less or equal than bold italic x

Worked Example

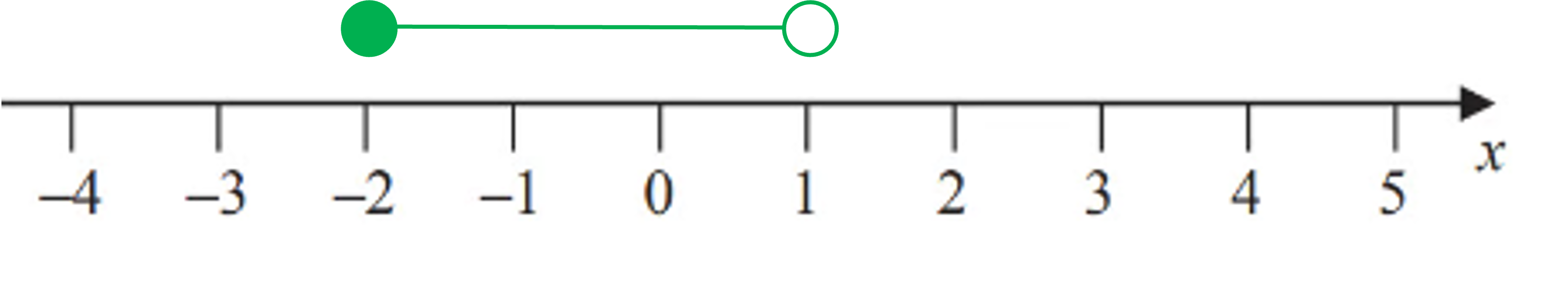
Solve the inequality negative 7 space less or equal than space 3 x space minus space 1 space less than space 2, illustrating your answer on a number line.

This is a double inequality, so any operation carried out to one side must be done to all three parts
Use the expression in the middle to choose the inverse operations needed to isolate x

Add 1 to all three parts
Remember not to change the inequality signs

negative 6 space less or equal than space 3 x space less than space 3

Divide all three parts by 3
3 is positive so there is no need to flip the signs

bold minus bold 2 bold space bold less or equal than bold space bold italic x bold space bold less than bold space bold 1

Illustrate the final answer on a number line, using an open circle at 1 and a closed circle at -2.

2-18-solving-inequalities

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Representing Inequalities on a Number LineNext:Introduction to Sequences
    Naomi C

    Author: Naomi C

    Expertise: Maths

    Naomi graduated from Durham University in 2007 with a Masters degree in Civil Engineering. She has taught Mathematics in the UK, Malaysia and Switzerland covering GCSE, IGCSE, A-Level and IB. She particularly enjoys applying Mathematics to real life and endeavours to bring creativity to the content she creates.