GCSEFurther MathsAQAExam Questions6. GeometryTrigonometry

Trigonometry (AQA GCSE Further Maths)

Exam Questions

1 hour17 questions
Medium
Hard
Very Hard
1
Sme Calculator3 marks

A B equals 4 space cm A C equals 7 space cm  cos space x equals negative 2 over 7

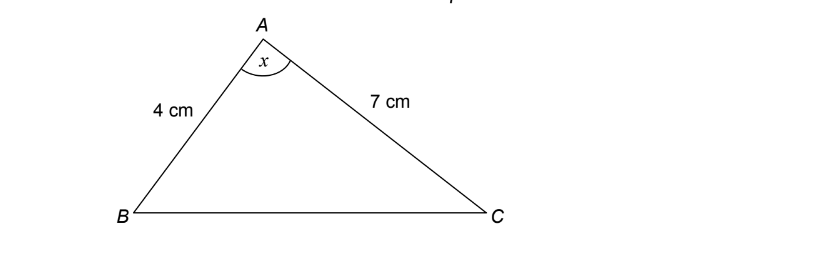
Triangle with vertices labelled A, B and C. Side AB is 4 cm and side AC is 7 cm. Angle BAC is labelled x.

Work out the length of B C.

....................... cm

How did you do?

Did this page help you?

2a
Sme Calculator2 marks

A B C D is a square.
C D E is a straight line.

A C is 3 square root of 2 cm and angle D E A equals 60 degree

A trapezium ABCE. Angle AED is 60 degrees and AC has length 3 square root 2 cm. A point D is positioned on CE vertically below A forming a square ABCD>

Show that the side of the square is 3 space cm

How did you do?

2b
Sme Calculator4 marks

Show that the perimeter of trapezium A B C E is 3 open parentheses 3 plus square root of 3 close parentheses space cm

How did you do?

Did this page help you?

3a
Sme Calculator1 mark

A D E F is a trapezium.

A B C D is a straight line.

B C E F is a square of side square root of 6 space end root space cm

A trapezium with vertices ADEF. Points B and C lie on line AD, with point B being vertically below F and C being vertically below E. BCEF forms a square. Angle BAF is 60 degrees and angle CDE is 30 degrees. The length of BF is square root 6 cm.

Show that A B equals square root of 2 space cm

How did you do?

3b
Sme Calculator1 mark

Show that D E equals 2 square root of 6 space cm

How did you do?

3c
Sme Calculator3 marks

Work out the perimeter of the trapezium A D E F.

Give your answer in the form t square root of 2 space end root plus w square root of 6 where t and w are integers.

You must show your working.

..........................cm

How did you do?

Did this page help you?

4
Sme Calculator4 marks

A B C D E F G H is a cuboid.

B C equals 15 space cm    C D equals 12 space cm     D H equals 8 space cm

Cuboid ABCDEFGH. A straight line connects diagonally opposite vertices C and E.

Work out the size of the angle between the line C E and the plane C D H G.

How did you do?

Did this page help you?

5
Sme Calculator5 marks

In triangle A B C,

A B equals 6 square root of 2 space cm, angle A B C equals 45 degree and angle A C B equals 60 degree

Triangle ABC. AB has length 6 square root 2 cm and AC has length x cm. Angle ABC is 45 degrees and angle ACB is 60 degrees.

Work out the value of x.

Give your answer in the form a square root of b, where a and b are integers.

You must show your working.

................................ cm

How did you do?

Did this page help you?

1
Sme Calculator3 marks

A B C D is a rhombus with side length 8 space cm

Angle A B C equals 60 degree

A rhombus with vertices labelled A, B, C and D. Angle ABC is 60 degrees and side BC has length 8 cm.

Work out the area of the rhombus.

Give your answer in the form a square root of b cm2 where a and b are integers.

............................cm squared

How did you do?

Did this page help you?

2
Sme Calculator3 marks

Use the sine rule to work out the size of obtuse angle x.

A scalene triangle. One angle is labelled 18 degrees and the length opposite it is labelled y. Another angle is labelled x and the length opposite it is labelled 2y.

.............................. degrees

How did you do?

Did this page help you?

3
Sme Calculator3 marks

Here is a triangle.

A scalene triangle with lengths a, 3a and b. All lengths are in centimetres. The angle between the sides of length a and 3a is 120 degrees.

Use the cosine rule to work out the ratio b squared space colon space a squared

How did you do?

Did this page help you?

4
Sme Calculator4 marks

Here is a triangle.

A scalene triangle with vertices labelled P, Q and R. PR has length 7 cm, RQ has length 3 cm and PQ has length x cm. Angle PQR is 60 degrees.

Use the cosine rule to work out the value of x.

How did you do?

Did this page help you?

5
Sme Calculator3 marks

S Q R is a right-angled triangle.

P is a point on S Q.
Angle S P R = 45°
M is the midpoint of Q R.
k is a constant.

The right-angled triangle QRS is plotted on a graph with x and y axes. Point Q has coordinates (1, 1) and point R has coordinates (k, 15). Angle QSR is a right angle. The midpoint of QR is labelled M. A point P(1, 6) is labelled on the side RQ. A straight line joining P and R forms a second right-angled triangle PRS. Angle SPR is 45 degrees.

Work out the coordinates of M.

How did you do?

Did this page help you?

6a
Sme Calculator1 mark

A B C is a right-angled triangle.

A C D is an isosceles triangle.

All dimensions are in centimetres.

A right-angled triangle with vertices ABC that shares the edge AC with the isosceles triangle ACD. This forms a quadrilateral ABCD. Angle ABC is a right-angle. AB has length 3x and BC has length 4x. AD and CD both have a length of 6.5x.

Show that A C equals 5 x

How did you do?

6b
Sme Calculator5 marks

Work out an expression, in cm squared, for the area of quadrilateral A B C D.

Give your answer in the form p x squared where p is an integer.

..........................cm squared

How did you do?

Did this page help you?

7a
Sme Calculator4 marks

P Q R S T U is a triangular prism.

P Q R S is a rectangle and angle Q R U equals 90 degree

P Q equals 10 space cm Q R equals 12 space cm U R equals 7 space cm     

M is the midpoint of P Q.

A right -angled triangular prism PQRSTU. PQRS is the rectangular base. UR has length 7 cm, QR has length 12 cm and PQ has length 10 cm. The midpoint of PQ is labelled M.

Calculate the size of the angle between the line U M and the plane P Q R S.

 ........................ degrees

How did you do?

7b
Sme Calculator2 marks

Calculate the size of the angle between the planes U M R and U Q R.

........................... degrees

How did you do?

Did this page help you?

8
Sme Calculator4 marks

In triangle P Q R, cos space P equals 1 third

Triangle PQR. PR has length 2n, PQ has length 3n and QR has length w.

Show that triangle space P Q R spaceis isosceles.

How did you do?

Did this page help you?

9
Sme Calculator5 marks

V A B C D is a pyramid with a horizontal rectangular base A B C D.

V is directly above the centre of the base.

V A equals V B equals V C equals V D equals 10 space cm

A B equals 8 space cm B C equals 6 space cm

M is the midpoint of B C.

A rectangular based pyramid. The vertices of the base are labelled A, B, C and D. The vertex at the top of the pyramid is labelled V. The midpoint of the length BC is labelled M. Dotted lines connect D and M, and V and M.

Work out the size of angle V M D.

............................ degrees

How did you do?

Did this page help you?

1
Sme Calculator5 marks

A B C D E F G H is a cube with side length 32 space cm

M and N are points on D H and C G respectively.

A cube ABCDEFGH. A point N is labelled on the edge CG and a point M is labelled on the edge DH. The angle GMN is 28 degrees and angle MNG is 90 degrees.

Work out the size of the angle that the line B M makes with the plane A B C D.

...................degrees

How did you do?

Did this page help you?

2
Sme Calculator4 marks

Angle y is acute.

tan space y equals fraction numerator p plus 1 over denominator p minus 1 end fraction where p is a constant greater than 1

Work out the expression for sin space y

Give your answer in the form fraction numerator a p plus b over denominator square root of c p squared plus d end root end fraction where a comma space b comma space c and d are integers.

You may use a diagram to help you.

How did you do?

Did this page help you?

3
Sme Calculator4 marks

Pyramid V A B C D has a horizontal rectangular base.

X is the centre of the base.
V is vertically above X.

V B equals V C equals 17 space cm     A B equals 22 space cm     B C equals 16 space cm

Rectangular-based pyramid VABCD. X is the point on the base vertically below the vertex V.

Work out the angle between the planes V B C and A B C D.

.............................degrees

How did you do?

Did this page help you?