Chemical Measurements (AQA GCSE Chemistry)

Uncertainty & error

• An error is the difference between a value or quantity obtained in an experiment and an accepted or literature value for an experiment

• There are two types of errors in experiments, random errors and systematic errors

• Uncertainties are the same as random errors

• Uncertainties express the confidence to which the measurement can be taken

Random Errors

• When you are reading an instrument and estimate the final digit, there is an equal chance that you may read it slightly too high or slightly too low

  • This is a random error

• Random errors are can be affected by:

  • How easily the instrument or scale is to read

  • The person reading the scale poorly

  • Changes in the environment, for example

    • fluctuations in the temperature of the lab

    • air currents in the room

• Random errors will pull a result away from an accepted value in either direction (either too high or too low)

Systematic Errors

• Systematic errors are errors that occur as a result of a faulty or poorly designed experimental procedure

• Systematic errors will always pull the result away from the accepted value in the same direction (always too high or always too low)

• For example,

  • If you forget to zero an electronic balance (using the tare button) the mass weighings will always be higher than they should be

  • If you don’t read the volume in a burette at eye level, the volumes will always be smaller than they should be due 

  • If you fail to keep a cap on a spirit burner in a calorimetry experiment, the alcohol will evaporate and give you a larger mass loss

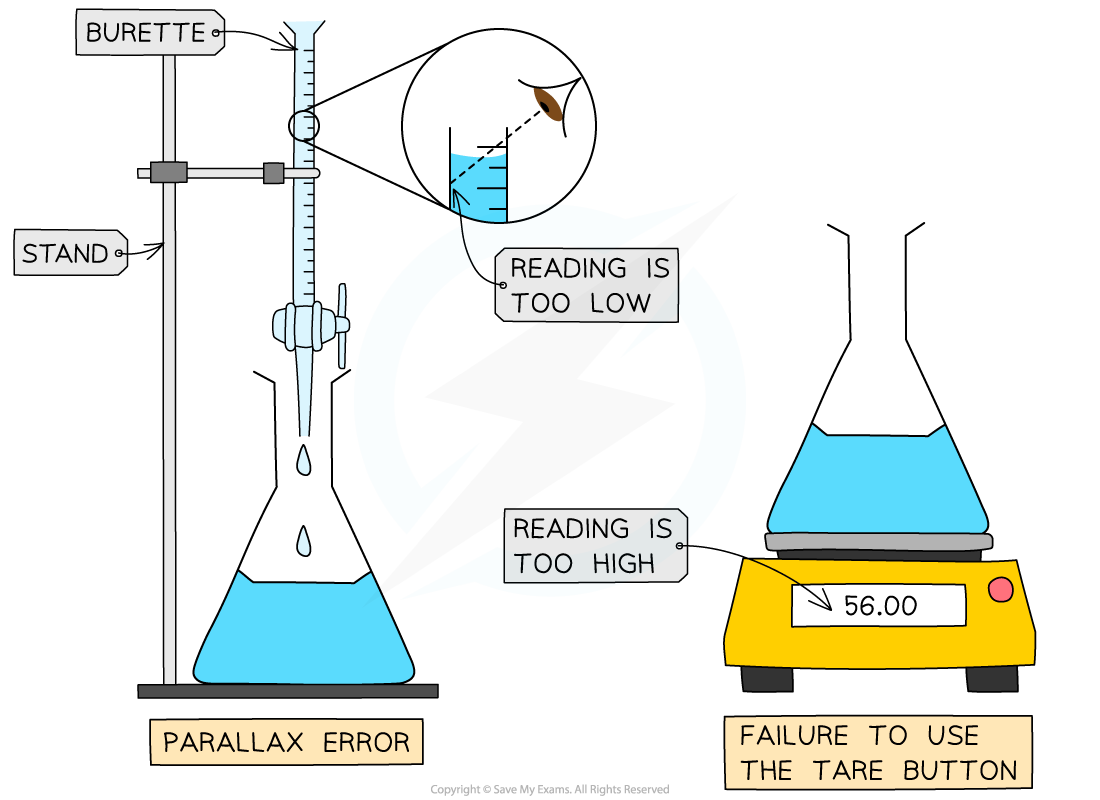
Systematic errors always pull the result away from the accepted value in the same direction: either too high or too low

How to calculate uncertainty

• Treatment of uncertainties depends on the type of instrument used

Using analogue instruments

• Any instruments that have an analogue scale, the uncertainty is taken as half the smallest division on the scale

• For example,

  • A thermometer that reads to 1^oC, the uncertainty would be +0.5 ^o C

  • A burette that reads to 0.10 mL, the uncertainty would be +0.05 mL

Using digital instruments

• Any instruments that have a digital scale , the uncertainty is taken as the smallest division on the scale

• For example,

  • An electronic balance that reads to 0.01 g, the uncertainty would be +0.01 g

Uncertainty in results

• For results that are obtained from a series of repeated experiments, the uncertainty is ± half of the range of results 

• This can be estimated by:

  • Calculating the mean average and then determining the deviation of the highest and lowest results from the mean value

  • An alternative method is to calculate the range of the results and then divide this value by 2

Other uncertainties

• Other sources of uncertainty can arise where the judgement of the experimenter is needed to determine a changing property

• For example,

  • Judging the end point of a titration by looking at the colour of the indicator

  • Controlling a stopwatch in a rate of reaction experiment

  • Deciding when to extinguish the flame in an  experiment

• These uncertainties are very difficult to quantify, but they should be commented on as a source of error in an evaluation