Using Units (Edexcel GCSE Biology): Revision Note
Exam code: 1BI0
Converting Units
You may be given a question in your Biology exam where the measurements for a magnification calculation have different units
You need to ensure that you convert them both into the same unit before proceeding with the calculation (usually to calculate the magnification)
Remember the following to help you convert between mm (millimetres), µm (micrometres) and nm (nanometres):

Converting between mm (millimetres), µm (micrometres) and nm (nanometres)
If you are given a question with two different units in it, make sure you make a conversion so that both measurements have the same unit before doing your calculation
For example:
Worked Example
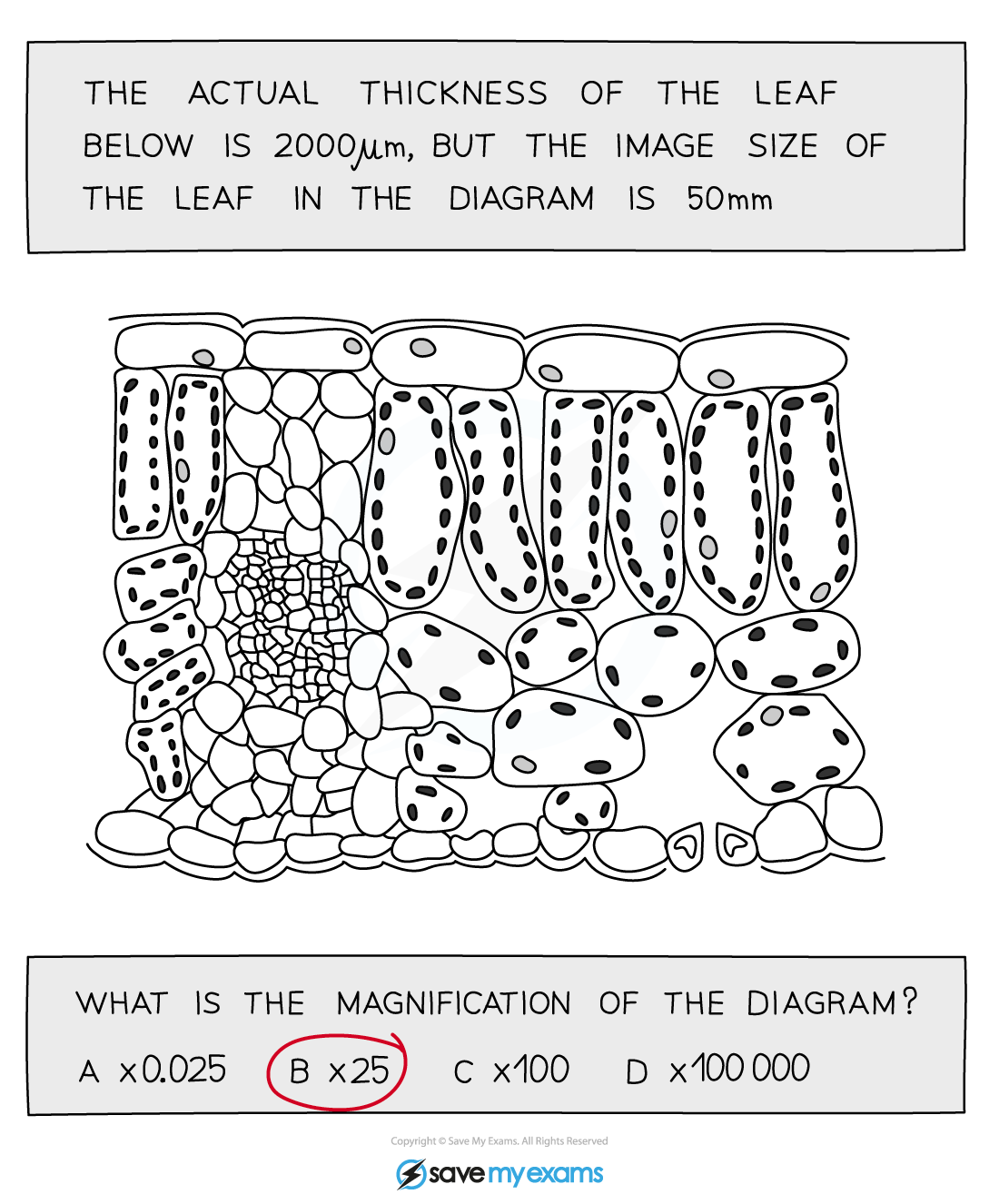
Answer:
Step One:
Remember that 1 mm = 1000 µm
So to get from µm to mm you need to divide by 1000
Step Two: Calculate the thickness of the leaf in mm
2000 ÷ 1000 = 2, so the actual thickness of the leaf is 2 mm and the drawing thickness is 50 mm
Step Three: Put these values into the equation for calculating magnification
Magnification = image size ÷ actual size
= 50 ÷ 2
= 25
So the magnification is x 25
Standard form
When doing calculations and unit conversions, it is common to come across very big or very small numbers
Standard form can be useful when working with these numbers
Standard form is a way of writing very big and very small numbers using powers of 10
How to use standard form
Using standard form, numbers are always written as follows: a × 10n
The rules:
1 ≤ a < 10 (the number 'a' must always be between 1 and 10)
n > 0 for LARGE numbers ('n' = how many times 'a' is multiplied by 10)
n < 0 for SMALL numbers ('n' = how many times 'a' is divided by 10)
Using standard form to convert between units
For example, you can write 1 metre in millimetres using standard form:
1 m = 1000 mm
So, 1 m = 1 mm × 1000
So, 1 m = 1 mm × 10 × 10 × 10
So, as we had to multiply 1 mm by 10 three times to get 1 m, we write this as:
1 m = 1 × 103 mm
Writing 1 millimetre in metres using standard form is also possible and is just the opposite:
1 mm = 0.001 m
So, 1 mm = 1 m ÷ 1000
So, 1 mm = 1 m ÷ 10 ÷ 10 ÷ 10
So, as we had to divide 1 m by 10 three times to get 1 mm, we write this as:
1 mm = 1 × 10-3 m
Exactly the same process can be used if you needed to convert micrometres into millimetres. For example:
1 µm = 0.001 mm
So, 1 µm = 1 mm ÷ 1000
So, 1 µm = 1 mm ÷ 10 ÷ 10 ÷ 10
So, as we had to divide 1 mm by 10 three times to get 1 µm, we write this as:
1 µm = 1 × 10-3 mm
Examples of using standard form in conversion calculations
You could be asked to state 45 centimetres in millimetres using standard form:
1 cm = 10 mm
So, 45 cm = 450 mm
So, 45 cm = 4.5 mm × 10 × 10
So, as we had to multiply 4.5 mm by 10 two times to get 45 cm, we write this as:
45 cm = 4.5 × 102 mm
You could also be asked to state 250 micrometres in millimetres using standard form:
1 µm = 0.001 mm
So, 250 µm = 0.25 mm
So, 25 µm = 2.5 mm ÷ 10
So, as we had to divide 4.5 mm by 10 just once to get 250 µm, we write this as:
250 µm = 2.5 × 10-1 mm

Unlock more, it's free!
Did this page help you?