Stellar Parallax (DP IB Physics)

Astronomical Unit Conversions

• Astronomical distances are very large and as a result, are usually measured using:

  • Astronomical Units (AU)

  • Light–years (ly)

  • Parsecs (pc)

Astronomical Unit (AU)

• The astronomical unit (AU) is defined as

  The mean distance from the centre of the Earth to the centre of the Sun

• As the Earth’s orbit around the Sun is elliptical it will be slightly closer to the Sun in January (1.471 × 10¹¹ m) than it is in July (1.521 × 10¹¹ m)

• Calculating the mean of these two values gives:

= 1.496 × 10¹¹ m

• Therefore, 1 astronomical unit = 1.496 × 10¹¹ m ≈ 1.5 × 10¹¹ m

• The astronomical unit is useful for studying distances on the scale of the solar system

Light–year (ly)

• A light-year is defined as:

  The distance travelled by light in one year

• This can be calculated using:

Distance = speed × time

• Where:

  • The speed of light is 3 × 10⁸ m s^–1

  • 1 year = 60 × 60 × 24 × 365 = 3.15 × 10⁷ s

• Hence, the distance travelled by light in one year = (3 × 10⁸) × (3.15 × 10⁷) = 9.46 × 10¹⁵ m

  • Therefore, 1 light–year ≈ 9.5 × 10¹⁵ m

Parsec (pc)

• Angles smaller than 1 degree can be measured in arcminutes or arcseconds

  • 1 degree = 60 arcminutes

  • 1 arcminute = 60 arcseconds

  • Therefore, 1 degree = 60 x 60 = 3600 arcseconds

  • 1 arcsecond = 1/3600 degree

• The parsec is defined as

  A unit of distance that gives a parallax angle of 1 second of an arc (of a degree), using the radius of the Earth’s orbit (1 AU) as the baseline of a right–angled triangle

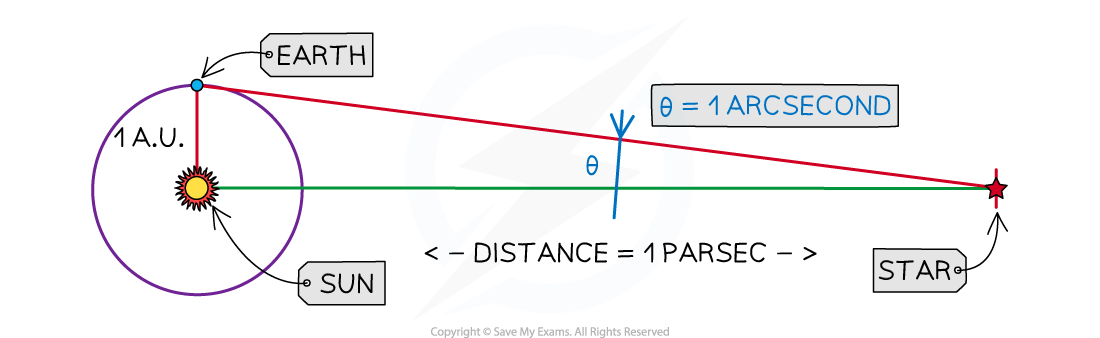
• Given that 1 AU = 1.496 × 10¹¹ m, trigonometry can be used to express 1 parsec in metres:

1 pc =  = 3.09 × 10¹⁶ m

• Therefore, 1 parsec ≈ 3.1 × 10¹⁶ m

• The parsec (1 pc = 3.1 × 10¹⁶ m) and the light-year (1 ly = 9.5 × 10¹⁵ m) are much greater in size than the astronomical unit (1 AU = 1.496 × 10¹¹ m)

• This makes them useful when studying interstellar distances

  • For example, on the scale of distances between the Earth and stars, or neighbouring galaxies

Parallax Calculations

• The principle of parallax is based on how the position of an object appears to change depending on where it is observed from

• When observing the volume of liquid in a measuring cylinder the parallax principle will result in the observer obtaining different values based on where they viewed the bottom of the meniscus from

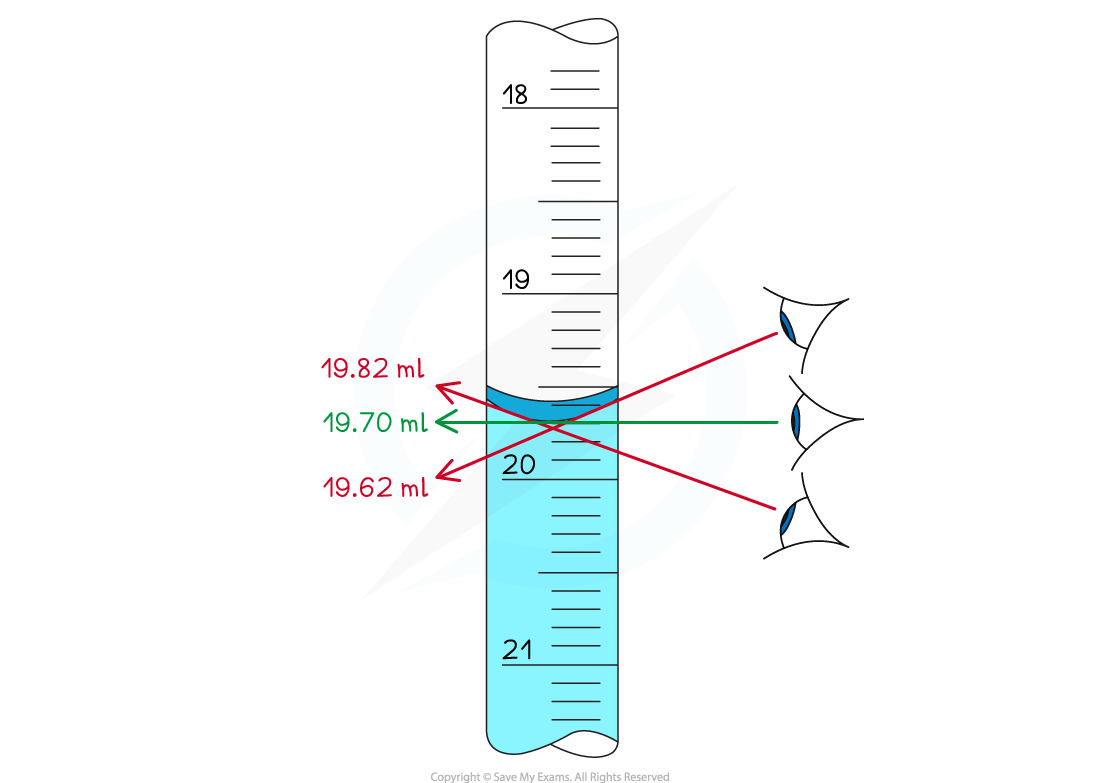
Parallax error describes the false readings that can be made by taking measurements from different angles

• Stellar parallax can be used to measure the distance to nearby stars

• Stellar Parallax is defined as:

  The apparent shifting in position of a nearby star against a background of distant stars when viewed from different positions of the Earth, during the Earth’s orbit about the Sun

• It involves observing how the position of a nearby star changes over a period of time against a fixed background of distant stars

  • To an observer, the position of distant stars does not change with time

• If a nearby star is viewed from the Earth at 6 months intervals (e.g. once in January and once again in July), the Earth will be at a different position in its orbit around the Sun

  • The nearby star will appear in a different position compared to the backdrop of distant stars

  • The distant stats will appear to not have moved

• This apparent movement of the nearby star is called the stellar parallax

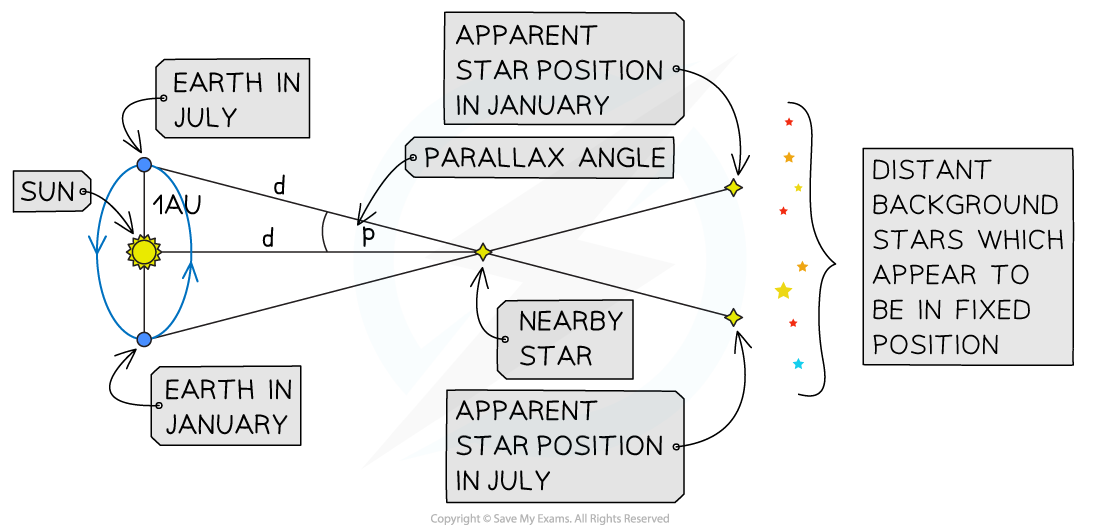
• Applying trigonometry to the parallax equation:

• Where:

  • 1 AU = radius of the Earth's orbit around the sun

  • p = parallax angle from Earth to the nearby star

  • d = distance to the nearby star

• For small angles, expressed in radians, , therefore:

• If the distance to the nearby star is to be measured in parsec, then it can be shown that the relationship between the distance to a star from Earth and the angle of stellar parallax is given by

• Where:

  • p = parallax (")

  • d = the distance to the nearby star (pc)

• This equation is accurate for distances of up to 100 pc

• For distances larger than 100 pc, the angles involved are so small they are too difficult to measure accurately