Define radioactive decay.
The graph shows the count rate of a radioactive substance measured by a Geiger-Müller tube.
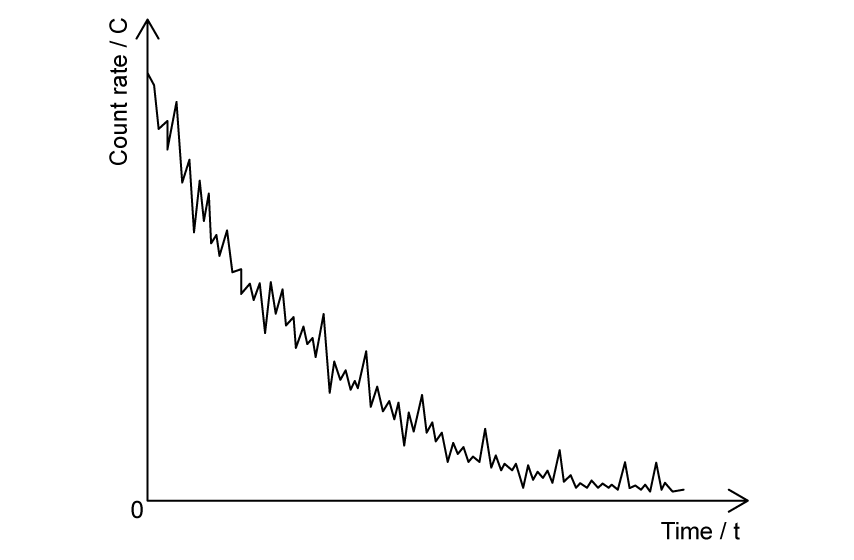
State what the fluctuations in the count rate provide evidence for.
Did this page help you?
Select a download format for Radioactive Decay
Select an answer set to view for
Radioactive Decay
Define radioactive decay.
How did you do?
The graph shows the count rate of a radioactive substance measured by a Geiger-Müller tube.

State what the fluctuations in the count rate provide evidence for.
How did you do?
Did this page help you?
The number of neutrons and number of protons for different isotopes can be plotted on a graph called a nuclear stability curve. Different regions on the graph represent the type of decay which is expected.
The three types of radioactive particles shown are alpha emitters, beta−minus emitters and beta−positive emitters.
Annotate the graph to indicate which type of radioactive particle is expected to be emitted in which region.

How did you do?
Background radiation comes from a variety of sources, some are natural and some are man-made.
i) State four examples of natural sources.
[4]
ii) State four examples of man-made sources.
[4]
How did you do?
Radiation is emitted as various different types of particle.
State 4 types of radioactive particle.
How did you do?
Did this page help you?
Complete the table with the correct properties of alpha, beta−minus, beta−positive and gamma radiation.

How did you do?
Plutonium−239 decays to Uranium−235 through the emission of an alpha particle.
Determine the missing values in the decay equation:
How did you do?
Strontium−90 decays through beta−minus decay to form Yttrium−90.
Determine the missing values in the decay equation.
How did you do?
Fluorine−18 decays through beta−plus decay to form oxygen−18.
Determine the missing values in the decay equation.
How did you do?
Did this page help you?
State the meaning of the following terms:
(i) Binding energy.
[1]
(ii) Mass defect.
[1]
How did you do?
The nuclear rest mass of oxygen−16 is 15.994 914 u.
The mass defect, Δm, equation describes the relationship between the proton number, Z, the number of neutrons, N, the proton rest mass, mp, the neutron rest mass, mn, and the nuclear rest mass, mtotal.
Calculate the mass defect of oxygen−16. Give your answer to 6 d.p.
How did you do?
The mass defect (from part (b)) can be used to calculate the binding energy.
Calculate the total binding energy for a nucleus of oxygen−16 in J
How did you do?
Determine the binding energy per nucleon of oxygen−16 in J.
How did you do?
Did this page help you?
The chart shows the binding energy per nucleon for a number of nuclei.

Annotate the chart to show:
(i) Where fusion of these elements occurs to release energy
[1]
(ii) Where fission of these elements occurs to release energy
[1]
(iii) The location of Iron by drawing an X
[1]
How did you do?
In terms of the forces acting within the nucleus, explain why:
(i) Fusion occurs for nuclides with low nucleon numbers.
[2]
(ii) Fission occurs for nuclides with high nucleon numbers.
[2]
How did you do?
The graph shows the binding energy per nucleon in MeV plotted against nucleon number, A.

Use the graph to find the binding energy of the following nuclei.
(i) Platinum−190.
[1]
(ii) Silicon−28.
[1]
(iii) Tellurium−120.
[1]
How did you do?
Did this page help you?
The graph below shows the binding energy per nucleon against the number of nucleons in the nucleus.

There are three nuclei, labelled X, Y and Z, which do not sit on the line of the graph.
Match up the labels to the correct element by drawing a line between the boxes

How did you do?
Helium can fuse together to form beryllium as shown in the reaction below:

State and explain which is larger, the mass of the reactants or the mass of the products.
How did you do?
The table shows the mass of each reactant and daughter nucleus:

Using the information in the table:
(i) Calculate the mass of the reactants, mR in atomic mass units.
[2]
(ii) Calculate the mass defect, Δm, between the reactants and the daughter nuclei in atomic mass units.
[3]
How did you do?
Helium−3 and helium−4 fuse together to form beryllium−7.
The mass defect, Δm for this fusion reaction is equal to 2.8 × 10–30 kg.
Calculate the energy released, ΔE, in the fusion of beryllium−7.
How did you do?
Did this page help you?
The decay series of an isotope of thorium produces an isotope of radium
. This process involves four separate decays.
The first decay involves the emission of an alpha particle.
Determine the decay equation for this process, including the symbol of the daughter product.
How did you do?
The first decay can be represented on an N-Z diagram as an arrow from point A to point B.

Three more decays occur before is produced, denoted by “C” on the N-Z diagram.
Outline the possible sequence of decays which lead from point B to C.
How did you do?
Nuclei can be unstable for a number of reasons.
In terms of forces within the nucleus, explain why large nuclei emit alpha radiation.
How did you do?
then decays four more times, shown below.

The first three decays result in the emission of an alpha particle each time. The fourth and final decay results in the emission of a beta-plus particle.
Calculate the nucleon number and atomic number of nuclide A.
How did you do?
Did this page help you?
A radioactive source is used to measure the thickness of paper. A Geiger counter is used to measure the count rate on the opposite side of the paper to the radioactive source. The radioactive source used must be chosen carefully.
(i) State and explain the type of radioactive source that should be used for this process.
[2]
(ii) A new type of paper is placed between the Geiger counter and the radioactive source. Explain how the equipment can be used to show if the new paper is thicker or thinner than the previous type.
[2]
How did you do?
The arrangement below is used to maintain a constant 0.10 m thickness of aluminium sheets. Alpha, beta or gamma sources are available to be used.

Outline the most suitable radioactive source for this arrangement and explain why the other sources may not be appropriate.
How did you do?
The source used in part (b) has a half-life of 14 days and it has an initial count rate of 240 counts per minute when first used in the apparatus.
Giving your answer in weeks, calculate the length of time it takes for the Geiger counter to detect a count rate of 0.25 s–1.
How did you do?
Once the source has reached an activity of 0.25 s–1, it is replaced as the count rate of the source is comparable with that of background radiation.
State two natural sources of background radiation and two man-made sources of background radiation.
How did you do?
Did this page help you?
A sample’s count rate in counts per minute (cpm) is measured using a ray detector. This data is plotted on a graph.

(i) Use the graph to determine the half-life of this sample.
[2]
(ii) Explain why the distance between the detector and the source is a control variable.
[2]
How did you do?
The scientist wonders how the experiment in part (a) would have changed if the sample was twice the size.
Assuming the experiment from part (a) was repeated with a sample the exact same age but twice the mass, calculate the length of time it would have taken to reach a count rate of 22.5 cpm.
How did you do?
In reality the detector will measure a count rate of more than 5 cpm long after the length of time in part (b) has passed.
(i) Outline the reason for this larger-than-expected count rate.
[2]
(ii) Describe the measurements the scientist could take to accurately account for this additional count rate in the final data.
[2]
How did you do?
The scientist can measure the count rate of the source but is unable to directly measure the activity of the source using their detector. Activity is the total number of particles emitted from the sample per unit time.
Explain why this is not possible.
How did you do?
Did this page help you?
An unstable isotope of mercury, Hg-203, is tested for its radioactive emissions in a laboratory that has a background rate of 0.3 s–1.
A source is placed a fixed distance from a Geiger-Muller tube. Various materials are placed in between the detector and the source while the count rate is recorded. The results are shown below.
Material | Count rate / s–1 |
None | 68 |
0.5 mm thick paper | 69 |
2.0 mm thick paper | 65 |
5 cm thick aluminium foil | 15 |
State and explain what types of radiation are being emitted by the Hg-203 source.
How did you do?
A student notices that the count rate recorded actually increases when 0.5 mm thick paper was placed between the Geiger-Muller tube and the source.
(i) Suggest one cause of this increase.
[1]
(ii) Describe what the experimenter could do to check if this data point was anomalous.
[2]
How did you do?
Did this page help you?
Fluorescent tubes operate by exciting the electrons of mercury atoms.
The energy levels of a mercury atom are shown below (not to scale):

An electron is excited to n = 4. On the diagram, draw all the possible de-excitation routes from n = 4 to the ground state.
How did you do?
State and explain which energy transition will emit the photon with the lowest frequency.
How did you do?
An unstable isotope of mercury, Hg-203, is tested for its radioactive emissions in a laboratory that has a background rate of 0.3 s–1.
A source is placed a fixed distance from a Geiger-Muller tube. Various materials are placed in between the detector and the source while the count rate is recorded. The results are shown below.
Material | Count rate / s–1 |
None | 68 |
0.5 mm thick paper | 69 |
2.0 mm thick paper | 65 |
5 cm thick aluminium foil | 15 |
State and explain what types of radiation are being emitted by the Hg-203 source.
How did you do?
A student notices that the count rate recorded actually increases when 0.5 mm thick paper was placed between the Geiger-Muller tube and the source.
(i) Suggest one cause of this increase.
[1]
(ii) Describe what the experimenter could do to check if this data point was anomalous.
[2]
How did you do?
Did this page help you?
The image below shows how the binding energy per nucleon varies with nucleon number.

Fission and fusion are two nuclear processes in which energy can be released.
(i) On the image, mark the element with the highest binding energy per nucleon.
[1]
(ii) Explain why nuclei that undergo fission are restricted to a different part of the graph than those that undergo fusion.
[2]
How did you do?
Explain with reference to the figure in part (a), why the energy released per nucleon from fusion is greater than that from fission.
How did you do?
Explain how the binding energy of an oxygen nucleus can be calculated with information obtained in the figure from part (a).
How did you do?
The mass of an nucleus is 15.991 u.
Calculate:
(i) The mass difference, in kg, of the nucleus.
[2]
(ii) The binding energy, in MeV, of an oxygen nucleus.
[1]
How did you do?
Did this page help you?
Bismuth-214 () decays into Polonium-214 (
) by beta minus decay.
The binding energy per nucleon of Bismuth-214 is 7.774 MeV and the binding energy per nucleon of Polonium-214 is 7.785 MeV.
Beta-minus decay is described by the following equation:
Show that the energy released in the decay of bismuth is about 2.35 MeV and state where the energy comes from.
How did you do?
If an additional neutron is accelerated into the Polonium-214 () to produce the isotope Polonium-215 (
), use the following information to deduce the binding energy per nucleon of this new isotope.
Mass of nucleus = 3.571140 × 10−25 kg
How did you do?
Polonium-215 () is radioactive and decays by the producing alpha radiation, which is known to be a particularly stable.
Determine the binding energy of alpha radiation.
The following information is available:
Mass of a Helium-4 nucleus: 4.001265 u
How did you do?
A student claims that the amount of matter within a marble directly converted into energy would be enough to provide 1 year of current human energy consumption globally which is estimated to be 5.80 × 1018 J.
If the matter within marble is approximately 6.02 × 1023 u, determine if this statement is true, using the mass-energy equivalence.
How did you do?
Did this page help you?
Explain why the mass of an alpha-particle (α) is less than the total mass of two individual protons and two individual neutrons.
How did you do?
Show that the energy equivalence of 1.0 u is 931.5 MeV.
How did you do?
Data for the masses of some nuclei are given below
Nuclei | Mass / u |
Deuterium ( | 2.0141 |
Zirconium ( | 97.0980 |
Use the data to determine the binding energy of deuterium in MeV.
How did you do?
Using the data given in part (c), determine the binding energy per nucleon of zirconium in MeV.
How did you do?
Did this page help you?
A radioactive nucleus undergoes a beta−minus decay followed by an alpha decay to form a daughter nucleus
.
State the decay equation for this interaction and hence determine the values of A and Z.
How did you do?
Thorium, decays to an isotope of Radium (Ra) through a series of transformations. The particles emitted in successive transformations are:
Determine the resulting nuclide after these successive transformations.
How did you do?
Through a combination of successive alpha and beta decays, the isotope of any original nucleus can be formed.
Explain the simplest sequence of alpha and beta decays required to do this
How did you do?
A nucleus of Bohrium decays to Mendelevium
by a sequence of three alpha particle emissions.
Determine the number of neutrons in a nucleus of
How did you do?
Did this page help you?
The table shows some of the isotopes of phosphorus and, where they are unstable, the type of decay.
Isotope | |||||
Type of decay | stable |
|
Suggest whether the isotope is stable or not. If not, determine, with a reason, the type of decay it experiences.
How did you do?
The isotope of phosphorus decays into an isotope of silicon
.
(i) Determine the values of A and Z and write a decay equation for this process.
[2]
(ii) Explain why each emission product occurs.
[2]
How did you do?
Did this page help you?
The radioactive isotope uranium−238 decays in a decay series to the stable lead−206.
The half−life of is 4.5 × 109 years, which is much larger than all the other half−lives of the decays in the series.
A rock sample, when formed originally, contained 6.0 × 1022 atoms of and no
atoms. At any given time, most of the atoms are either
or
with a negligible number of atoms in other forms in the decay series.
Sketch on the axes below the variation of number of atoms and the number of
atoms in the rock sample as they vary over a period of 1.0 × 1010 years from its formation. Label the lines U and Pb.

How did you do?
A certain time, t, after its formation, the sample contained twice as many atoms as
atoms.
Show that the number of atoms in the rock sample at time t was 4.0 × 1022.
How did you do?
Lead−214 is an unstable isotope of lead−206. It decays by emitting a particle to form bismuth−214 (Bi)
Bismuth is also unstable and has two decay modes:
Emitting an α particle to form thallium−210 (Tl) + energy
Emitting a β particle to form polonium−214 (Po) + energy
Write decay equations for the decay chain of lead−214 to thallium−210 and to polonium−214. Comment on the nature of the energy released.
How did you do?
Did this page help you?
Xenon−140 is one of the waste products from the fission of uranium-235. Xenon−140 is radioactive and decays through
decay.
The graph shows the variation with time of the mass of 1kg of xenon−140 remaining in the sample.

(i) Calculate the proton and mass numbers of nuclide Z.
[1]
(ii) Calculate the mass of xenon−140 remaining in the sample after 2.5 minutes
[3]
How did you do?
An alternative nuclear fuel to the traditionally used uranium-235 is thorium-232. When thorium-232 is exposed to neutrons, it will undergo a series of nuclear reactions until it eventually emerges as an isotope of uranium-233, which will readily split and release energy the next time it absorbs a neutron.
Part of the thorium fuel cycle is shown below.
Once the uranium-233 nucleus absorbs a neutron, it undergoes fission, releasing energy and two neutrons and forming the fission products Xenon and Strontium as in parts a-c. Any isotopes of uranium-233 which do not undergo fission decay through a chain ending with a stable nucleus of thallium-205 .
Show that 12 particles, not including neutrons, are emitted during this combination of decay chains. Explain your reasoning.
How did you do?
Did this page help you?