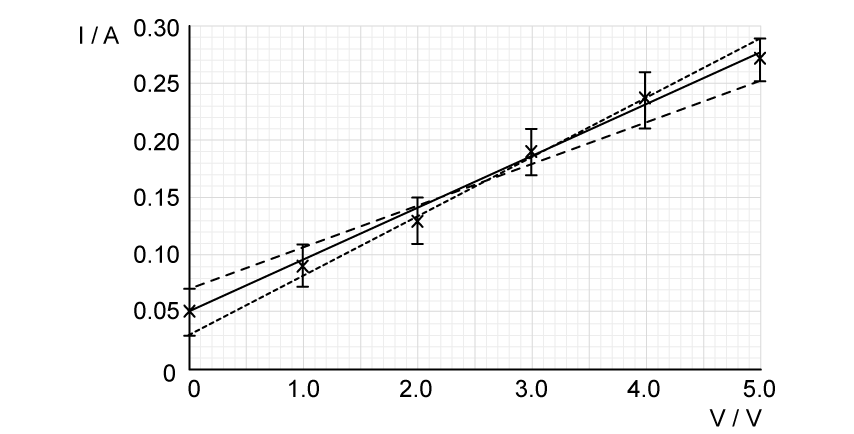
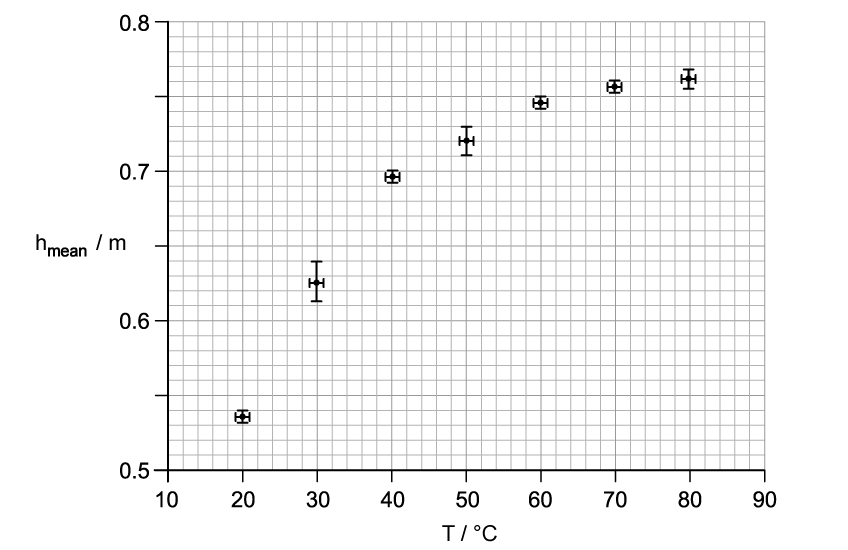
A student uses a stopwatch to measure the time taken for a pendulum to complete one swing.
The diagram shows the display on the stopwatch after the pendulum completes 10 swings.

For this reading, determine:
(i) the absolute uncertainty
[1]
(ii) the fractional uncertainty
[1]
(iii) the percentage uncertainty.
[1]
Calculate the mean time for one complete swing with its absolute uncertainty and percentage uncertainty.
Give your answer to an appropriate number of significant figures.
When carrying out the same experiment, another student measured the time taken for the pendulum to complete 20 swings instead of 10.
Explain how this procedure leads to a more accurate measurement of the time for one oscillation.
Did this page help you?