Resistivity (DP IB Physics: SL)

Resistance, Length and Cross-Sectional Area

• The resistance of a sample depends on:
  • The material it is made of
  • The length of the sample
  • The cross-sectional area of the sample

• The resistance of a conductor (e.g. a wire) is:
  • Directly proportional to its length
  • Inversely proportional to its cross-sectional area 

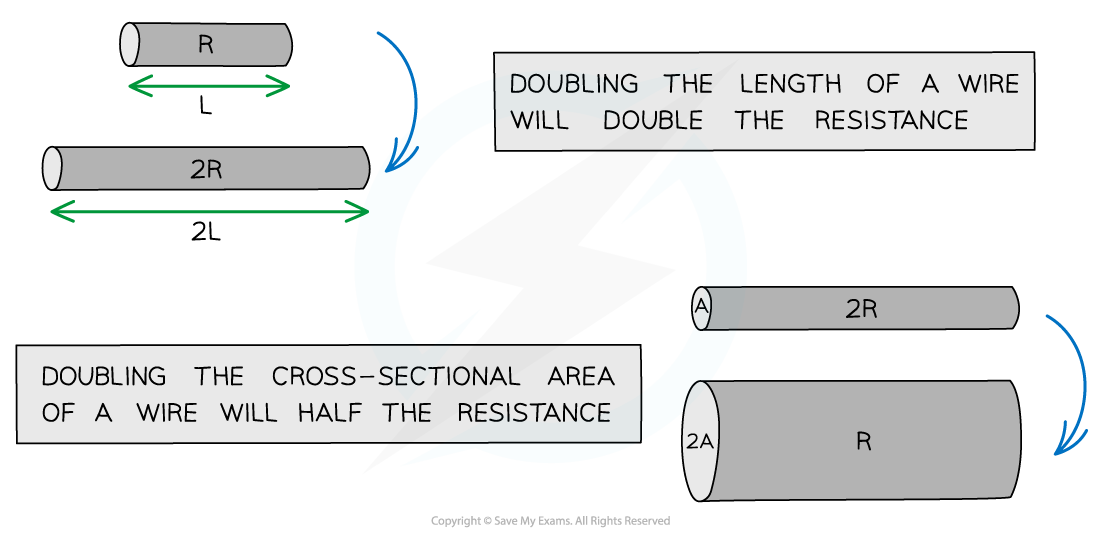
How the length and width of a wire affect its resistance

Defining Resistivity

• This leads to the definition of a new quantity, called resistivity
• Resistivity is a property describing the extent to which a material opposes the flow of electric current through it
• It is defined as follows:

The resistivity of a material is equal to the resistance per unit length of a material with unit cross-sectional area 

• The equation for the resistivity is:

• Where:
  • ρ = resistivity in ohm-metres (Ω m)
  • R = resistance in ohms (Ω)
  • A = cross sectional area of material in square metres (m²)
  • L = length of material in metres (m)

Resistivity of Conductors and Insulators

• Resistivity is dependent on temperature
  • In conductors, the resistivity increases with increasing temperature
  • In insulators, the resistivity decreases with increasing temperature

• Since ρ ∝ R, when a wire or the filament inside a lamp heat up, their resistance increases

Resistivity of some materials at room temperature

• Conductors have the lowest values of resistivity
• Wires are made from copper because of its relatively low resistivity at room temperature
• Insulators have such a high resistivity that virtually no current will flow through them