Revision NotesExam QuestionsFlashcardsPast Papers
IBPhysicsDPHLRevision NotesWave BehaviourSimple Harmonic MotionCalculating Energy Changes in SHM

Calculating Energy Changes in SHM (DP IB Physics): Revision Note

Katie M

Author

Katie M

Last updated

Calculating Energy Changes in Simple Harmonic Motion

Equations for Energy in SHM

  • Potential energy:

E subscript P space equals space 1 half m omega squared x squared

  • Total energy: 

E subscript T space equals space 1 half m omega squared x subscript 0 squared

  • The kinetic energy–displacement relation for SHM is:

E subscript K space equals space 1 half m omega squared open parentheses x subscript 0 squared minus x squared close parentheses

  • Where:

    • m = mass (kg)

    • ω = angular frequency (rad s−1)

    • x0 = amplitude (m)

Calculating Total Energy in SHM

  • Using the expression for the velocity v of a simple harmonic oscillator that begins oscillating from its equilibrium position:

v space equals space omega x subscript 0 cos space open parentheses omega t space plus space capital phi close parentheses

  • Where:

    • phase difference, Φ = 0 

    • v = velocity of oscillator (m s−1)

    • ⍵ = angular frequency (rad s−1)

    • x0 = amplitude (m)

    • t = time (s)

  • The kinetic energy EK of an oscillator can be written as:

E subscript K space equals space 1 half m v squared

E subscript K space equals space 1 half m open parentheses omega x subscript 0 cos space open parentheses omega t close parentheses close parentheses squared

E subscript K space equals space 1 half m omega squared x subscript 0 squared space cos squared space open parentheses omega t close parentheses

  • Since the maximum value of sin space open parentheses omega t close parentheses or cos space open parentheses omega t close parentheses is 1, maximum kinetic energy is given by:

E subscript K left parenthesis m a x right parenthesis end subscript space equals space 1 half m omega squared x subscript 0 squared

  • When the kinetic energy of the system is at a maximum, the potential energy is zero

    • Hence this represents the total energy of the system

  • The total energy ET of a system undergoing simple harmonic motion is, therefore, defined by:

E subscript T space equals space 1 half m omega squared x subscript 0 squared

  • Where:

    • ET = total energy of a simple harmonic system (J)

    • m = mass of the oscillator (kg)

    • ⍵ = angular frequency (rad s−1)

    • x0 = amplitude (m)

  • Note: The same expression for total energy will be achieved if the other expression for velocity is used, for an object that begins oscillation at t = 0 from the amplitude position:

v space equals space minus omega x subscript 0 sin space open parentheses omega t close parentheses

Calculating Potential Energy in SHM

  • An expression for the potential energy of a simple harmonic oscillator can be derived using the expressions for velocity and displacement for an object starting its oscillations when t = 0 in the equilibrium position, so x = 0:

x space equals space x subscript 0 sin space open parentheses omega t close parentheses

v space equals space omega x subscript 0 cos space open parentheses omega t close parentheses

sin squared space open parentheses omega t close parentheses space plus space cos squared space open parentheses omega t close parentheses space equals space 1

  • In a simple harmonic oscillation, the total energy of the system is equal to:

Total energy = Kinetic energy + Potential energy

E subscript T space equals space E subscript K space plus space E subscript P

  • The potential energy of an oscillator can be written as:

E subscript P space equals space E subscript T space minus space E subscript K

E subscript P space equals space 1 half m omega squared x subscript 0 squared space minus space 1 half m v squared

  • Substitute in for v:

E subscript P space equals space 1 half m omega squared x subscript 0 squared space minus space 1 half m open parentheses omega x subscript 0 cos space open parentheses omega t close parentheses close parentheses squared

E subscript P space equals space 1 half m omega squared x subscript 0 squared space minus space 1 half m omega squared x subscript 0 squared cos squared space open parentheses omega t close parentheses

  • Taking out a factor of 1 half m omega squared x subscript 0 squared spacegives:

E subscript P space equals space 1 half m omega squared x subscript 0 squared space open parentheses 1 space minus space cos squared space open parentheses omega t close parentheses close parentheses

E subscript P space equals space 1 half m omega squared x subscript 0 squared sin squared space open parentheses omega t close parentheses

E subscript P space equals space 1 half m omega squared open square brackets x subscript 0 sin space open parentheses omega t close parentheses close square brackets squared

  • Since x space equals space x subscript 0 sin space open parentheses omega t close parentheses, the potential energy of the system can be written as:

E subscript P space equals space 1 half m omega squared x squared

  • Since the maximum potential energy occurs at the maximum displacement open parentheses x space equals space x subscript 0 close parentheses of the oscillation,

E subscript P left parenthesis m a x right parenthesis end subscript space equals space 1 half m omega squared x subscript 0 squared

  • Therefore, it can be seen that:

E subscript T space equals space E subscript K left parenthesis m a x right parenthesis end subscript space equals space E subscript P left parenthesis m a x right parenthesis end subscript

Kinetic Energy–Displacement Relation for SHM

  • Using the displacement–velocity relation for SHM:

v space equals space plus-or-minus omega square root of x subscript 0 squared minus x squared end root

  • Substituting into the equation for kinetic energy:

E subscript K space equals space 1 half m v squared

E subscript K space equals space 1 half m open parentheses omega square root of x subscript 0 squared minus x squared end root close parentheses squared

  • This leads to the kinetic energy–displacement relation for SHM:

E subscript K space equals space 1 half m omega squared open parentheses x subscript 0 squared minus x squared close parentheses

Worked Example

A ball of mass 23 g is held between two fixed points A and B by two stretched helical springs, as shown in the diagram below.

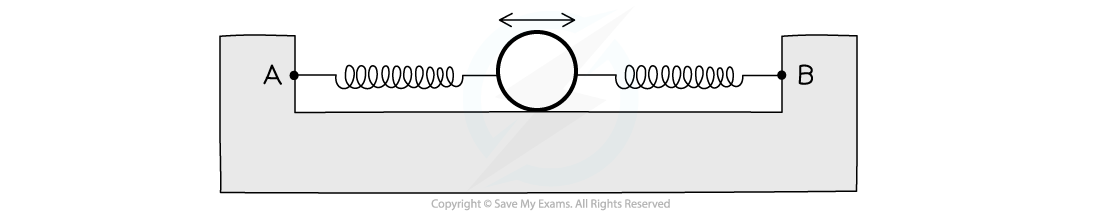
Worked example horizontal mass on spring, downloadable AS & A Level Physics revision notes

The ball oscillates with simple harmonic motion along line AB. The oscillations are of frequency 4.8 Hz and amplitude 1.5 cm.

Calculate the total energy of the oscillations.

Answer:

Step 1: Write down the known quantities 

  • Mass, m = 23 g = 23 × 10–3 kg

  • Amplitude, x subscript 0 = 1.5 cm = 0.015 m

  • Frequency, f = 4.8 Hz

Step 2: Write down the equation for the total energy of SHM oscillations:

E space equals space 1 half m omega squared x subscript 0 superscript 2

Step 3: Write an expression for the angular frequency

omega space equals space 2 pi f space equals space 2 pi space cross times space 4.8

Step 4: Substitute values into the energy equation

E space equals space 1 half space cross times space left parenthesis 23 space cross times space 10 to the power of negative 3 end exponent right parenthesis space cross times space left parenthesis 2 straight pi space cross times space 4.8 right parenthesis squared space cross times space left parenthesis 0.015 right parenthesis squared

Total energy:  E = 2.354 × 10–3 = 2.4 mJ

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourselfFlashcards

    Did this page help you?

    Previous:Equations for Simple Harmonic Motion (SHM)Next:Phase Angles in Simple Harmonic Motion (SHM)
    Katie M

    Author: Katie M

    Expertise: Physics

    Katie has always been passionate about the sciences, and completed a degree in Astrophysics at Sheffield University. She decided that she wanted to inspire other young people, so moved to Bristol to complete a PGCE in Secondary Science. She particularly loves creating fun and absorbing materials to help students achieve their exam potential.