Revision NotesExam QuestionsFlashcardsPast Papers
IBPhysicsDPHLRevision NotesThe Particulate Nature of MatterThermodynamicsCalculating Changes in Entropy

Calculating Changes in Entropy (DP IB Physics)

Revision Note

Katie M

Author

Katie M

Last updated

Calculating Changes in Entropy

  • At a constant temperature T, the change in entropy on a macroscopic level can be calculated using the equation

increment S space equals space fraction numerator increment Q over denominator T end fraction

  • Where:

    • ΔS = change in entropy (J K−1)

    • ΔQ = heat given to or removed from the system (J)

    • T = temperature of the system (K)

  • When heat is given to a system (ΔQ = positive), entropy increases

increment S space equals space plus fraction numerator increment Q over denominator T end fraction

increment Q space greater than space 0

increment S space greater than space 0

  • When heat is removed from a system (ΔQ = negative), entropy decreases

increment S space equals space minus fraction numerator increment Q over denominator T end fraction

increment Q space less than space 0

increment S space less than space 0

  • For a reversible process that returns the system to its original state, entropy is constant

increment Q space equals space 0

increment S space equals space 0

Entropy & Microstates

  • The entropy of a system, on a microscopic level, can be calculated using the equation

S space equals space k subscript B space ln space straight capital omega

  • Where:

    • S = entropy of a system of microscopic particles (J K−1)

    • kB = the Boltzmann constant

    • Ω = the number of possible microstates of the system

  • Similarly, the change in entropy when the number of microstates increases from straight capital omega subscript 1 to straight capital omega subscript 2 is given by 

increment S space equals space k subscript B space ln space straight capital omega subscript 2 over straight capital omega subscript 1

  • A microstate describes one state or possible arrangement of the particles in the system 

    • A state can be defined by any microscopic or macroscopic property that is known about the system e.g. positions or velocities of molecules, energy, volume etc.

  • An example that helps illustrate this is a two-compartment container which holds N distinguishable particles (i.e. each particle can be identified individually)

  • Initially, all N particles are sealed in one of two compartments

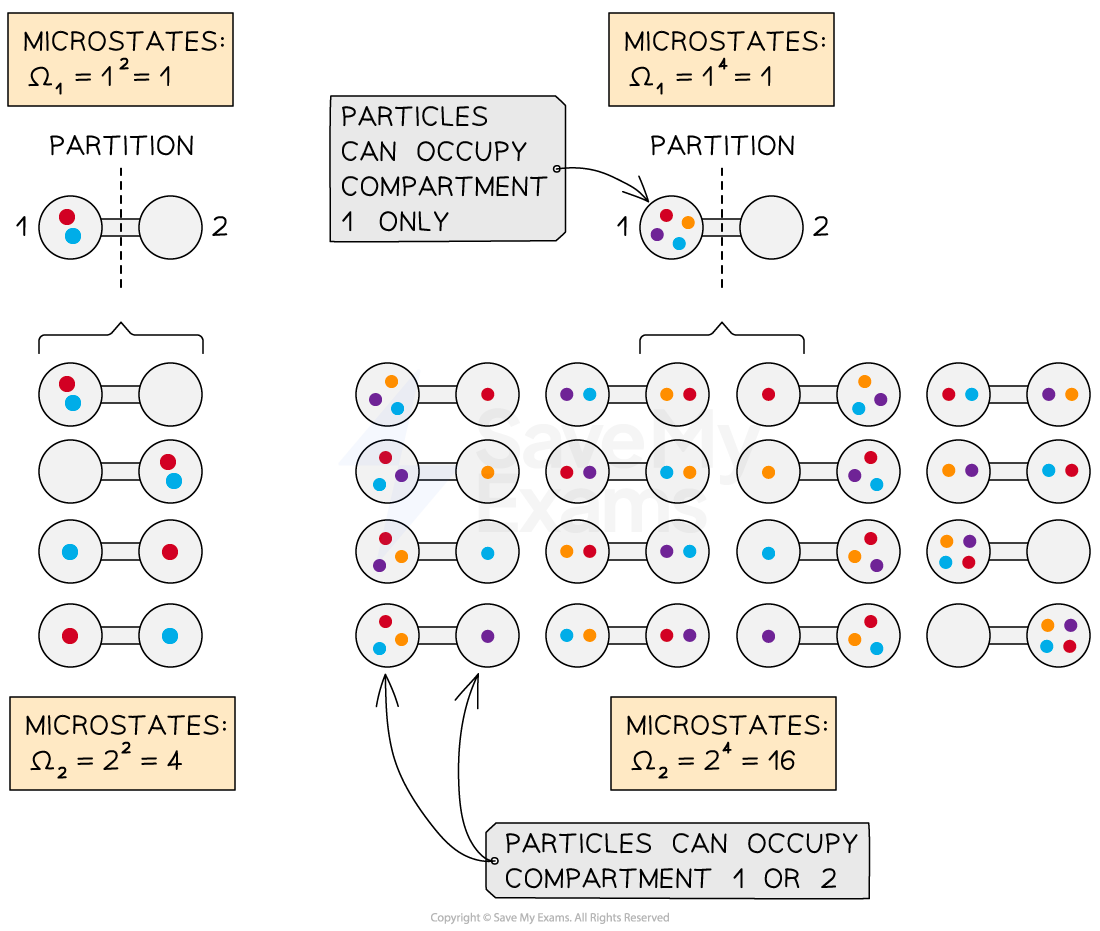
2-4-4-entropy-microstates-example

The number of possible microstates describes the number of different possible arrangements of particles in a system

  • When the particles are confined to one compartment, we know the location of all the particles

    • Therefore, the number of microstates (possible arrangements) in the initial volume is straight capital omega subscript 1 space equals space 1 to the power of N space equals space 1

    • It is always equal to 1, for example, when N = 2 or N = 4:  straight capital omega subscript 1 space equals space 1 squared space equals space 1 to the power of 4 space equals space 1

  • Once the partition is removed, the particles can spread out and occupy either one of the two compartments

    • The number of microstates (possible arrangements) in the final volume is straight capital omega subscript 2 space equals space 2 to the power of N

    • For example, when N = 2, the particles can be arranged 22 = 4 different ways

    • Or, when N = 4, the particles can be arranged 24 = 16 different ways

  • The change in the entropy is therefore:

increment S space equals space k subscript B space ln space open parentheses straight capital omega subscript 2 over straight capital omega subscript 1 close parentheses space equals space k subscript B space ln space open parentheses 2 to the power of N over 1 to the power of N close parentheses

increment S space equals space k subscript B space ln space open parentheses 2 to the power of N close parentheses

  • It follows that the number of possible microstates can be equated to macroscopic properties of the gas, such as its volume increasing from V to 2V

  • As the gas expands, the space it can occupy doubles, hence it gains an amount of entropy equal to:

increment S space equals space N k subscript B space ln space open parentheses 2 V close parentheses space minus space N k subscript B space ln space open parentheses V close parentheses

increment S space equals space N k subscript B space ln space open parentheses fraction numerator 2 V over denominator V end fraction close parentheses space equals space N k subscript B space ln space open parentheses 2 close parentheses

  • This gives the same result as above:

increment S space equals space k subscript B space ln space open parentheses 2 to the power of N close parentheses

Examiner Tips and Tricks

Entropy is an incredibly important topic in physics and underpins many fundamental ideas from quantum mechanics to the determination of the Schwarzchild radius of a black hole, so don't worry if you feel a bit lost at first as it is quite a challenging concept to get your head around initially!

You might find it useful to think of microstates as a way of quantifying the certainty of information we have about the system

For example:

  • A solid has lower entropy than a gas because we can be more certain about the location of the atoms in the solid

  • A gas at a higher temperature (or pressure or volume) has a higher entropy than a similar gas at a lower temperature (or pressure or volume) because we become less certain about the location of the atoms by further increasing the possible locations they could occupy

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourselfFlashcards

    Did this page help you?

    Previous:EntropyNext:Second Law of Thermodynamics
    Katie M

    Author: Katie M

    Expertise: Physics

    Katie has always been passionate about the sciences, and completed a degree in Astrophysics at Sheffield University. She decided that she wanted to inspire other young people, so moved to Bristol to complete a PGCE in Secondary Science. She particularly loves creating fun and absorbing materials to help students achieve their exam potential.