Reference Frames (DP IB Physics) : Revision Note
Reference Frames
The term relative is used often in Physics to make it clear which point of view we are referring to
For example, the velocity of a car relative to someone stationary is different to the velocity measured by another car travelling alongside the initial car at the same speed
A reference frame, or a frame of reference, refers to the position of an object, it is defined as:
A set of coordinates to record the position and time of events
For example, you currently sitting on your chair at your desk is your current reference frame
You feel as if you are stationary, despite the fact the Earth is revolving on its axis and orbiting the sun
It is the point of view where an object, at a specific co-ordinate, is at rest
Examples of Reference Frames
An everyday example is the direction of an object from your point of view in comparison to someone else
In this example, a car is driving down a road and two people are standing on opposite sides of that road
Despite the car moving in one direction, each person will view its direction relative to them differently
The person on one side of the road would say the car is moving to the right, and the person on the other side of the road would say the car is moving to the left
Both are correct, but they are viewing the car's motion from different points of reference
Diagram showing different points of reference for a moving car
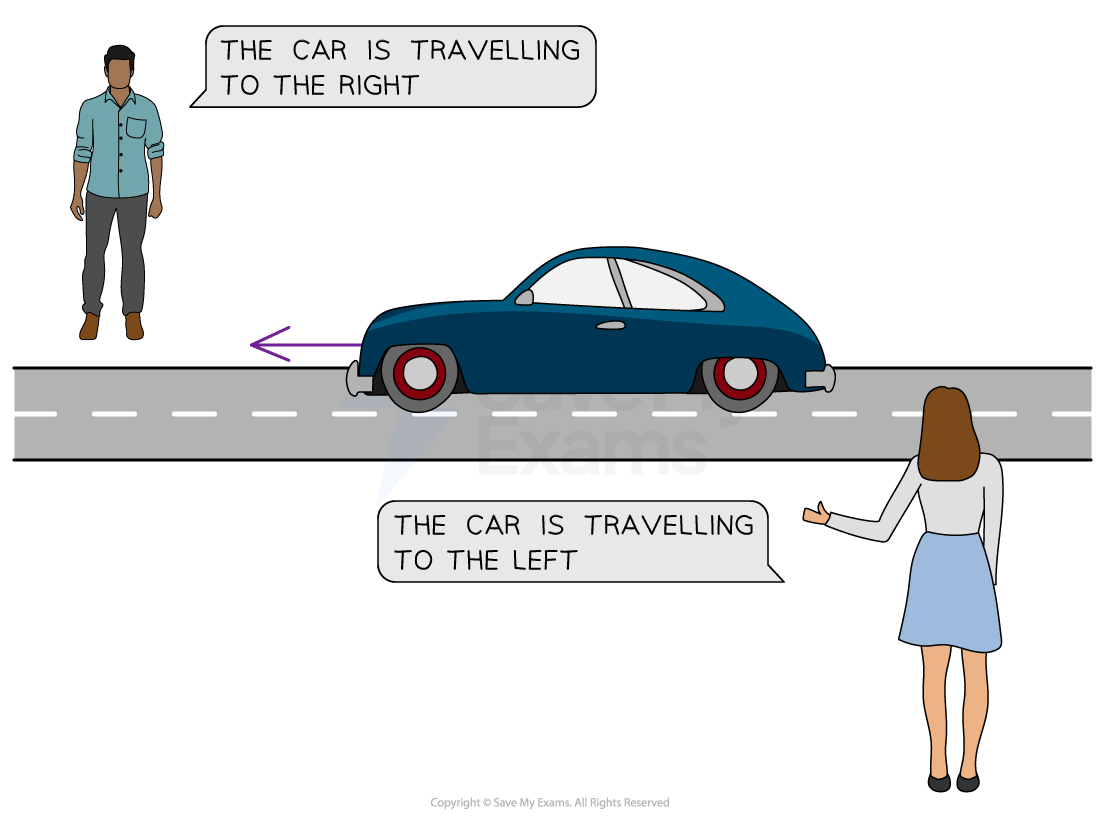
Each person has a different frame of reference, so they interpret the direction of the car differently relative to themselves
Another common example is of a train pulling out of a station, where Person A is on the platform and Person B is on the train
As the train begins to move, Person A, on the platform, views Person B, on the train, moving to the right
Therefore, according to Person A, they, themselves, are stationary and Person B is moving to the right
Things look a little different from Person B's perspective
As the train begins to move (to the left from Person B's perspective), Person B, on the train, views Person A, on the platform, moving to the right
Therefore, according to Person B, they, themselves, are stationary (as they cannot feel the train moving to the left) and Person A is moving to the right
Diagram demonstrating different reference frames for a train leaving a station
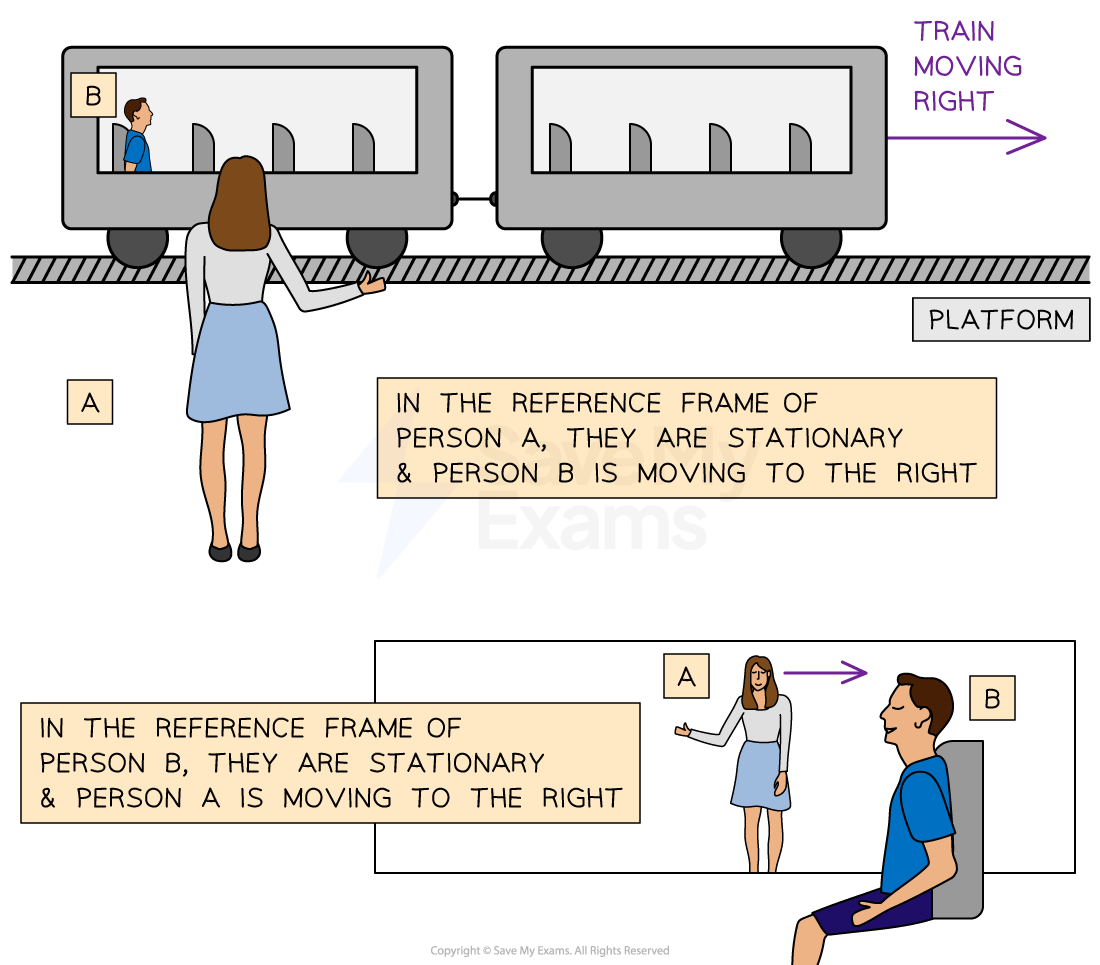
Person A and B are both stationary in their own reference frames and see the other as moving
Therefore, frames of reference are used to specify the relationship between a moving and stationary object
Inertial Frames of Reference
An inertial reference frame is
A reference frame that is non-accelerating
Therefore, all inertial reference frames are moving at constant velocity with respect to each other
There is no such thing as an absolute reference frame in our Universe
In other words, there is no place in the Universe that is completely stationary
Everything is always moving relative to everything else
Worked Example
A student is cycling to school with their friend who is also cycling exactly in line. As they cycle past a bus stop, they wave to their aunt who is stationary at the bus stop as she waits for her bus.
The student's aunt estimates the speed of the students to be 5 m s–1.
At what speed would the friend measure the student to be travelling?
A. 5 m s–1
B. -5 m s–1
C. 0 m s–1
D. 2 m s–1
Answer:
The correct answer is C because:
We must think about the friend's reference frame for this question, in which they are stationary (according to them)
Since the friend is cycling in line with the student, this means they measure the student to be travelling at 0 m s–1 relative to them
Examiner Tips and Tricks
In exam questions, look out for terms such as 'for the reference frame of...', 'in the reference frame of...' or 'relative to ...' to know which reference frame is being referred to. You can think of it as 'What do they see from their point of view?'. This becomes important when you learn about Galilean relativity and Lorentz transformations.
You will not come across non-inertial reference frames (i.e. ones where a frame is accelerating) in your exam.

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
