Revision NotesExam QuestionsFlashcardsPast Papers
IBPhysicsDPHLRevision NotesFieldsGravitational FieldsGravitational Potential Energy Equation

Gravitational Potential Energy Equation (DP IB Physics)

Revision Note

Katie M

Author

Katie M

Last updated

Work Done on a Mass

  • When a mass is moved against the force of gravity, work is required

    • This is because gravity is attractive, therefore, energy is needed to work against this attractive force

  • The work done in moving a mass m is given by:

increment W space equals space m increment V subscript g

  • Where:

    • ΔW = change in work done (J)

    • m = mass (kg)

    • ΔVg = change in gravitational potential (J kg−1)

Worked Example

A particle of mass 50 g is moved vertically from point A to point B, as shown in the diagram.

Take the gravitational field strength to be 10 N kg−1.

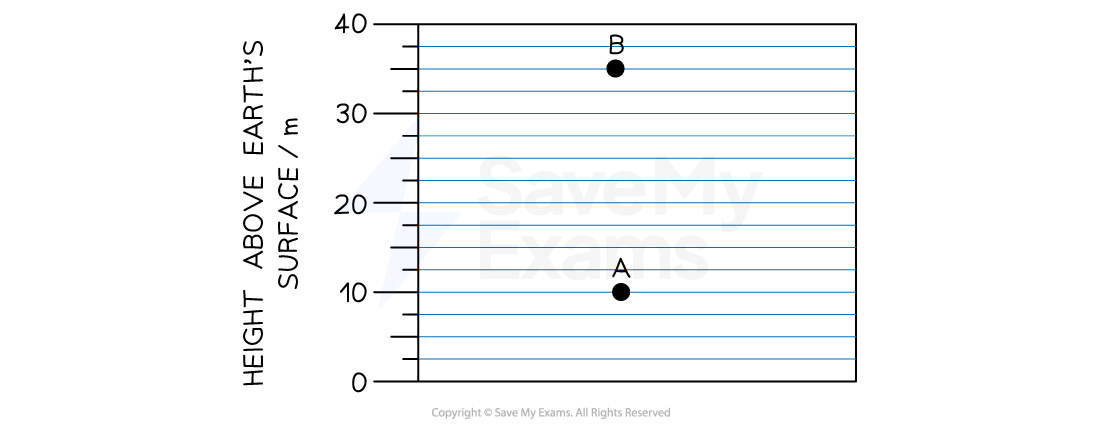
4-1-6-work-done-moving-a-mass

Determine 

(a) the potential difference between A and B

(b) the work done in moving the mass from A to B

Answer:

(a)

  • The work done in moving a mass in a gravitational field is:

W space equals space m increment V and W space equals space m g increment h (close to the Earth's surface)

m increment V space equals space m g increment h space space space space space rightwards double arrow space space space space space increment V space equals space g increment h

  • Where the change in height is increment h = 35 − 10 = 25 m

  • Therefore, the potential difference between A and B is:

increment V space equals space 10 space cross times space 25 space equals space 250 space straight J space kg to the power of negative 1 end exponent

(b)

  • The work done in moving the mass from A to B is:

W space equals space m increment V

W space equals space open parentheses 50 cross times 10 to the power of negative 3 end exponent close parentheses space cross times space 250 space equals space 12.5 space straight J

Gravitational Potential Energy Equation

  • In a radial field, gravitational potential energy (GPE) describes the energy an object possesses due to its position in a gravitational field

  • The gravitational potential energy of a system is defined as:

    The work done to assemble the system from infinite separation of the components of the system

  • Similarly, the gravitational potential energy of a point mass is defined as:

    The work done in bringing a mass from infinity to a point

  • The equation for GPE of two point masses m and M at a distance r is:

E subscript p space equals space minus fraction numerator G m subscript 1 m subscript 2 over denominator r end fraction

  • Where:

    • G = universal gravitational constant (N m2 kg–2)

    • m1 = larger mass producing the field (kg)

    • m2 = mass moving within the field of M (kg)

    • r = distance between the centre of m and M (m)

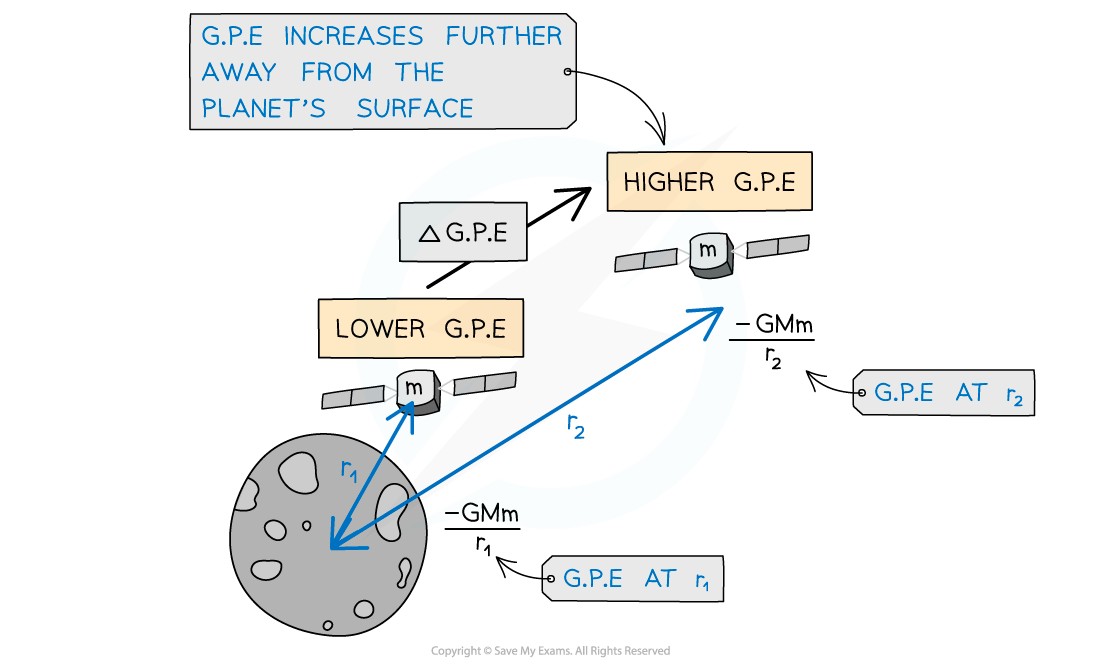
Change in GPE, downloadable AS & A Level Physics revision notes

Gravitational potential energy increases as a satellite leaves the surface of the Moon (of mass M)

  • Recall that Newton's Law of Gravitation relates the magnitude of the force F between two masses M and m:

F space equals space fraction numerator G m subscript 1 m subscript 2 over denominator r squared end fraction

  • Therefore, a force-distance graph would be a curve, because F is inversely proportional to r2, or:

F space proportional to space 1 over r squared 

  • The product of force and distance is equal to work done (or energy transferred) 

  • Therefore, the area under the force-distance graph for gravitational fields is equal to the work done

    • In the case of a mass m moving further away from a mass M, the potential increases

    • Since gravity is attractive, this requires work to be done on the mass m

    • The area between two points under the force-distance curve, therefore, gives the change in gravitational potential energy of mass m

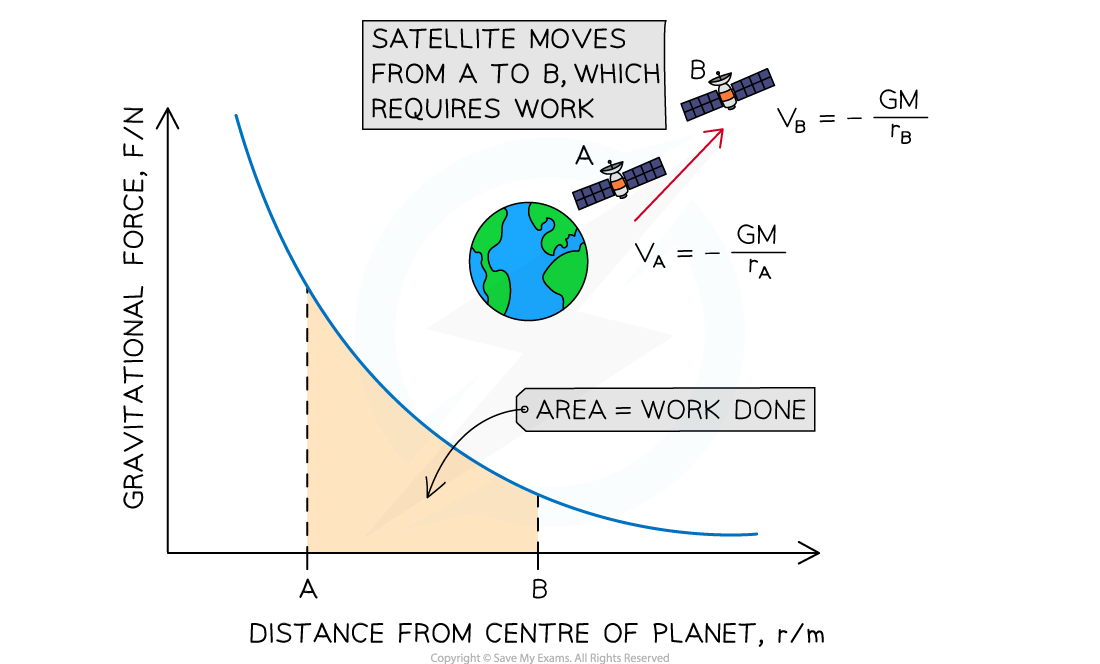
5-9-3-force-distance-graph_ocr-al-physics

Work is done on the satellite of mass m to move it from A to B, because gravity is attractive. The area under the curve represents the magnitude of energy transferred

Change in Gravitational Potential Energy

  • Two points at different distances from a mass will have different gravitational potentials

    • This is because the gravitational potential increases with distance from a mass

  • Therefore, there will be a gravitational potential difference ΔV between the two points

increment V space equals space V subscript f space minus space V subscript i

  • Where:

    • V subscript i = initial gravitational potential (J kg–1)

    • V subscript f = final gravitational potential (J kg–1)

  • The change in work done against a gravitational field is equal to the change in gravitational potential energy (GPE)

    • When V = 0, then the GPE = 0

  • It is usually more useful to find the change in the GPE of a system

    • For example, a satellite lifted into space from the Earth’s surface

  • The change in GPE when a mass moves towards, or away from, another mass is given by:

increment E subscript p space equals space minus fraction numerator G m subscript 1 m subscript 2 over denominator r subscript 2 end fraction space minus space open parentheses negative fraction numerator G m subscript 1 m subscript 2 over denominator r subscript 1 end fraction close parentheses

increment E subscript p equals space G m subscript 1 m subscript 2 open parentheses 1 over r subscript 1 minus 1 over r subscript 2 close parentheses

  • Where:

    • m1 = mass that is producing the gravitational field (e.g. a planet) (kg)

    • m2 = mass that is moving in the gravitational field (e.g. a satellite) (kg)

    • r1 = first distance of m from the centre of M (m)

    • r2 = second distance of m from the centre of M (m)

  • The change in potential ΔVg is the same, without the mass of the object m2:

increment V subscript g space equals space minus fraction numerator G m subscript 1 over denominator r subscript 2 end fraction space minus space open parentheses negative fraction numerator G m subscript 1 over denominator r subscript 1 end fraction close parentheses

increment V subscript g space equals space G m subscript 1 open parentheses 1 over r subscript 1 minus 1 over r subscript 2 close parentheses

  • Work is done when an object in a planet's gravitational field moves against the gravitational field lines i.e. away from the planet

Worked Example

A spacecraft of mass 300 kg leaves the surface of Mars up to an altitude of 700 km.

Calculate the work done by the spacecraft. 

  • Radius of Mars = 3400 km

  • Mass of Mars, m1 = 6.40 × 1023 kg

Answer:

  • The change in GPE is equal to

increment E subscript p space equals space G m subscript 1 m subscript 2 open parentheses 1 over r subscript 1 minus 1 over r subscript 2 close parentheses

  • Where

    • r1 = radius of Mars = 3400 km

    • r2 = radius + altitude = 3400 + 700 = 4100 km

increment E subscript p space equals space left parenthesis 6.67 cross times 10 to the power of negative 11 end exponent right parenthesis space cross times space left parenthesis 6.40 cross times 10 to the power of 23 right parenthesis space cross times space 300 space cross times space open parentheses fraction numerator 1 over denominator 3400 cross times 10 cubed end fraction minus fraction numerator 1 over denominator 4100 cross times 10 cubed end fraction close parentheses

    Work done by satellite:  increment E subscript p space equals space 643.1 cross times 10 to the power of 6 space equals space 640 space MJ (2 s.f.)

Worked Example

A satellite of mass 1450 kg moves from an orbit of 980 km above the Earth’s surface to a lower orbit of 480 km.

Calculate the change in gravitational potential energy of the satellite.

  • Mass of the Earth = 5.97 × 1024 kg

  • Radius of the Earth = 6.38 × 106 m

Answer:

Step 1: Write down the known quantities

  • Initial height above Earth’s surface, h1 = 980 km

  • Final height above Earth’s surface, h2 = 480 km

  • Mass of the satellite, m1 = 1450 kg

  • Mass of the Earth, m2 = 5.97 × 1024 kg

  • Radius of the Earth, R = 6.38 × 106 m

Step 2: Write down the equation for change in gravitational potential energy

increment E subscript p space equals space G m subscript 1 m subscript 2 open parentheses 1 over r subscript 1 minus 1 over r subscript 2 close parentheses

Step 3: Convert distances into standard units and include Earth radius

  • Distance from centre of Earth to higher orbit:

r subscript 1 space equals space h subscript 1 space plus space R

r subscript 1 = (980 × 103) + (6.38 × 106) = 7.36 × 106 m

  • Distance from centre of Earth to lower orbit:

r subscript 2 space equals space h subscript 2 space plus space R

r subscript 2 = (480 × 103) + (6.38 × 106) = 6.86 × 106 m

Step 4: Substitute values into the equation

increment E subscript p space equals space open parentheses 6.67 cross times 10 to the power of negative 11 end exponent close parentheses space cross times space open parentheses 5.97 cross times 10 to the power of 24 close parentheses space cross times space 1450 space cross times space open parentheses fraction numerator 1 over denominator 7.36 cross times 10 to the power of 6 end fraction minus fraction numerator 1 over denominator 6.86 cross times 10 to the power of 6 end fraction close parentheses

Change in gravitational potential energy:  ΔEp = 5.72 × 109 J

Examiner Tips and Tricks

Make sure to not confuse the ΔEp equation with ΔE = mgΔh, they look similar but refer to quite different situations.

The more familiar equation is only relevant for an object lifted in a uniform gravitational field, meaning very close to the Earth’s surface, where we can model the field as uniform.

The new equation for Ep  does not include g. The gravitational field strength, which is different on different planets, does not remain constant as the distance from the surface increases. Gravitational field strength falls away according to the inverse square law.

The change in gravitational potential energy is the work done.

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourselfFlashcards

    Did this page help you?

    Previous:Gravitational Potential Energy in a Non-Uniform FieldNext:Gravitational Potential Gradient
    Katie M

    Author: Katie M

    Expertise: Physics

    Katie has always been passionate about the sciences, and completed a degree in Astrophysics at Sheffield University. She decided that she wanted to inspire other young people, so moved to Bristol to complete a PGCE in Secondary Science. She particularly loves creating fun and absorbing materials to help students achieve their exam potential.