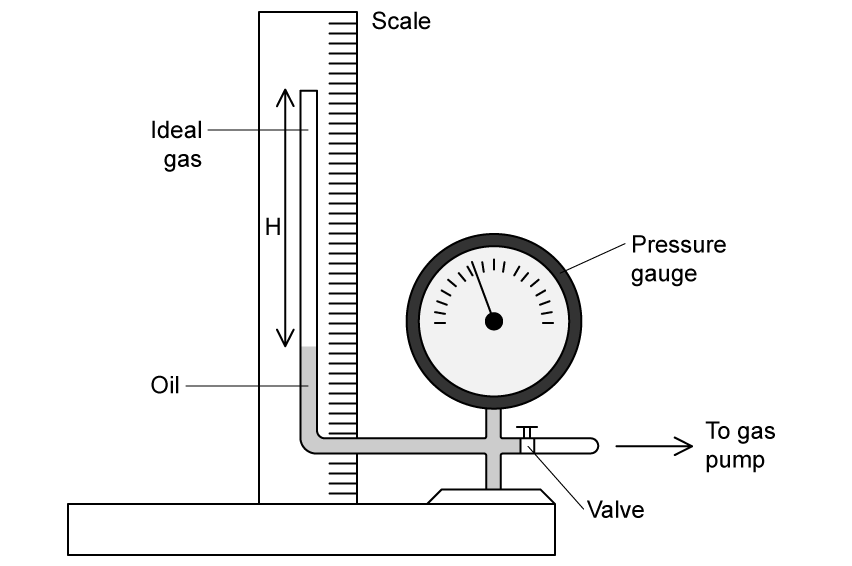
Define the mole.
4.7 × 1023 molecules of neon gas is trapped in a cylinder.
Calculate the number of moles of neon gas in the cylinder.
The molar mass of neon gas is 20 g mol–1.
Calculate the mass of the neon gas in the cylinder.
The cylinder containing the neon gas has a volume 5.2 m3 and pressure of 600 Pa.
Calculate the temperature of the gas.
Did this page help you?