The Doppler Equation

Calculating Doppler Shift

When a source of sound waves moves relative to a stationary observer, the observed frequency can be calculated using the equation below:

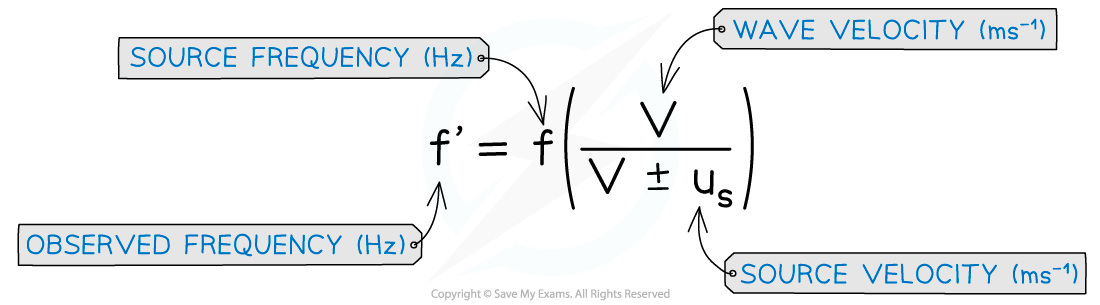
9-5-3-doppler-calculation-1-ib-hl

Doppler shift equation for a moving source

The wave velocity for sound waves is 340 ms^-1
The ± depends on whether the source is moving towards or away from the observer
- If the source is moving towards the observer, the denominator is v - u_s
- If the source is moving away from the observer, the denominator is v + u_s

When a source of sound waves remains stationary, but the observer is moving relative to the source, the observed frequency can be calculated using the equation below:

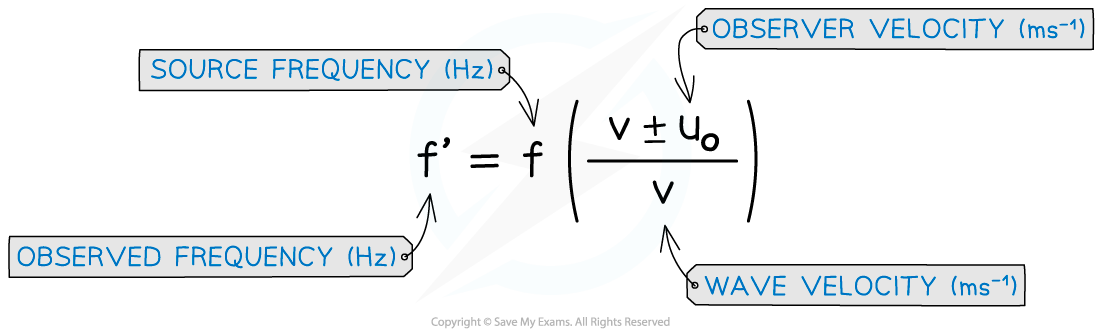
9-5-3-doppler-calculation-2-moving-observer-ib-hl

Doppler shift equation for a moving observer

The ± depends on whether the observer is moving towards or away from the source
- If the observer is moving towards the source, the numerator is v + u_o
- If the observer is moving away from the source, the numerator is v − u_o

These equations can also be written in terms of wavelength
- For example, the equation for a moving source is shown below:

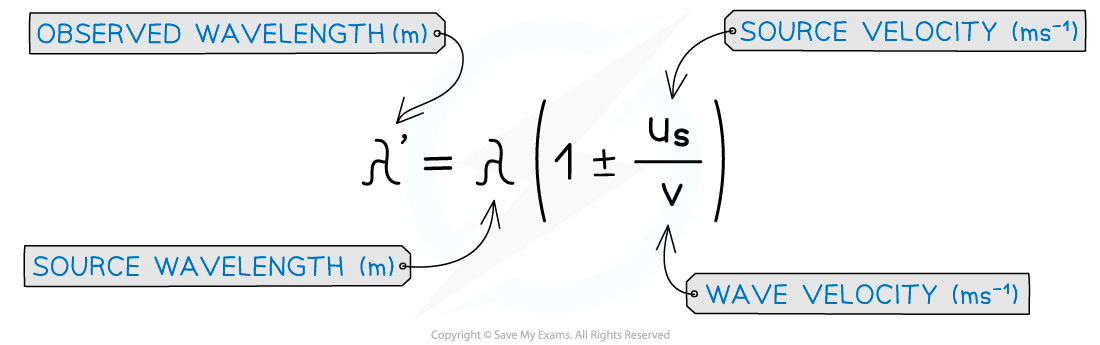
Doppler shift equation for a moving source in terms of wavelength

The ± depends on whether the source is moving towards or away from the observer
- If the source is moving towards, the term in the brackets is $1 - \frac{u_{S}}{v}$
- If the source is moving away, the term in the brackets is $1 + \frac{u_{S}}{v}$

Worked example

A police car siren emits a sound wave with a frequency of 450 Hz. The car is traveling away from an observer at speed of 45 m s^-1. The speed of sound is 340 m s^-1. Which of the following is the frequency the observer hears?

A. 519 Hz B. 483 Hz C. 397 Hz D. 358 Hz

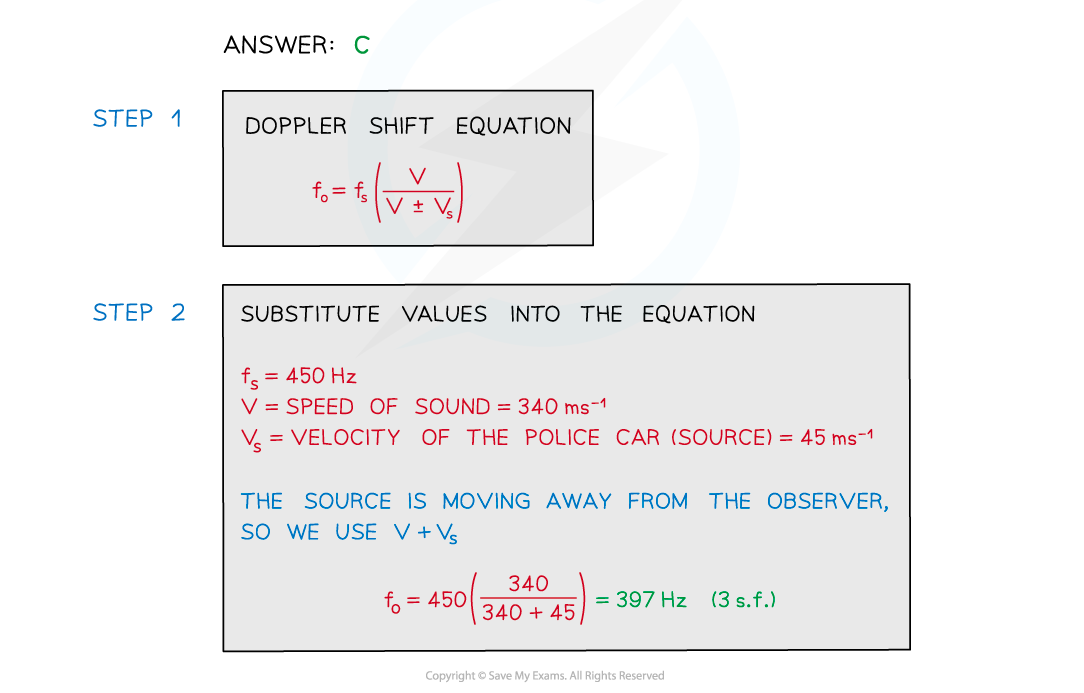
WE - Doppler shift equation answer image

Worked example

A bank robbery has occurred and the alarm is sounding at a frequency of 3 kHz. The thief jumps into a car which accelerates and reaches a constant speed. As he drives away at a constant speed, the frequency decreases to 2.85 kHz. The speed of sound is 340 m s^-1. Determine at what speed must he be driving away from the bank.

Step 1: List the known quantities

- Source frequency: $f$ = 3 kHz = 3000 Hz
- Observed frequency: $f^{'}$ = 2.85 kHz = 2850 Hz
- Speed of sound: $v$ = 340 m s^-1
- The observer is moving away from a stationary source of sound

Step 2: write the doppler shift equation

f^{'} = f (\frac{v - u_{o}}{v})

Step 3: rearrange to find the desired quantity

\frac{f^{'}}{f} = (\frac{v - u_{o}}{v})

\frac{f^{'}}{f} \times v = v - u_{o}

(\frac{f^{'}}{f} \times v) + u_{o} = v

u_{o} = v - (\frac{f^{'}}{f} \times v)

Step 4: substitute in values

u_{o} = v - (\frac{f^{'}}{f} \times v) = 340 - (\frac{2850}{3000} \times 340) = 340 - 323 = 17 m s^{- 1}

Step 5: State final answer

- The bank robber must be driving away at a constant speed of 17 ms^-1 based on the change in frequency heard

Calculating Doppler Shift of Light

Doppler shift can be calculated with relation to a light emitting source
- For example, a galaxy moving towards or away from Earth
Doppler shift for light is complicated, however if the speed of the observer or source is small (non-relativistic) compared to the speed of light, then this equation becomes simpler
The Doppler shift for a light-emitting non-relativistic source is described using the equation:

9-5-3-doppler-light-related-ib-hl

Doppler shift equation relating wavelength change for a moving source

Where:

Δf = change in frequency in Hertz (Hz)
f₀= reference frequency in Hertz (Hz)
λ = observed wavelength of the source in metres (m)
λ₀ = reference wavelength in metres (m)
Δλ = change in wavelength in metres (m)
v = velocity of a galaxy in metres per seconds (m/s)
c = the speed of light in metres per second (m/s)

This means that the change in wavelength, Δλ:

Δλ = λ – λ₀

This equation can be used to calculate the velocity of a galaxy if its wavelength can be measured and compared to a reference wavelength
Since the fractions have the same units on the numerator (top number) and denominator (bottom number), the Doppler shift has no units

Worked example

Light emitted from a star has a wavelength of 435 × 10^-9 m. A distance galaxy emits the same light but has a wavelength of 485 × 10^-9 m. Calculate the speed at which the galaxy is moving relative to Earth. The speed of light = 3 × 10⁸ m/s.

8.3.2 Doppler Shift Worked Example

The Doppler Equation (DP IB Physics: HL)

Revision Note

Calculating Doppler Shift

Calculating Doppler Shift of Light

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

1. Measurement & Uncertainties

1.1 Measurements in Physics

1.1.1 SI Units

1.1.2 Using Scientific Notation

1.1.3 Estimating Physical Quantities

1.2 Uncertainties & Errors

1.2.1 Random & Systematic Errors

1.2.2 Calculating Uncertainties

1.2.3 Determining Uncertainties from Graphs

1.3 Vectors & Scalars

1.3.1 Vector & Scalar Quantities

1.3.2 Combining & Resolving Vectors

1.3.3 Solving Vector Problems

2. Mechanics

2.1 Motion

2.1.1 Distance & Displacement

2.1.2 Speed & Velocity

2.1.3 Acceleration

2.1.4 Graphs Describing Motion

2.1.5 Uniform Acceleration

2.1.6 Acceleration of Free Fall Experiment

2.1.7 Projectile Motion

2.1.8 Terminal Speed

2.2 Forces

2.2.1 Free-Body Diagrams

2.2.2 Newton’s First Law

2.2.3 Newton’s Second Law

2.2.4 Newton’s Third Law

2.2.5 Applying Newton’s Laws of Motion

2.2.6 Friction

2.3 Work, Energy & Power

2.3.1 Kinetic Energy

2.3.2 Gravitational Potential Energy

2.3.3 Elastic Potential Energy

2.3.4 Work Done

2.3.5 Power

2.3.6 Principle of Conservation of Energy

2.3.7 Efficiency

2.4 Momentum & Impulse

2.4.1 Force & Momentum

2.4.2 Impulse

2.4.3 Conservation of Linear Momentum

2.4.4 Collisions & Explosions

3. Thermal Physics

3.1 Thermal Concepts

3.1.1 Solids, Liquids & Gases

3.1.2 Temperature

3.1.3 Internal Energy

3.1.4 Specific Heat Capacity

3.1.5 Specific Latent Heat

3.1.6 Phase Change

3.1.7 Investigating Thermal Energy

3.2 Modelling a Gas

3.2.1 Ideal Gas Laws

3.2.2 Ideal Gas Equation

3.2.3 Kinetic Model of an Ideal Gas

3.2.4 Mole Calculations

3.2.5 Investigating Gas Laws

4. Waves

4.1 Oscillations

4.1.1 Properties of Oscillations

4.1.2 Simple Harmonic Oscillations

4.1.3 SHM Graphs

4.1.4 Energy in SHM

4.2 Travelling Waves

4.2.1 Properties of Waves

4.2.2 Transverse & Longitudinal Waves

4.2.3 Electromagnetic Waves

4.2.4 Sound Waves

4.3 Wave Characteristics

4.3.1 Wavefronts

4.3.2 Amplitude & Intensity

4.3.3 Superposition