Ideal Gas Laws
Boyle's Law
- Boyle's Law states:
For a fixed mass of gas at a constant temperature, the pressure p is inversely proportional to the volume V
- This can be expressed in equation form as:
![]()
- Plotting the pressure against the volume for a gas at a constant temperature on a graph (i.e. p-V graph) gives the so-called isothermal curve for the gas
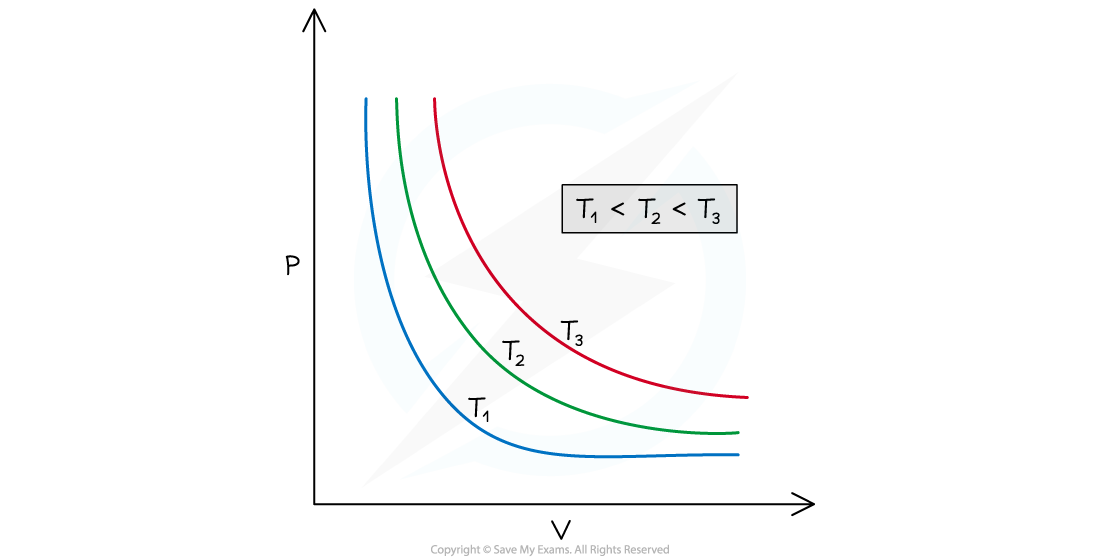
Graph of pressure against volume for a fixed mass of gas at three different temperatures, with T1 < T2 < T3. The curves are called isotherms (i.e. the temperature along each curve is constant)
- Plotting the pressure against the reciprocal of the volume (i.e. 1/V) for a gas at constant temperature still gives an isothermal, but this time, the line is straight

Graph of pressure against reciprocal of the volume for a fixed mass of gas at three different temperatures, with T1 < T2 < T3. In this case, the isotherms are straight lines
- Boyle's law can be rewritten as follows:
pV = constant
- Which means that:
p1V1 = p2V2
- Where:
- p1 = initial pressure in pascals (Pa) or atmospheres (atm)
- V1 = initial volume in metres cubed (m3) or litres (L)
- p2 = the final pressure in pascals (Pa) or atmospheres (atm)
- V2 = the final volume in metres cubed (m3) or litres (L)
Charles's Law
- Charle's Law states:
For a fixed mass of gas at constant pressure, the volume V is directly proportional to the absolute temperature T
- This can be expressed in equation form as:
![]()
- The direct proportionality relationship is only valid if the gas temperature is measured in kelvin (K)
- Plotting the volume against the temperature for a gas at constant pressure gives a straight line along which the gas pressure does not change

Graph of volume against temperature for a fixed mass of gas at three different pressures, with p1 < p2 < p3
- Charles's law can be rewritten as follows:
![]()
- Which means that:
![]()
- Where:
- V1 = initial volume in metres cubed (m3) or litres (L)
- T1 = initial temperature in kelvin (K)
- V2 = final volume in metres cubed (m3) or litres (L)
- T2 = final temperature in kelvin (K)
Gay Lussac's Law
- Gay Lussac's Law states:
For a fixed mass of gas at constant volume, the pressure p is directly proportional to the absolute temperature T
- This can be expressed in equation form as:
![]()
- The direct proportionality relationship is only valid if the gas temperature is measured in kelvin (K)
- Plotting the pressure against the temperature for a gas at constant volume gives a straight line along which the gas volume is the same

Graph of pressure against temperature for a fixed mass of gas at three different volumes, with V1 < V2 < V3
- Gay Lussac's law can be rewritten as follows:
![]()
- Which means that:
![]()
- Where:
- p1 = initial pressure in pascals (Pa) or atmospheres (atm)
- T1 = initial temperature in kelvin (K)
- p2 = final pressure in pascals (Pa) or atmospheres (atm)
- T2 = final temperature in kelvin (K)
Gas Laws Combined
- The three gas laws can be combined into one
- For a fixed mass of gas, the following holds:
![]()
- Which means that:
![]()
- Where:
- p1 = initial pressure in pascals (Pa) or atmospheres (atm)
- V1 = initial volume in metres cubed (m3) or litres (L)
- T1 = initial temperature in kelvin (K)
- p2 = final pressure in pascals (Pa) or atmospheres (atm)
- V2 = final volume in metres cubed (m3) or litres (L)
- T2 = final temperature in kelvin (K)
Worked example
An ideal gas occupies a volume equal to 5.0 × 10–4 m3. Its pressure is 2.0 × 106 Pa and its temperature is 40°C. The gas is then heated and reaches a temperature of 80°C. It also expands to a new volume of 6.0 × 10–4 m3.Determine the new pressure of the gas.
Step 1: Write down the given quantities
- Initial volume, V1 = 5.0 × 10–4 m3
- Initial pressure, p1 = 2.0 × 106 Pa
- Initial temperature, T1 = 40°C = 313 K
- Final volume, V2 = 6.0 × 10–4 m3
- Final temperature, T2 = 80°C = 353 K
Remember to:
- Use the appropriate subscripts for initial (i.e. 1) and final (i.e. 2) values
- Convert the temperature from degrees Celsius into Kelvin (K)
Step 2: Write down the equation for the three gas laws combined
![]()
Step 3: Rearrange the equation to calculate the unknown final pressure p2
![]()
Step 4: Substitute numbers into the equation

p2 = 1.9 × 106 Pa
Examiner Tip
When dealing with gas laws problems, always remember to convert temperatures from degrees Celsius (°C) to kelvin (K).After you solve a problem using any of the gas laws (or all of them combined), always check whether your final result makes physically sense - e.g. if you are asked to calculate the final pressure of a fixed mass of gas being heated at constant volume, your result must be greater than the initial pressure given in the problem (since Gay- Lussac's law states that pressure and absolute temperature are directly proportional at constant volume).

