Collisions & Explosions in One-Dimension (DP IB Physics) : Revision Note
Collisions & Explosions in One-Dimension
In both collisions and explosions, momentum is always conserved
However, kinetic energy might not always be
Elastic and inelastic collisions
Collisions are when two or more moving objects come together and exert a force on one another for a relatively short time
Explosions are when two or more objects that are initially at rest are propelled apart from one another
Collisions and explosions are either:
Elastic – if the kinetic energy is conserved
Inelastic – if the kinetic energy is not conserved
A perfectly elastic collision is an idealised situation that does not actually occur everyday life
Perfectly elastic collisions do occur commonly between particles
All collisions occurring on a macroscopic level are inelastic collisions
However, exam questions can use the theoretical idea of an elastic collision on a macroscopic level
A totally inelastic collision is a special case of an inelastic collision where the colliding bodies stick together and move as one body
In a totally inelastic collision, the maximum amount of kinetic energy is transferred away from the moving bodies and is dissipated to the surroundings
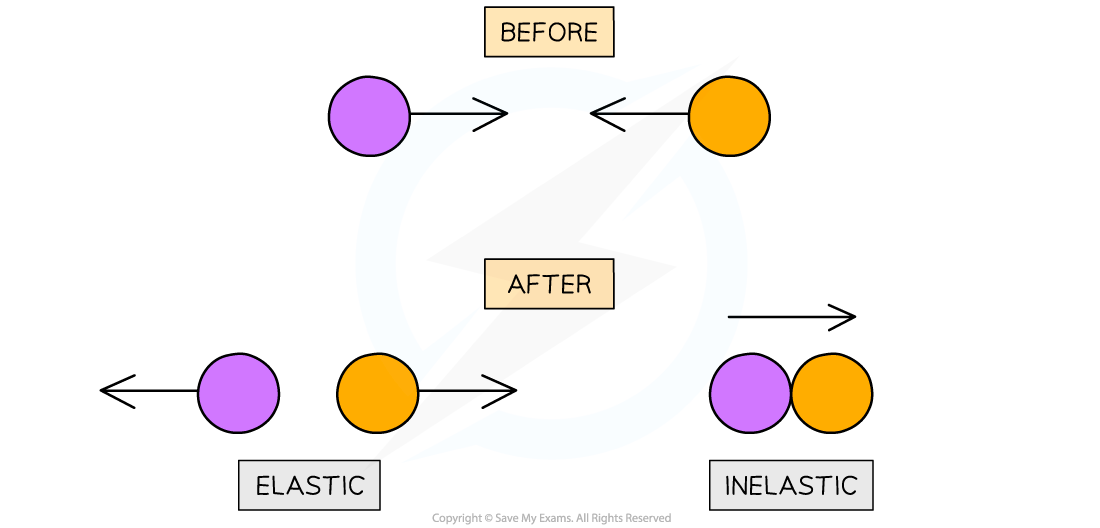
Elastic collisions are where two objects move in opposite directions. Inelastic collisions are where two objects stick together
An explosion is commonly to do with recoil
For example, a gun recoiling after shooting a bullet or an unstable nucleus emitting an alpha particle and a daughter nucleus
To find out whether a collision is elastic or inelastic, compare the kinetic energy before and after the collision
The equation for kinetic energy is:
Where:
Ek = kinetic energy (J)
m = mass (kg)
v = velocity (m s–1)
Examiner Tips and Tricks
It can be helpful to think about collisions and explosions as if there are four types rather than two:
elastic - kinetic energy conserved
perfectly elastic - kinetic energy conserved and no energy transferred between objects
inelastic - kinetic energy not conserved
totally inelastic - kinetic energy not conserved and maximum energy transferred to surroundings
Worked Example
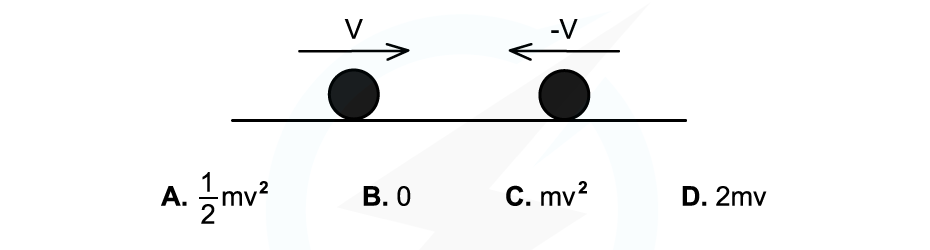
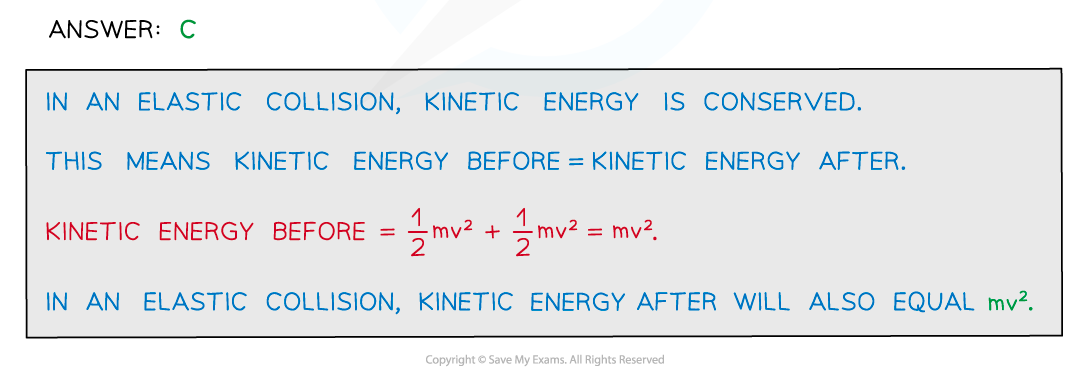
Two similar spheres, each of mass m and velocity v are travelling towards each other. The spheres have a head-on elastic collision.
What is the total kinetic energy after the impact?
Worked Example
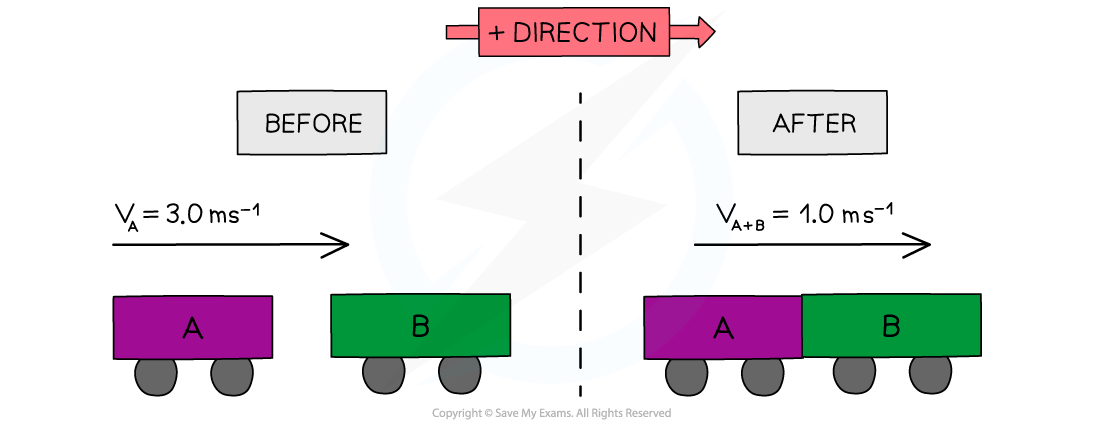
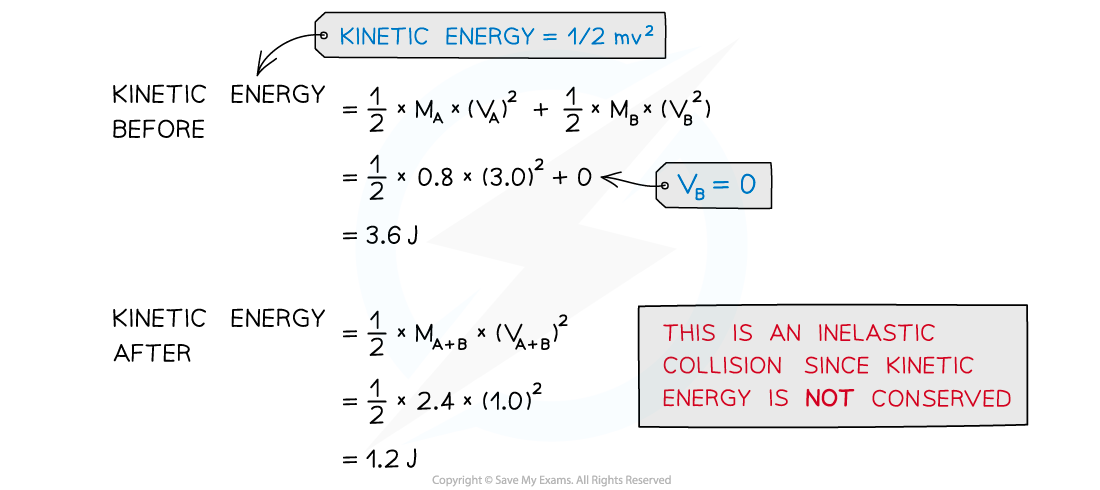
Trolley A of mass 0.80 kg collides head-on with stationary trolley B at speed 3.0 m s–1. Trolley B has twice the mass of trolley A. The trolleys stick together and travel at a velocity of 1.0 m s–1.
Determine whether this is an elastic or inelastic collision.
Answer:
Examiner Tips and Tricks
If an object is stationary or at rest, its initial velocity is 0, therefore, the momentum and kinetic energy are also equal to 0.
When a collision occurs in which two objects are stuck together, treat the final object as a single object with a mass equal to the sum of the masses of the two individual objects.
Despite velocity being a vector, kinetic energy is a scalar quantity and therefore will never include a minus sign - this is because in the kinetic energy formula, mass is scalar and the v2 will always give a positive value whether it's a negative or positive velocity.

You've read 0 of your 5 free revision notes this week
Unlock more, it's free!
Did this page help you?
