Orbital Motion, Speed & Energy (DP IB Physics): Revision Note
Orbital Motion, Speed & Energy
Since most planets and satellites have near-circular orbits, the gravitational force FG between two bodies (e.g. planet & star, planet & satellite) provides the centripetal force needed to stay in an orbit
Both the gravitational force and centripetal force are perpendicular to the direction of travel of the planet
Consider a satellite with mass m orbiting Earth with mass M at a distance r from the centre travelling with linear speed v
Equating the gravitational force to the centripetal force for a planet or satellite in orbit gives:
The mass of the satellite m will cancel out on both sides to give:
Where:
= orbital speed of the smaller mass (m s−1)
G = Newton's Gravitational Constant
M = mass of the larger mass being orbited (kg)
r = orbital radius (m)
This means that all satellites, whatever their mass, will travel at the same speed v in a particular orbit radius r
Since the direction of a planet orbiting in circular motion is constantly changing, the centripetal acceleration acts towards the planet
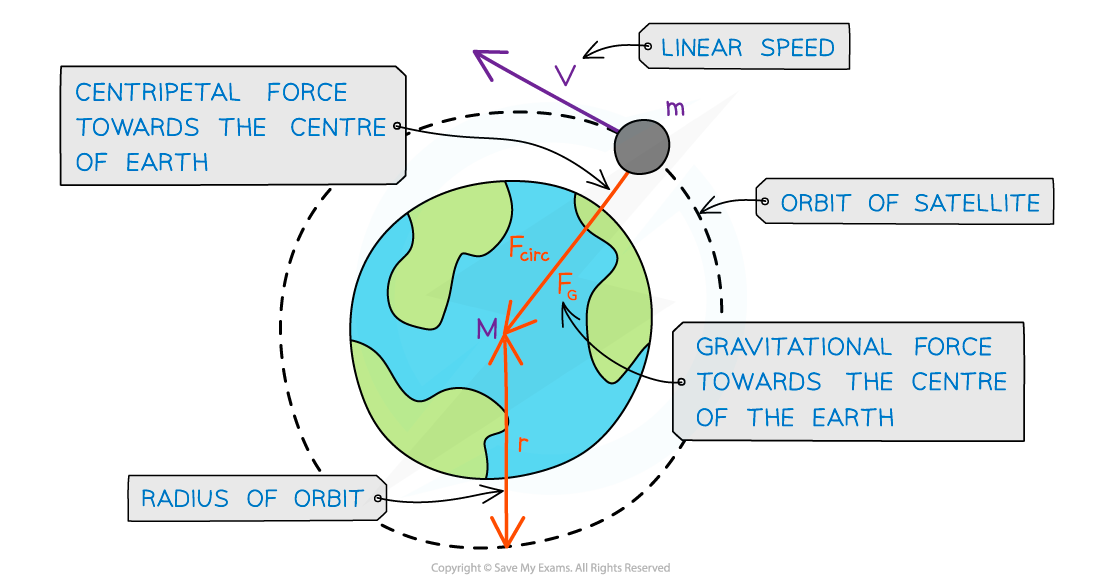
A satellite in orbit around the Earth travels in circular motion
Energy of an Orbiting Satellite
An orbiting satellite follows a circular path around a planet
Just like an object moving in circular motion, it has both kinetic energy (Ek) and gravitational potential energy (Ep) and its total energy is always constant
An orbiting satellite's total energy is calculated by:
Total energy = Kinetic energy + Gravitational potential energy
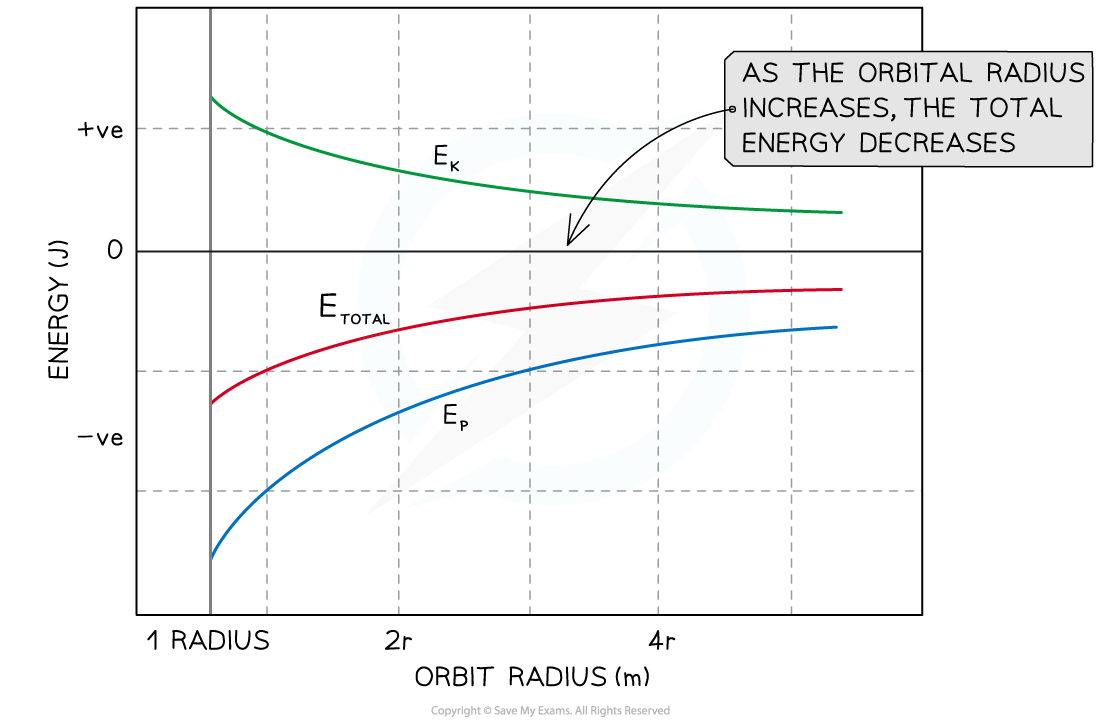
A graph showing the kinetic, potential and total energy for a mass at varying orbital distances from a massive body
This means that the satellite's Ek and Ep are also both constant in a particular orbit
If the orbital radius of a satellite decreases its Ek increases and its Ep decreases
If the orbital radius of a satellite increases its Ekdecreases and its Epincreases
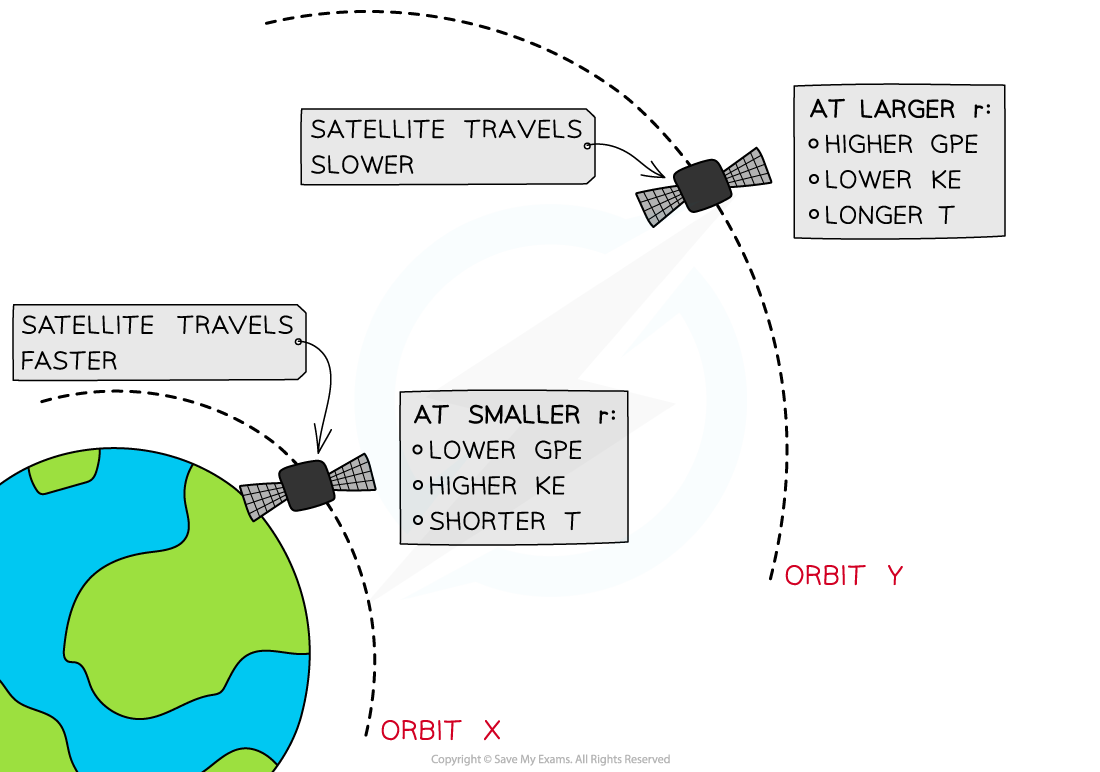
At orbit Y, the satellite has greater GPE and less KE than at at orbit X
A satellite is placed in two orbits, X and Y, around Earth
At orbit X, where the radius of orbit r is smaller, the satellite has a:
Larger gravitational force on it
Higher speed
Higher Ek
Lower Ep
Shorter orbital time period, T
At orbit Y, where the radius of orbit r is larger, the satellite has a:
Smaller gravitational force on it
Smaller speed
Lower Ek
Higher Ep
Longer orbital time period, T
Worked Example
A binary star system constant of two stars orbiting about a fixed point B.
The star of mass M1 has a circular orbit of radius R1 and mass M2 has a radius of R2. Both have an angular speed ⍵ about B.
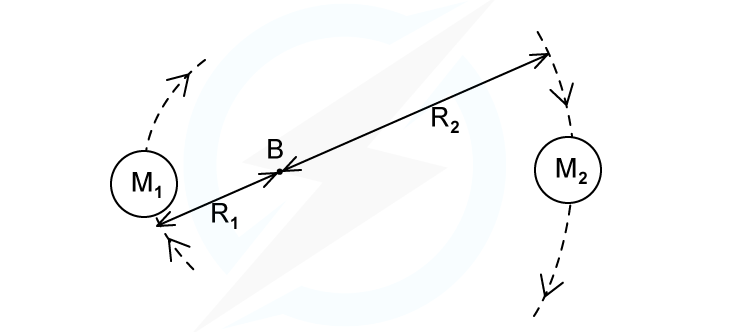
In terms of G, M2, R1 and R2, write an expression for
(a) the angular speed ⍵ of mass M1
(b) the time period T of each star
Answer:
(a) Angular speed:
The centripetal force on mass M1 is:
The gravitational force between the two masses is:
Equating these expressions gives:
Rearrange for angular velocity
(b) Orbital period:
The relation between angular speed and orbital period is
Using the expression for angular velocity from part (a)
Worked Example
Two identical satellites, X and Y, orbit a planet at radii R and 3R respectively.
Which one of the following statements is incorrect?
A. Satellite X has more kinetic energy and less potential energy than satellite Y
B. Satellite X has a shorter orbital period and travels faster than satellite Y
C. Satellite Y has less kinetic energy and more potential energy than satellite X
D. Satellite Y has a longer orbital period and travels faster than satellite X
Answer: D
Satellite Y is at a larger orbital radius, therefore it will have a longer orbital period, since
Being at a larger orbital radius means the gravitational force will be weaker for Y than for X
So, satellite Y will travel much slower than X as centripetal force:
Travelling at a slower speed means satellite Y will have less kinetic energy, as
, and, therefore, more potential energy than X
| Satellite X | Satellite Y |
orbital radius orbital period orbital speed kinetic energy potential energy | smaller shorter faster greater lower | larger longer slower lower greater |
Therefore, all statements are correct except in D where it says 'Satellite Y travels faster than satellite X'
Examiner Tips and Tricks
If you can't remember which way around the kinetic and potential energy increases and decreases, think about the velocity of a satellite at different orbits.
When it is orbiting close to a planet, it experiences a larger gravitational pull and therefore orbits faster. Since the kinetic energy is proportional to v2, it, therefore, has higher kinetic energy closer to the planet. To keep the total energy constant, the potential energy must decrease too.

Unlock more, it's free!
Did this page help you?