Gravitational Potential Gradient (DP IB Physics): Revision Note
Gravitational Potential Gradient
A gravitational field can be defined in terms of the variation of gravitational potential at different points in the field:
The gravitational field at a particular point is equal to the negative gradient of a potential-distance graph at that point
The potential gradient is defined by the equipotential lines
These demonstrate the gravitational potential in a gravitational field and are always drawn perpendicular to the field lines
The potential gradient in a gravitational field is defined as:
The rate of change of gravitational potential with respect to displacement in the direction of the field
Gravitational field strength, g and the gravitational potential, V can be graphically represented against the distance from the centre of a planet, r
Where:
g = gravitational field strength (N kg-1)
ΔVg = change in gravitational potential (J kg-1)
Δr = distance from the centre of a point mass (m)
The graph of Vg against r for a planet is:
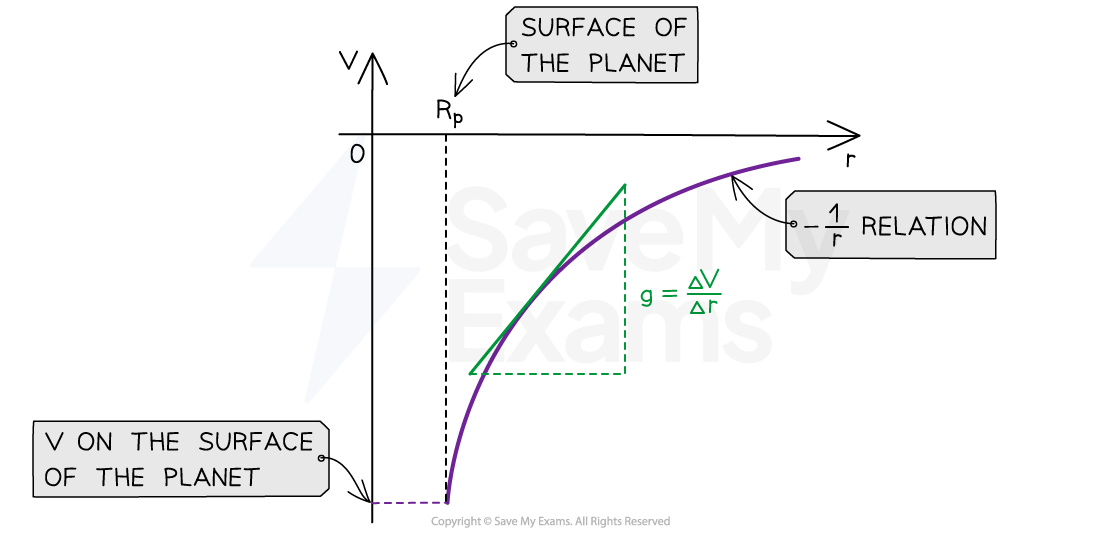
The gravitational potential and distance graphs follow a -1/r relation
The key features of this graph are:
The values for Vg are all negative (because the graph is drawn below the horizontal r axis)
As r increases, Vg against r follows a
relation
The gradient of the graph at any particular point is the value of g at that point,
The graph has a shallow increase as r increases
To calculate g, draw a tangent to the graph at that point and calculate the gradient of the tangent
This is a graphical representation of the gravitational potential equation:
where G and M are constant
Worked Example
Determine the change in gravitational potential when travelling from 3 Earth radii (from Earth’s centre) to the surface of the Earth.
Take the mass of the Earth to be 5.97 × 1024 kg and the radius of the Earth to be 6.38 × 106 m.
Answer:
Step 1: List the known quantities
Mass of the Earth, ME = 5.97 × 1024 kg
Radius of the Earth, rE = 6.38 × 106 m
Initial distance, r1 = 3rE = 3 × (6.38 × 106) m = 1.914 × 107 m
Final distance, r2 = rE = 6.38 × 106 m
Gravitational constant, G = 6.67 × 10−11 m3 kg−1 s−2
Step 2: Write down the equation for potential difference
Step 3: Substitute the values into the equation
ΔVg = −4.16 × 107 J kg−1

Unlock more, it's free!
Did this page help you?