Combining & Resolving Vectors (DP IB Physics): Revision Note
Combining & Resolving Vectors
Vectors can be changed in a variety of ways, such as
Combining through vector addition or subtraction
Combining through vector multiplication
Resolving into components through trigonometry
Combining Vectors
Vectors can be combined by adding or subtracting them to produce the resultant vector
The resultant vector is sometimes known as the ‘net’ vector (e.g. the net force)
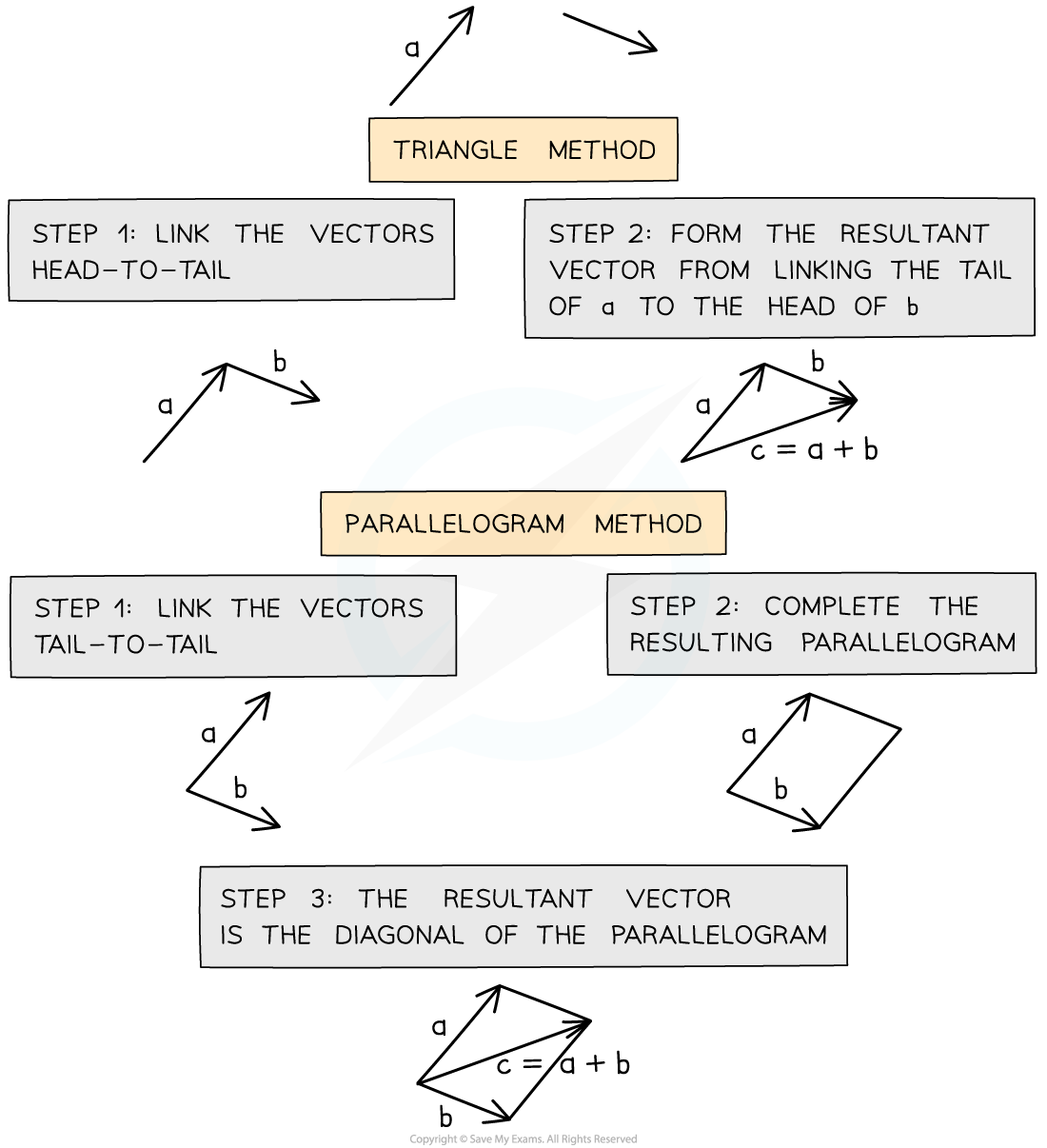
There are two methods that can be used to combine vectors: the triangle method and the parallelogram method
Triangle method
To combine vectors using the triangle method:
Step 1: link the vectors head-to-tail
Step 2: the resultant vector is formed by connecting the tail of the first vector to the head of the second vector
Parallelogram method
To combine vectors using the parallelogram method:
Step 1: link the vectors tail-to-tail
Step 2: complete the resulting parallelogram
Step 3: the resultant vector is the diagonal of the parallelogram
Worked Example
Draw the vector c = a + b.
Answer:
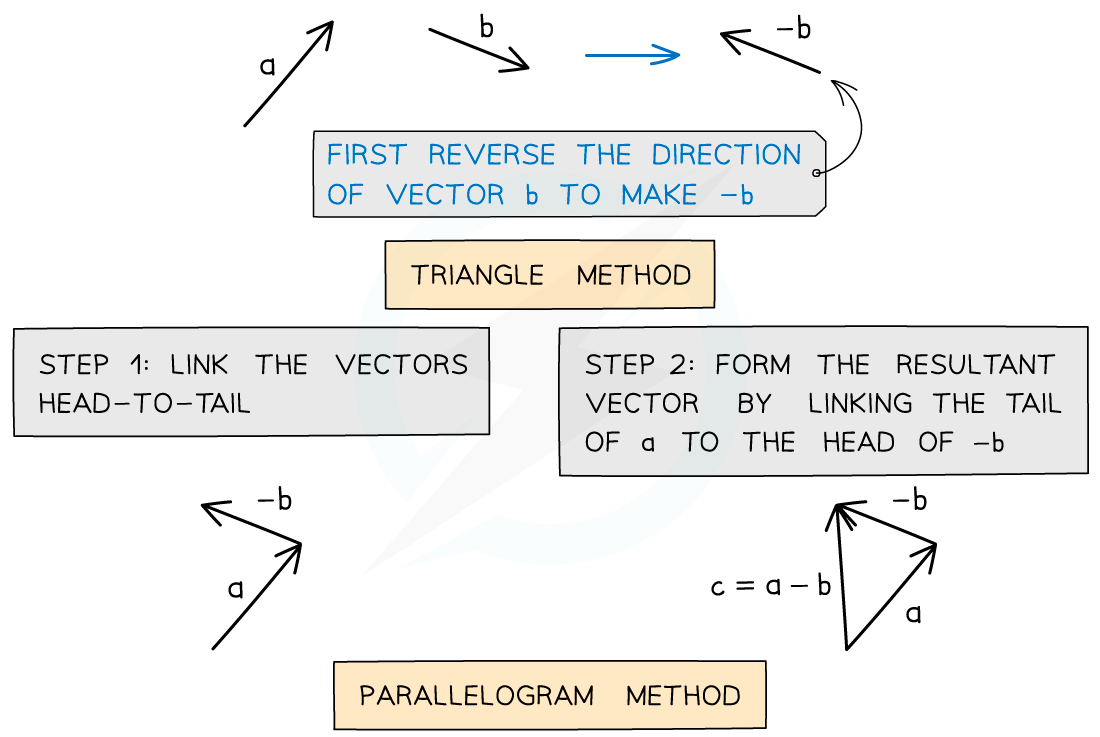
Worked Example
Draw the vector c = a – b.
Answer:
Vector Multiplication
The product of a scalar and a vector is always a vector
For example, consider the scalar quantity mass
and the vector quantity acceleration
The product of mass
and acceleration
gives rise to a vector quantity force
For another example, consider the scalar quantity mass
and the vector quantity velocity
The product of mass
and velocity
gives rise to a vector quantity momentum
Resolving Vectors
Two vectors can be represented by a single resultant vector
Resolving a vector is the opposite of adding vectors
A single resultant vector can be resolved
This means it can be represented by two vectors, which in combination have the same effect as the original one
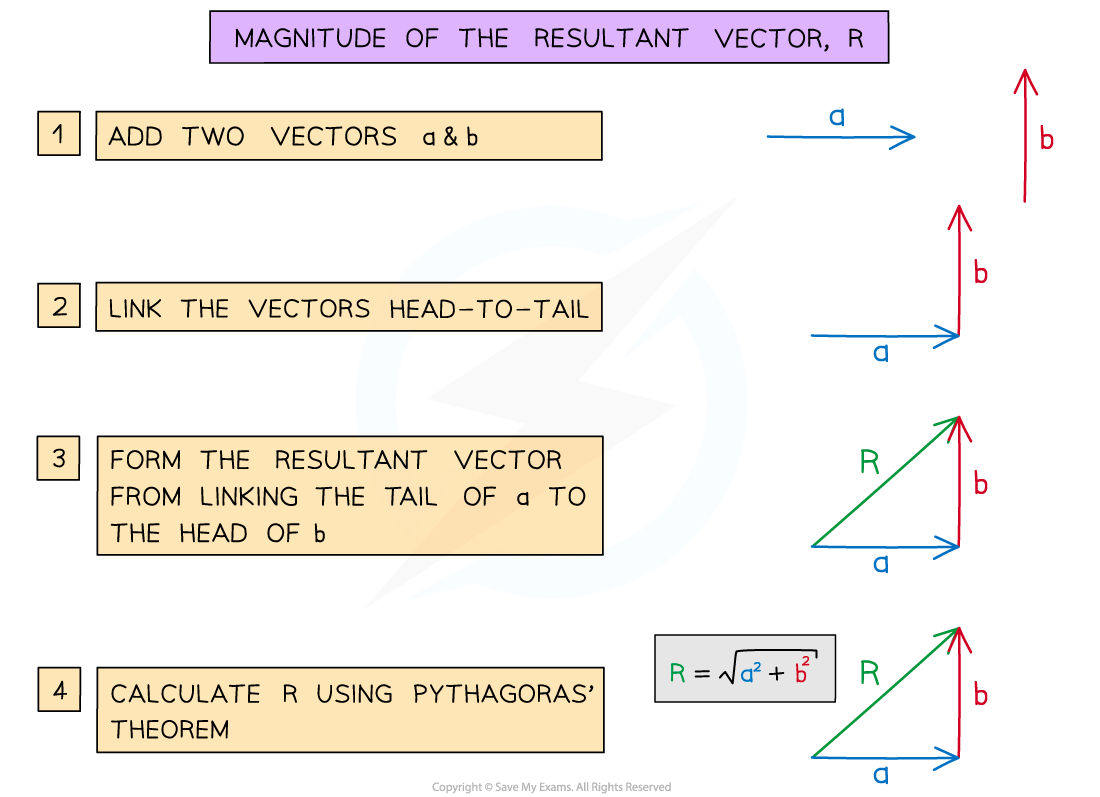
The magnitude of the resultant vector is found by using Pythagoras’ Theorem
When a single resultant vector is broken down into its parts, those parts are called components
For example, a force vector of magnitude FR and an angle of θ to the horizontal is shown below

Resolving two force vectors F1 and F2 into a resultant force vector FR
It is possible to resolve this vector into its horizontal and vertical components using trigonometry
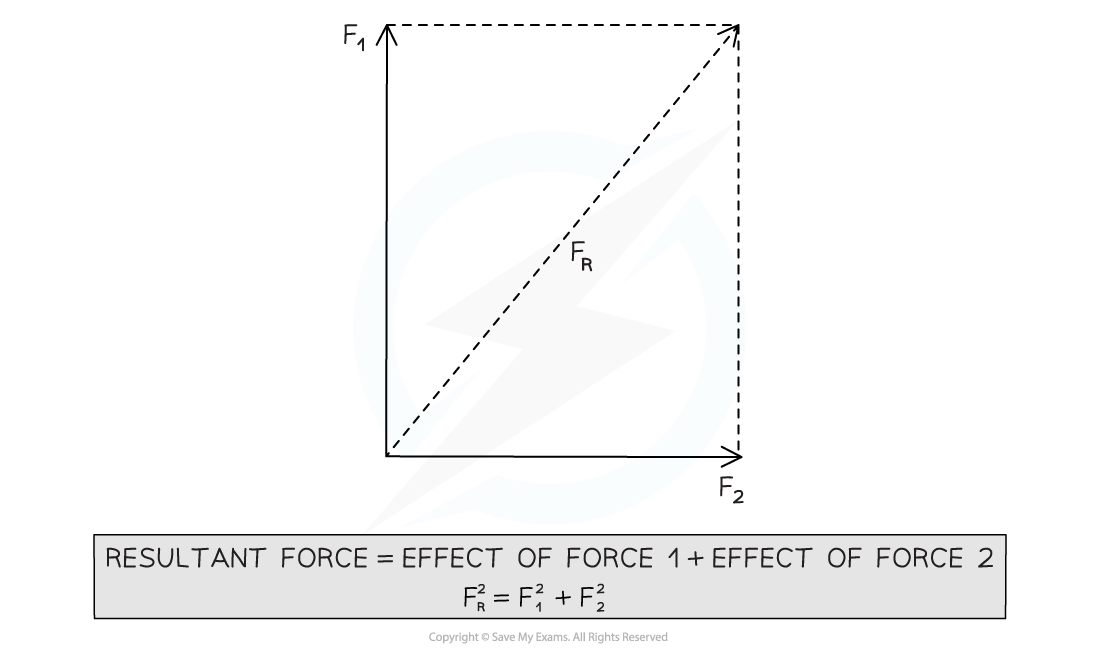
The resultant force FR can be split into its horizontal and vertical components
The direction of the resultant vector is found from the angle it makes with the horizontal or vertical
The question should imply which angle it is referring to (i.e. calculate the angle from the x-axis)
Calculating the angle of this resultant vector from the horizontal or vertical can be done using trigonometry
Either the sine, cosine or tangent formula can be used depending on which vector magnitudes are calculated
For the horizontal component, Fx = F cos θ
For the vertical component, Fy = F sin θ
Worked Example
A hiker walks a distance of 6 km due east and 10 km due north.
Calculate the magnitude of their displacement and its direction from the horizontal.
Answer:
Step 1: Draw a vector diagram
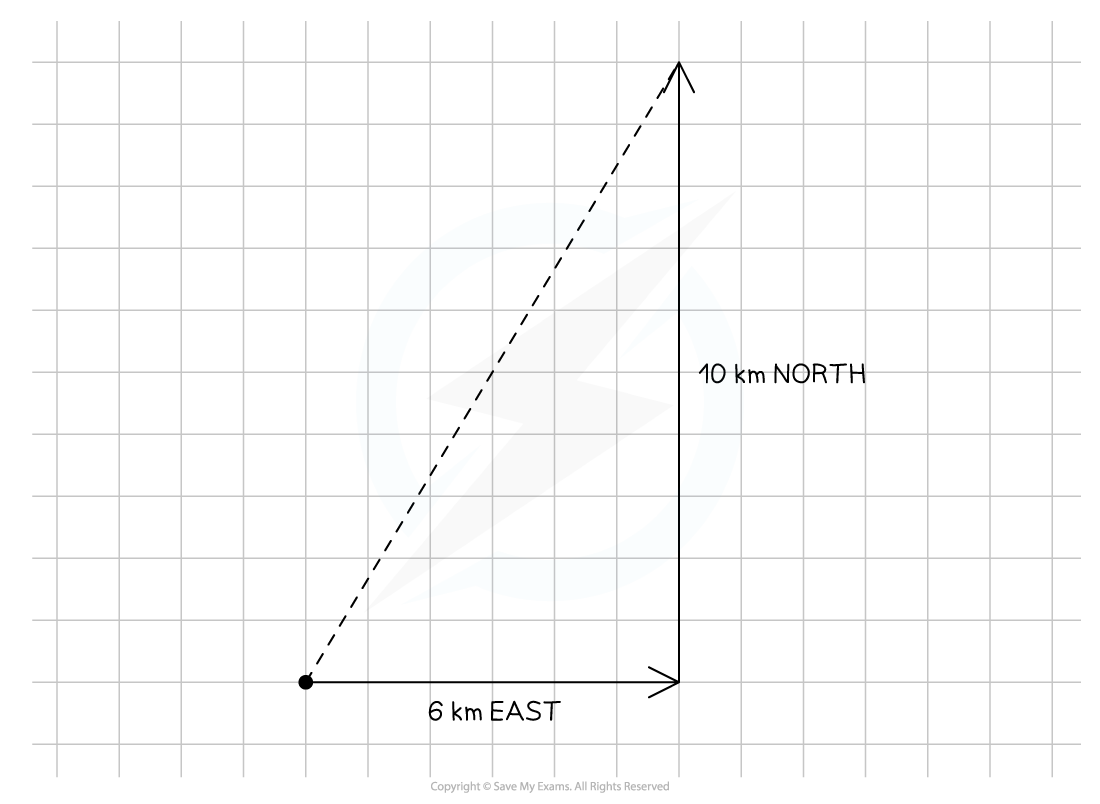
Step 2: Calculate the magnitude of the resultant vector using Pythagoras' Theorem
Resultant vector = 11.66
Step 3: Calculate the direction of the resultant vector using trigonometry
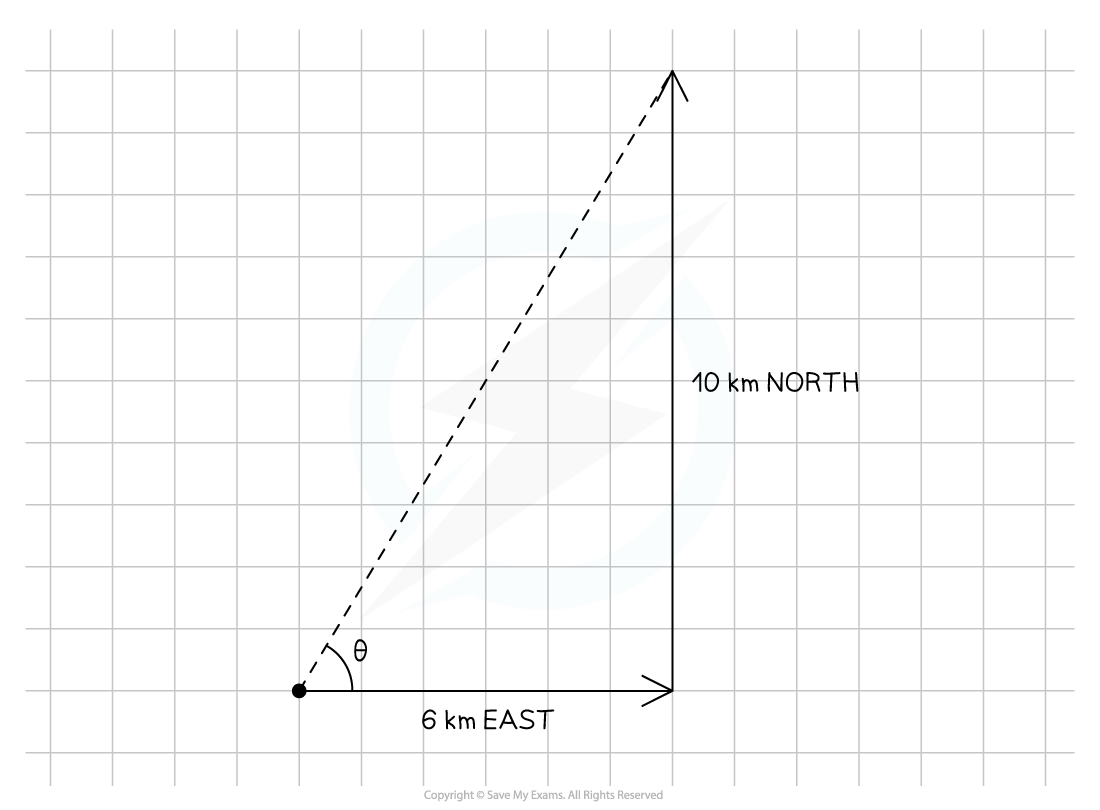
Step 4: State the final answer complete with direction
Vector magnitude: 12 km
Direction: 59° east and upwards from the horizontal
Examiner Tips and Tricks
Make sure you are confident using trigonometry as it is used a lot in vector calculations!
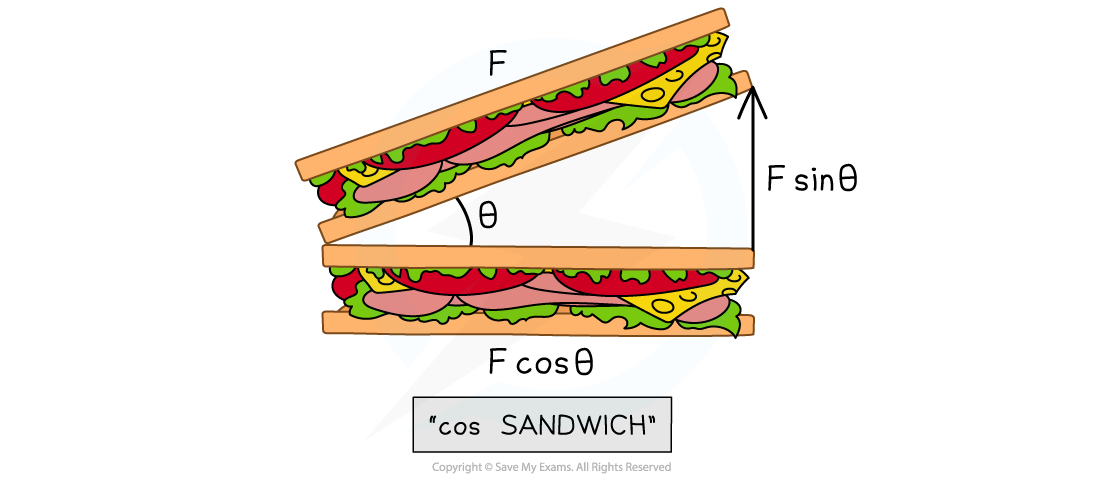
If you're unsure as to which component of the force is cos θ or sin θ, just remember that the cos θ is always the adjacent side of the right-angled triangle AKA, making a 'cos sandwich'
Force as a Vector
In physics, vectors appear in many different topic areas
Specifically, vectors are often combined and resolved to solve problems when considering motion, forces, and momentum
Forces vector diagrams are often represented by free-body force diagrams
The rules for drawing a free-body diagram are the following:
Rule 1: Draw a point in the centre of mass of the body
Rule 2: Draw the body free from contact with any other object
Rule 3: Draw the forces acting on that body using vectors with length in proportion to its magnitude
Rule 4: Draw the tail of the vector from the centre of mass and use the tip to indicate the direction
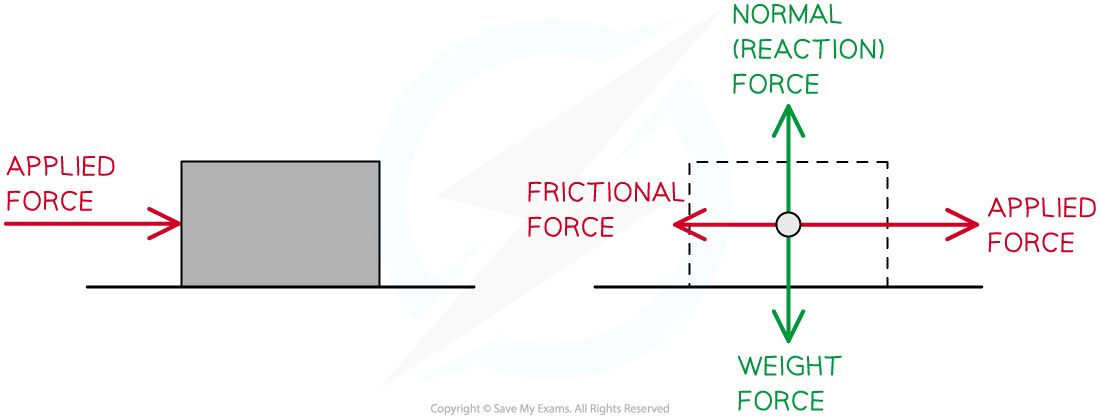
Point particle representation of the forces acting on a moving object on a rough horizontal surface
The below example shows the forces acting on an object suspended from a stationary rope
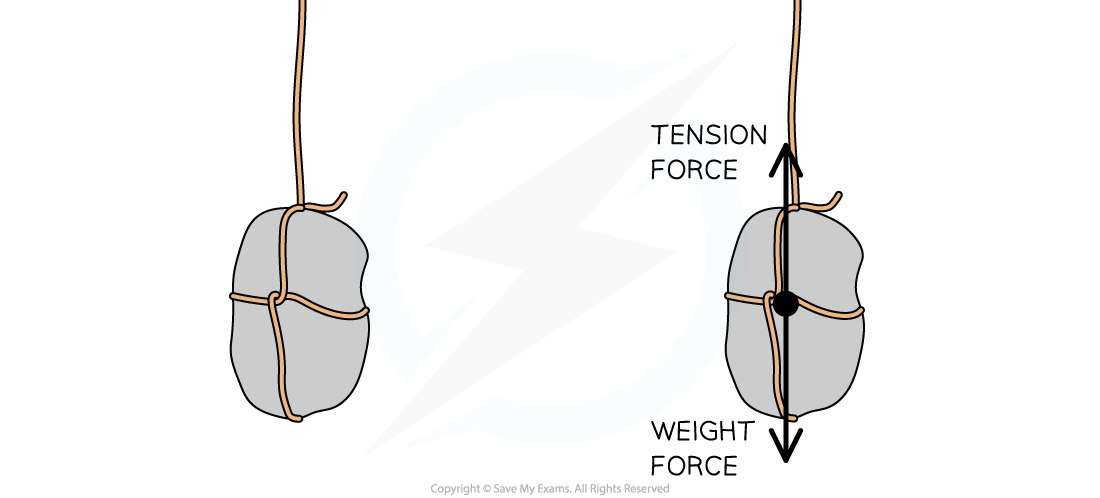
Free-body diagram of an object suspended from a stationary rope
Forces on an Inclined Plane
A common scenario is an object on an inclined plane
An inclined plane, or a slope, is a flat surface tilted at an angle, θ
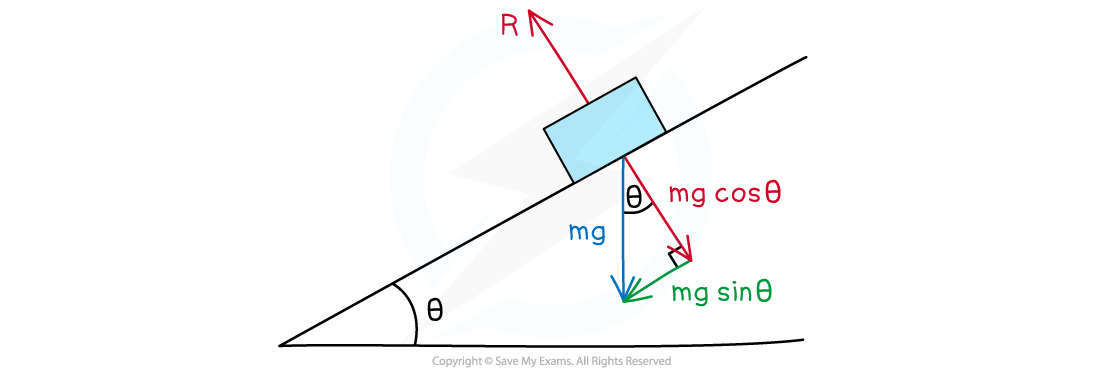
The weight vector of an object on an inclined plane can be split into its components parallel and perpendicular to the slope
Inclined slope problems can be simplified by considering the components of the forces as parallel or perpendicular to the slope
The weight (W = mg) of the object is always directed vertically downwards
On the inclined slope, weight can be split into the following components:
Perpendicular to the slope:
Parallel to the slope:
The normal (or reaction) force R is always vertically upwards, or perpendicular to the surface
If there is no friction, the parallel component of weight, mg sin θ, causes the object to move down the slope
If the object is not moving perpendicular to the slope, the normal force is R = mg cos θ
Equilibrium
Coplanar forces can be represented by vector triangles
Forces are in equilibrium if an object is either
At rest
Moving at constant velocity
In equilibrium, coplanar forces are represented by closed vector triangles
The vectors, when joined together, form a closed path
The most common forces on objects are
Weight
Normal reaction force
Tension (from cords and strings)
Friction
The forces on a body in equilibrium are demonstrated below:
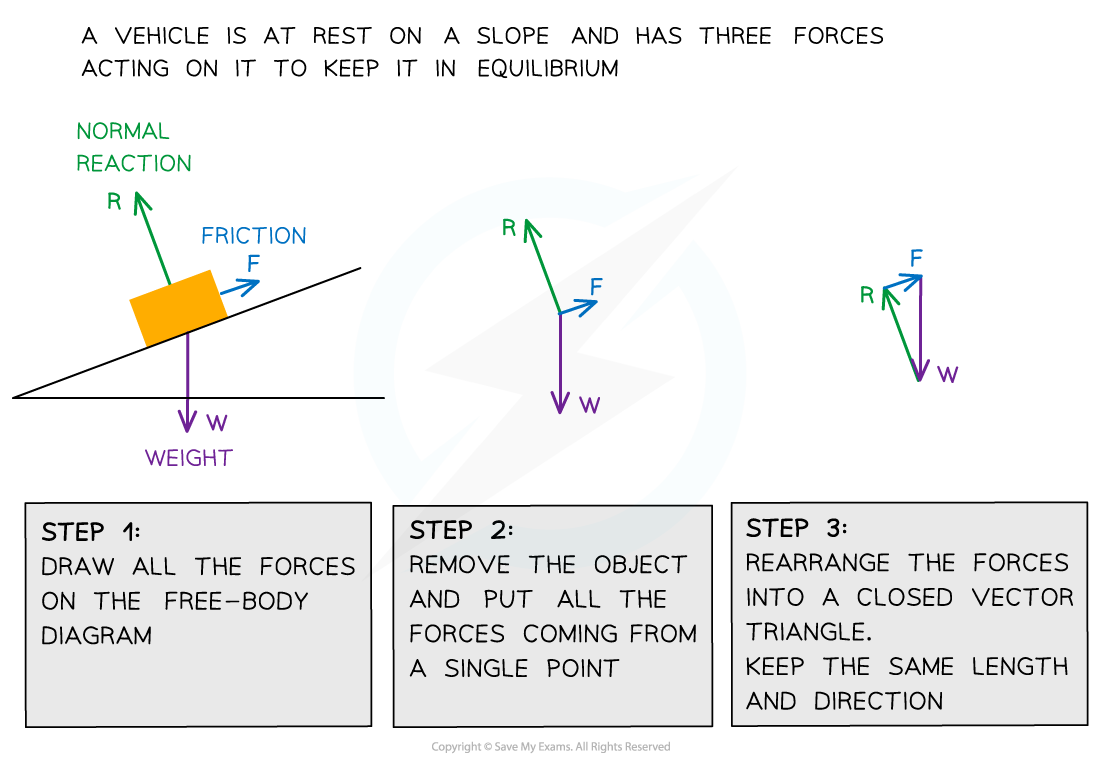
Three forces on an object in equilibrium form a closed vector triangle
Worked Example
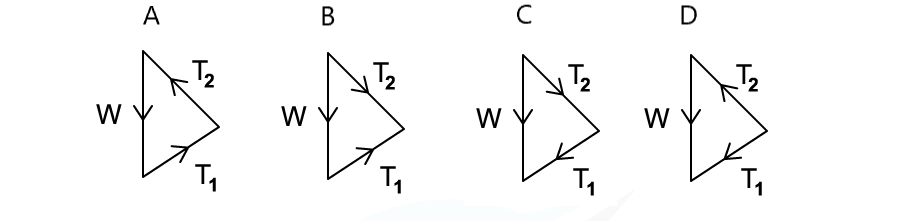
A weight hangs in equilibrium from a cable at point X. The tensions in the cables are T1 and T2 as shown.

Which diagram correctly represents the forces acting at point X?

Worked Example
A helicopter provides a lift of 250 kN when the blades are tilted at 15º from the vertical.

Calculate the horizontal and vertical components of the lift force.
Answer:
Step 1: Draw a vector triangle of the resolved forces
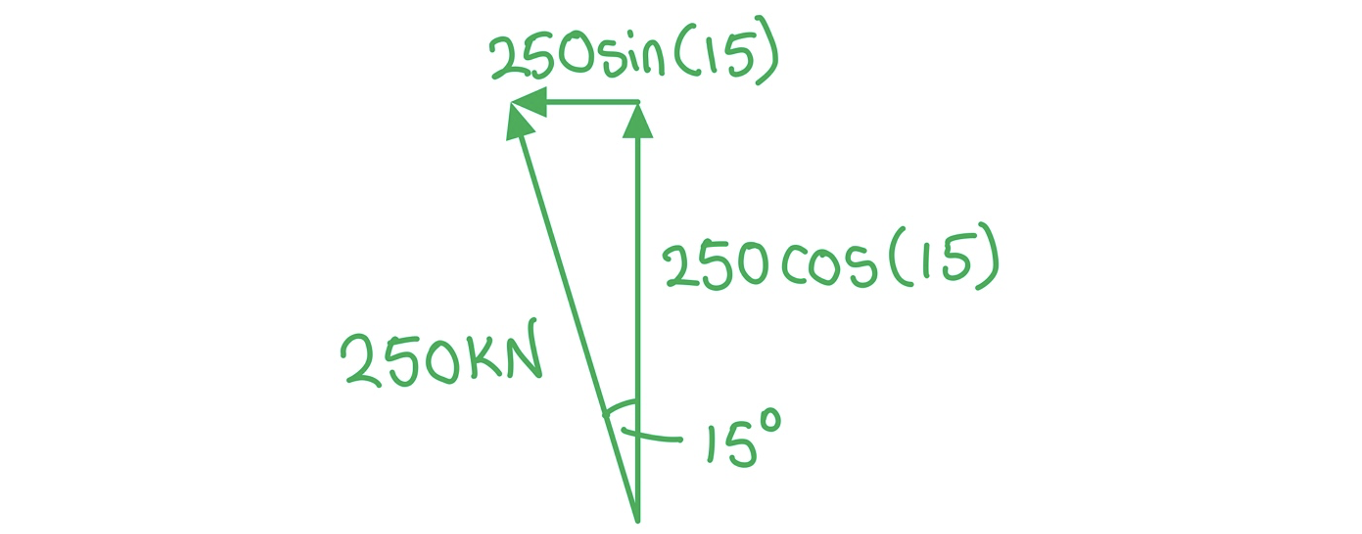
Step 2: Calculate the vertical component of the lift force
Vertical component of force = 250 × cos(15) = 242 kN
Step 3: Calculate the horizontal component of the lift force
Horizontal component of force = 250 × sin(15) = 64.7 kN
Examiner Tips and Tricks
When labelling force vectors, it is important to use conventional and appropriate naming or symbols such as:
w or weight force or mg
N or R for normal reaction force (depending on your local context either of these could be acceptable)
Using unexpected notation can lead to losing marks so try to be consistent with expected conventions.

Unlock more, it's free!
Did this page help you?