Superposition of Waves (DP IB Physics): Revision Note
Superposition of Waves
When two or more waves arrive at the same point and overlap, their amplitudes combine
This is called superposition
The principle of superposition states that:
When two or more waves overlap at a point, the displacement at that point is equal to the sum of the displacements of the individual waves
The superposition of surface water waves shows the effect of this overlap
There are areas of zero displacement, where the water is flat
There are areas of increased displacement, where the water waves are higher
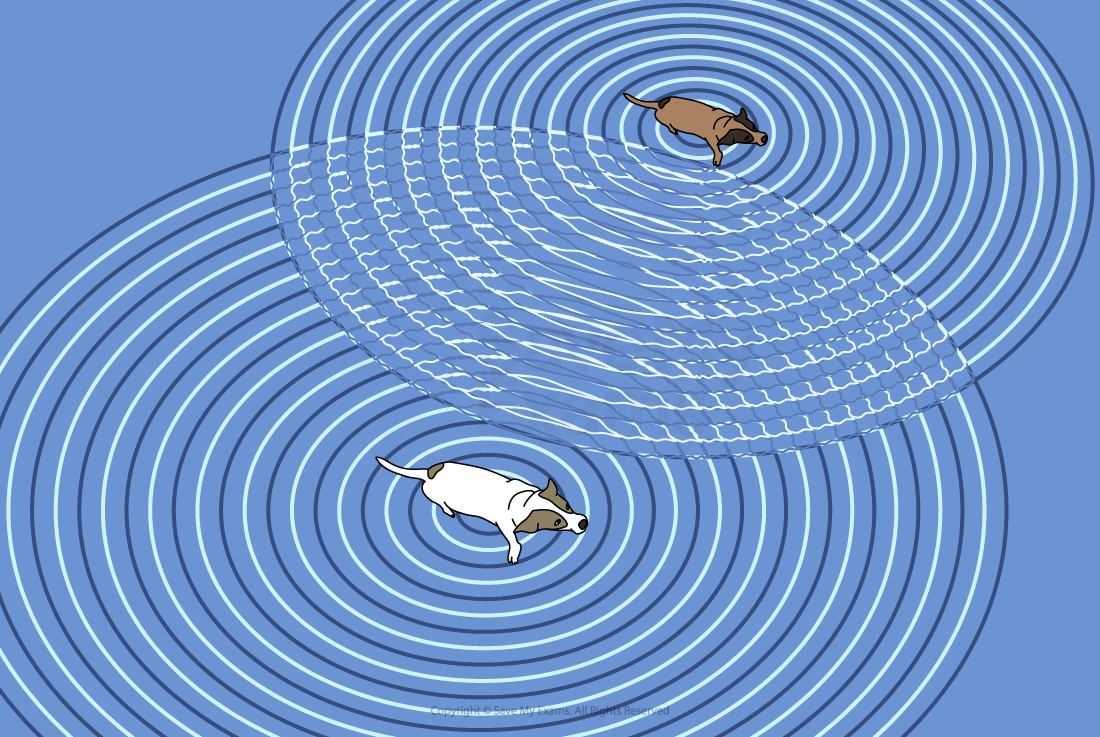
The dogs make waves in the water which superimpose to give areas of both zero and increased displacement.
It is possible to analyse superposition clearly when the waves are drawn on a vertical displacement (amplitude)-displacement graph
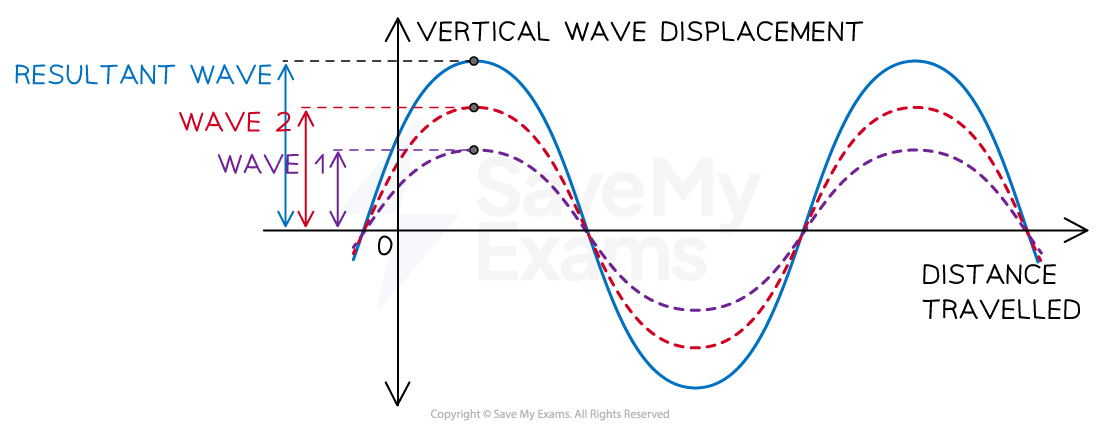
Waves can superimpose so their amplitudes are added together often creating a larger resultant amplitude
Interference is the effect of this overlap
This is explained in the next Interference of Waves
Individual wave displacements may be positive or negative and are combined in the same way as other vector quantities
It is possible to analyse superposition clearly when the waves are drawn on a displacement-time graph
Superposition can also be demonstrated with two pulses
When the pulses meet, the resultant displacement is also the algebraic sum of the displacement of the individual pulses
After the pulses have interacted, they then carry on as normal
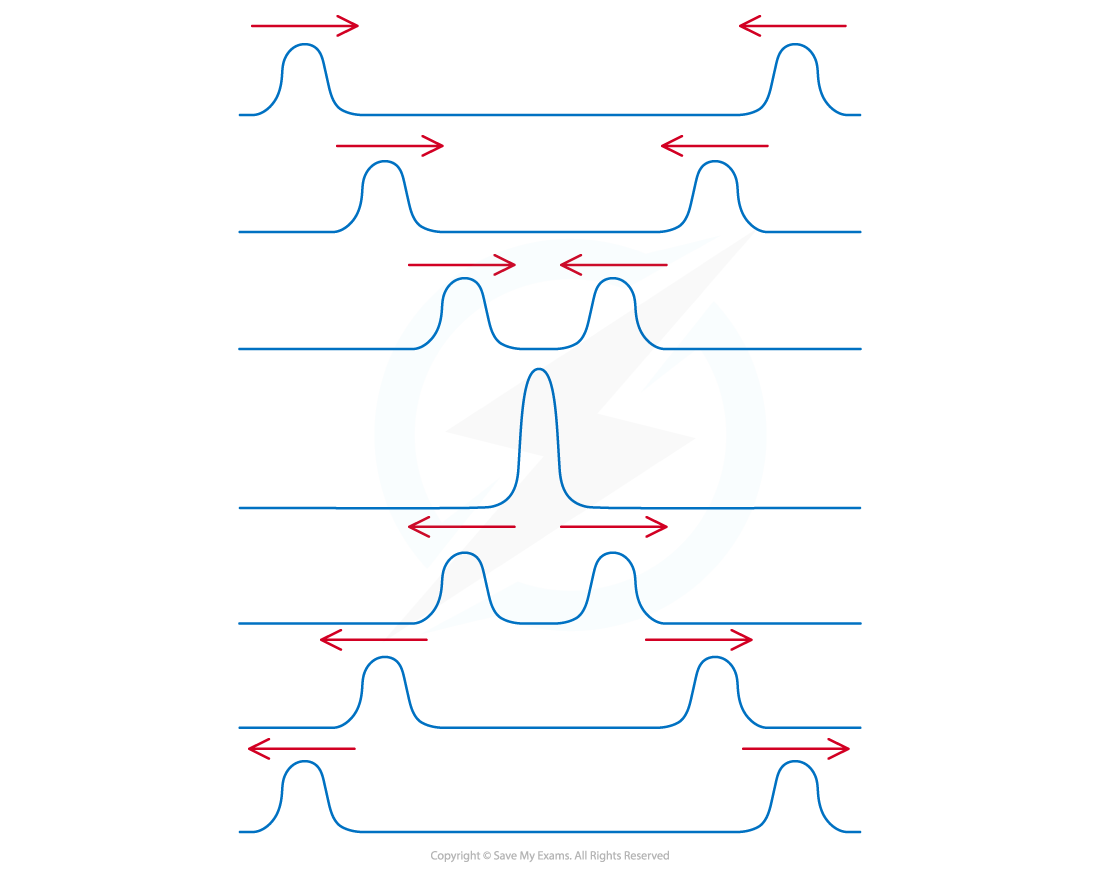
When two pulses overlap their displacements combine to form a resultant displacement
Worked Example
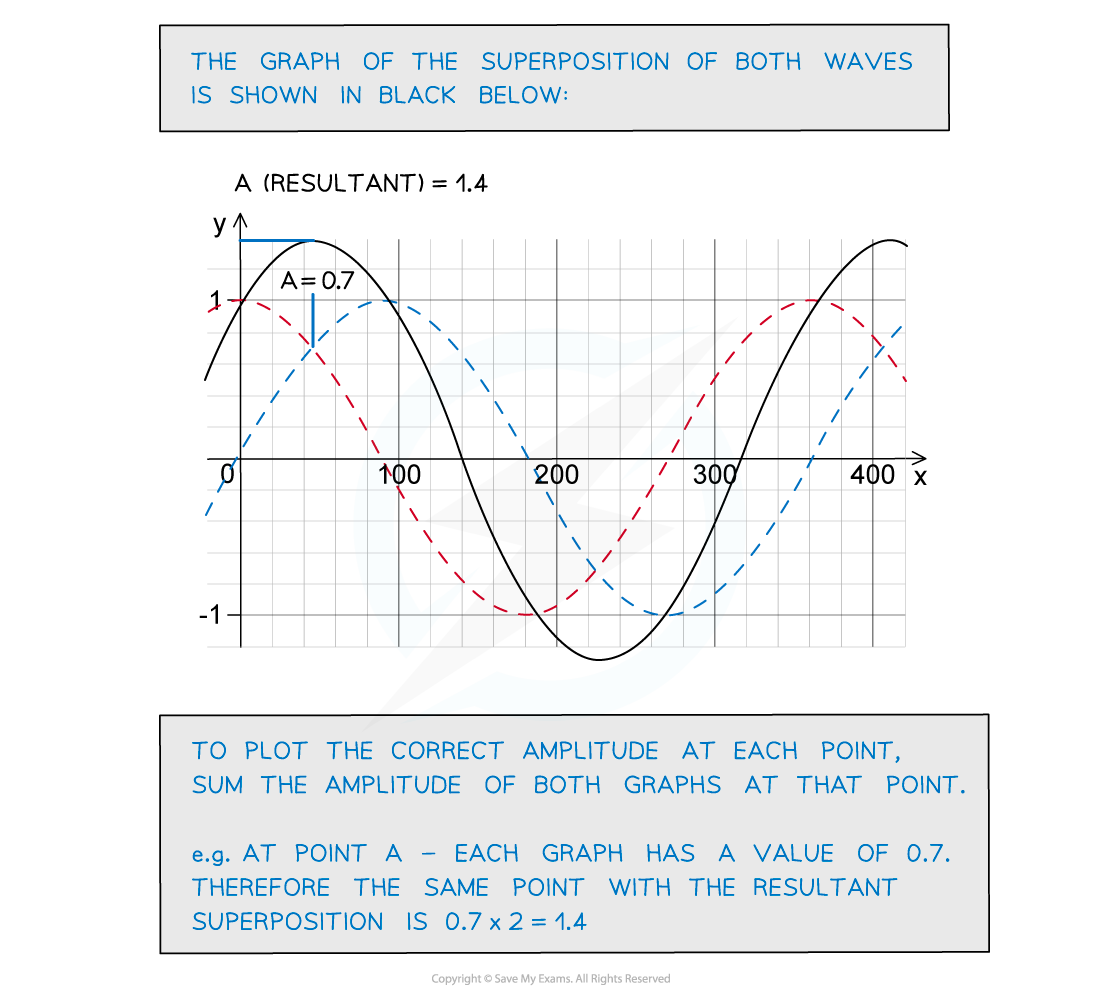
Two overlapping waves of the same type travel in the same direction. The variation with x and y displacement of the wave is shown in the figure below.
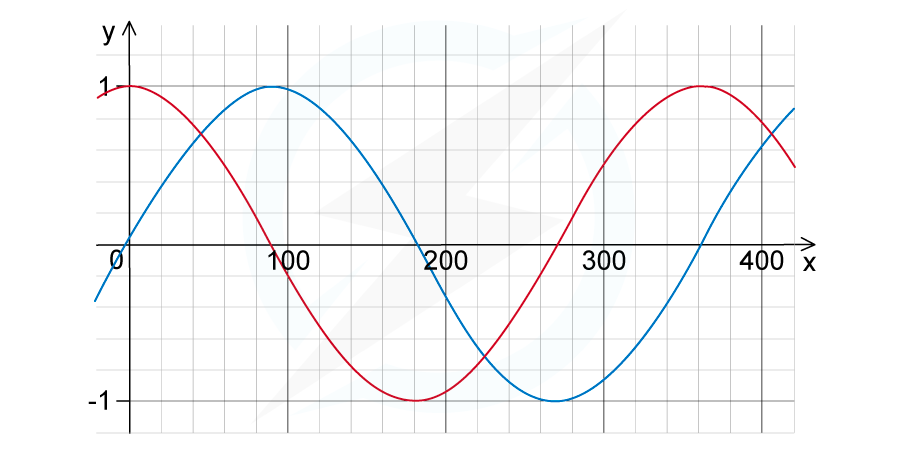
Use the principle of superposition to sketch the resultant wave.
Answer:

Unlock more, it's free!
Did this page help you?