Diffraction of Waves

Diffraction is defined as:

The spreading out of waves after they pass through a narrow gap or around an obstruction

Diffraction can occur when waves:
- pass through an aperture
- pass around a barrier

$diffraction-in-a-harbour$

Water waves diffract through a gap in a barrier such as in a harbour

Diffraction through an aperture

When a wave passes through a gap or aperture:
- The waves spread out so they have curvature
- The amplitude of the wave is less because the barrier on either side of the gap absorbs wave energy

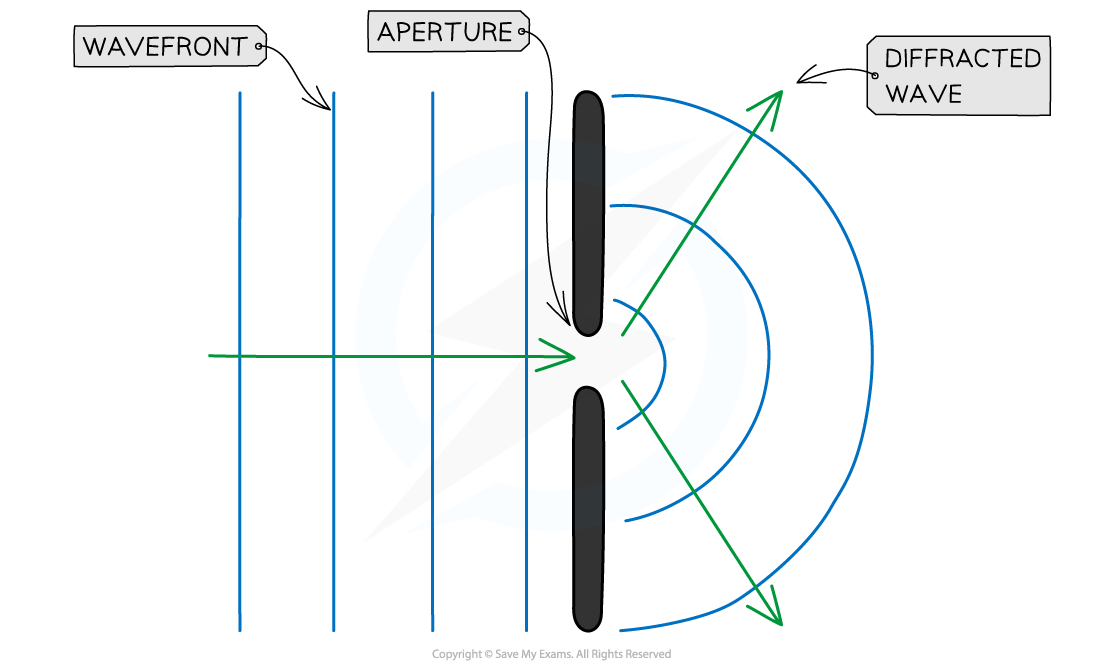
$Diffraction Wavefronts, downloadable AS & A Level Physics revision notes$

Diffraction causes waves to spread out and become curved after passing through a narrow gap

When the wavelength of the wave and the width of the gap is similar then diffraction occurs:
- When the wavelength is bigger than the gap then more diffraction occurs, so the wave spreads out more after passing through
- When the wavelength is smaller than the gap then less diffraction occurs, so the wave spreads out less after passing through
When the wavelength of the wave and the width of the gap are not similar then diffraction does not occur:
- For gaps that are much smaller than the wavelength of the wave, the wave passes over the gap easily so no diffraction occurs
- For gaps that are much bigger than the wavelength of the wave, the majority of the wave passes straight through the gap so no diffraction occurs

As the gap size increases, compared to the wavelength, the amount of curvature on the waves gets less pronounced

Effect of aperture size on diffraction

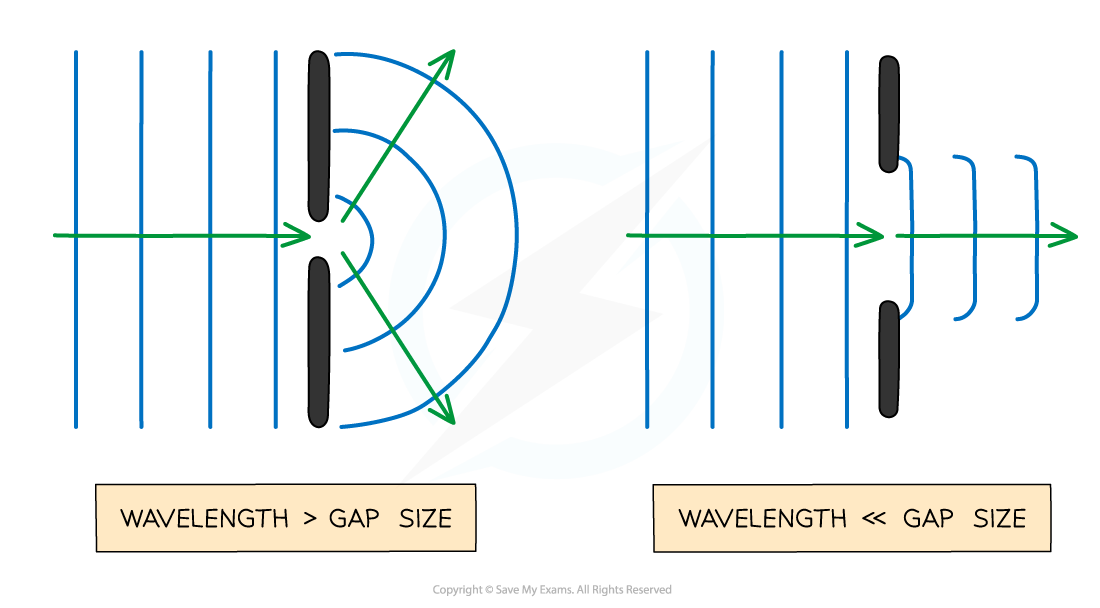
$Diffraction gap size, downloadable AS & A Level Physics revision notes$

The size of the gap (compared to the wavelength) affects how much the waves spread out when diffracted through a gap

Diffraction around a barrier

Diffraction can also occur when waves curve around an edge or barrier
- The waves spread out to fill the gap behind the object
The extent of this diffraction also depends upon the wavelength of the waves
- The greater the wavelength then the greater the diffraction

$long-and-short-wavelength-barrier-diffraction-1$

When a wave goes past the edge of a barrier, the waves can curve around it. Shorter wavelengths undergo less diffraction than longer wavelengths

When the barrier is larger than the wavelength:
- There is some diffraction around the barrier
- A lot of incident waves are reflected back towards the source
- There is a "shadow" region behind the barrier where no wavefronts are present

When the barrier is the same size as the wavelength:
- There is more diffraction around the barrier
- There is a smaller "shadow" region behind the barrier where no wavefronts are present

When the barrier is smaller than the wavelength:
- No diffraction occurs around the barrier
- The "shadow" region behind the barrier is very small

$diffraction-around-a-body$

The size of the barrier in relation to the wavelength affects how the waves diffract around it

Worked example

When a wave is travelling through the air, which scenario best demonstrates diffraction?

A. UV radiation through a gate post

B. Sound waves passing a diffraction grating

C. Radio waves passing between human hair

D. X-rays passing through atoms in a crystalline solid

Answer: D

Diffraction is most prominent when the wavelength is close to the aperture size

Consider option A:

UV waves have a wavelength between (4 × 10^–7) and (1 × 10^–8) m so would not be diffracted by a gate post
Radio waves, microwaves or sound waves would be more likely to be diffracted at this scale

Consider option B:

Sound waves have a wavelength of (1.72 × 10^–2) to 17 m so would not be diffracted by the diffraction grating
Infrared, light and ultraviolet waves would be more likely to be diffracted at this scale

Consider option C:

Radio waves have a wavelength of 0.1 to 10⁶ m so would not be diffracted by human hair
- Infrared, light and ultraviolet waves would be more likely to be diffracted at this scale

Consider option D:

X-rays have a wavelength of (1 × 10^–8) to (4 × 10^–13) m
- This is a suitable estimate for the size of the gap between atoms in a crystalline solid
- Hence X-rays could be diffracted by a crystalline solid
Therefore, the correct answer is D

Worked example

An electric guitar student is practising in his room. He has not completely shut the door of his room, and there is a gap of about 10 cm between the door and the door frame.

Determine the frequencies of sound that are best diffracted through the gap.

The speed of sound can be taken to be 340 m s^–1

Answer:

Step 1: Optimal diffraction happens when the wavelength of the waves is comparable to (or larger than) the size of the gap

λ = 10 cm = 0.1 m

Step 2: Write down the wave equation

$v = f λ$

Where speed of sound, v = 340 m s^–1

Step 3: Rearrange the above equation for the frequency f

$f = \frac{v}{λ}$

Step 4: Substitute the numbers into the above equation

$f = \frac{340}{0.1} = 3400 Hz$

The frequencies of sound that are best diffracted through the gap are:

f ≤ 3400 Hz

Examiner Tip

When drawing diffracted waves, take care to keep the wavelength (the distance between each wavefront) constant.

Diffraction of Waves (SL IB Physics)

Revision Note