Nodes & Antinodes
- A standing wave is made up nodes and antinodes
- Nodes are locations of zero amplitude and they are separated by half a wavelength (λ/2)
- Antinodes are locations of maximum amplitude
- The nodes and antinodes do not move along the wave
- Nodes are fixed and antinodes only oscillate in the vertical direction
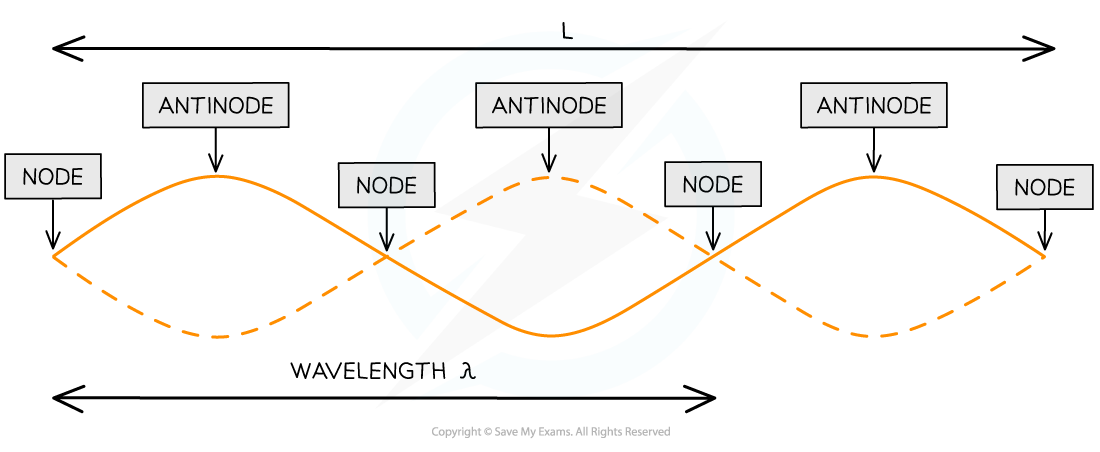
Nodes and antinodes of a stationary wave of wavelength λ on a string of length L at a point in time
The Formation of Nodes and Antinodes
- At the nodes:
- The waves are in anti-phase meaning destructive interference occurs
- The crest of one wave meets the trough of another
- This causes the two waves to cancel each other out
- At the antinodes:
- The waves are in phase meaning constructive interference occurs
- The crest of one wave meets the crest of another (same for troughs)
- This causes the waves to add together
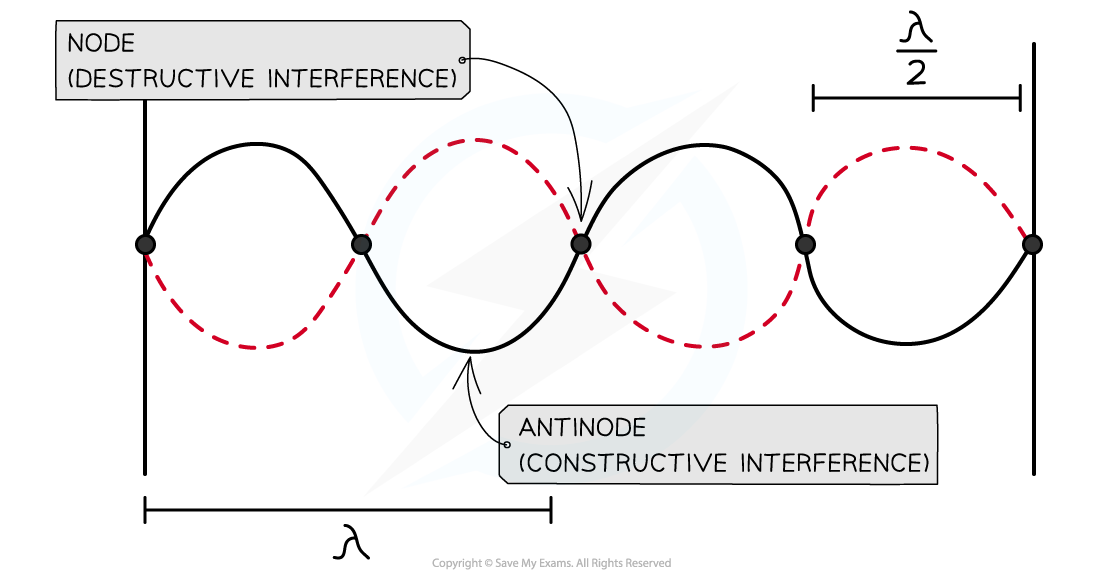
Nodes and antinodes are a result of destructive and constructive interference respectively
Phase on a Standing Wave
- Two points on a standing wave are either in phase or in anti-phase
- Points that have an odd number of nodes between them are in anti-phase
- Points that have an even number of nodes between them are in phase
- All points within a "loop" are in phase
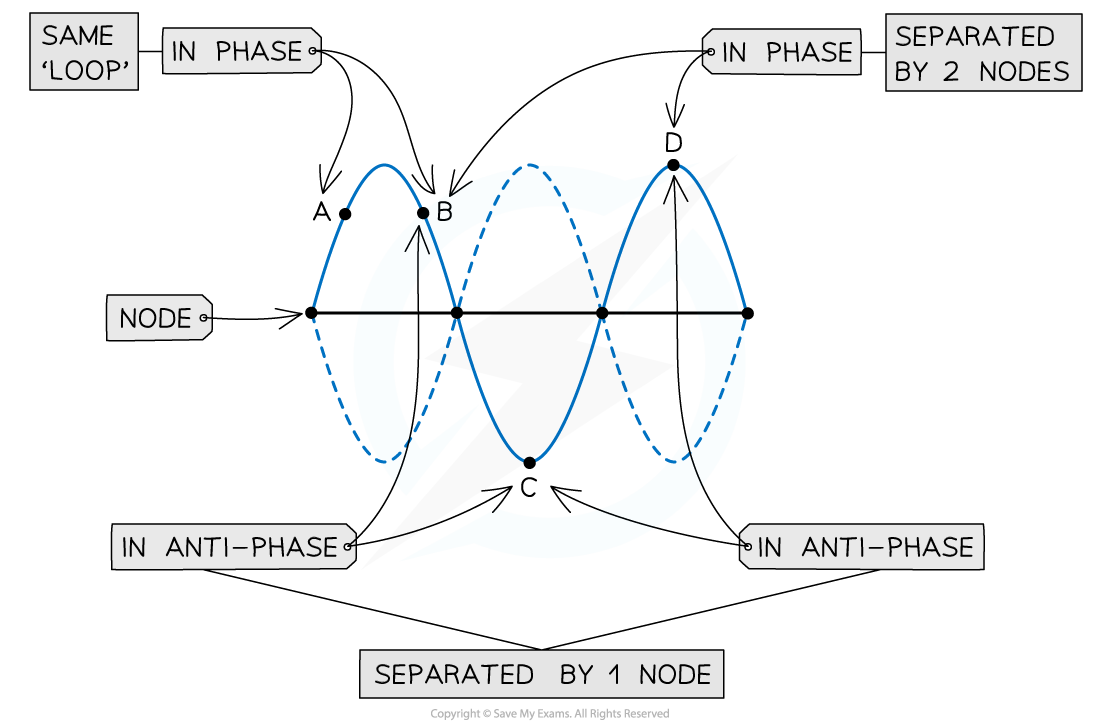
Points A, B and D are all in phase. While points A and D are in antiphase with point C
- Constructive and destructive interference can be seen from the phase differences between two waves
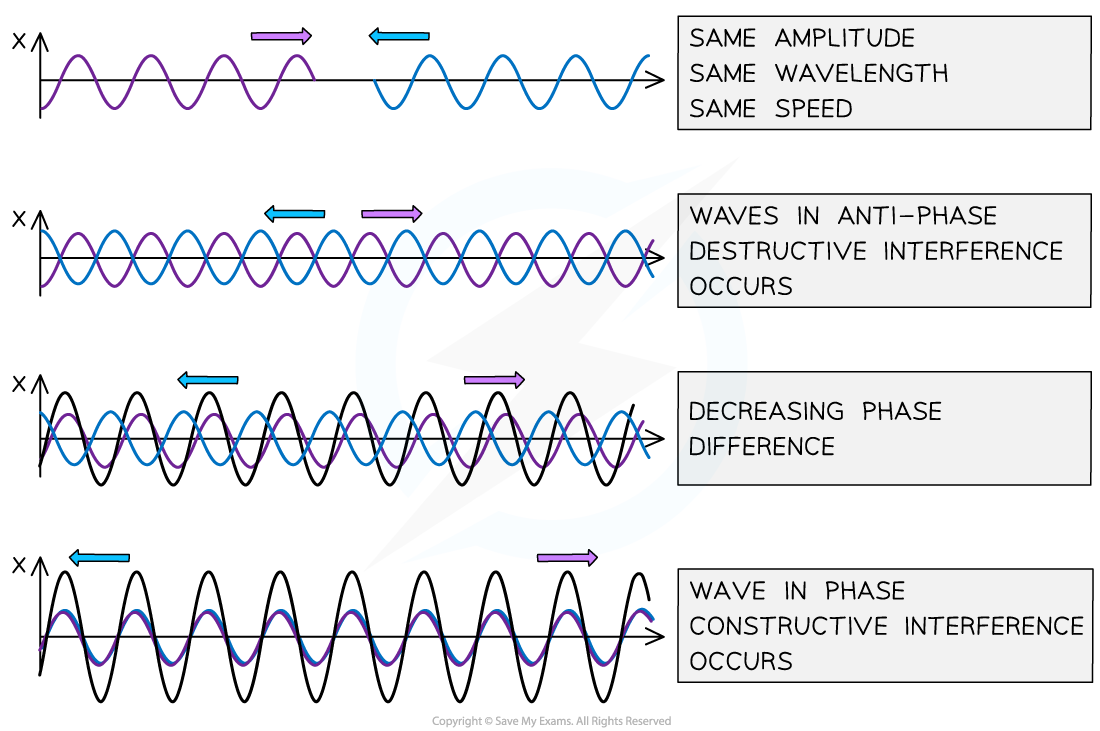
A graphical representation of how stationary waves are formed - the black line represents the resulting wave
Worked example
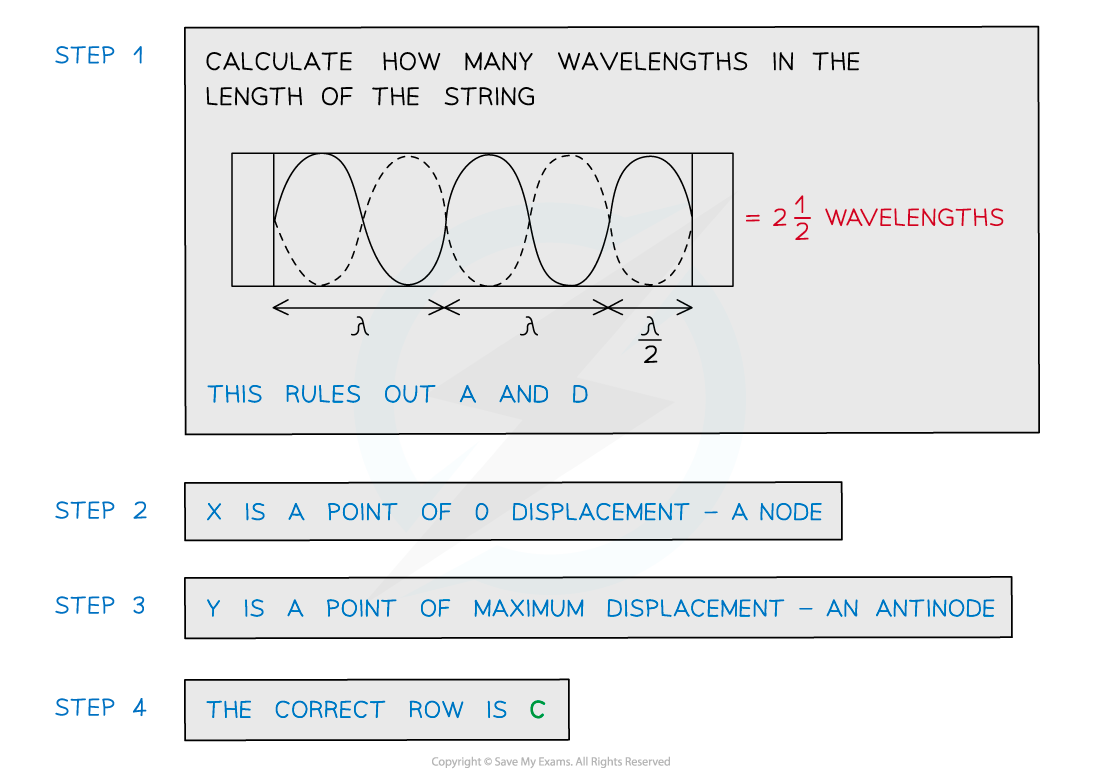
Which row in the table correctly describes the length of L and the name of X and Y?
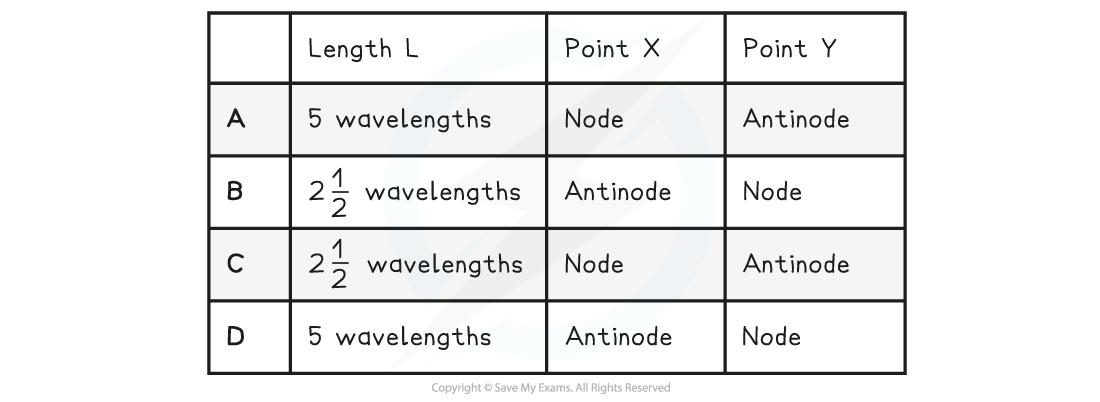
Answer: C
Examiner Tip
Phase difference on standing waves is different to travelling (progressive) waves.
Phase differences between two points on travelling waves can be anything from 0 to 2π. Between two points on a standing wave can only be in-phase (0 phase difference) or anti-phase (π out of phase).
