Syllabus Edition

First teaching 2023

First exams 2025

Describing Oscillations (SL IB Physics)

Revision Note

Test yourself Flashcards

Author

Katie M

Last updated

7 May 2024

Properties of Oscillations

An oscillation is defined as follows:

The repetitive variation with time t of the displacement x of an object about the equilibrium position (x = 0)

4-1-1-graphing-oscillations_sl-physics-rn

A pendulum oscillates between A and B. On a displacement-time graph, the oscillating motion of the pendulum is represented by a wave, with an amplitude equal to x₀

A particle undergoing an oscillation can be described using the following properties:
- Equilibrium position (x = 0) is the position when there is no resultant force acting on an object
  - This is the fixed central point that the object oscillates around
- Displacement (x) is the horizontal or vertical distance of a point on the wave from its equilibrium position
  - It is a vector quantity
  - It can be positive or negative depending on which side of the oscillation it is
  - It is measured in metres (m)
- Period (T) or time period, is the time interval for one complete oscillation measured in seconds (s)
  - If the oscillations have a constant period, they are said to be isochronous

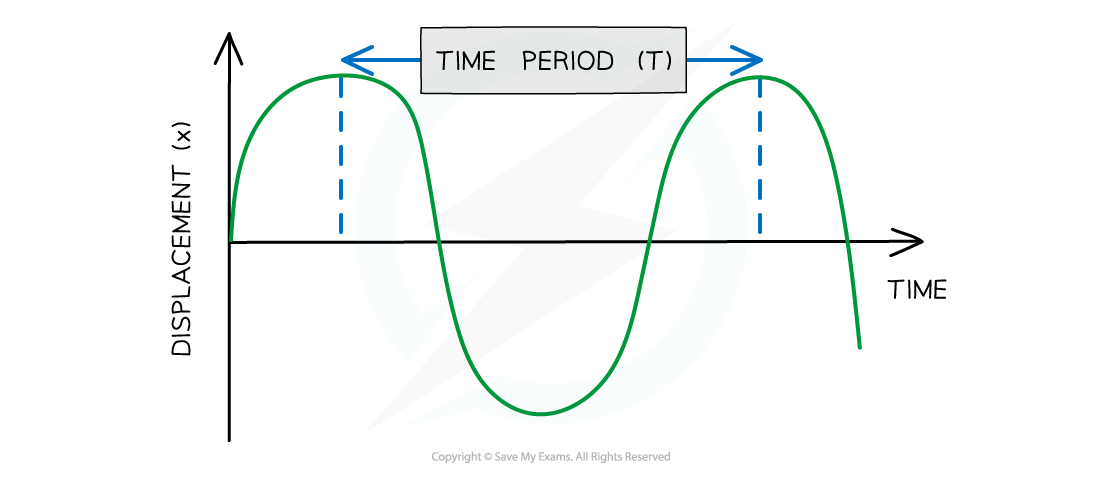
Displacement time wave, downloadable AS & A Level Physics revision notes

Diagram showing the time period of a wave

- Amplitude (x₀) is the maximum value of the displacement on either side of the equilibrium position
  - Amplitude is measured in metres (m)

5-5-4-amplitude-explanation

When the pendulum is in its extreme position this is its amplitude

- Frequency (f) is the number of oscillations per second and it is measured in hertz (Hz)
  - Hz has the SI units per second s⁻¹ because $f = \frac{1}{T}$ see below
- Angular frequency (ω) is the rate of change of angular displacement with respect to time
  - It is measured in radians per second (rad s⁻¹)

Worked example

The diagram below shows plane waves on the surface of water at a particular instant. A and B are two points on the wave.

WE - Wave properties question image, downloadable AS & A Level Physics revision notes

Determine:

(a) The amplitude

(b) The wavelength

Answer:

worked-example

Examiner Tip

When labelling the amplitude and time period on a diagram:

Make sure that your arrows go from the very top of a wave to the very top of the next one
If your arrow is too short, you will lose marks
The same goes for labelling amplitude, don’t draw an arrow from the bottom to the top of the wave, this will lose you marks too.

Calculating Time Period of an Oscillation

This equation relates the frequency and the time period of an oscillation:

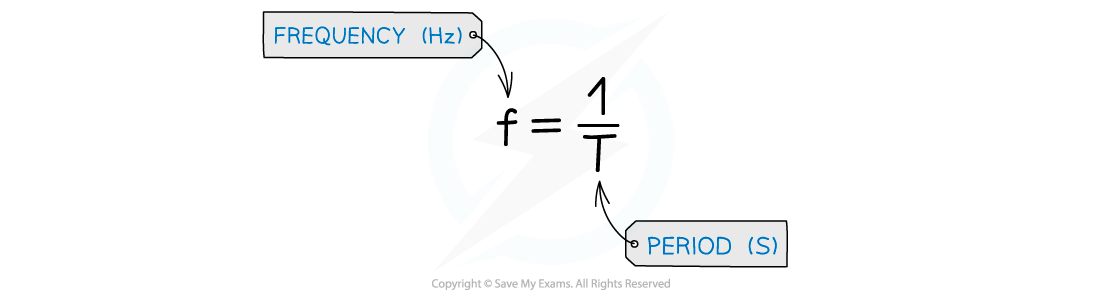
The equation linking time period and frequency

Angular frequency (⍵) can be calculated using the equation:

$ω = \frac{2 π}{T} = 2 π f$

Where:
- ⍵ = angular frequency (rad s^-1)
- 2π = circumference of a circle
- T = time period (s)
- f = frequency of oscillation (Hz)
The angular displacement of objects in oscillation can be determined by matching the displacement to an object in circular motion:
- After moving from one amplitude position x = −A to the equilibrium position x = 0 the mass on the spring has moved an angular displacement of $\frac{1}{4}$ of a circle = $\frac{1}{4} \times 2 π$ = $\frac{π}{2}$ radians
- Continuing the oscillation from the equilibrium position to the other amplitude position the angular displacement is also $\frac{π}{2}$ radians
- Continuing the oscillation back to the starting point means the mass travels a further angular displacement of $\frac{π}{2} + \frac{π}{2} = π$ radians
- Hence, the total angular displacement in one oscillation is $π + π = 2 π$ radians

5-5-2-angular-frequency-oscillation-link

The motion of an oscillating object can be analysed in terms of a fraction of an object in circular motion

Worked example

A child on a swing performs 0.2 oscillations per second.

Calculate the time period of the oscillation.

Answer:

Step 1: Write down the known quantities

Frequency, f = 0.2 Hz

Step 2: Write down the relationship between the period T and the frequency f

$T = \frac{1}{f}$

Step 3: Substitute the value of the frequency into the above equation and calculate the period

$T = \frac{1}{0.2} = 5.0 s$

Worked example

A cuckoo in a cuckoo clock emerges from a fully compressed position to a fully extended position in 1.5 seconds.

Calculate the angular frequency of the cuckoo as it emerges from the clock.

Answer:

Step 1: Consider the motion of the cuckoo

The cuckoo goes from being fully compressed to fully extended which means that it travels for an angular displacement of half a circle and not a full circle
So, the angular displacement will be π

5-5-2-angular-frequency-we_ocr-al-physics