Measurement Techniques in IB Physics (DP IB Physics): Revision Note
Measurement Techniques
Common instruments used in Physics are:
Metre rules - to measure distance and length
Thermometers - to measure temperature
Measuring cylinders - to measure to volume of liquid or the volume of displaced liquid
Balances - to measure mass
Newtonmeters - to measure force
Protractors - to measure angles
Stopwatches - to measure time
Ammeters - to measure current
Voltmeters - to measure potential difference
Sound meter - to measure the intensity of sound
Light meter - to measure the intensity of light
More complicated instruments such as the micrometer screw gauge and Vernier calipers can be used to measure thicknesses, diameters and lengths to a greater degree of accuracy
When using measuring instruments like these you need to ensure that you are fully aware of what each division on a scale represents
This is known as the resolution
The resolution is the smallest change in the physical quantity being measured that results in a change in the reading given by the measuring instrument
The smaller the change that can be measured by the instrument, the greater the degree of resolution
For example, a standard mercury thermometer has a resolution of 1°C whereas a typical digital thermometer will have a resolution of 0.1°C
The digital thermometer has a higher resolution than the mercury thermometer
Measuring Instruments Table
Quantity | Instrument | Typical Resolution |
|---|---|---|
Length | Meter Rule | 1 mm |
Thickness or length | Vernier Calipers | 0.01 mm |
Thickness or length | Micrometer | 0.001 mm |
Mass | Top-Pan Balance | 0.01 g |
Angle | Protractor | 1° |
Time | Stopwatch | 0.01 s |
Temperature | Thermometer | 1 °C |
Potential Difference | Voltmeter | 1 mV - 0.1 V |
Current | Ammeter | 1 mA - 0.1 A |
Controlling Variables
For an experiment to be valid, it is essential that any variable that may affect the outcome of an experiment is controlled
Some of the key practical skills that are required to do so are as follows:
Calibration of measuring apparatus
Keeping certain environmental conditions constant
Insulation against heat loss or gain
Reduction of friction
Reduction of electrical resistance
Taking background radiation into account
Calibration of Measuring Apparatus
Calibration is a comparison between a known measurement and the measurement you achieve using the instrument
This checks the accuracy of the instrument, especially for higher readings
For example, checking whether a meter (e.g., voltmeter, micrometer, ammeter) reads zero before measurements are made
This helps to avoid zero error
Calibrating sensors
Calibration curves are used to convert measurements made on one measurement scale to another measurement scale
These are useful in experiments when the instruments used have outputs which are not proportional to the value they are measuring
For example, e.m.f and temperature (thermocouple) or resistance against temperature (thermistor)
The calibration curve for a thermocouple, in which the e.m.f varies with temperature, is shown below:
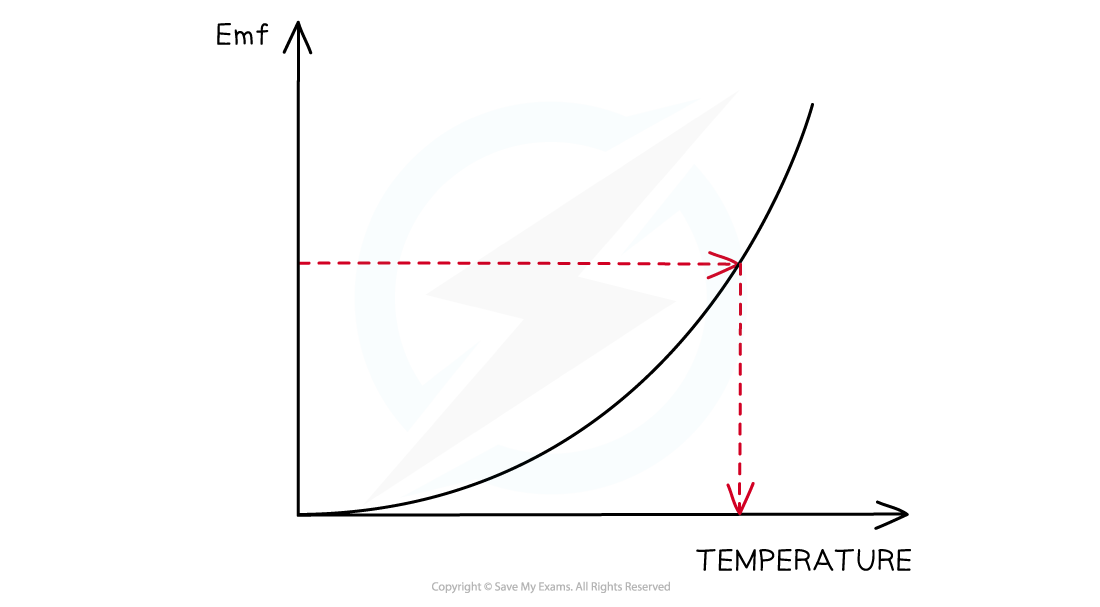
A curve of voltage against temperature can be used as a temperature sensor
Maintaining Constant Conditions
In an experiment, a variable is any factor that could change or be changed
There are different types of variables within an experiment
The independent variable: the only variable that should be changed throughout an experiment
The dependent variable: the variable that is measured to determine the outcome of an experiment (the results)
The controlled variables: any other variables that may affect the results of the experiment that need to be controlled or monitored
It is essential that any variable that may affect the outcome of an experiment is controlled in order for the results to be valid and to have a fair test
A fair test is one in which only the independent variable has been allowed to affect the dependent variable
Controlling Heat Losses & Gains
Energy transfers by heating due to conduction are one of the most common sources of dissipated energy
To reduce energy transfers by conduction, materials with a low thermal conductivity should be used
Materials with low thermal conduction are called insulators
Insulation reduces energy transfers from both conduction and convection
The effectiveness of an insulator is dependent upon:
1. The thermal conductivity of the material
The lower the conductivity, the lower the amount of heat loss
2. The density of the material
The more dense the insulator, the more conduction can occur
In a denser material, the particles are closer together so they can transfer energy to one another more easily
3. The thickness of the material
The thicker the material, the lower the amount of heat loss

Less energy is transferred by conduction and convection if the cavity is insulated
Reducing Friction
In a mechanical system, there is often friction between the moving parts of the machinery
This results in unwanted energy transfers by heating the machinery and the surroundings
Friction can be reduced by:
Adding bearings to prevent components from directly rubbing together
Lubricating parts
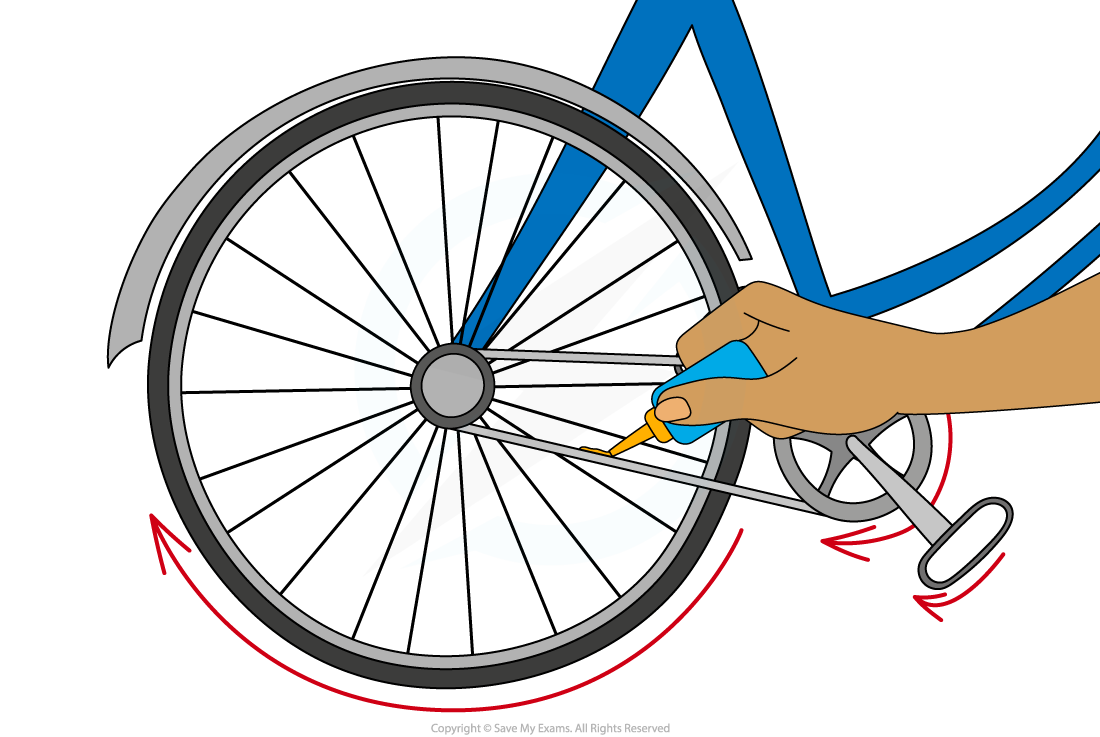
Lubricating parts of a bicycle to reduce friction
Reducing Electrical Resistance
In electric circuits, there is resistance as current flows through the wires and components
This results in unwanted energy transfers by heating to the wires, components and the surroundings
Resistance can be reduced by:
Using components with lower resistance
Reducing the current
Adjusting for Background Radiation
Although most background radiation is natural, a small amount of it comes from artificial sources, such as medical procedures (including X-rays)
Levels of background radiation can vary significantly from place to place
When conducting experiments to measure the radiation coming from radioactive sources, the background radiation must be taken into account, to do this:
Place a Geiger-Muller tube away from any radioactive sources and measure the background count
Carry out the experiment with the radioactive source
Subtract the background count from each reading to obtain the count rate from the source only
Worked Example
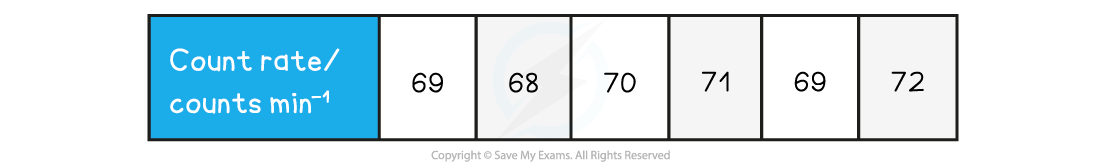
A student measures the background radiation count in a laboratory and obtains the following readings:
The student is trying to verify the inverse square law of gamma radiation on a sample of Radium-226. He collects the following data:
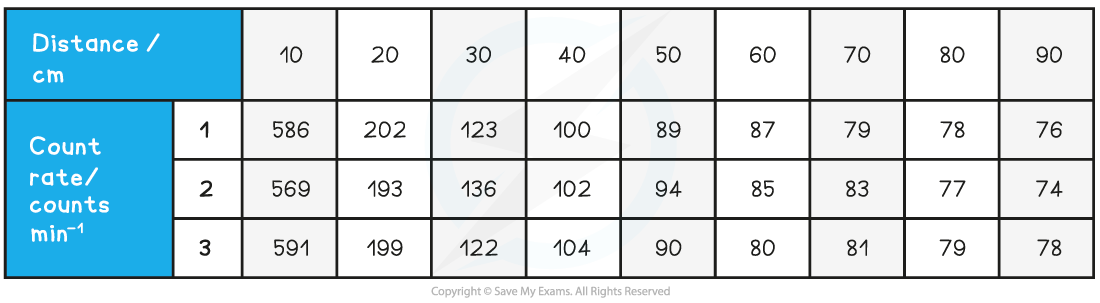
Use this data to determine if the student’s data follows an inverse square law.
Answer:
Step 1: Determine a mean value of background radiation
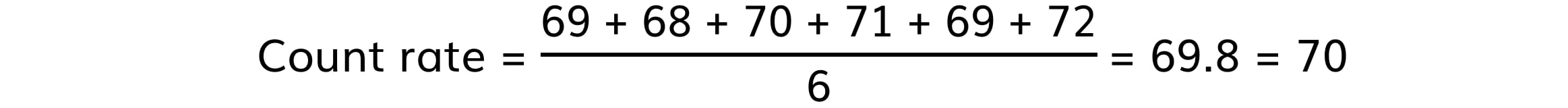
The background radiation must be subtracted from each count rate reading to determine the corrected count rate, C
Step 2: Compare the inverse square law to the equation of a straight line
According to the inverse square law, the intensity, I, of the γ radiation from a point source depends on the distance, x, from the source
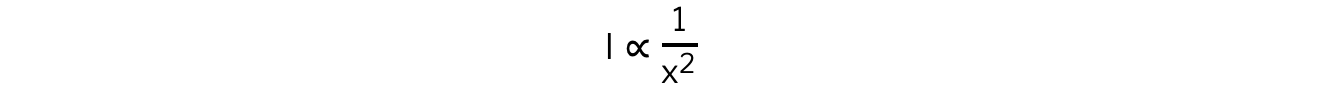
Intensity is proportional to the corrected count rate, C, so
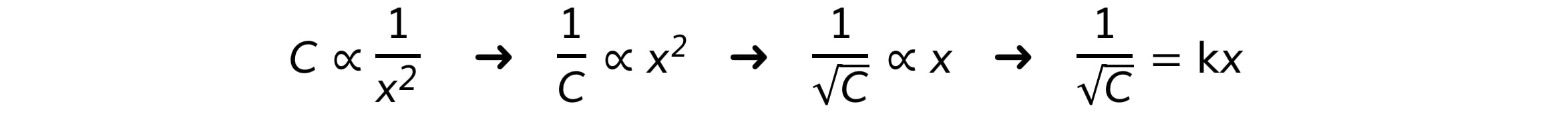
The graph provided is of the form 1/C–1/2 against x
Comparing this to the equation of a straight line, y = mx
y = 1/C–1/2 (counts min–1/2)
x = x (m)
Gradient = constant, k
If it is a straight line graph through the origin, this shows they are directly proportional, and the inverse square relationship is confirmed
Step 3: Calculate C (corrected average count rate) and C–1/2
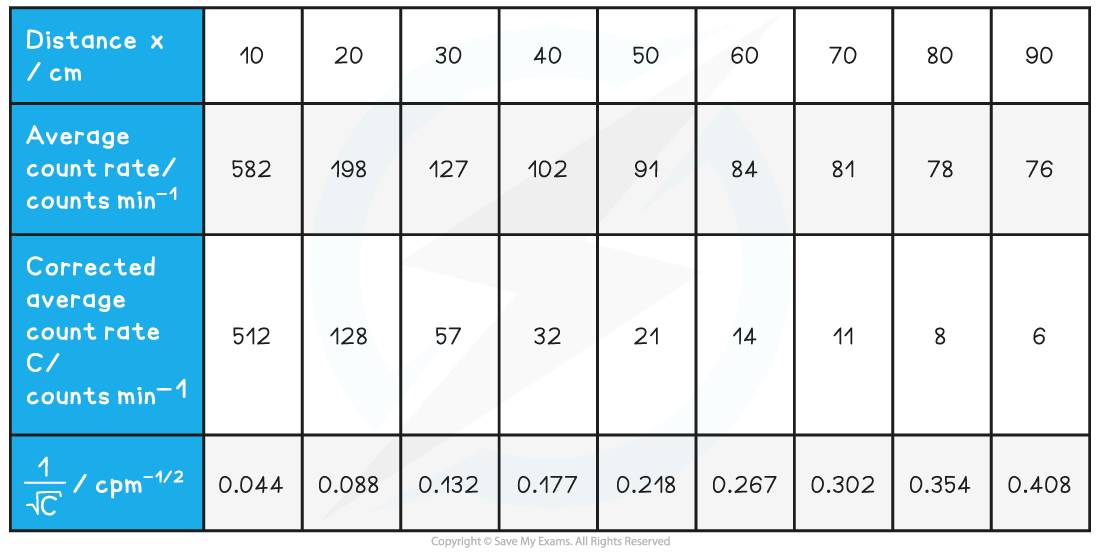
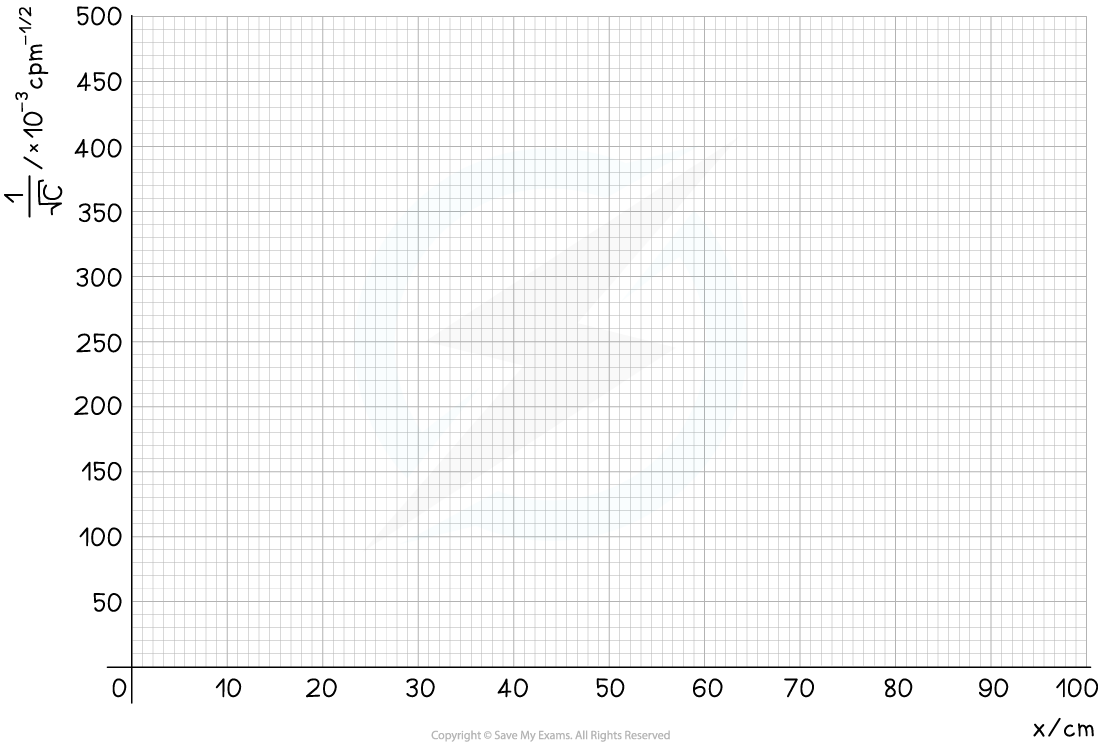
Step 4: Plot a graph of C–1/2 against x and draw a line of best fit

The graph shows C–1/2 is directly proportional to x, therefore, the data follows an inverse square law

Unlock more, it's free!
Did this page help you?