Standing Waves & Resonance (DP IB Physics)
Flashcards
1/560Still learning
Know0
- FrontStanding Waves
What is a standing wave?
BackStanding WavesA standing wave is produced by the superposition of two waves traveling in opposite directions with the same frequency.
- FrontStanding Waves
True or False?
Standing waves transfer energy.
BackStanding WavesFalse.
Standing waves store energy. Progressive waves transfer energy.
- FrontStanding Waves
What is the main difference between progressive and standing waves?
BackStanding WavesThe main difference is that progressive waves transfer energy, while standing waves store energy.
Did this page help you?
Cards in this collection (56)
What is a standing wave?
A standing wave is produced by the superposition of two waves traveling in opposite directions with the same frequency.
True or False?
Standing waves transfer energy.
False.
Standing waves store energy. Progressive waves transfer energy.
What is the main difference between progressive and standing waves?
The main difference is that progressive waves transfer energy, while standing waves store energy.
Define a progressive wave.
A progressive wave is a wave that transfers energy from one place to another.
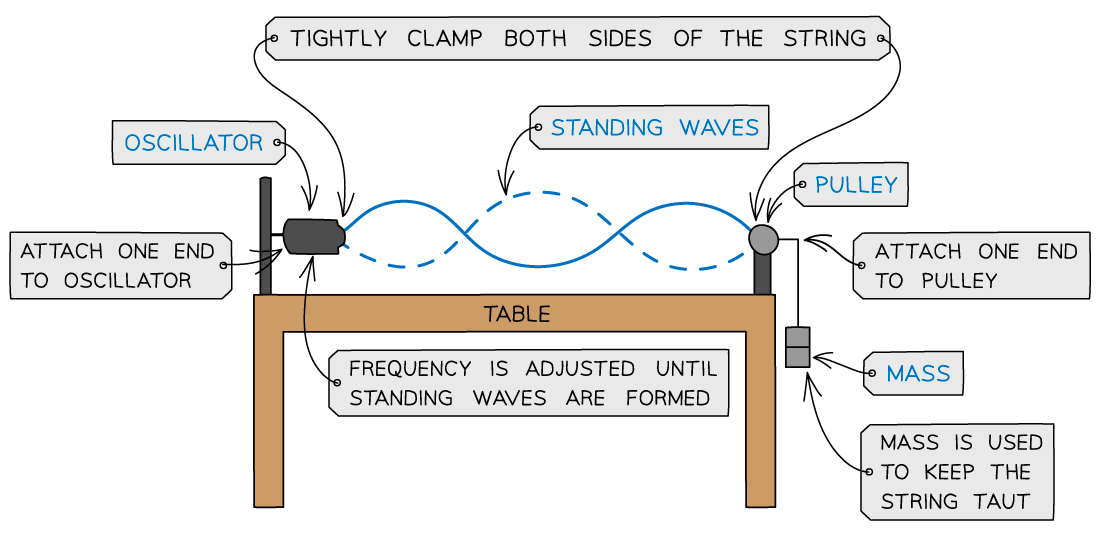
What conditions must be met for a standing wave to form?
For standing waves to form, the waves must have the same wavelength and a similar amplitude.
True or False?
The principle of superposition applies only to transverse waves.
False.
The principle of superposition applies to all types of waves, including transverse, longitudinal, progressive, and stationary waves.
State how a standing wave can be formed from the transverse wave shown in the diagram.
A standing wave can be formed from the transverse wave shown in the diagram when it is reflected from the barrier at the fixed end.
State the type of wave shown in the diagram.
The type of wave shown in the diagram is a standing wave.
What features does the wave formed on the string in the diagram have that mean it is a standing wave?
The features of the standing wave are:
the string is fixed at one end
so it is reflected back in the opposite direction to the original wave
so the original wave is superimposed by its reflection
State the location(s) of the node(s) on the stationary wave in the diagram.
The location(s) of the node(s) on the stationary wave in the diagram are at P and Q.
State the location(s) of the antinode(s) on the stationary wave in the diagram.
The location(s) of the antinode(s) on the stationary wave in the diagram is at X.
What is a node in a standing wave?
A node in a standing wave is a location of zero amplitude, where destructive interference occurs.
Define an antinode.
An antinode is a location of maximum amplitude in a standing wave, where constructive interference occurs.
True or False?
Nodes are locations of maximum amplitude.
False.
Nodes are locations of zero amplitude.
True or False?
Points within a "loop" of a standing wave are in phase.
True.
Points within a "loop" of a standing wave are in phase.
What is the phase difference between two points with an odd number of nodes between them?
The phase difference between two points with an odd number of nodes between them is
(anti-phase).
Explain the difference between phase differences in standing and travelling waves.
The phase difference between two points on travelling waves can range from
to
, while in standing waves, they can only be
(in-phase) or
(anti-phase).
State the length of the standing wave shown in the diagram in terms of wavelength,
.
The length of the standing wave shown in the diagram in terms of wavelength,
is
.
True or False?
A node is formed at the free end of a string or pipe.
False.
At the free end of a string or pipe an antinode is formed (a location of maximum displacement).
What does boundary condition mean in the context of standing waves?
Boundary condition refers to whether the ends of the medium (string or pipe) are fixed or free.
How do boundary conditions affect the formation of standing waves?
Boundary conditions affect the formation of standing waves because they determine the positions of nodes and antinodes and the possible standing wave patterns.
Define natural frequency.
Natural frequency is the specific frequency at which an integer number of half wavelengths fit on the length of the string or pipe.
What is the effect of tension on the frequency of a vibrating string?
The frequency of a vibrating string increases with increasing tension.
Which boundary conditions can exist for a pipe?
The boundary conditions that can exist for a pipe are:
closed at both ends
open at both ends
closed at one end and open at the other
What is the meaning of a fixed end in a standing wave?
A fixed end in a standing wave is a location of zero displacement, i.e., a node, where the reflected wave is in anti-phase with the incident wave.
True or False?
The reflected wave at a free end of a standing wave is in phase with the incident wave.
True.
The reflected wave at the free end of a standing wave is in phase with the incident wave.
State the harmonic shown by the guitar string in the diagram.
The first harmonic is shown by the guitar string in the diagram because it has one loop, two nodes, and an antinode.
Define harmonic.
A harmonic is a wave pattern formed at specific frequencies where standing waves occur on strings or in pipes.
What is the first harmonic?
The first harmonic is the simplest standing wave pattern formed on a string or in a pipe with one loop, two nodes, and an antinode, also known as the fundamental frequency.
State the equation for the wavelength of the
th harmonic on a string fixed at both ends.
The equation for the wavelength of the
th harmonic on a string fixed at both ends is
Where:
= wavelength of standing wave, measured in metres (m)
= length of the string, measured in metres (m)
What is the wavelength of the first harmonic on a string of length,
?
The wavelength of the first harmonic on a string of length
is
Where:
= wavelength of the first harmonic, measured in metres (m)
= length of string, measured in metres (m)
How do the boundary conditions of a string determine its harmonic?
The boundary conditions of a string determine its harmonic because:
whether the ends are fixed or free dictates the positions of the nodes and antinodes
this provides the harmonic frequencies of the string
True or False?
The
th harmonic has
nodes and
antinodes on a string fixed at both ends.
True.
The
th harmonic has
nodes and
antinodes on a string fixed at both ends.
Define natural frequency for a standing wave on a string or in a pipe.
Natural frequency in terms of a standing wave is the specific frequency at which an integer number of half wavelengths fit on the length of a string or pipe.
State the equation for the frequency of the
th harmonic of a standing wave.
The equation for the frequency of the
th harmonic of a standing wave is
Where:
= the wave speed, measured in metres per second (m s-1)
= the length of the string or pipe, measured in metres (m)
What is the equation for the wavelength of the
th harmonic in a pipe open at one end?
The equation for the wavelength of the nth harmonic in a pipe open at one end is
Where:
= an odd integer (1, 3, 5, ...).
= the length of the string or pipe, measured in metres (m)
Define a free oscillation.
A free oscillation is an oscillation where there are only internal forces (and no external forces) acting and no energy input.
What is a forced oscillation?
A forced oscillation is an oscillation produced by a periodic external force.
Define resonance.
Resonance is when the frequency of the applied force to an oscillating system is equal to its natural frequency, resulting in an oscillation at a maximum amplitude.
Explain what happens to the amplitude of an object oscillating at resonance?
When oscillating at resonance the driving frequency equals the natural frequency of the system, causing the amplitude of the oscillations to reach its maximum.
True or False?
Free oscillations occur with no external forces acting on the system.
True.
Free oscillations occur with no external forces acting on the system.
How do forced oscillations affect the frequency of osciilation?
Forced oscillations make the system oscillate at the same frequency as the external periodic driving force.
What is the driving frequency
of an oscillation?
The driving frequency
of an oscillation is the frequency of the periodic external force applied to an oscillating system.
What is an example of resonance in everyday life?
An example of resonance in everyday life is pushing a child on a swing, where small pushes at the natural frequency increase the amplitude of the swing's motion.
What is the effect of a periodic force on replacing the energy lost in damping?
The periodic force does work on resistive forces, sustaining oscillations by replacing the energy lost in damping.
Define the damping of an oscillator.
The damping of an oscillator is the reduction in energy and amplitude of oscillations due to resistive forces on the oscillating system.
When does light damping occur?
Light damping occurs when the amplitude of oscillations decreases exponentially with time.
What is critical damping?
Critical damping is when an oscillator returns to its equilibrium position in the shortest possible time without oscillating.
What is heavy damping?
Heavy damping is when an oscillator takes time to return to its equilibrium position without oscillating.
True or False?
The frequency of damped oscillations changes as the amplitude decreases.
False.
The frequency of damped oscillations does not change as the amplitude decreases.
How does light damping affect a pendulum's motion?
Light damping causes a pendulum to oscillate with gradually decreasing amplitude until it stops.
Which type of damping is used in car suspension systems?
The type of damping used in car suspension systems is critical. This prevents the car oscillating further after travelling over a bump.
What is the effect of heavy damping on a door damper?
The effect of heavy damping causes a door damper to return to its equilibrium position slowly without oscillating.
State the key feature of a displacement-time graph showing critical damping.
The key feature of a displacement-time graph showing critical damping is the object returns to its equilibrium position in the quickest possible time without oscillating.
What happens to the amplitude of resonance vibrations as damping increases?
The amplitude of resonance vibrations decreases as damping increases.
Add an arrow to the diagram to show the direction of increased damping on the amplitude of the oscillations shown in the graph.
An arrow added to the diagram to show the direction of increased damping on the amplitude of the oscillations shown in the graph is as follows: