Torque & Couples (DP IB Physics) : Revision Note
Torque & Couples
Moment of a Force
A moment is the turning effect of a force around a particular point
Moments occur when forces cause objects to rotate about some pivot
The moment of a force is given by
Moment (N m) = Force (N) × perpendicular distance from the pivot (m)
The SI unit for the moment is newton metres (N m)
Moments of perpendicular & non-perpendicular forces
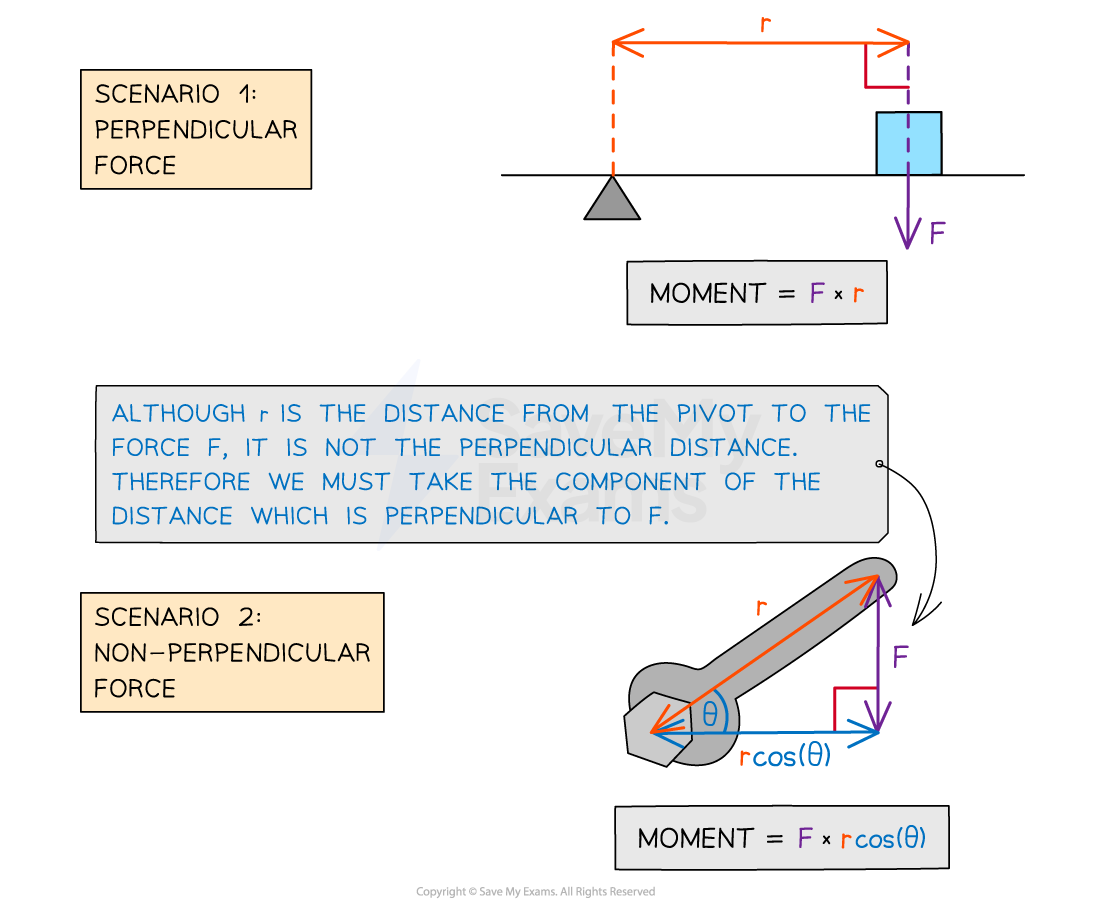
The force might not always be perpendicular to the distance. In this case, use trigonometry to resolve in the perpendicular direction
Couples
A couple is a pair of equal and opposite coplanar forces that act to produce rotation only
A couple consists of a pair of forces that are:
Equal in magnitude
Opposite in direction
Perpendicular to the distance between them
Force couple diagram
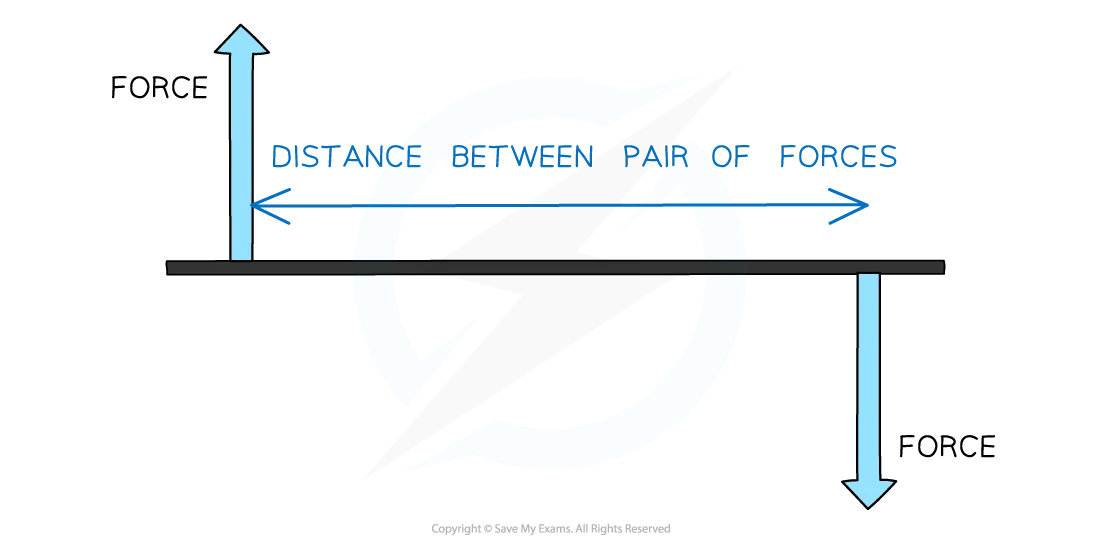
A couple must consist of two equal and opposite forces separated by a perpendicular distance
Unlike moments of a single force, the moment of a couple doesn’t depend on a pivot
The moment of a couple is equal to:
Moment (N m) = Force (N) × Perpendicular distance between the lines of action of the forces (m)
A couple does not produce a net linear force
However, it does produce a turning effect called a torque
Torque
The change in rotational motion due to a turning force is called torque
The torque of a force F about an axis is given by
Torque of a non-perpendicular force
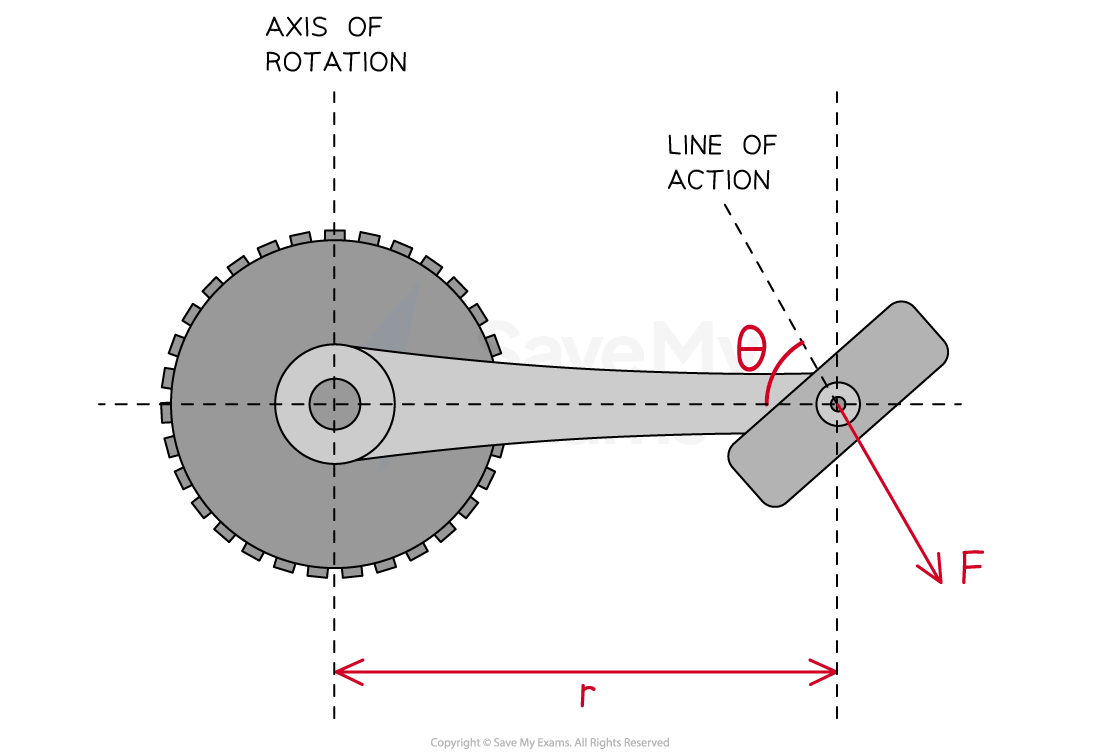
The torque applied by a cyclist on a bicycle pedal can be determined using the magnitude of the applied force and the perpendicular component of the distance between the line of action of the force and the axis of rotation
For scenarios where the forces are perpendicular (θ = 90°) to one another, the equation simplifies to
Where:
τ = torque (N m)
F = applied force (N)
r = perpendicular distance between the axis of rotation and the line of action of the force (m)
θ = angle between the force and the axis of rotation (°)
Torque of a couple on a steering wheel
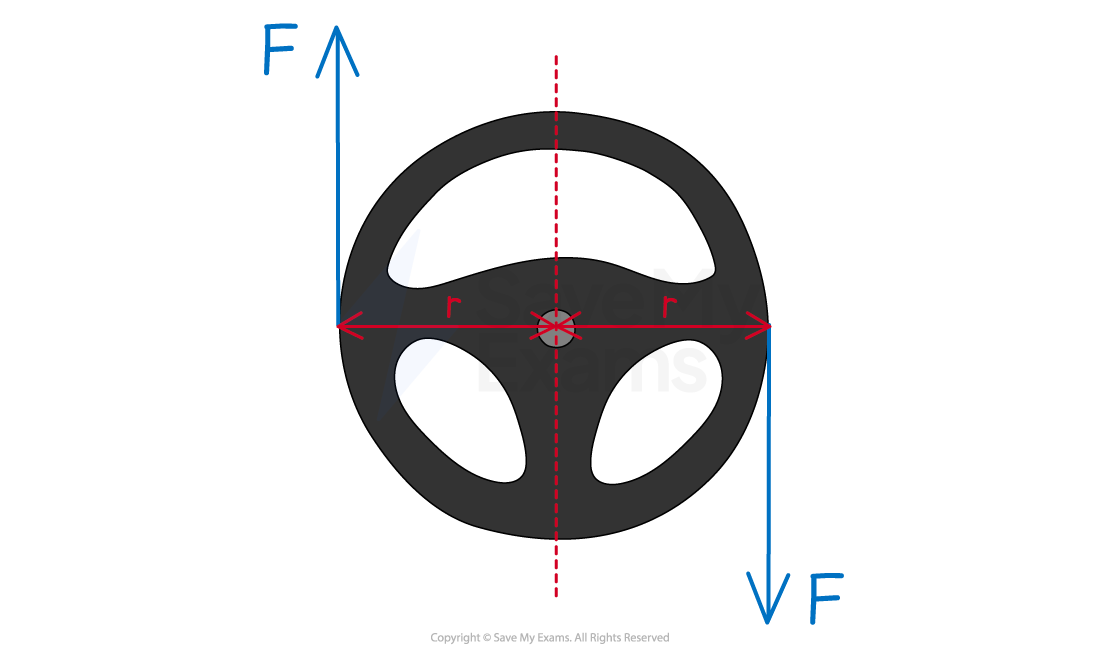
The steering wheel is acted on by a couple; it has zero resultant force but a net torque. This causes angular acceleration.
When applied to a couple, torque can be described as:
The sum of the moments produced by each of the forces in the couple
For example, the net torque provided by a couple on a steering wheel of radius
is:
Since , then
Therefore, the net torque of a couple is equal to double the magnitude of the torque of the individual forces
The forces in a couple are equal in magnitude and act in opposite directions, which means:
the resultant force is zero
the wheel does not have a linear acceleration
the wheel is in translational equilibrium
However, since the couple produces a net torque, the wheel experiences an angular acceleration
In other words, when the force is applied, the steering wheel rotates and remains in the same location, but its angular speed increases
The effect of angle on torque
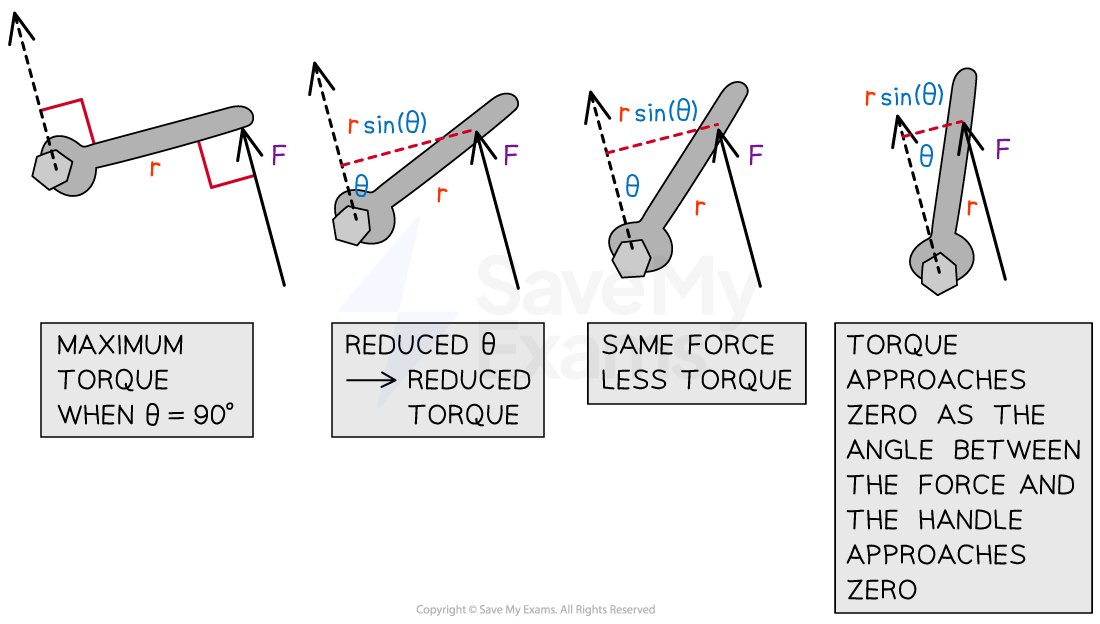
The force produces a maximum torque when applied perpendicular to the wrench. The same force is less effective when applied at non-perpendicular angles
Worked Example
Which pair of forces acts as a couple on the circular object?

Answer: A
In diagram A, the forces are:
Equal in size
In opposite directions
Perpendicular to the distance between them
B is incorrect as the forces are in the same direction
C is incorrect as the forces are different in size
D is incorrect as the distance between the forces is not perpendicular
Worked Example
A ruler of length 0.3 m is pivoted at its centre.
Two equal and opposite forces of magnitude 4 N are applied to the ends of the ruler, creating a couple as shown in the diagram.
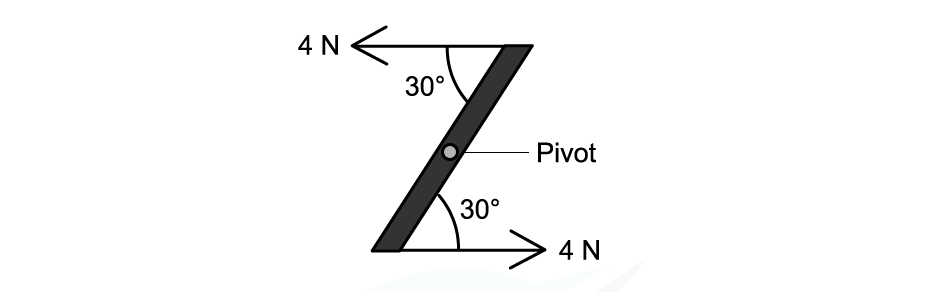
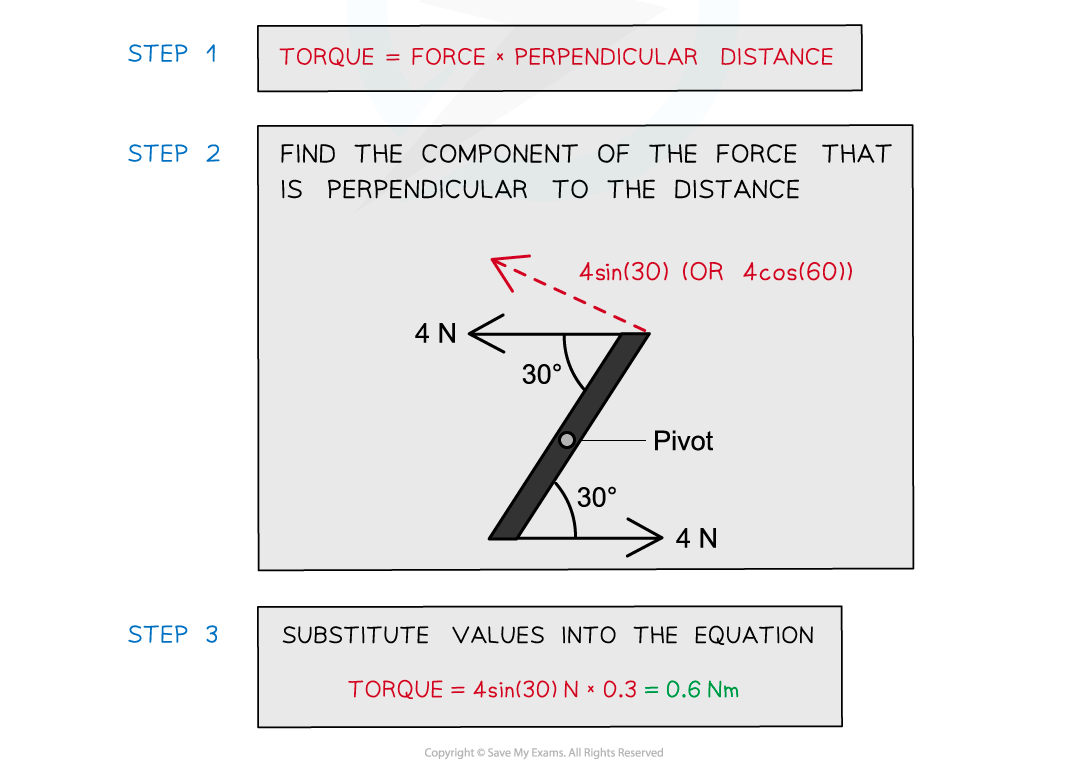
Determine the magnitude of the torque of the couple on the ruler.
Answer:
Worked Example
The forces acting on a bicycle pedal at different positions during a ride are shown in the diagram below.
The distance from the pedal to the axis of rotation is 24 cm.
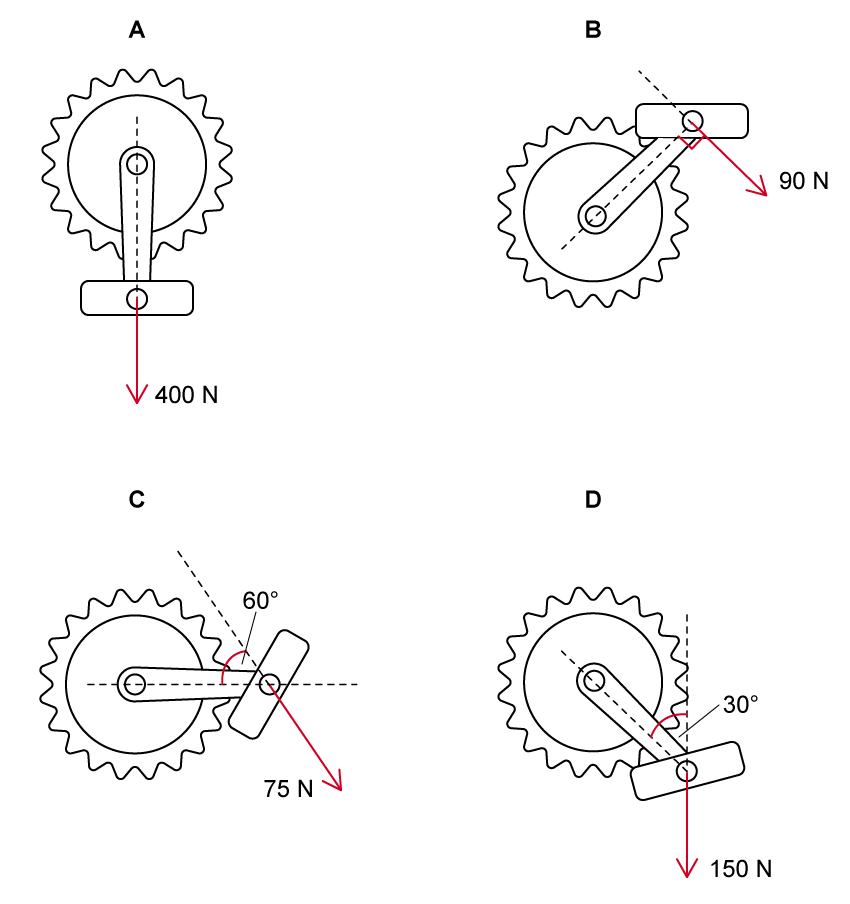
At which position is the magnitude of the torque the greatest?
Answer: B
In position A: When θ = 180°, sin θ = 0, so τ = 0 i.e. the force exerts no torque on the pedal. When the pedal is at the bottom, no amount of pushing down will produce any torque on the pedal.
In position B: When the angle θ is 90° and the force F is 90 N, the torque τ = 0.24 × 90 × sin 90° = 21.6 N m
In position C: When the angle θ is 60° and the force F is 75 N, the torque τ = 0.24 × 75 × sin 60° = 15.6 N m
In position D: When the angle θ is 30° and the force F is 150 N, the torque τ = 0.24 × 150 × sin 30° = 18.0 N m
Examiner Tips and Tricks
The terminology in this section can get confusing. For example, a moment is not a 'turning force' - the turning force is only part of the moment, the moment is the effect that the turning force has on the system when applied at a distance from a turning point, or pivot.
Also, torque is another term for 'moment', but in mechanics, torque can be used to describe a couple as a specific case of a moment.
Ultimately, when you carry out calculations, make sure you can identify
the magnitude of the applied force
the perpendicular distance between the force and the turning point (along the line of action)
Make sure you understand that the forces that make up a couple cannot share the same line of action (the line through the point at which the force is applied).

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
