Revision NotesExam QuestionsFlashcardsPast PapersMock Exams
IBPhysicsDPHLRevision NotesSpace, Time & MotionRigid Body MechanicsMoment of Inertia

Moment of Inertia (DP IB Physics) : Revision Note

Katie M

Author

Katie M

Last updated

Moment of Inertia

  • In linear motion, the resistance to a change of motion, i.e. linear acceleration, is known as inertia

    • The larger the mass an object has, the greater its inertia

  • In rotational motion, the distribution of mass around an axis must be considered, using moments of inertia

  • The moment of inertia of a rigid, extended body is defined as:

The resistance to a change of rotational motion, depending on the distribution of mass around a chosen axis of rotation

  • Moment of inertia is measured in kg m2

  • The moment of inertia of a body corresponds to how 'easy' or 'hard' it is to rotate, and this is dependent on many factors, including

    • its shape

    • its density

    • its orientation (relative to an axis of rotation)

  • These factors allow an object's distribution of mass to be taken into account 

    • It also means that the moment of inertia of a singular object can change depending on its orientation in relation to the chosen axis of rotation

  • For example, the moment of inertia of a thin rod is different for each of the following orientations:

    • Rotation about its vertical axis

    • Rotation about its centre of mass

    • Rotation about one end

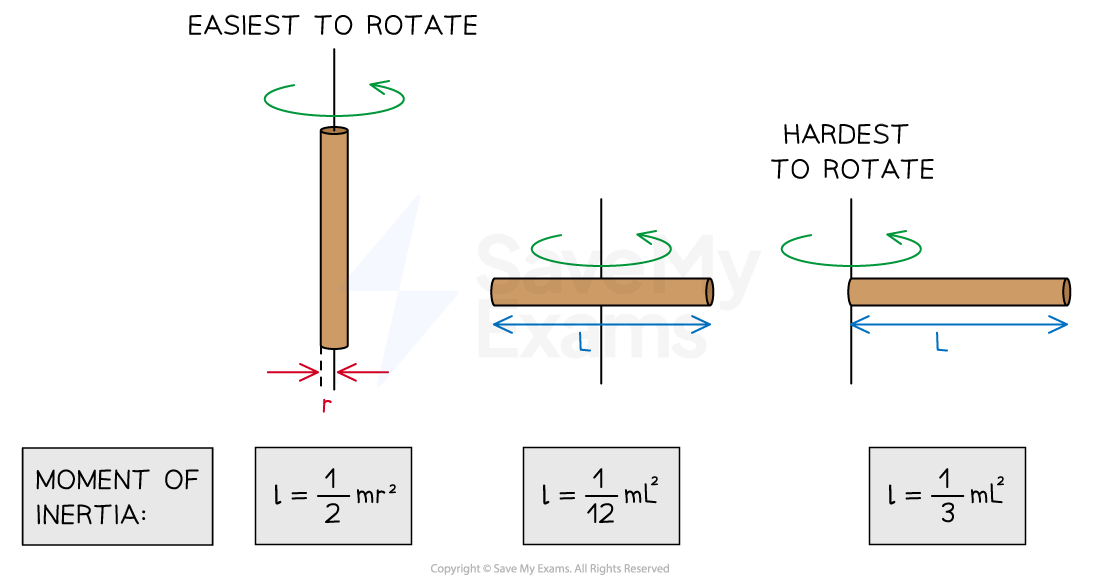
5vz_Kx4c_1-4-5-moment-of-inertia-different-orientations

The moment of inertia of a body can change depending on its orientation relative to the axis of rotation 

  • These are just a few of the possible orientations of the axis of rotation for a thin rod

    • There is an infinite range of possible axes, and therefore moments of inertia

    • This also applies to nearly all rigid, extended objects that could be considered

Examiner Tips and Tricks

Make sure you are clear on the distinction between linear motion and rotational motion here. The implications of considering the distribution of masses in relation to an axis of rotation, as opposed to considering them as uniform, have important consequences when carrying out calculations

Calculating Moments of Inertia

  • The moment of inertia I of a point mass is equal to

I space equals space m r squared

  • Where:

    • I = moment of inertia (kg m2)

    • m = mass of the object (kg)

    • r = distance from its axis of rotation (m)

  • The total moment of inertia of a system can be calculated using:

I subscript t o t end subscript space equals space sum m r squared

  • This means that the sum of the moments of inertia of all the point masses in the system gives the total moment of inertia of the system

  • Some moments of inertia of common shapes are shown below:

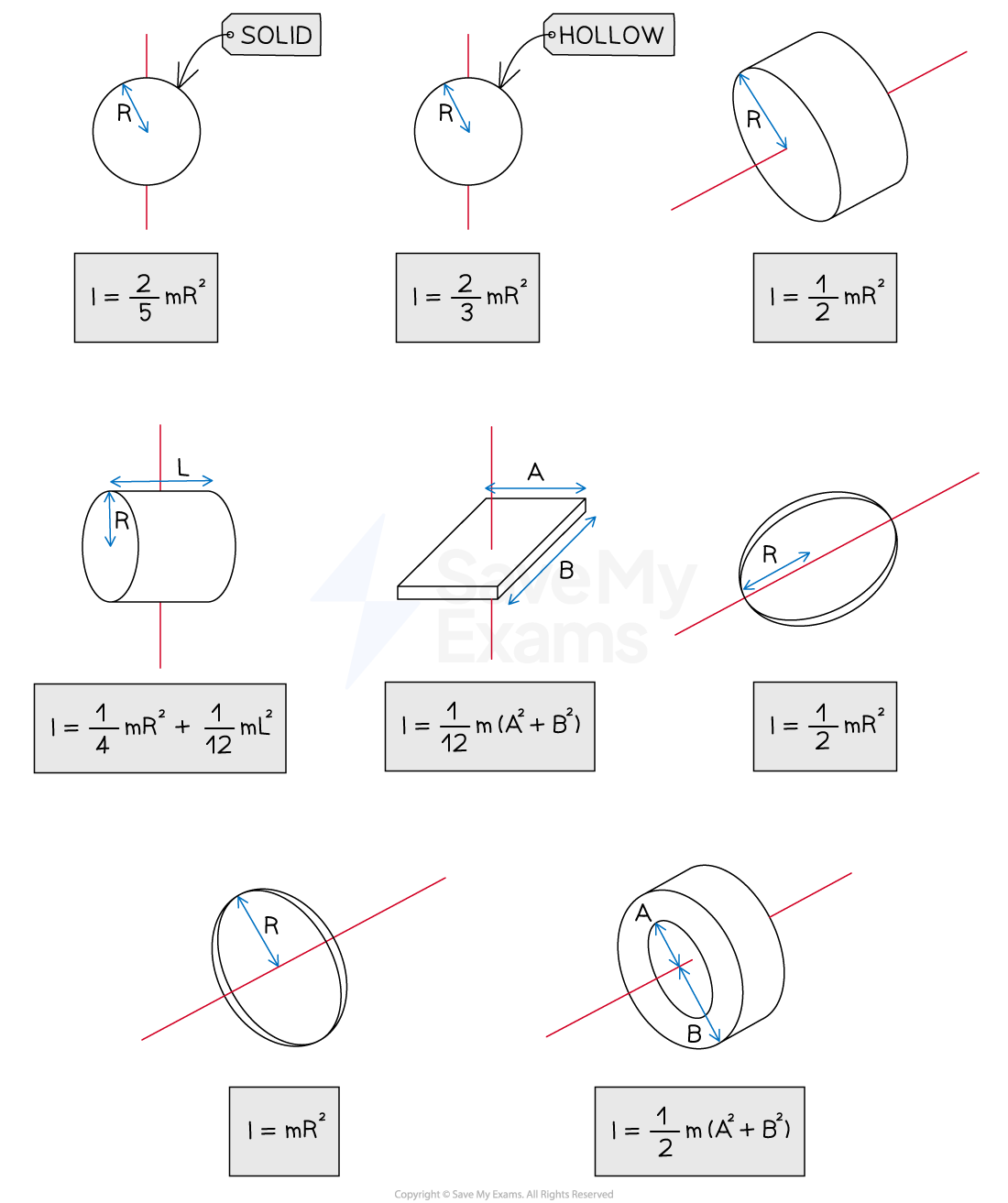
1-4-5-moments-of-inertia-for-different-shapes-1

Moments of inertia of common shapes, where R represents radius and L represents length, as shown

Worked Example

Two solid spheres form a dumbbell when attached to each end of a thin rod. The dumbbell rotates with the centre of mass of each sphere at a distance of 22 cm from the axis of rotation, as shown in the diagram.

The thin rod has a mass of 150 g. Each sphere has a radius of 4 cm and a moment of inertia of 0.04 kg m2 relative to the axis of rotation.

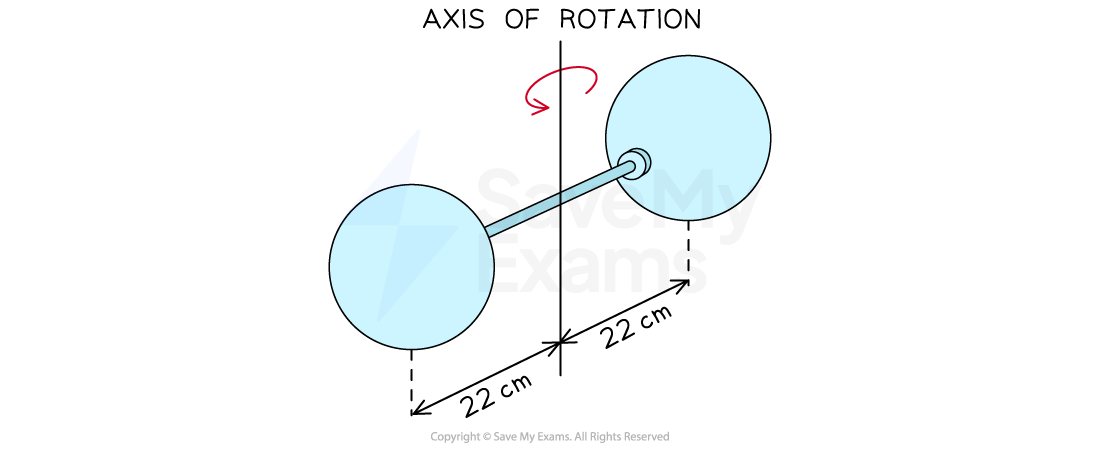
1-4-5-moments-of-inertia-dumbbell-arrangement-ib-2025-physics

The moment of inertia of a thin rod about its centre is 1 over 12 m L squared.

(a) Calculate the total moment of inertia of the dumbbell arrangement.

(b) Determine the proportion of the moment of inertia that the thin rod contributes to the dumbbell arrangement.

Answer:

Part (a)

Step 1: Calculate the moment of inertia of the rod

  • The mass of the rod is m space equals space 150 space straight g space equals space 0.15 space kg

  • The length of the rod is L space equals space 2 cross times open parentheses 22 minus 4 close parentheses space equals space 36 space cm space equals space 0.36 space straight m

I subscript r o d end subscript space equals space 1 over 12 m L squared

I subscript r o d end subscript space equals space 1 over 12 open parentheses 0.15 close parentheses open parentheses 0.36 close parentheses squared space equals space 1.62 cross times 10 to the power of negative 3 end exponent space kg space straight m squared

Step 2: Calculate the overall moment of inertia of the system

  • The overall moment of inertia of the dumbbell is the sum of all the moments of inertia in the arrangement

I subscript t o t end subscript space equals space sum m r squared

I subscript t o t end subscript space equals space 2 I subscript s p h e r e end subscript space plus space I subscript r o d end subscript

  • Where I subscript s p h e r e end subscript space equals space 0.04 space kg space straight m squared

I subscript t o t end subscript space equals space 2 open parentheses 0.04 close parentheses space plus space open parentheses 1.62 cross times 10 to the power of negative 3 end exponent close parentheses space equals space 0.0816 space kg times straight m squared

Part (b)

  • The proportion of the moment of inertia contributed by the thin rod is:

I subscript r o d end subscript over I subscript t o t end subscript space equals space fraction numerator 1.62 cross times 10 to the power of negative 3 end exponent over denominator 0.0816 end fraction space equals space 0.0198 space almost equal to space 2 percent sign

  • This means the rod contributes about 2% to the overall moment of inertia of the dumbbell

Examiner Tips and Tricks

You will never be expected to memorise the moments of inertia of different shapes, they will always be given in an exam question where required

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourselfFlashcards

    Did this page help you?

    Previous:Angular Acceleration FormulaNext:Newton’s Second Law for Rotation
    Katie M

    Author: Katie M

    Expertise: Physics Content Creator

    Katie has always been passionate about the sciences, and completed a degree in Astrophysics at Sheffield University. She decided that she wanted to inspire other young people, so moved to Bristol to complete a PGCE in Secondary Science. She particularly loves creating fun and absorbing materials to help students achieve their exam potential.